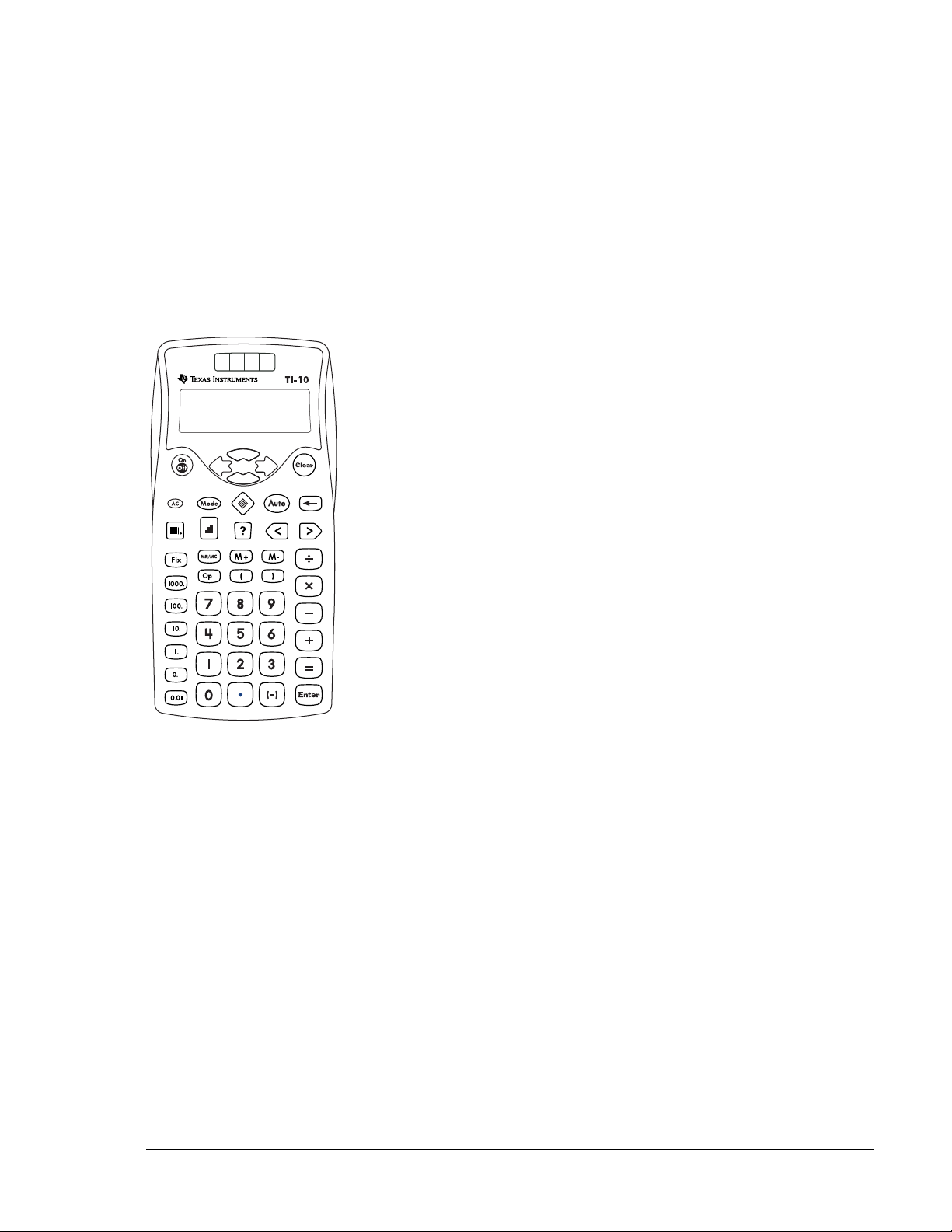
Texas instruments TI-10 A Guide for Teachers

TI.10:

A Guide for Teachers

Developed by

Texas Instruments Incorporated

Activities developed by

Dr. Jane Schielack

About the Author

Dr. Jane Schielack is an Associate Professor of Mathematics Education in the Department of Mathematics at Texas A&M University. She developed the examples in the

How to Use the TI-10

Activities

section of this guide.

section and assisted in evaluating the appropriateness of the

Important Notice Regarding Book Materials

Texas Instruments makes no warranty, either expressed or implied, including but not limited to any implied warranties of merchantability and fitness for a particular purpose, regarding any programs or book materials and makes such materials available special, collateral, incidental, or consequential damages in connection with or arising out of the purchase or use of these materials, and the sole and exclusive liability of Texas Instruments, regardless of the form of action, shall not exceed the purchase price of this book. Moreover, Texas Instruments shall not be liable for any claim of any kind whatsoever against the use of these materials by any other party.

Note

: Using calculators other than the TIN10 may produce results different from those described in these

materials.

solely

on an “as-is” basis. In no event shall Texas Instruments be liable to anyone for

Permission to Reprint or Photocopy

Permission is hereby granted to teachers to reprint or photocopy in classroom, workshop, or seminar quantities the pages or sheets in this book that carry a Texas Instruments copyright notice. These pages are designed to be reproduced by teachers for use in classes, workshops, or seminars, provided each copy made shows the copyright notice. Such copies may not be sold, and further distribution is expressly prohibited. Except as authorized above, prior written permission must be obtained from Texas Instruments Incorporated to reproduce or transmit this work or portions thereof in any other form or by any other electronic or mechanical means, including any information storage or retrieval system, unless expressly permitted by federal copyright law.

If you request photocopies of all or portions of this book from others, you must include this page (with the

permission statement above) to the supplier of the photocopying services.

Send inquiries to this address:

Texas Instruments Incorporated 7800 Banner Drive, M/S 3918 Dallas, TX 75251 Attention: Manager, Business Services

education.ti.com

ti-cares@ti.com

Except for the specific rights granted herein, all rights are reserved.

Printed in the United States of America.

Automatic Power Down, APD, and EOS are trademarks of Texas Instruments Incorporated.

About the Teacher Guide

p

pprop

p

pprop

p



How the Teacher Guide Is Organized

This guide consists of two sections: and

How to Use the TI-10

section is a collection of activities for integrating the TI-10 into mathematics instruction. The is designed to help you teach students how to use the TI-10 features.

•

Activities

The activities are designed to be teacherdirected. They are intended to hel develop mathematical concepts while

orating the TI-10 as a teaching tool.

incor Each activity is self-contained and includes the following:

An overview of the mathematical

−

purpose of the activity.

The mathematical conce

−

developed.

The materials needed to

−

activity.

A student activity sheet.

−

How To Use the TI-10

Section

. The

Activities

section

ts being

erform the

Things to Keep in Mind

While many of the exam

•

trans develo were not designed s purpose.

For maximum flexibility, each exam

•

and activity is inde others. Select the trans master for the key your students need to use to develo conce an a mathematical conce teaching.

If an exam

•

a grade level, use it to teach the function of the key(s), and then provide relevant examples of your own.

To ensure that students start at the

•

same clear the TI-10, and then clear the display.

arency masters may be used to

mathematical concepts, they

the mathematical

ts you are teaching. Then select

riate activity for the

le does not seem

riate for your curriculum or

oint, have them press b to

les on the

ecifically for that

endent of the

arency

t you are

ress ” to

le

How to Use the TI.10

• •

This section contains examples on trans numbered and include:

−

arency masters. Chapters are

An introductory TI-10 keys the location of those keys on the TI-10 keyboard, and the functions of those keys.

The trans the introductory

ractical applications of the keys

of being discussed. The keys being discussed are shown in black on an illustration of the TI-10 keyboard.

EXAS INSTRUMENTS INCORPORATED

arency masters following

age describing the

resented in the examples,

ertinent notes about

age provide examples

Section

How to Order Additional Teacher Guides

To place an order or to request additional information about Texas Instruments (TI) products, contact us.

Phone:

E-mail:

Home page:

You may download and print your own copy of this Teacher Guide from this web page.

education.ti.com/guides

TI-10: A Guide for Teachers

1-800-TI-CARES

(1-800-842-2737)

ti-cares@ti.com

education.ti.com

iv

About the TI.10

p

pp

p

Two-Line Display

The first line displays an entry of up to 11 characters. Entries begin on the to the entry does not fit on the first line, it

s to the second line. When space

wra

ermits, both the entry and the result

appear on the first line.

The second line dis If the entry is too long to fit on the first line, it wra result do not fit on the first line, the result is dis Results longer than 10 digits are dis scientific notation.

If an entry does not fit on two lines, it continues to wra the last two lines of the entry. You can view the beginning of the entry by scrolling u this case, only the result a press

s to the second line. If both entry and

layed right-justified on the second line.

®

.

lays up to 11 characters.

, so that you always see

ears when you

left. If

layed in

. In

Display Indicators

Refer to Appendix B for a list of the display indicators.

Error Messages

Refer to Appendix D for a listing of the error messages.

Order of Operations

The TI-10 uses the Equation Operating System (EOSé) to evaluate ex

eration priorities are listed on the

o

Scrolling, Order of O

transparency master in the

Section.

TI-10

Because o

erformed first, you can use X and Y to change the order of o change the result.

erations inside parentheses are

erations, and Parentheses

erations and, therefore,

ressions. The

Display,

How to Use the

Menus

‡

The

• To move u

• To move the cursor and underline a

• To return to the

• To select a menu item,

• To return to the

Refer to Appendix C for more information about menus.

key displays menus.

or down through the

menus, press

menu item, press ! or ".

without selecting the menu item,

or

”

‡

while the item is underlined.

‡

or $.

#

revious screen

.

®

ress

revious screen, press

.

ress

Previous Entries (History)

After an expression is evaluated, use and $ to scroll through previous entries and results, which are stored in the TI-10 history.

#

Problem Solving (‹)

The Problem Solving tool has three features that students can use to challenge themselves with basic math o place value.

• Problem Solving Automatic mode

rovides a set of exercises to challenge the students’ skills in addition, subtraction, multiplication, and division.

Problem Solving Automatic mode lets

students select the level of difficulty (1, 2, or 3) and the ty

P

, or ?).

x,

• Problem Solving Manual mode lets

students com which may include missing elements or inequalities.

e of operation (+, –,

ose their own problems,

erations and

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

v

About the TI.10

(Continued)

• Problem Solving Place Value has three

options that let students:

− Find the place value of a given digit.

− Find how many ones, tens, hundreds,

thousands, tenths, or hundredths are in a given number.

− Find the digit in the ones, tens,

hundreds, thousands, tenths, or hundredths place of a given number.

Resetting the TI.10

You can reset the TI-10 in any of the following ways:

• Press

Y

When you reset the TI-10, displayed. Press

b

.

−

‡

(yes), and then press

”

and

simultaneously.

, select the

”

to clear the display.

MEM CLEARED

RESET

®

menu, select

.

Automatic Power DownTM (APDTM)

If the TI-10 remains inactive for about five minutes, Automatic Power Down (APD) turns it off automatically. To turn the TI-10 on again after APD, press −. The display,

pending operations, settings, and memory are retained.

is

Resetting the TI-10:

• Returns settings to their defaults:

− Standard notation (floating decimal)

− Problem Solving

− Quotient and Remainder results for

division

− Difficulty Level 1 (addition) in Problem

Solving tool

− Type of Operation — addition

• Clears:

− Pending operations

− Entries in history

− The constant (stored operation)

− Any error condition

AUTO

mode

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

vi

Activities

The Value of Place Value 2

What’s the Problem? 7

Comparing Costs 11

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

1

The Value of Place Value

Overview

Students will build their flexibility in using numbers by exploring the connections between the number symbols and their representations with base-ten materials.

Introduction

1. Read

2. Divide students into several groups. Give each

3. Tell students you have run out of unit pieces and

4. Have students explore the answer to this

5. Have students compare their solutions with the

Counting On Frank

by Rod Clement. Discuss other kinds of questions that a person could ask about how many objects fit in or on other objects.

group a large pile of units (over 300) from the base-ten materials. Tell them that this is how many jelly beans fit into a jar that you filled. Ask them to count the “jelly beans.” Observe the techniques they use to count (counting one at a time, making groups of 10, etc.).

then ask:

How many rods (groups of 10) would I need to use to make a pile of “jelly beans” the same size as yours?

problem with their units, or they can apply their knowledge of place value. Then show them how to explore the answer using the TI-10.

base-ten materials to the TI-10 display. (They can make 31 tens rods from the 314 units, with 4 units left over.)

Math Concepts

Grades 2 - 4

whole number

•

place value (through thousands)

•

money

Grades 4 - 6

decimal place value

•

(through hundredths)

metric units

•

(meters, decimeters, centimeters)

³

To use the Place Value feature for this activity:

1. Press

2. Press

3. Press

4. Press

³

To explore answers to this problem on the TI-10:

1. If necessary, press

2. Enter 314, the number of

3. Press

‹ "

MAN

(manual) mode, if

necessary.

$

Place Value feature to

11 –.

This lets you find out how many ones, tens, hundreds, etc., there are in a number.

Note:

You can use the

1 –.

Place Value feature to find what digit is in the ones, tens, hundreds, etc., place.

‡ to return to the

entry screen.

enter the Problem Solving tool.

units.

Œ

many tens there are in

314. The display shows

31

, indicating there are

ê

31 tens in 314.

Materials

•

‡.

®

to select

®

to set the

’ to see how

TI-10

pencil

Counting on

by

Frank

Rod Clement

base-ten materials

student activity (pages 5 and 6)

–

‹ to

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

2

The Value of Place Value

(Continued)

Collecting and Organizing Data

Have students use their base-ten materials and TI-10s to continue exploring other numbers and identifying how many units, tens, hundreds, and thousands those numbers contain. Older students can find how many tenths and hundredths are in the numbers. Encourage exploration with questions such as:

How many hundreds are in 120? 2478? 3056?

•

How many tens are in 120? 2478? 3056?

•

How many units (ones) are in 120? 2478?

•

3056?

What numbers can you find that have 12 units?

•

12 tens? 12 hundreds?

What numbers can you find that have 60 units?

•

60 tens? 60 hundreds?

Analyzing Data and Drawing Conclusions

Have students use the tables on

Value

Student Activity pages to record their findings and identify the patterns they see. To help them focus on the patterns, ask questions such as:

The Value of Place

³

In ‹ (Problem Solving) (manual) mode, students can use the feature to test their conjectures. For example, if they think 1602 has 160 hundreds, they enter press They can then use base-ten materials to see why there are only 16 hundreds in 1602.

Note: – 1 –.

find what digit is in the hundreds place. They enter

1602

ê6êê

is the digit in the hundreds place.

11 –.

Place Value

Œ ‘

Students can use the

place value feature to

, press

. This result means that

, and see

Œ ‘

, and see

MAN

1602

16

,

êê

.

6

How does the number of tens in 1314 compare

•

to the number 1314? How about 567? 2457? 4089, etc.?

If you cover the digit in the units place, you see how many tens are in a number.

How does the number of hundreds in 1314

•

compare to the number 1314? How about 567? in 2457? in 4089, etc.?

If you cover the digits to the right of the hundreds place, you see how many hundreds are in a number.

How does the display on the TI-10 compare to

•

what you can do with the base-ten materials?

If the TI-10 displays

31_

, for 316, you should be

able to make 31 tens rods out of 316 units.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

3

The Value of Place Value

Continuing the Investigation

Connect the place-value patterns to money. For example, ask students:

If each one of your “jelly beans” costs a penny,

•

how many pennies would you spend for 1,314 jelly beans?

1,314 pennies

How many dimes (tens) would you spend?

•

131 dimes and 4 more pennies

How many dollars (hundreds)?

•

13 dollars, plus 14 more pennies, or 1 dime and 4 pennies

Older students can record the money and enter it into the TI-10 in decimal form, 13.14. Then, they can use the TI-10 to connect dimes to 1/10 (0.1) of a dollar ($13.14 has 131 dimes or tenths). They can also connect pennies to 1/100 (0.01) of a dollar ($13.14 has 1314 pennies or hundredths).

(Continued)

Older students can also connect the place-value patterns to conversions between metric units. For example, a measurement of 324 centimeters can also be recorded as 32.4 decimeters (or rounded to 32 dm) because 1 dm = 10 cm, or it can be recorded as 3.25 meters (or rounded to 3 m) because 1 m = 100 cm.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

4

The Value of Place

Name _____________________________

Date _____________________________

Value, Part A

Collecting and Organizing Data

1. Use your base-ten materials and your TI-10 to explore how many tens, hundreds, and thousands are in a number. Record your observations in the table.

Number

Number of

Thousands

2. What patterns do you see?

Number of

Hundreds

Number of

Tens

Analyzing Data and Drawing Conclusions: Patterns

1. Write 5 numbers that have 15 tens.

__________________________________________________________________________________

2. Write 5 numbers that have 32 hundreds.

__________________________________________________________________________________

3. Write 5 numbers that have 120 tens.

__________________________________________________________________________________

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

5

The Value of Place

Name _____________________________

Date _____________________________

Value, Part B

Collecting and Organizing Data

1. Use your base-ten materials and your TI-10 to explore how many tenths and hundredths are in a number. Record your observations in the table. What patterns do you see?

Number Number of

Tenths

2. What patterns do you see?

Number of

Hundredths

Analyzing Data and Drawing Conclusions: Patterns

1. Write 5 numbers that have 15 tenths.

__________________________________________________________________________________

2. Write 5 numbers that have 32 hundredths.

__________________________________________________________________________________

3. Write 5 numbers that have 120 tenths.

__________________________________________________________________________________

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

6

What’s the Problem?

p

Overview

Students will connect number sentences to problem situations and use addition, subtraction, multiplication, and division to solve the problems.

Introduction

1. On a sentence strip or on the overhead, display a number sentence, such as 8 + 2 = ?. Have students brainstorm situations and related questions that this number sentence could represent and then ask:

If I bought eight postcards on my vacation, and I had two postcards already at home, how many postcards do I have now?

2. If necessary, have students act out the situation with counters and determine that the value of ? is

10.

3. Demonstrate how to display this equation on the TI-10 and how to tell the TI-10 what the value of ? is.

Math Concepts

Grades 1 - 5

addition,

•

subtraction

number sentences

•

(equations)

Grades 3 - 5

lication,

multi

•

division

inequalities

•

³

To display

1. Press put the TI-10 in Problem Solving mode.

2. Press menu.

3. Press The TI-10 displays (one whole number solution).

4. Enter 10 and press to test the solution. The TI-10 displays the equation and

8 + 2 = ?

‹

‡ "

MAN

‡ to exit the

8 T 2 Z

Materials

TI-10

•

counters

•

pencil

•

student

•

activity (page 10)

:

®

(manual)

 ®

1 SOL

®

YES

.

to

.

³

4. Now display an equation such as ? - 10 = 4. Have students brainstorm situations and related questions that this number sentence could represent.

I had some money in my pocket, and I spent 10 cents of it. I only have 4 cents left. How much money did I have in my pocket to begin with?

Have students practice the keystrokes necessary to display this equation and test the value they

If an incorrect value is tested

no

,

, shows

for ?, the TI-10 displays and provides a hint. For example, if a student tests 15 for the equation the TI-10 displays

15 - 10 > 4

to the original equation.

Note:

After three incorrect answers, the TI-10 displays the correct answer.

? - 10 = 4

, and then returns

determine for ?.

5. Over a period of time, continue to introduce students to different types of number sentences to explore. For example, ? x ? = 24 (which has 8 solutions of whole number factor pairs) and ? x 4 = 2 (which has no whole number solution).

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

7

What’s the Problem?

Collecting and Organizing Data

As an ongoing activity, have students work in pairs and use the page to create problem-solving cards. One partner is responsible for the calculations on the TI-10, and the other partner is responsible for writing number sentences.

1. Have the partner responsible for writing number sentences create an addition, subtraction, multiplication, or division number sentence, using the ?, and then, record the number sentence in the top box on Page 10.

2. Have the partner responsible for the TI-10 enter the number sentence into the calculator.

3. Have the partner responsible for writing number sentences create a situation and question to go with the number sentence and then record this information in the bottom box.

What’s the Problem?

Student Activity

(Continued)

The partners can work together to glue or tape the two boxes to opposite sides of an index card.

Have students work together using the TI-10 to explore how many whole number solutions the equation has and test those solutions. Provide ideas for exploration by asking questions such as:

What actions could be happening in your story

•

to go with addition (subtraction, multiplication, or division)?

How could you use the counters to act out this

•

number sentence?

What could this number in the number sentence

•

represent in your story?

What could the question mark in the number

•

sentence represent in your story?

Can you make a story for a number sentence

•

that begins with a question mark?

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

8

What’s the Problem?

(Continued)

Analyzing Data and Drawing Conclusions

To help student focus on thinking about the relationships between their stories and the numbers and operations in their number sentences, ask questions such as:

How would using a different number here

•

change your story?

How would using a greater than or less than

•

symbol instead of an equal sign in the number sentence change your story?

How would using a different operation in your

•

number sentence change your story?

Continuing the Investigation

•

Have partners create stories and trade them. Each partner can then write a number sentence to go with the other partner’s story.

•

Have students sort their number sentences into categories. For example, those with no whole number solutions, those with 1 whole number solution, those with 2 whole number solutions, and those with infinite whole number solutions.

•

Have students try to find an equation or inequality with exactly no whole number solutions, exactly 1 whole number solution, exactly 2 whole number solutions, more than 5 whole number solutions, etc.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

9

Name _____________________________

What’s the Problem?

Date _____________________________

1. Write a number sentence using an operation and the ?.

2. Write a story that describes a situation and asks a question that can be represented by the number sentence.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

10

Comparing Costs

Overview

Students will solve a problem using division with an integer quotient and remainder, solve the same problem using division with the quotient in decimal form, and then compare the results.

Introduction

1. Introduce this problem.

The maintenance department has determined that it will cost $.40 per square yard to maintain the district’s soccer field each year. The soccer field is 80 yards wide and 110 yards long. The six schools that play on the field have decided to split the cost evenly. How much should each school contribute to the soccer field maintenance fund this year?

2. Have students use the TI-10 to solve this problem in two ways:

Find the integer quotient and remainder.

•

Find the quotient in decimal form.

•

Math Concepts

division

•

multiplication

•

fractions

•

decimals

•



The TI-10 default division setting ( integer quotient with a remainder.

QR

) displays an

Materials

TI-10

•

pencil

•

student

•

activity (page 14)

Collecting and Organizing Data

Students should record their procedures and results on the help them focus on their thinking, ask questions such as

•

Comparing Costs

Student Activity page. To

:

What did you enter into the TI-10 to solve the problem?

80

110

−

V

Z to determine the area of the

soccer field.

0.40

V

−

W

Z to find the total maintenance cost.

6

Z to find the cost for each school in

decimal form.



To display a quotient in decimal form:

1. Press TI-10.

2. Press ‡ " to underline

(decimal format).

Ù

3. Press decimal setting.

4. Press ‡ to exit the

”

b

®

to select the

to reset the

P (division) menu and

return to the previous screen.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

11

Comparing Costs

Could you have solved the problem more

•

efficiently? How?

80 x 110 can be calculated mentally, and the key presses can be simplified to

How are your procedures alike for each type of

•

solution?

The procedures all involve finding how many square yards in the soccer field; they all involve multiplication and division.

How are they different?

•

You use different keys to tell the TI-10 in what form you want the answer displayed.

(Continued)

8800

V

.4

W

6

Z.

Analyzing Data and Drawing Conclusions

To guide students in the analysis of their data, ask questions such as:

How are your solutions in the two forms alike?

•

The solutions all have a whole number component of 586.

How are your two solutions different?

•

The remainder form just tells how many dollars are left over. The decimal form tells how much more than $586 each school has to pay.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

12

Comparing Costs

What happens if you multiply each solution by

•

6 to check it?

For the quotient and remainder form, when you multiply 586 by 6, you get 3516, so you must then add the remainder, 4, to get the total cost of $3520.

If you multiply the decimal result 586.6666667 by

586.6666667

6 ( this result does not make sense because 6 x 7 does not end in a 0!

Because we are referring to money calculations, you can fix the decimal result, 586.6666667, to 2 decimal places ( you multiply the rounded number 586.67 by 6

586.67

(

V 6 Z), you get 3520.00. This result still

does not make sense because 6 x 7 = 42.

If you reset and clear the TI-10, and then multiply

586.67 by 6 (

3520.02. This calculation does make sense.

As a school, which form of the quotient would

•

you want to use?

Responses may vary. Some students may want to use the decimal form, since it is the closest to the representation of money. Some students may want to use the integer quotient and remainder form and suggest that the Central Office pay the $4.00 remainder.

V 6 Z), you get 3520. However,

586.6666667

b ”

586.67

Š ™

V 6 Z), you get

(Continued)

®

). When



For an integer division (with quotient and remainder), the TI-10 uses only the quotient of the result in subsequent calculations. The remainder is always droped.



The TI-10 only rounds the displayed result. The internally

not

stored value is When you fix 586.6666667 to 2 decimal places (586.67), and then multiply by 6, the TI-10

remembers

number (586.6666667), and uses it as the factor. But when you enter the rounded number, 586.67, the TI-10 uses the number you entered for the factor, calculating a product of 3520.02.

rounded.

the original

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

13

Name _____________________________

Comparing Costs

Date _____________________________

Collecting and Organizing Data

The Maintenance department has determined that it will cost $4.00 per square yard to maintain the district’s soccer field each year. The soccer field is 80 yards wide and 110 yards long. The 6 schools that play on the field have decided to split the cost evenly. How much should each school contribute to the soccer field maintenance fund this year?

1. Use division with an integer quotient and remainder to find the answer.

2. Use division with a quotient in decimal form to find the answer.

Analyzing Data and Drawing Conclusions

Write a short paragraph comparing the two solutions.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

14

How to Use

the TI.10

1 Display, Scrolling, Order of

Operations, and Parentheses 16

2 Clearing and Correcting 19

3 Menus 23

4 Basic Operations 26

5 Constant Operation 33

6 Whole Numbers and Decimals 38

7 Memory 42

8 Problem Solving: Auto Mode 47

9 Problem Solving: Manual Mode 54

10 Place Value 62

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

15

Display, Scrolling, Order of

p

j

p

Operations, and Parentheses

1

Keys

1.

2.

3.

o

X

You can have as many as eight parentheses at one time.

Y

!

right.

#

down through entries/results and menus.

ens a parenthetical expression.

closes a parenthetical expression.

and " move the cursor left and

and $ move the cursor up and

revious

Notes

The examples on the transparency

•

masters assume all default settings. Press b the TI-10 to the default settings and clear the display.

The EOSTM transparency master

•

shows the order in which the TI-10 completes calculations.

When using parentheses, if you

•

ress Z before pressing Y,

Syn Error is dis return to the previous display.

Operations inside parentheses are

•

erformed first. Use X and Y to change the order of o and, therefore, change the result.

layed. Press ” to

to reset

”

erations

Example: 1 + 2 x 3 = 7

3

(1 + 2) x 3 = 9

The first and second lines display

•

entries u begin on the left and scroll to the right. An entry always wra

1

2

the operator.

Results are displayed right-

•

ustified. If a complete problem does not fit on the first line, the result displays on the second line.

If an entry does not fit on two

•

lines, it continues to wra view the beginning of the entry by using

to 11 characters. Entries

to scroll.

#

s at

. You can

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

16

Equation Operating System (EOS™)

q

p

Priority Functions

1 (first) Expressions inside

parentheses ( ).

Functions that re

uire a

2

closing parenthesis ) and

recede the argument.

3 Negation (N).

4 Multiplication, implied

multiplication, division (

Q P

5 Addition and subtraction (

6 (last)

completes all operations.

Z

).

+

N

).

Because operations inside parentheses are

erformed first, you can use parentheses to

change the order of operations and,

therefore, change the result.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

17

Order of Operations

(

1 + 2 x 3 =

Press Display

b”

á

1

T

2

V

3

1Û2Ý3

Z

Add

T

Multiply

V

Parentheses

Ú

7

X Y

1 + 2) x 3 =

Press Display

X V

1 3

T

Z

2

Y

Å1Û2ÆÝ3Ú

9

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

18

Clearing and Correcting

p

2

Keys

1.

2.

turns the TI-10 on and off.

−

clears the last digit you entered,

w

allowing you to correct an entry without re-entering the entire number.

3. In the Calculator tool, last entry, all

ending operations,

clears the

”

and any error conditions. You can then enter a new

roblem and

continue your calculation.

4.

resets the TI-10.

b

Notes

The examples on the transparency

•

masters assume all default settings. Press b

to reset

”

the TI-10 and clear the display.

You can reset the TI-10 in any of

•

the following ways:

−

Press b.

−

Press − and ” simultaneously.

−

Press

, select the RESET

‡

menu, select Y (Yes), and then press

When you reset the TI-10, the

•

®

.

message MEM CLEARED is dis

layed. Press ” to clear the

display.

Resetting the TI-10 returns

•

settings to their defaults:

−

Standard notation (floating decimal)

−

Problem Solving Auto mode

1

4

3

−

Quotient and Remainder result

2

for division

−

Difficulty Level 1 (addition) in Problem Solving

−

Addition as the Ty

e of

Operation

Clears:

•

−

Pending operations

−

Entries in history

−

The constant (stored operation)

−

Any error condition

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

19

Clearing and Correcting

p

Notes (continued)

Pressing ” alone does not affect

•

the mode settings, memory, history, or constant.

In ‹ (Problem Solving)

•

(automatic) mode, you clear your answer from the display before you press

In ‹ (Problem Solving)

•

(manual) mode, clear your

®

or it lets you clear your answer

before you press

roblem before you press

ressing ” lets you

®

AUTO

ressing ” lets

®

.

MAN

.

2

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

20

Clearing entries

p

1. Enter 35 + 10.

2. Clear the entry and

o

eration.

ending

3. Enter 35 N 9.

4. Complete the calculation.

Press Display

b”

á

35

T

10

35Û10

á

”

á

Clear

”

35

Z

U

9

35Ü9

Ú

26

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

21

Correcting entry errors using

w

1. Enter 69 + 3.

2. Change the 9 to an 8.

3. Add 3.

4. Complete the calculation.

Press Display

b”

á

69

w w w

T

3

8

69Û3

á

68

á

Backspace

w

T

Z

3

68Û3

á

Ú

71

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

22

Menus

p

3

Keys

1.

2.

dis

‡

menus (See page 24).

‹ ‡

Solving tool menus (See page 25).

lays the Calculator tool

dis

lays the Problem

Notes

The examples on the transparency

•

masters assume all default settings. Press b the TI-10 and clear the display.

The Calculator tool is active when

•

you turn the TI-10 on. When the Calculator tool is active,

to access the Calculator

‡

menus. See Calculator menu options.

Press ‹ to access the Problem

•

Solving tool. When the Problem Solving tool is active, the

indicator shows on the to

‹

of the TI-10 dis Problem Solving tool is active,

ress

‡

Solving menus. See the Problem Solving menu options.

age 24 for all the

lay. While the

to access the Problem

to reset

”

ress

age 25 for all

line

EXAS INSTRUMENTS INCORPORATED

See Appendix C for a complete

•

menu map.

21

To select an option from any

•

menu, highlight the item you want and menu, press

Press

•

AUTO (automatic) and MAN (manual) modes in the Problem Solving tool.

Press c to change the level of

•

difficulty, while you’re in the Problem Solving reaching Level 3, the TI-10 cycles back to Level 1.

TI-10: A Guide for Teachers

ress

‡

to toggle between the

]

. To exit the

®

again.

AUTO

mode. After

23

Menus

Calculator Tool

Press Menu Option Explanation Example

(Continued)

3

b ‡

b

‡

b

‡

”

$

”

$$

P

(Division)

OP

(Constant Operation)

CLEAR OP1 Y

QR

Ù

+ 1

?

(Yes)

(No)

n

Displays division results as a quotient and remainder.

Displays division results as a decimal.

Shows the constant operation on the display.

Hides the constant operation.

Clears the operation and value stored in OP1.

Retains the value and operation stored in OP1.

2 r 1

2.25

1 x 5 15

15

Y

CLEAR OP1

Y

CLEAR OP1

n

b

”

‡

RESET

$$$

EXAS INSTRUMENTS INCORPORATED

(No)

n

(Yes)

Y

Does not reset the calculator.

Resets the calculator.

TI-10: A Guide for Teachers

n

Y

RESET

Y

RESET

24

Menus

(Continued)

Problem Solving Tool

Press Menu Option Explanation Example

3

b

”

(Mode)

AUTO

(automatic)

‹ ‡

MAN

(manual)

Problem Solving AUTO (automatic) Mode

Press Menu Option Explanation Example

b

”

‹ ‡

$

OR

ê ë ì

(Level of

Difficulty)

1 2 3

c

b

”

‹ ‡

$$

(Type of

Operation)

Û Ü Ý Þ Ã

Default. Presents problems with one element missing.

Lets you compose your own problems with missing elements.

After Level 3 the TI-10 cycles back to Level 1.

Add, subtract, multiply, divide, find the operation.

‹

5 + 2 = ?

‹

5 + ?= 7

‹

4 + ? = 10

‹

10 - ? = 4

Auto

Problem Solving MAN (manual) Mode

Display options for Problem Solving Place Value only.

Press Menu Option Explanation Example

b

”

‹ ] ‡

$

EXAS INSTRUMENTS INCORPORATED

å

(Place

Value)

11 -.

- 1 -.

Lets you find out how many ones, hundreds, thousands, tenths, or hundredths a number contains.

Lets you find out what digit of a number is in a specified place.

TI-10: A Guide for Teachers

Press 1234

Œ‘

‹ ƒ

1234 12_ _

Press 1234

Œ‘

‹ ƒ

1234 _2_ _

25

Basic Operations

pp

q

p

Keys

1.

2.

3.

4.

5.

6.

7. M lets you enter a negative number.

Notes

•

adds.

T

subtracts.

U

multiplies.

V

divides.

W

completes the operation.

Z

completes the entry.

®

The examples on the transparency masters assume all default settings. Press b

to reset the

”

TI-10 and clear the display.

There are two options for

•

displaying division results.

−

When the QR setting is selected, the result of division a

ears as quotient and remainder (__ r __). QR is the default setting.

−

When the decimal (

) setting is

Ù

selected, the result of division is displayed as a decimal.

If you use the result of division in a

•

subse

uent calculation, only the

uotient is used. The remainder is

dropped.

The maximum number of digits for

•

uotient and remainder is 5. The

uotient, remainder, and the r character together can total u 11 characters.

4

to

EXAS INSTRUMENTS INCORPORATED

When the QR setting is selected

•

for division all numbers must be positive whole numbers.

If you attempt to divide by 0, a

•

0 ERROR message is displayed.

P

T, U, V, W, ®

•

, and Z work

with the built-in constants.

4

The negative symbol (L) will appear

3

2

1

5

6

7

•

smaller than the minus sign(N) in the display.

TI-10: A Guide for Teachers

26

7

Basic operations

2 + 5 N 6 =

Press Display

b”

2 6

T

Z

5

U

á

2Û5Ü6

3 x 4 P 2 =

Add, Subtract

T U

Multiply, Divide

V W

Ú

1

Equals

Z

Press Display

b”

3

V

4

W

2

á

3Ý4Û 2

Z

EXAS INSTRUMENTS INCORPORATED

Ú

6½0

TI-10: A Guide for Teachers

2

Finding patterns in previous problems

p

Enter the problems. 1 + 1 = 1 + 2 = 1 + 3 = Review history (

revious problems)

to determine the pattern.

Press Display

b”

á

1

T

1

Z

1Û1

Ú

Add

T

History

# $

2

1

T

2

3

##

$

Z

1Û2

1Û3

1Û1 1Û2

1Û2 1Û3

Ú

Ú Ú

3

4

2 3

3 4

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

28

Entering negative numbers

p

The temperature in Utah was L3° C

A.M

at 6:00 tem

erature had risen 12° C. What

was the temperature at 10:00

Press Display

. By 10:00 A.M., the

A.M

b”

á

M

3

T

12

Z

3Û12

ä

Ú

9

Negative

M

.?

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

29

Division with remainders

p

Chris has 27 pieces of gum. He wants to share the

ieces evenly among himself and 5 friends. How many

ieces will each person get?

How many pieces will be left over?

Press Display

b”

á

27

W

6

Z

27

Ú

6

Þ

4½3

Divide

W

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

30

Division with decimal result

Set the division display option to decimal and divide 27 by 6.

Press Display

b ”

á

Ù Þ

‡ " ®

Â·

Þ

Ù Þ

‡

á

Divide

W

Ù

ê

27

W

6

Z

27

Ù Þ

Ú

6

Þ

4Ù5

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

31

Calculating equivalent units of time

Sara ran 2 kilometers in 450 seconds. Convert her time to minutes and seconds.

450 seconds = ? minutes

? seconds

Press Display

b”

á

Divide

W

450

Z

W

60

450Þ60

(7 minutes, 30 seconds)

Ú

7½30

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

32

Constant Operation

p

j

p

pp

p

q

p

Keys

1.

Notes

•

lets you store an o

›

(+ N Q P) and a number as a constant for repeated use.

The examples on the transparency masters assume all default settings. Press b clear the display.

The constant operation is set in con

unction with

erform a calculation that uses the

T, U, V

to reset the TI-10 and

”

›

, or W key.

erator

when you

The constant operation works with

•

whole numbers and decimals.

When you use the constant

•

o

eration (›), a counter appears on the left side of the dis the result a side of the dis shows how many times the constant has been re number at the right exceeds 6 digits, the counter will not be shown. The counter returns to 0 after it reaches 99.

When you use division as the

•

o

eration for a constant, you can choose either division format setting (QR) or decimal ( only the subse remainder is dropped.

uent calculations; the

ears on the right

lay. The counter

uotient with a remainder

). For QR format,

.

uotient is used in

lay and

eated. If the

5

1

EXAS INSTRUMENTS INCORPORATED

To clear a stored constant

•

operation:

−

Press b to reset the TI-10. (Press previous screen.)

−

Press the CLEAR OP1 menu, select Y, and then

ress

previous screen.

Note:

does not clear the constant.

TI-10: A Guide for Teachers

to return to the

”

‡

Pressing

$ $

ress

®

to return to the

to scroll to

. Then

by itself

”

33

Addition as “counting on”

j

p

There are 4 frogs in a pond. If 3 more frogs

ump into the pond one at a time, how many frogs will be in the

Press Display

ond?

b”

á

Constant

operation

›

Add

T

› T

(store operation)

1

›

4

(initialize using 4)

›

(add 1, one at a time)

›

Op1

Û

1

Op1

4á

Op1

4Û1 15

Op1

5Û1 26

Op1

6Û1

37

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

34

Multiplication as “repeated addition”

p

Maria put new tile in her kitchen. She made 4 rows with 5 tiles in each row. Use re

eated addition to find how many tiles she used. Before you begin, set the TI-10 to hide the constant operation.

Press Display

b”

á

Constant

operation

›

Add

T

‡ $ " ®

(hide constant operation)

‡

(clear the menu)

› T

(store the constant

eration)

o

5

›

0

(initialize using 0)

(Continued)

Û1

á

Û

5

á

0

Op1

¼Á

Ã

ííí

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

35

Multiplication as “repeated addition”

Press Display

(Continued)

›

Op1

1 5

Op1

210

Op1

315

Op1

420

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

36

Clearing the constant operation

Before entering a new operation in OP1, you must clear the current values.

Press Display

b”

á

‡

$ $

Â·

êê

À»

ê

Op1

ê

Op1

ç

Ù

Þ

î

Mode menu

‡

®

(clear OP1)

‡

(exit mode menu)

EXAS INSTRUMENTS INCORPORATED

Op1

À»

ê

ç

á

¼ÁÏ

TI-10: A Guide for Teachers

37

Whole Numbers and Decimals

p

Keys

6

1.

2.

enters a decimal point.

r

sets the number of decimal

Š

laces in conjunction with the Place

Value keys.

3.

Š 

rounds results to the

nearest thousand.

4. Š

rounds results to the

‘

nearest hundred.

5. Š ’ rounds results to the

nearest ten.

6. Š “ rounds results to the

nearest one (unit).

7.

Š ˜

rounds results to the

nearest tenth.

8. Š

rounds results to the

™

nearest hundredth.

Notes

The examples on the transparency

•

masters assume all default settings. Press b

to reset

”

the TI-10 and clear the display.

The TI-10 automatically rounds the

•

result to the number of decimal

laces selected. Only the displayed value is rounded. The internally stored value is not rounded. The calculated value is

added with

trailing zeros if needed.

To remove the fixed decimal

•

setting, press Š r.

You must press Š before you

•

ress a Place Value key each time you want to change the number of places for rounding.

2

3

4

5

6

7

8

1

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

38

Whole Numbers and Decimals

p

6

Notes

•

(Continued)

All results are displayed to the fixed setting until you either clear the setting by the TI-10.

You can use r to enter a decimal with any number of regardless of the fixed decimal setting.

You can apply the Fix setting to an individual number that you entered, or to a result.

ressing

Š r

laces,

or reset

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

39

Setting the number of decimal places

p

Round 12.345 to the hundredths

lace, the tenths place, and the

tens

lace. Then cancel the Fix

setting.

Press Display

b”

á

12

r

345

12Ù345

Ú

®

12Ù345

Š ™

Fix

12Ù345Ú12Ù35

Fix decimal

Š

Hundredths

™

Tenths

˜

Tens

’

Š

˜

Š ’

Š r

(cancel Fix)

Fix

12Ù345

Fix

12Ù345

Ú

12Ù3

Ú

12Ù345

10

Ù

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

40

Addition with money

José bought ice cream for $3.50, cookies for $2.75, and a large soda for $.99. How much did he spend?

Press Display

b”

á

Š ™

r

3

r

2

r

99

50

75

Z

T

Fix

3Ù50Û2Ù75

ÛÙ

99

Ú

7Ù24

Fix decimal

Š

Hundredths

™

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

41

Memory

p

7

Keys

1.

adds the dis

`

memory.

2.

subtracts the dis

a

from the value in memory.

3.

|

recalls the contents of

memory to the dis

ressed twice, it clears the

memory.

layed result to the

layed result

lay. When

Notes

The examples on the transparency

•

masters assume all default settings. Press b ” to reset the TI-10 and clear the display.

You can store integers and

•

decimals in memory.

M is dis

•

layed anytime a value

other than 0 is in memory.

To clear the memory, press

•

twice.

You must press Z to complete an

•

o

eration before the result can be

entered into memory.

|

1

3

EXAS INSTRUMENTS INCORPORATED

2

TI-10: A Guide for Teachers

42

Using memory to add products

p

(

)

Hamburgers 2 $1.19 = Milk shakes 3 $1.25 = Cou

on for each

milk shake 3 $.20 =

Total cost =

Press Display

b ”

á

Fix

Š ™

á

2

V

1

r

19

Z

Fix

2Ý1Ù19

Ú

2Ù38

Add to Memory

`

Subtract from

Memory

a

Memory

Recall

|

`

(add hamburgers to memory)

3

V

1

r

25

Z

`

(add milk shakes to memory)

3

V r

(compute discount)

Continued

20

Z

Fix M

2Ý1Ù19

Fix M

3Ý1Ù25

Fix M

3Ý1Ù25

Fix M

3

ÝÙ

20

Ú

2Ù38

3Ù75

0Ù60

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

43

Using memory to add products

Press Display

(Continued)

a

(deduct coupon from memory)

|

(recall total cost)

|

(clear the memory)

Fix M

ÝÙ

3

20

Fix M

5Ù53

Fix

5Ù53

Ú

0Ù60

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

44

Using memory to find averages

p

Dai has test scores of 96 and 85. He has weekly scores of 87 and 98. Find the average for each grou

of scores and then find the average of his two averages together in decimal form.

Press Display

b”

á

Ù Þ

‡"®

Â·

Þ

Ù

Ü

Add to memory

`

Add

T

Memory recall

|

‡

85

2

W

`

87

96

Z

T

98

Z

96Û85

181Þ2

M

181Þ2

M

87Û98

Ù Þ

Ú

181

Ù Þ

90Ù5

Ù Þ

90Ù5

Ù Þ

185

(Continued)

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

45

Using memory to find averages

Press Display

(Continued)

W

T

W

2

Z

_ Z

2

Z

M

185Þ2

92Ù5Û90Ù5

183Þ2

Ú

M

183

M

Ú

Ù Þ

92Ù5

Ù Þ

Ú

Ù Þ

91Ù5

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

46

Problem Solving: Auto Mode

p

)

p

Keys

8

1.

activates the Problem Solving tool.

‹

In

AUTO

mode, Problem Solving dis exercises in addition, subtraction, multiplication, and division.

2.

lets you access the mode menu

‡

to change between Automatic (

AUTO

In

) and Manual (

AUTO

mode, you can access the

MAN

modes.

Level of Difficulty, and the Ty Operation menus.

Note:

Auto, Level 1 , and Addition are the default

settings.

3.c changes to the next level of difficulty. After Level 3, it cycles back to Level

e of

lays

4.

toggles between MAN and

]

AUTO modes in the Problem Solving tool.

Notes

The examples on the transparency

•

masters assume all default settings. Press b

to reset

”

the TI-10 and clear the display.

In AUTO mode (default), the TI-10

•

resents problems with one element missing (for exam 5+2=? or 5+?=7 or 5?2=7).

If the answer entered by the

•

student is not correct, the TI-10 dis

lays no and gives a hint

in the form of < or >.

le,

After three incorrect answers, the

•

TI-10 provides the correct answer.

After every fifth problem, the TI-10

•

dis

lays a Scoreboard that tallies

4

1

2

3

the correct and incorrect answers.

You can check progress at any

•

time by dis momentarily. Press

ressing

‡

, which

lays the Scoreboard

#

to review

previous problems.

In Problem Solving, you can view the

•

history, but you cannot edit.

To exit Problem Solving, press

•

again. The Scoreboard and

‹

revious

entries are cleared when you exit.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

47

Select level of difficulty

Choose the level of difficulty.

Press Display

b ”

á

‹ ‡

‹

жи ккккк

Auto

Problem Solving

‹

Mode

‡

$

®

"

‡

(to exit)

(Problems will vary from the example shown here.)

‹

1 2 3

ê

êëì

‹

1 2 3

êëì

‹

ÛÃÚ

8

808

Auto

ê

Auto

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

48

Select level of difficulty using

c

Choose the level of difficulty.

Press Display

b ”

á

‹

(default to Level 1)

‹

3Û20Ú á

Auto

Ã

Problem Solving

‹

Mode

‡

c

(change to Level 2)

‡

(view level of difficulty setting)

$

‡

(return to problem)

‹

2Û70Ú

Ã

á

‹

1 2 3

êëì

‹

2Û70

ÚÃ

á

Auto

ê

Auto

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

49

Select type of operation

p

Choose the type of operation:

– addition (+) – subtraction (N) – multi

lication (Q) – division (P) – find the operator (?)

Press Display

b ”

á

‹ ‡

‹

жи ккккк

Auto

Problem Solving

‹

Mode

‡

$

" ®

‡

(to exit)

‹

1 2 3

ê

‹

Û Ü Ý Þ Ã ê

‹

Û

Ü Ý Þ

‹

4Ü1Ú

Auto

êëì

Auto

Ü

Auto

Ã

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

50

Test your skills

Enter solutions to the problems that the TI-10 presents.

Press Display

b ”

á

‹ ‡

‡

(problem is displayed)

‹

жи ккккк

‹

8Û3Ú á

Auto

Ã

Problem solving

‹

Mode

‡

11

®

(next problem; problems are random)

4

®

(problem is displayed again)

5

®

‹

8Û3Ú11

À¹¾

‹

ÛÃÚ

2

7

á

‹

2Û4Ç7

»¼

‹

ÛÃÚ

2

7

á

‹

2Û5Ú7

Auto

EXAS INSTRUMENTS INCORPORATED

À¹¾

TI-10: A Guide for Teachers

51

View the Scoreboard

p

After every fifth problem, the TI-10 dis

lays a scoreboard that tallies your correct and incorrect solutions.

You can also dis

lay the Scoreboard momentarily at any time by pressing

Press Display

‹

(problem is displayed)

‡

‹

ХЫГЪЧ

.

Auto

Problem solving

‹

Mode

‡

(Scoreboard is displayed momentarily)

‡

(exit menu; return to

roblem)

‹

À¹¾ »¼

‹

æè êêêê

‹

ХЫГЪЧ

Auto

50

Auto

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

52

Find the operator

p

Change the type of operation to “find the o

erator” (?) and solve

the problems the TI-10 presents.

Press Display

b ”

á

Auto

‹ ‡

‹

À¹¾ »¼

Problem solving

‹

Mode

‡

$ $

" " " " ®

‡

V ®

00

Auto

‹

Û Ü Ý Þ Ã ê

Auto

‹

Û Ü Ý Þ Ã

Auto

‹

8Ã6Ú48

Auto

‹

8Ý6Ú48

À¹¾

ê

(Problems will vary from exam

le shown here.)

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

53

Problem Solving: Manual Mode

p

q

Keys

9

1.

activates the Problem Solving

‹

tool.

2.

lets you access the mode menu

‡

to change between Automatic (

AUTO

In

) and Manual (

mode, you can access the

MAN

Place Value menu.

Note: 11-.

3.



missing element in

is the default Place Value setting.

lets the student indicate a

MAN

mode.

4.

[, \

, and Z let the student test

inequalities and equalities

) modes.

(manual)

.

5.

toggles between MAN and

]

AUTO modes in the Problem Solving tool.

Notes

The examples on the transparency

•

masters assume all default settings. Press b

to reset

”

the TI-10 and clear the display.

In MAN mode, the student

•

composes his or her own problems.

In MAN mode, for all operations

•

exce

t inequalities, the TI-10

accepts only integers.

You can enter no more than 11

•

characters into the display.

You can enter a problem that has

•

one solution, multi

le solutions, or

no solution. For example:

1

2

EXAS INSTRUMENTS INCORPORATED

−

1 solution: 2+5=?, 2+?=7, 2?5=7

5

3

−

Multiple solutions: ?+?=1, ?+?=6

−

0 solutions: 3P2=? (There is no

ositive, whole

number solution.)

4

When a problem has no solution,

•

the TI-10 dis continues to until you press

Problem Solving Manual mode was

•

designed to deal with

lays no and

resent the problem

.

”

ositive whole numbers. In some cases, the e

uality/inequality feature allows

you to enter decimals.

TI-10: A Guide for Teachers

54

Problem Solving: Manual Mode

p

Notes (Continued)

Problems with two missing elements

•

may have multi has eight solutions.) These must be in the form of ?operator?=number.

You can check progress by pressing

•

to dis

‡

Press problems.

to review previous

#

le solutions. (?x?=24

roblems

lay the Scoreboard.

9

When you first press

•

dis

lay shows the Scoreboard for a

moment before showing the menu.

For tests of inequalities, when the

•

TI-10 returns a no, the correct response is displayed immediately.

In Problem Solving, you can view the

•

history, but you cannot edit.

To exit Problem Solving, press ‹.

•

‡

, the

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

55

Problems with one solution

q

p

Problems with one solution are

uations with one missing element

e (for exam

le 7+2=? or 7+?=9).

Enter a problem and find a solution.

Press Display

b ”

á

‹

‹ ‡"

æè

®

êêêê

Problem solving

‹

Mode

‡

Missing element



‡

5

U

3

 ®

2

®

5

T  Z

9

®

Z

‹

á

‹

5Ü3

‹

5Ü3Ú2

‹

5

ÚÃ

1

ÛÃÚ

1

¾¼º

À¹¾

9

¾¼º

(Continued)

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

56

7

Problems with one solution

Press Display

(Continued)

3

4

®

‹

5Û3Ç9

»¼

‹

5Û4Ú9

À¹¾

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

5

Problems with more than one solution

p

Problems with two missing elements may have more than one solution. Enter a

roblem, find the number of

solutions, and then find a solution.

Press Display

b ”

á

‹ ‡ " ®

‡

‹

æè

‹

á

êêêê

Problem solving

‹

Mode

‡

Missing element



 T  Z

3

®

2

®

1

®

(Try another solution, or

ress ” to enter a new roblem.)

EXAS INSTRUMENTS INCORPORATED

‹

ÃÛÃÚ

4

‹

ÛÃÚ

2

‹

ÛÏÚ

2

3

¾¼º

3

À¹¾

S

TI-10: A Guide for Teachers

58

Problems with no solution

p

The TI-10 is not designed to handle certain ty result in a

es of problems. These will

0 SOL

(no solution)

response from the TI-10.

Press Display

b ”

á

‹ ‡ " ®

‹

æè

êêêê

Problem solving

‹

Mode

‡

Missing element



‡

1

U

2

Z

 ®

”

‹

á

‹

1Ü2ÚÃ

0

‹

á

¾¼º

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

59

Less than, greater than, equal to

q

You can test inequalities and

ualities using the Problem Solving

e tool.

Press Display

b ”

á

‹‡ "

‹

æè

®

‡

‹

á

Problem solving

‹

Mode

‡

Equals

Z

êêêê

Greater Than

\

2

T

2

T

5

T

®

5

r

50

r

1

Z

®

4

[

\

®

1

10

‹

2Û1Ú1Û2

À¹¾

‹

5Û4Ç10

À¹¾

‹

Ù5ÈÙ

‹

Ù5ÚÙ

50

»¼

50

À¹¾

Less Than

[

S

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

60

View the Scoreboard

p

After every fifth problem, the TI-10 dis

lays a scoreboard that tallies your correct and incorrect solutions.

You can also dis

lay the Scoreboard momentarily at any time by pressing

Press Display

‹

(problem is displayed)

‡

‹

ХЫГЪЧ

.

Auto

Problem solving

‹

Mode

‡

(Scoreboard is displayed momentarily)

‡

(exit menu; return to

roblem)

‹

À¹¾ »¼

‹

æè êêêê

‹

ХЫГЪЧ

Auto

50

Auto

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

61

Place Value

j

Keys

10

1.

activates the Problem Solving

‹

tool.

2.

lets you access the mode menu

‡

to change between Automatic (

AUTO

In

) and Manual (

mode, you can access the

MAN

Place Value menu.

Note: 11-.

is the default Place Value setting.

) modes.

3.

activates the Place Value

Œ

feature when the TI-10 is in Problem Solving MAN mode. It also works in con

unction with these

keys:

Key Displays

 ‘ ’ “ ˜ ™

Number of thousands Number of hundreds Number of tens Number of ones Number of tenths Number of hundredths

Notes

The examples on the transparency

•

masters assume all default settings. Press b ” to reset the TI-10 and clear the display.

The Place Value feature works only

•

if you are in the Problem Solving MAN (manual) mode.

When the TI-10 Place Value feature

•

2

1

is active, the ƒ indicator shows on the top line of the display.

To exit Place Value, press ”. The

•

TI-10 returns to Problem Solving

3

MAN mode.

To exit Problem Solving completely,

•

press ‹.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

62

Place Value

What is the Place Value of a Given Digit?

(Continued)

10

The ‹ (Problem Solving) mode setting must be

Follow these steps (See example on page 65).

1. Press b ” to reset the TI-10 and clear the display.

2. Press 

3. Press

4. Press Œ.

5. Press the desired digit.

If you enter 6, the TI-10 will display ____._6 briefly showing the location of the 6 and then displaying its value, 6 –> 0.01, meaning that the 6 is in the hundredths place.

Once Place Value is active, it is not necessary to press Œ before each digit you want to test. To test a new number; however, you must press ”, enter the new number, and then press Œ again.

How Many Ones, Tens, Hundreds, Thousands, Tenths, or Hundredths are in a Given Number?

‡ " ®

to exit the menu and then enter 1234.56.

‡

to select Problem Solving

MAN

(manual).

mode.

MAN

The ‹ (Problem Solving) mode setting must be option must be set to

Follow these steps (See example on page 67).

1. Press b ” to reset the TI-10 and clear the display.

2. Press 

3. Press

4. Underline

5. Press

6. Press Œ.

7. Press “, ’,

If you press hundreds in the number. When you press meaning that there are 123,456 hundredths in the number.

‡ " ®

.

$

and press

11 –.

to exit the menu and then enter 1234.56.

‡

‘, , ˜

‘

11 –.

to select Problem Solving

.

®

, or

, the TI-10 will display 1 2 _ _ . _ _ , meaning that there are 12

™

.

, the TI-10 displays 1 2 3 4 5 6

™

(manual) and the display

MAN

mode.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

63

Place Value

When a number includes a repeated digit, the TI-10 first analyzes its occurrence in the right-most position. To find the place value of other instances of the same digit, press the digit twice for the second occurrence, three times for the third occurrence, etc. Each time you press the given digit in succession, the displays shifts to next occurrence to the left of the original occurrence. (See page 66 for an example.)

(Continued)

10

To exit Place Value, press

(manual) mode.

MAN

What Digit of a Number is in a Given Place?

The ‹ (Problem Solving) mode setting must be option set to

Follow these steps (See example on page 68).

1. Press b ” to reset the TI-10 and clear the display.

2. Press 

3. Press

4. Underline

5. Press

6. Press Œ.

7. Press “, ’,

If you press of the 2 is in the hundreds place. The answer then clears so you can press another digit or place value key.

– 1 –.

‡ " ®

.

$

and press

– 1 –.

‡

to exit the menu and then enter 1234.56.

‘, , ˜

‘

, the TI-10 displays _ 2 _ _ . _ _ briefly showing that the location

and the TI-10 returns to Problem Solving,

”

(manual) and the display

MAN

to select Problem Solving

®

.

™

, or

.

MAN

mode.

Press

to exit Place Value. The TI-10 returns to Problem Solving,

”

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

MAN

mode.

64

Determine place value

êêêê

Enter 1234.56. Determine the place value of 6 and 4.

Press Display

b ”

á

‹‡ "

‹

æè

®

‡

‹

á

Problem Solving

‹

Place Value

Œ

1234

Œ

6

4

r

56

‹

ƒ

1234Ù56

á

‹ ƒ

1234Ù56

ннннЩн

‹ ƒ

1234Ù56

ÜÈ0Ù

6

‹ ƒ

1234Ù56

ííí4Ùíí

‹ ƒ

1234Ù56

6

01

4

EXAS INSTRUMENTS INCORPORATED

ÜÈ

1

TI-10: A Guide for Teachers

65

Repeated digits

êêêê

Enter 123.43. Determine the place value of each 3.

Press Display

b ”

á

‹ ‡ "

‹

æè

®

‡

‹

á

Problem solving

‹

Place value

Œ

123

Œ

3

33

r

43

‹ ƒ

123Ù43

á

‹ ƒ

123Ù43

нннЩн

‹ ƒ

123Ù43

ÜÈ0Ù

3

‹ ƒ

123Ù43

íí3Ùíí

ƒ

‹

123Ù43

3

01

3

EXAS INSTRUMENTS INCORPORATED

ÜÈ

1

TI-10: A Guide for Teachers

66

How many?

êêêê

How many hundreds are in 1234.56? How many hundredths?

Press Display

b ”

á

‹ ‡"

‹

æè

®

‡

‹

á

Problem solving

‹

Place value

Œ

Hundreds

‘

Hundredths

™

1234

Œ

‘

™

r

56

‹ ƒ

1234Ù56

‹ ƒ

1234Ù56

ннЩнн

12

‹ ƒ

1234Ù56 123456

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

67

What’s the digit?

What digit is in the hundreds place in 1234.56?

Press Display

b ”

á

‹ ‡" ®

" ®

$

‹

æè

‹

ÜÙ ÜÏÜÙ

11

êêêê

кккккк

Problem solving

‹

Place value

Œ

‡

1234

Œ

‘

r

56

‹

á

‹

ƒ

1234Ù56

‹

ƒ

1234Ù56

н2ннЩнн

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

68

Quick Reference to Keys

Key Function

A

−

”

b

− ”

" !

# $

w

‡

Turns the calculator on. If already on, turns the calculator off.

Clears the display and error condition.

Resets the TI-10.

Resets the TI-10. Hold down − and ” simultaneously for a few

seconds and then release.

Resetting the TI-10 completely clears the calculator, including all

Note:

menu settings, all entries in history, all values in memory, and the display. All default settings are restored.

Moves the cursor right and left, respectively, so you can scroll the entry line or select a menu item.

Moves the cursor up and down, respectively, so you can see previous entries and results or access menu lists.

Deletes the character to the left of the cursor before

In the Calculator tool, displays menus that let you:

Select the format for results of division. Choose Quotient with Remainder (QR) or decimal (.).

MEM CLEARED

shows in the display.

shows on the display.

/Â·

êê

.

®

Þ

is pressed.

Ù

‡

$

‡

$ $

‡

$ $ $

Show (+1) or hide (?) the constant operator and operand in

Clear the constant operation (

Reset the TI-10.

EXAS INSTRUMENTS INCORPORATED

.

OP1

).

/Û1 Ã

êê

,

Àß ê

¼Á

/ç//î

//ß À// //ê ·¹Ó¹Ê

TI-10: A Guide for Teachers

A-1

Quick Reference to Keys

Key Function

A

‹

‹ ‡

$

$$

(

MAN

)

$

Toggles between the Calculator and Problem Solving tool.

In the Problem Solving tool, displays menus that let you:

Select (manual) mode.

Select Level of Difficulty.

(automatic) or

AUTO

MAN

‹

æè

‹

Auto

++1++2++3

êëì

Auto

ê

Auto

Select Type of Operation.

Select display options for Place Value feature. (This option is available only if

mode is selected.)

MAN

..ê

‹

+ЫЬЭЮГ

‹

ППЬЩО-- ÜÏÜÙ êêêê

]

c



[

\

Toggles between the

Moves to the next Level of Difficulty. After reaching Level 3, the TI-10 cycles back to Level 1.

Level 1: basic facts

Level 2: one digit numeral or two digit numeral with zeros in the

ones place

Level 3: up to three digit numerals

While in ‹ (Problem Solving)

missing element in an equation.

While in ‹ (Problem Solving)

inequalities.

While in ‹ (Problem Solving)

inequalities.

AUTO

and

MAN

mode in Problem Solving.

MAN

(manual) mode, lets you indicate a

(manual) mode, lets you test

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

A-2

Quick Reference to Keys

Key Function

A

Œ

d

Œ

Œ 

Œ ‘

Œ ’

Œ “

Œ ˜

Œ ™

Š

While in ‹ (Problem Solving)

the place value of a particular digit of a given number or, in conjunction with place value keys, you can determine how many thousands, hundreds, etc., a number contains or what digit is in a given place.

Determines the place value of the digit d (0 - 9) of a given number.

Tells how many thousands a given number contains or what digit is in the thousands place.

Tells how many hundreds a given number contains or what digit is in the hundreds place.

Tells how many tens a given number contains or what digit is in the tens place.

Tells how many ones a given number contains or what digit is in the ones place.

Tells how many tenths a given number contains or what digit is in the tenths place.

Tells how many hundredths a given number contains or what digit is in the hundredths place.

Sets the number of decimal places in conjunction with the Place Value keys. Only the displayed result is rounded; the internally stored value is not rounded. The calculated value is padded with trailing zeros if needed.

(manual) mode, you can determine

MAN

Š 

Š ‘

Š ’

Š “

Š ˜

Š ™

Š r

q h i j k l m n o p

T

U

V

Rounds results to the nearest thousand.

Rounds results to the nearest hundred.

Rounds results to the nearest ten.

Rounds results to the nearest one.

Rounds results to the nearest tenth.

Rounds results to the nearest hundredth.

Removes fixed decimal setting and returns to floating decimal.

Enters the numerals 0 through 9.

Adds. Enters the addition symbol in Problem Solving mode.

Subtracts. Enters the subtraction symbol in Problem Solving mode.

Multiplies. Enters the multiplication symbol in Problem Solving mode.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

A-3

Quick Reference to Keys

p

Key Function

A

W

Z

®

r

M

X

Y

`

a

|

Divides. Enters the division symbol in Problem Solving mode with the result displayed as a quotient and remainder or a decimal, as determined by the division menu setting.

Completes operations. While in ‹ (Problem Solving)

mode, lets you enter = to test equalities.

Tests a solution in the Problem Solving tool. Selects a menu item in either the Calculator or Problem Solving tool. Completes an operation.

Inserts a decimal point.

Enters a negative sign. Does not act as an operator.

Opens a parenthetical expression.

Closes a parenthetical expression.

Adds displayed result to the value in memory.

Subtracts displayed result from the value in memory.

Recalls the memory value for use in a calculation when pressed once. When pressed twice, clears memory.

MAN

(manual)

›

‡

$ $

®

Stores an operation with a constant value, which can be repeated by pressing the › key, as many times as desired. To store an operation

to

1. Press ›, enter the o

2. Press › to recall the stored operation.

To clear the contents of

®

and recall it:

OP1

erator and the value, and press › to save

the operation.

, press

OP1

. New operations can now be stored for repeated use.

‡

$ $

, underline Y, and press

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

A-4

Display Indicators

B

Indicator

‹

ƒ

Fix

M

Op1

Auto

Ù

P

y

z

w

x

Meaning

TI-10 is in the Problem Solving tool.

TI-10 is in the Place Value feature.

TI-10 is rounding to a specified place.

Indicates that a value other than zero is in memory.

An operator and a value is stored in

Indicates ‹ (Problem Solving) tool is in

Indicates that the result from the division feature will be displayed as a decimal.

Previous entries are stored in history, or more menus are available. Press menu lists.

Press

®

to access history. Press $ and # to access additional

#

!

and " to scroll and underline from a menu. You must press

to complete the selection.

OP1

.

AUTO

mode.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

B-1

Menu Map

The TI-10 has two tools—the Calculator tool and the Problem Solving tool.

Calculator Tool Problem Solving Tool

C

P

Division Format menu

OP

Constant Operation

menu

CLEAR OP1

Clear Constant Operation

menu

RESET

Reset menu

AUTO MAN

Mode menu

êëì

Level of Difficulty

menu

+ - x P ?

Type of Operation

menu

ƒ

Place Value

menu

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

C-1

Menu Map

(

p

(continued)

C

Calculator Tool

The calculator tool is active when you turn on the TI-10. When the Calculator tool is active, press

to access the Calculator tool menus. Below are the Calculator menu screens.

‡

P

Division Format menu

OP

Constant Operation menu

CLEAR OP1

Clear Constant Operation menu

RESET

Reset menu

Display division result as Quotient & Remainder format

Â·

êêê

Þ

Û1

êêê

¼Á

À»

ê

ç

ß À

ê

·¹¾¹Ê

¼ÁÏ

Ù

Ã

Display division result as Decimal format.

Show the line containing the constant operation (default).

Hide the line containing the constant o

Clear the constant operation

Yes. Clear the constant operation (default).

(default).

No. Do not clear the constant o

eration.

No. Do not reset the TI-10. (default).

Yes. Reset the TI-10 to default settings.

eration.

default).

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

C-2

Menu Map

Problem Solving

(continued)

C

The Problem Solving tool has two modes— choose menu. When you choose

Mode menu— Choose from two modes:

AUTO

•

(default) mode presents problems to solve.

MAN

lets you create problems to solve.

‹

1 2 3

ê

mode, you can access the Level of Difficulty menu and the Type of Operations

AUTO

mode, you can access the Place Value menu.

MAN

‹

AUTO

mode

жи ккккк

Auto

êëì

Level of Difficulty menu— Choose from three levels:

•

1 - easiest (default)

•

2

•

3 - hardest

(Automatic) and

AUTO

Auto

(Manual). When you

MAN

mode

‹

11

ÜÙ ÜÏÜÙ

ккккк

å

‹

Û Ü Ý Þ Ã

ê

Auto

EXAS INSTRUMENTS INCORPORATED

Type of Operation menu— Choose from five options:

•

Add (default)

•

Subtract

•

Multiple

•

Divide

•

Find the operator

TI-10: A Guide for Teachers

Place Value menu— Choose from two options:

•

How many ones, tens, etc. are in a number? (default)

•

What digit is in the ones, tens, etc. place?

C-3

Error Messages

Message Meaning

D

Arith Error

Syn Error

P

0 Error

Mem Error

Op Error

Overflow Error

Underflow Error

Arithmetical error. You entered an invalid entry or an invalid parameter.

Syntax error. You entered an invalid or incorrect equation; for example,

or

5++2

Divide by 0 error. You attempted to divide by 0.

Error in attempting to store an entry in memory.

Error following steps for using

Overflow. The result is too large to fit within the boundaries of the display.

Underflow. The result is too small to fit within the boundaries of the display.

(5+2

.

OP1

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

D-1

Support, Service, and Warranty

E

Product Support

Customers in the U.S., Canada, Puerto Rico, and the Virgin

Islands

For general questions, contact Texas Instruments Customer Support:

phone:

e-mail:

For technical questions, call the Programming Assistance Group of Customer Support:

phone:

1-800-TI-CARES (1-800-842-2737)

ti-cares@ti.com

1-972-917-8324

Customers outside the U.S., Canada, Puerto Rico, and the

Virgin Islands

Contact TI by e-mail or visit the TI Calculator home page on the World Wide Web.

e-mail:

Internet:

ti-cares@ti.com

education.ti.com

Product Service

Other TI Products and Services

Customers in the U.S. and Canada Only

Always contact Texas Instruments Customer Support before returning a product for service.

All Customers outside the U.S. and Canada

Refer to the leaflet enclosed with this product or contact your local Texas Instruments retailer/distributor.

Visit the TI calculator home page on the World Wide Web.

education.ti.com

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

E-1

Support, Service, and Warranty

(Continued)

E

Warranty Information

Customers in the U.S. and Canada Only

One-Year Limited Warranty for Commercial Electronic Product

This Texas Instruments electronic product warranty extends only to the original purchaser and user of the product.

Warranty Duration.

warranted to the original purchaser for a period of one (1) year from the original purchase date.

Warranty Coverage.

warranted against defective materials and construction.

WARRANTY IS VOID IF THE PRODUCT HAS BEEN DAMAGED BY ACCIDENT OR UNREASONABLE USE, NEGLECT, IMPROPER SERVICE, OR OTHER CAUSES NOT ARISING OUT OF DEFECTS IN MATERIALS OR CONSTRUCTION.

Warranty Disclaimers. ANY IMPLIED WARRANTIES ARISING OUT OF THIS SALE, INCLUDING BUT NOT LIMITED TO THE IMPLIED WARRANTIES OF MERCHANTABILITY AND FITNESS FOR A PARTICULAR PURPOSE, ARE LIMITED IN DURATION TO THE ABOVE ONE-YEAR PERIOD. TEXAS INSTRUMENTS SHALL NOT BE LIABLE FOR LOSS OF USE OF THE PRODUCT OR OTHER INCIDENTAL OR CONSEQUENTIAL COSTS, EXPENSES, OR DAMAGES INCURRED BY THE CONSUMER OR ANY OTHER USER.

Some states/provinces do not allow the exclusion or limitation of implied warranties or consequential damages, so the above limitations or exclusions may not apply to you.

Legal Remedies.

may also have other rights that vary from state to state or province to province.

This Texas Instruments electronic product is

THIS

This warranty gives you specific legal rights, and you

Warranty Performance.

your defective product will be either repaired or replaced with a reconditioned model of an equivalent quality (at TI’s option) when the product is returned, postage prepaid, to Texas Instruments Service Facility. The warranty of the repaired or replacement unit will continue for the warranty of the original unit or six (6) months, whichever is longer. Other than the postage requirement, no charge will be made for such repair and/or replacement. TI strongly recommends that you insure the product for value prior to mailing.

Software.

warrant that the software will be free from errors or meet your specific requirements.

Copyright.

product are protected by copyright.

Software is licensed, not sold. TI and its licensors do not

All software is provided “AS IS.”

The software and any documentation supplied with this

During the above one (1) year warranty period,

All Customers outside the U.S. and Canada

For information about the length and terms of the warranty, refer to your package and/or to the warranty statement enclosed with this product, or contact your local Texas Instruments retailer/distributor.

EXAS INSTRUMENTS INCORPORATED

TI-10: A Guide for Teachers

E-2

Texas instruments TI-10 A Guide for Teachers

Specifications and Main Features

Frequently Asked Questions

User Manual

Table of Contents

About the Teacher Guide

Activities

The Value of Place Value

What’s the Problem?

Comparing Costs

Equation Operating System (EOS™)

Order of Operations

Clearing and Correcting

Clearing entries

Menus

Basic Operations

Finding patterns in previous problems

Entering negative numbers

Division with remainders

Division with decimal result

Calculating equivalent units of time

Constant Operation

Addition as “counting on”

Multiplication as “repeated addition”

Clearing the constant operation

Whole Numbers and Decimals

Setting the number of decimal places

Addition with money

Memory

Using memory to add products

Using memory to find averages

Problem Solving: Auto Mode

Select level of difficulty

Select type of operation

Test your skills

View the Scoreboard

Find the operator

Problem Solving: Manual Mode

Problems with one solution

Problems with more than one solution

Problems with no solution

Less than, greater than, equal to

View the Scoreboard

Place Value

Determine place value

Repeated digits

How many?

What’s the digit?

Quick Reference to Keys

Display Indicators

Menu Map

Error Messages

Support, Service, and Warranty