Texas instruments TI-15 A Guide for Teachers

TI-15

A Guide for Teachers

TI.15

:

A Guide for Teachers

Developed by

Texas Instruments Incorporated

Activities developed by

Jane Schielack

About the Author

Jane Schielack is an Associate Professor of Mathematics Education in the Department of Mathematics at Texas A&M University. She developed the the examples in the

How to Use the TI-15

Activities

section of this guide.

section and assisted in evaluating the appropriateness of

Important Notice Regarding Book Materials

Texas Instruments makes no warranty, either expressed or implied, including but not limited to any implied warranties of merchantability and fitness for a particular purpose, regarding any programs or book materials and makes such materials available anyone for special, collateral, incidental, or consequential damages in connection with or arising out of the purchase or use of these materials, and the sole and exclusive liability of Texas Instruments, regardless of the form of action, shall not exceed the purchase price of this book. Moreover, Texas Instruments shall not be liable for any claim of any kind whatsoever against the use of these materials by any other party.

Note

: Using calculators other than the TIN15 may produce results different from those described in these

materials.

solely

on an “as-is” basis. In no event shall Texas Instruments be liable to

Permission to Reprint or Photocopy

Permission is hereby granted to teachers to reprint or photocopy in classroom, workshop, or seminar quantities the pages or sheets in this book that carry a Texas Instruments copyright notice. These pages are designed to be reproduced by teachers for use in classes, workshops, or seminars, provided each copy made shows the copyright notice. Such copies may not be sold, and further distribution is expressly prohibited. Except as authorized above, prior written permission must be obtained from Texas Instruments Incorporated to reproduce or transmit this work or portions thereof in any other form or by any other electronic or mechanical means, including any information storage or retrieval system, unless expressly permitted by federal copyright law.

Send inquiries to this address:

Texas Instruments Incorporated 7800 Banner Drive, M/S 3918 Dallas, TX 75251 Attention: Manager, Business Services

If you request photocopies of all or portions of this book from others, you must include this page (with the permission statement above) to the supplier of the photocopying services.

www.ti.com/calc

ti-cares@ti.com

Except for the specific rights granted herein, all rights are reserved.

Printed in the United States of America.

Automatic Power Down, APD, and EOS are trademarks of Texas Instruments Incorporated.

About the Teacher Guide



How the Teacher Guide is Organized

This guide consists of two sections: and

How to Use the TI-15

section is a collection of activities for integrating the TI-15 into mathematics instruction. to help you teach students how to use the calculator.

How To Use the TI-15

. The

Activities

is designed

Activities

The activities are designed to be teacherdirected. They are intended to help develop mathematical concepts while incorporating the TI-15 as a teaching tool. Each activity is self-contained and includes the following:

An overview of the mathematical purpose

•

of the activity.

The mathematical concepts being

•

developed.

The materials needed to perform the

•

activity.

A student activity sheet.

•

How to Use the TI.15

This section contains examples on transparency masters. Chapters are numbered and include the following:

An introductory page describing the

•

calculator keys presented in the examples, the location of those keys on the TI-15, and any pertinent notes about their functions.

Transparency masters following the

•

introductory page provide examples of practical applications of the key(s) being discussed. The key(s) being discussed are shown in black on an illustration of the TI-15 keyboard.

Things to Keep in Mind

While many of the examples on the

•

transparency masters may be used to develop mathematical concepts, they were not designed specifically for that purpose.

For maximum flexibility, each example and

•

activity is independent of the others. Select the transparency master that emphasizes the key your students need to use to develop the mathematical concepts you are teaching. Select an appropriate activity for the mathematical concept you are teaching.

If an example does not seem

•

appropriate for your curriculum or grade level, use it to teach the function of a key (or keys), and then provide relevant examples of your own.

To ensure that everyone starts at the

•

same point, have students reset the calculator by pressing − and simultaneously or by pressing selecting RESET, selecting Y (yes), and then pressing

<

.

”

‡

,

How to Order Additional Teacher Guides

To place an order or to request additional information about Texas Instruments (TI) calculators, call our toll-free number:

1-800-TI-CARES (1-800-842-2737)

Or use our e-mail address:

ti-cares@ti.com

Or visit the TI calculator home page:

http://www.ti.com/calc

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

iv

About the TI.15

Two-Line Display

The first line displays an entry of up to 11 characters. Entries begin on the top left. If the entry will not fit on the first line, it will wrap to the second line. When space permits, both the entry and the result will appear on the first line.

The second line displays up to 11 characters. If the entry is too long to fit on the first line, it will wrap to the second line. If both entry and result will not fit on the first line, the result is displayed right-justified on the second line. Results longer than 10 digits are displayed in scientific notation.

If an entry will not fit on two lines, it will continue to wrap; you can view the beginning of the entry by scrolling up. In this case, only the result will appear when you press

®

.

Display Indicators

Refer to Appendix B for a list of the display indicators.

Error Messages

Refer to Appendix C for a listing of the error messages.

Order of Operations

The TI-15 uses the Equation Operating System (EOSé) to evaluate expressions. The operation priorities are listed on the transparency master in Chapter 1,

Scrolling, Order of Operations, and Parentheses

Because operations inside parentheses are performed first, you can use X or Y to change the order of operations and, therefore, change the result.

Display,

Menus

Two keys on the TI-15 display menus: and

Press $ or # to move down or up through the menu list. Press ! or " to move the cursor and underline a menu item. To return to the previous screen without selecting the item, press ”. To select a menu item, press

®

Previous Entries

After an expression is evaluated, use and $ to scroll through previous entries and results, which are stored in the TI-15 history.

.

¢

while the item is underlined.

# $

Problem Solving (‹)

The Problem Solving tool has three features that students can use to challenge themselves with basic math operations or place value.

Problem Solving (Auto Mode) provides a set of electronic exercises to challenge the student’s skills in addition, subtraction, multiplication, and division. Students can select mode, level of difficulty, and type of operation.

Problem Solving (Manual Mode) lets students compose their own problems, which may include missing elements or inequalities.

Problem Solving (Place Value) lets students

.

display the place value of a specific digit, or display the number of ones, tens, hundreds, thousands, tenths, hundredths, or thousandths in a given number.

‡

#

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

v

About the TI.15

(Continued)

Resetting the TI.15

Pressing − and ” simultaneously or pressing (yes), and then pressing calculator.

Resetting the calculator:

Returns settings to their defaults:

•

Standard notation (floating decimal), mixed numbers, manual simplification, Problem Solving Auto mode, and Difficulty Level 1 (addition) in Problem Solving.

Clears pending operations, entries in

•

history, and constants (stored operations).

, selecting RESET, selecting

‡

resets the

®

Automatic Power DownTM (APDTM)

Y

If the TI-15 remains inactive for about 5 minutes, Automatic Power Down (APD) turns it off automatically. Press − after APD. The display, pending operations, settings, and memory are retained.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

vi

Activities

Patterns in Percent 2

Fraction Forms 6

Comparing Costs 11

Number Shorthand 15

Related Procedures 20

In the Range 24

The Value of Place Value 29

What’s the Problem? 34

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

1

Patterns in Percent

Grades 4 - 6

Overview

Students will use the ª key to collect data about percentages of a given number. They will organize the data and look for patterns in percents. (For example, 10% of 20 is twice as much as 5% of 20.)

Introduction

1. After students use manipulatives to develop the meaning of percent (1% = 1 part out of 100 parts), have them explore what happens when they press ª on the calculator.

2. Present the following scenario to students:

Metropolis East (M.E.) and Metropolis West (M.W.) are neighboring cities. The sales tax in M.E. is 10%, but the sales tax in M.W. is only 5%. Collect data and display your results for each percent in a table to compare the amounts of money you would pay for tax on various items in each city.

3. Have students make conjectures about percent based on the patterns they observe. Students can then use manipulatives to verify their conjectures.

Examples:

•

Students may observe that for every item, 10% of its price is twice as much as 5% of its price.

•

Students may observe that it is easy to estimate 10% of a whole number by using place value and looking at the digits to the right of the ones place.

Math Concepts

• multiplication

• equivalent fractions, decimals, and percents

³

When a student enters

ª

6

a

, the TI-15 displays 6%. Then, when the student presses ®, the display changes to

6%= 0.06

6% is another way to write 0.06 or 6/100.

³

You will need to show students how to use multiplication on the TI-15 to express the percent of a given quantity. For example, to show 10% of $20:

1. Enter 10.

2. Press ª V.

3. Enter 20; press ®. Students can verify the

calculator display of by using manipulatives to show 10% of $20 = $2.

Materials

• TI-15

• pencil

• student activity (page 4)

to show that

2

Collecting and Organizing Data

To guide students in organizing their data to bring out patterns, ask questions such as:

How could you organize your data to compare

•

the 5% tax rate to the 10% tax rate?

Why would it be useful to keep 5% in the left-

•

hand column of one table all the way down and just change the total quantity?

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

2

Patterns in Percent

How can you make a similar table for 10% to

•

compare your data?

What do you think would happen if you order

•

the total quantity amounts from least to greatest?

How else might you organize your data to

•

compare the two tax rates and find patterns in the percents?

(Continued)

Analyzing Data and Drawing Conclusions

To focus students’ attention on looking for patterns in their data, ask questions such as:

How are the percentages (amounts of tax) in

•

your 5% table like the amounts in the 10% table?

How does 5% of a $20 item compare to 5% of a

•

$10 item?

How does 10% of a $20 item compare to 10% of

•

a $10 item?

How does 10% of the cost of an item compare to

•

the total cost of the item?

What conjectures can you make about finding

•

10% of a number?

What conjectures can you make about finding

•

5% of a number?

How can you use manipulatives to test your

•

conjectures?

Continuing the Investigation

Students can create other percent scenarios to investigate patterns in percents. For example, ask students:

What happens if you increase the sales tax by

•

one percentage point each day?

How does the tax on a $20 item change each

•

day?

•

How does the tax on a $40 item change each day?

How do the taxes on the 2 items compare?

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

3

Name ___________________________

Patterns in Percent

Date ___________________________

Collecting and Organizing Data

Use your calculator to collect data about percent, organize it in the table below, and then look for patterns.

Cost of Item Amount of Tax in

Metropolis West

Tax Rate: ____%

Amount of Tax in

Metropolis East

Tax Rate: ____%

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

4

Name ___________________________

Patterns in Percent

Date ___________________________

Analyzing Data and Drawing Conclusions

1. What patterns do you see in your tables?

__________________________________________________________________________

2. What conjectures can you make from these patterns?

__________________________________________________________________________

3. Repeat the activity with a different percent in the left column and compare your results.

__________________________________________________________________________

4. Repeat the activity, changing the percents in the left column while keeping the total quantity constant. Now what patterns do you see? What conjectures can you make?

__________________________________________________________________________

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

5

Fraction Forms

Grades 4 - 6

Overview

Students will compare the results of using division to create fractions under the different mode settings for fraction display and make generalizations from the patterns they observe.

Introduction

1. Present students with a problem such as:

In a small cafe, there are 6 cups of sugar left in the pantry to put into 4 sugar bowls. If you want them all to contain the same amount of sugar, how much sugar goes into each sugar bowl?

2. Have students present their solutions to the problem. Encourage them to find as many ways to represent the solution as possible.

Math Concepts

• division

• multiplication

• common factors

• equivalent fractions



Refer to page 45 for detailed information about mode settings on the TI-15.

Materials

• TI-15

• pencil

• student activity (page 9)

Examples:

•

By thinking of using a ¼ cup scoop to fill the bowls, each bowl would receive 6 scoops, or

6

/4 cups of sugar.

•

By thinking of separating each cup into half cups, there would be 12 half cups, and each bowl would receive 3 half cups, or

3

/2 cups of

sugar.

•

If a 1-cup measuring cup was used first, each bowl would receive 1 cup of sugar, then the last two cups could be divided into eight

2

fourths to give 1

•

The last two cups could be divided into 4 halves to give 1

/4 cups per bowl.

1

/2 cups per bowl.

3. Have students identify the operation and record the equation that they could use with the calculator to represent the action in the situation (6 cups ÷ 4 bowls = number of cups per bowl).



Division can be represented by 6 P 4 or 6/4 (entered on the calculator as 6  4 ¥). In this activity, the fraction representation is used.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

6

Fraction Forms

(Continued)

4. Have students enter the division to show the quotients in fraction form, and record the resulting displays.

5. Have students explore the quotient with the different combinations of settings and discuss the different displays that occur. If necessary, have them use manipulatives to connect the meanings of the four different fraction forms.

6. Have students, working in groups of four, choose a denominator and record the different fraction forms on the activity sheet provided.

7. Have students share their results, look for patterns, and make generalizations.

Collecting and Organizing Data

To guide students in creating data that will exhibit patterns in the fraction quotients, ask questions such as:

What denominator did you choose to explore

•

with? Why?



For example, for 6 ÷ 4 as a fraction, enter 6  4 ¥. The displays in the different modes will look like the following:

n

man

d n

auto

d

n

U

man 1

d n

U

auto 1

d

6 4

3 2

2 4

1 2

What denominators do you get with the settings

•

n

? With the settings

man

d

What denominators do you get with the settings

•

n d

What denominator are you going to choose to

•

? With the settings

auto

U

n d

man

auto

?

explore with next?

Example:

After exploring with denominators of 2 and 3, you might suggest exploring with a denominator of 6 and comparing results.

How can you organize your results to look for

•

patterns?

Example:

Continuing to increase the numerators by 1 each time.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

7

Fraction Forms

(Continued)

Analyzing Data and Drawing Conclusions

To focus students’ attention on the patterns in their fractions and the relationship of these patterns to the denominators, ask questions such as:

What patterns do you see in your results?

•

Example:

n

When a denominator of 4 is used in the

column, every fourth number is a whole number.

How do the results of using a denominator of 2

•

compare with the results of using a denominator of 4?

How does a denominator of 5 compare to a

•

denominator of 10?

Which other denominators seem to be related?

•

Example:

auto

d

The pattern using a divisor of 6 is related to the patterns for 2 and 3.)

What pattern do you see in the related

•

denominators?

Example:

They are related as factors and multiples.

Continuing the Investigation

Have students brainstorm situations in which they would prefer to use each of the combinations of settings of fraction forms.

Example:

•

When working with probabilities that may need to be added, using the would keep the denominators of the probabilities all the same and make mental addition easier.

•

In a situation where estimated results are close enough, using the would make it easier to see quickly the whole number component of the result and whether the additional fraction part was more or less

½.

than

U

n

man

d

n

auto

d

settings

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

8

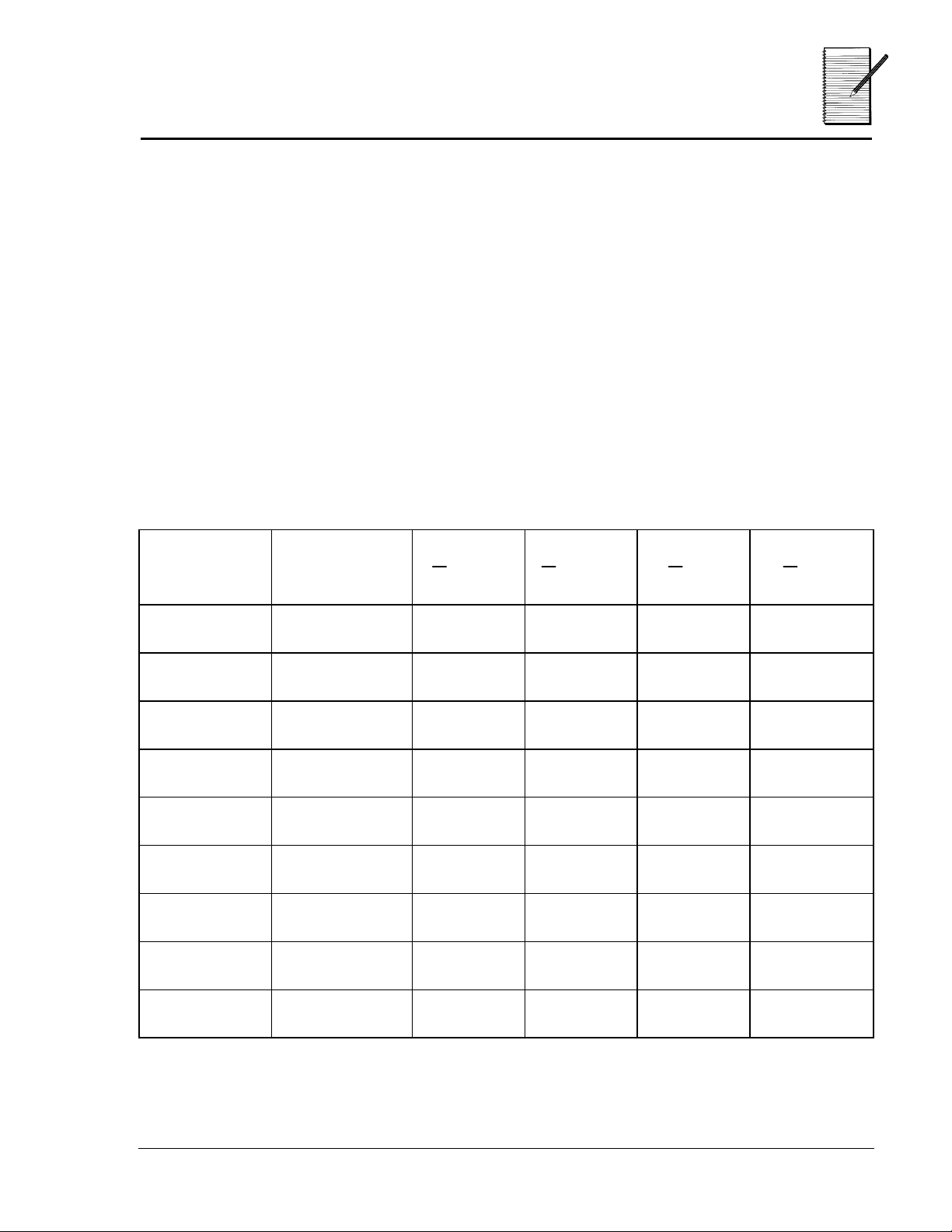
Name ___________________________

Fraction Forms

Date ___________________________

Collecting and Organizing Data

1. Have each person in your group set his/her calculator to one of the following combinations of modes for fraction display. (Each person should choose a different setting.)

improper/manual simp

•

improper/auto simp

•

mixed number/manual simp

•

mixed number/auto simp

•

2. Select a denominator: __________________

3. Use this denominator with several numerators and record each person’s results in the table below.

Numerator Denominator

n

Man

d

n

Auto U

d

n

Man U

d

n

d

Auto

0

2

3

4

1

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

9

Fraction Forms

(Continued)

Analyzing Data and Drawing Conclusions

1. What patterns do you see?

__________________________________________________________________________

2. What generalizations can you make?

__________________________________________________________________________

3. Try the activity again with a different denominator and compare your results with the two denominators.

__________________________________________________________________________

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

10

Comparing Costs

Grades 3 - 5

Overview

Students will solve a problem using division with an integer quotient and remainder, division with the quotient in fraction form, and division with the quotient in decimal form and compare the results.

Introduction

1. Introduce the following problem:

The maintenance department has determined that it will cost $.40 per square yard to maintain the district’s soccer field each year. The soccer field is 80 yards wide and 110 yards long. The six schools that play on the field have decided to split the cost evenly. How much should each school contribute to the soccer field maintenance fund this year?

2. Have students use the calculator to solve this problem in three ways:

•

Finding an integer quotient and remainder.

•

Finding the quotient in fraction form.

•

Finding the quotient in decimal form.

Collecting and Organizing Data

Students should record their procedures and results on the Student Activity page. To help them focus on their thinking, ask questions such as

:

Math Concepts

• division

• multiplication

• fractions

• decimals



To display an integer quotient with a remainder, use the key.



To display a quotient in fraction form, press

" ®

then use the W key.



To display a quotient in decimal form, press

‡ ! ®

then use the W key.

to select

Materials

• TI-15

• pencil

• student activity (page 14)

£

‡

n/d,

to select

.

,

•

What did you enter into the calculator to solve the problem?

Example:

80

A student may have entered determine the area of the soccer field, then

0.40

entered V cost, then W school in fraction or decimal form.

EXAS INSTRUMENTS INCORPORATED

®

to find the total maintenance

6

®

to find the cost for each

V

110

® to

TI-15: A Guide for Teachers

11

Comparing Costs

Could you have solved the problem more

•

efficiently? How?

Example:

A student may see that 80 x 110 could be done mentally, and the key presses could be simplified

8800

to

How are your procedures alike for each type of

•

solution?

Examples:

They all involve finding how many square yards in the soccer field; they all involve multiplication and division.

How are they different?

•

You use different keys to tell the calculator in what form you want the answer displayed.

V .

4

W

6

®

.

(Continued)

Analyzing Data and Drawing Conclusions

To guide students in the analysis of their data, ask questions such as:

How are your solutions in the three forms

•

alike?

They all have a whole number component of 586.

How are your three solutions different?

•

The remainder form just tells how many dollars are left over. The fraction and decimal forms tell how much more than $586 each school has to pay.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

12

Comparing Costs

What happens if you multiply each solution by

•

6 to check it?

(Continued)

For the remainder form, you have to multiply 586 x 6 and then add 4 to get the total cost of $3520. You can multiply 586 form to get $3520. If you enter press ®, you get

3520

2

/3 x 6 in fraction

586.666667 x 6

, but that doesn’t make

sense because 6 x 7 doesn’t end in a 0!

If you enter

586.66667

, then fix the decimal quotient to hundredths since it is money, and then find 586.67 x 6, you

still

get 3520.00, which still doesn’t make sense because 6 x 7 = 42. If you clear the calculator and enter press ® , then the display reads

586.67 x 6

3520.02

, and

does make sense.

As a school, which form of the quotient would

•

you want to use?

Responses may vary. Some students may want to use the decimal form, since it is the closest to the representation of money. Some students may want to use the integer quotient and remainder form and suggest that the Central Office pay the $4.00 remainder.

and

, which



When you fix

586.666667 to 2 decimal places, and then multiply by 6, the calculator “remembers” the original number and uses it as the factor. The product rounded to the nearest hundredth, using the original factor, is 3520.00. When you enter 586.67, the calculator uses this number for the factor, showing the actual product of 3520.02.

Although the fraction form of the quotient describes the exact quantity that each school should pay, most students will recognize, by comparing it to the decimal form, that the fraction form is not easily translated into money.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

13

Name ___________________________

Comparing Costs

Date ___________________________

Collecting and Organizing Data

The Maintenance department has determined that it will cost $4.00 per square yard to maintain the district’s soccer field each year. The soccer field is 80 yards wide and 110 yards long. The 6 schools that play on the field have decided to split the cost evenly. How much should each school contribute to the soccer field maintenance fund this year?

1. Use division with an integer quotient and remainder:

2. Use division with a quotient in fraction form:

3. Use division with a quotient in decimal form:

Analyzing Data and Drawing Conclusions

Write a short paragraph comparing the three solutions.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

14

Number Shorthand: Scientific Notation

Grades 5 - 6

Overview

Students will use patterns created on the calculator with the constant operation (› or to develop an understanding of scientific notation.

Introduction

1. Have students review the pattern created when using 10 as a factor.

Example:

1 x 10 = 10 2 x 10 = 20 3 x 10 = 30 10 x 10 = 100

2. Ask students:

Based on this pattern, what do you think happens when we multiply by 10 over and over again?

œ

Math Concepts

• multiplication

)

• powers of 10

• exponents

Materials

• TI-15

• pencil

• student activity (page 18)

3. After students share their conjectures, have them use › to test their conjectures. As students press ›, have them record the resulting displays on the Student Activity page.

4. When students reach the point where the lefthand counter is no longer displayed, ask them what they think has happened to the calculator. (The product has become so large that there is not room to display both the product and the counter, so the counter has been dropped.)

Have students continue to record the counter data, even though it no longer shows on the calculator.

5. When the left-hand counter reappears, have students describe what has happened to the display of the product. (It has been replaced with a right-hand display of scientific notation: for example,

1x10^11.

)



To multiply repeatedly by 10, enter:

› V

1. This “programs” the

constant operation.

2. Enter 1 as the starting factor.

3. Press ›.

When you press › the first time, the calculator performs the operation 1 x 10 and the display shows:

1x10 110

The 1 represents using

x 10

one time.

10

›

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

15

Number Shorthand: Scientific Notation

(Continued)

6. Have students continue to press › and record the results.

7. Have students analyze their data and make some conclusions about the scientific notation display.

For example,

1 x 10 x 10 x 10 x 10 x 10 x 10 x 10 x 10 x 10 x 10 x 10

Explain to students that exponential or scientific notation is a shorthand for repeated factors:

11

1 x 10

8. Have students continue to explore the use of scientific notation to represent repeated multiplication by 10 with other starting factors. (For example, using 2 as the starting factor, the display eleven times, or 2 x 10

.

1x10^11

2x10^11

represents the product:

represents multiplying 2 by 10

11

.

Collecting and Organizing Data

To focus students’ attention on the relevant changes in the calculator’s display, ask questions such as:

What does the display

•

When did the counter on the left disappear? Why

•

do you think that happened?

3 1000

mean?

.

•

When did the counter on the left reappear? What else has changed?

The product looks different. It changed from 1000000000 to 1x10^10.

What do the displays look like after this change takes place?

The 1x10 stays the same, but the right-hand number (the exponent) goes up one each time

›

is pressed, and it matches the left-hand

counter.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

16

Number Shorthand: Scientific Notation

(Continued)

Analyzing Data and Drawing Conclusions

To focus students’ attention on the connection between the repeated factors of 10 and the scientific notation display, ask questions such as:

What patterns do you see in your products

•

before the counter disappears?

They all have a 1 followed by the same number of zeroes as factors of 10 that were used in the product.

If you continued this pattern, what would the

•

product be at the point where the display of the product changed? How is the product related to the new display?

For example, product should be 100,000,000,000. The display

1x10^11

What happens if you use 2 as the starting factor

•

and multiply by 10 repeatedly?

The displays are the same, except the first number in all the products is 2. The display

2x10^11

1x10^11

represents the product 1 x 1011.

represents the product 2 x 1011.

is in the place where the

Continuing the Investigation

Students can use other powers of 10 as the repeating factor, record the results in the table, and look for patterns. For example, using 100 as the repeating factor causes the exponent part of the scientific notation display to increase by 2 every time › is pressed.

Students can use a starting factor of 10 or greater, record the results in the table, and look for patterns. For example, using 12 as the starting factor soon results in a display like exponent part of the display is one more than the number of times 10 has been used as a factor.

12 1.2 x10^13

, where the

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

17

Number Shorthand:

Name ___________________________

Date ___________________________

Scientific Notation

Collecting and Organizing Data

Program the constant operation feature on your calculator to multiply by

10. Record the results in the table below for each time you press ›.

Number of

Times

___

Used as a

Factor

0 (starting factor)

1

Display

2

3

4

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

18

Number Shorthand:

Name ___________________________

Date ___________________________

Scientific Notation

Analyzing Data and Drawing Conclusions

1. What patterns do you see?

__________________________________________________________________________

2. What does it mean when the right-hand display changes? (For example,

__________________________________________________________________________

1x10^15

.)

__________________________________________________________________________

3. Try the activity again with another multiple of 10 and compare your results.

__________________________________________________________________________

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

19

Related Procedures

Grades 2 - 6

Overview

Students will use the two constant operations ( and œ) to compare the results of different mathematical procedures and determine how they are related.

Introduction

1. Have students program › with +2 and with -2.

2. Have students enter

, and read the output (

›

adding 2 once to 8 gives 10).

3. Have students press œ to apply the second constant operation to the output of the first constant operation, and then read the output

1 8

(

, which means subtracting 2 once from 10

gives 8).

4. Have students continue this process with various numbers as their first input. Discuss their results. (Pressing › and then œ always gets you back to the first input number, which means › and

are inverse procedures.)

œ

5. Challenge students to find more pairs of procedures for › and œ that will follow the same pattern and record their investigations using the

Related Procedures

page.

8

on their calculators, press

1 10

, which means

student activity

œ

›

Math Concepts

• whole numbers

• addition, subtraction, multiplication, division

• fractions (Grades 5-6)

• decimals (Grades 5-6)

³

To use › and œ:

1. Press › (or œ).

2. Enter the operation and the number (for example, T 2).

3. Press › (or œ).

4. Enter the number to which you want to apply the constant operation.

5. Press › (or œ). The display will have

1

a

on the left and the result on the right. If you press › (or œ) again, the calculator will apply the constant operation to the previous output and display a indicating the constant operation has been applied twice to the original input.

Materials

• TI-15

• pencil

• student activity (page 23)

2

at the left,

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

20

Related Procedures

Collecting and Organizing Data

As students use › and œ, have them record their results in the appropriate tables on the Student Activity page. For example, if a student is exploring the relationship between look something like this:

x 2

and

÷ 2

, the tables might

(Continued)

Table for

›

Input Procedure Output

1x22 2x24 3x26

œ

Input Procedure Output

2 4 6

P

21

P

22

P

23

Analyzing Data and Drawing Conclusions

Ask students:

What patterns do you see in your data?

•

Are the procedures inverses of each other? How

•

do you know?

If the output number for › is used as the input number for œ and gives an output number equal to the original input number for ›, then the procedures may be inverses of each other, as in

x 2

and

÷ 2

.

³

To recognize the equivalent procedures, students may need to use the Ÿ key to change outputs from decimal to fraction form or vice versa.

•

Does the pattern work with special numbers like 1 and 0? With fractions and decimals? With positive and negative integers?

What happens if you use

?

›

EXAS INSTRUMENTS INCORPORATED

first, and then

œ

TI-15: A Guide for Teachers

21

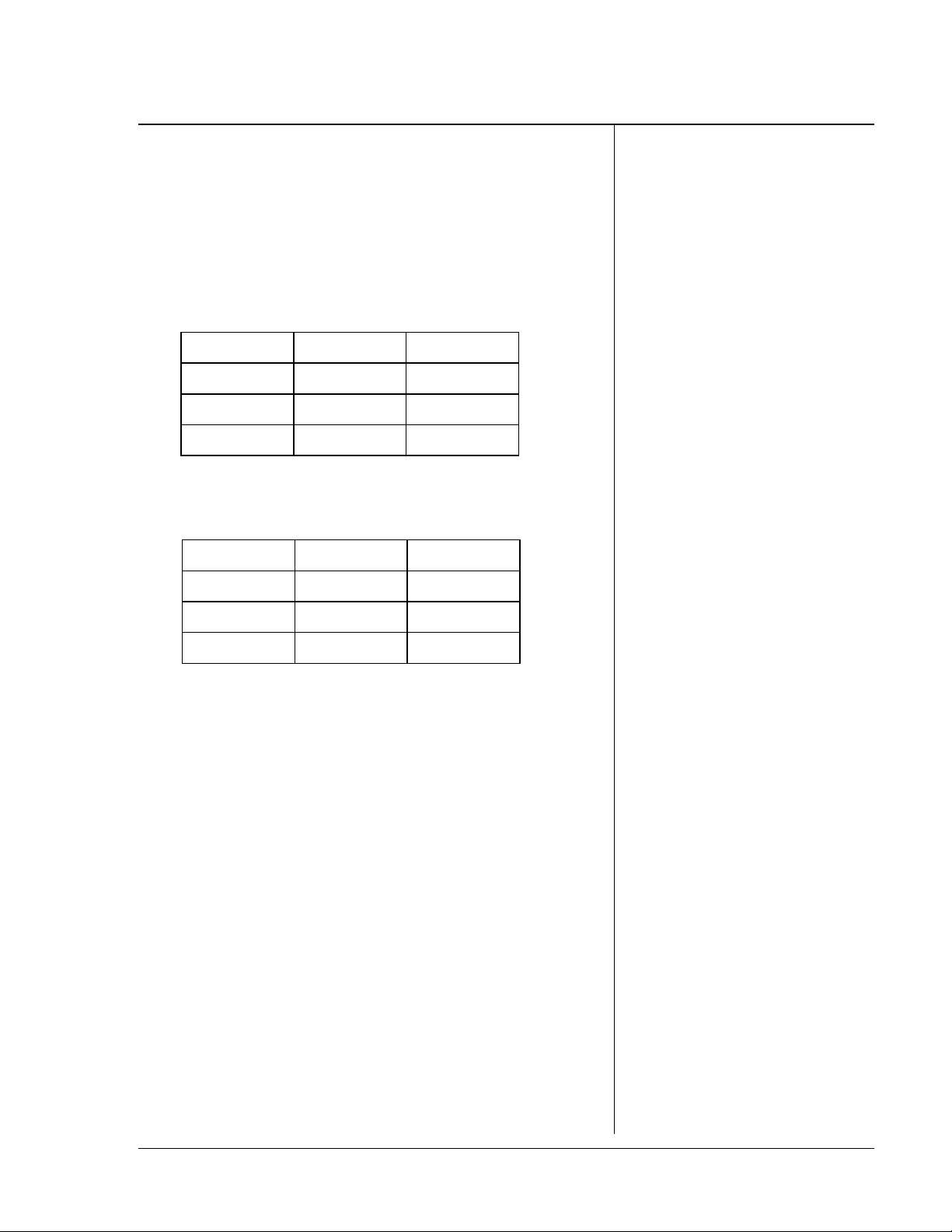
Related Procedures

Continuing the Investigation

Older students can investigate equivalent procedures, such as dividing by a number and multiplying by its reciprocal. For example, if a student is exploring the relationship between

÷ 2

and

, the tables might look something like this:

(Continued)

x ½

Table for

›

Input Procedure Output

1x½½ 2x½1 3 x½ 1.5 = 1½

œ

Input Procedure Output

1 2 3

P

2 0.5 = 5/

P

21

P

2 1.5 = 15/

10 =

½

1½

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

22

Name ___________________________

Related Procedures

Date ___________________________

Collecting and Organizing Data

1. Choose a procedure for › (for example,

2. Choose a procedure for

(for example,

œ

3. Select an input number to apply the procedure to and record both the input and output numbers in the appropriate table.

4. Use the tables below to record and compare your results using and

.

œ

Table for

›

Input Procedure Output Input Procedure Output

).

x ½

).

÷ 2

Table for

›

œ

Analyzing Data and Drawing Conclusions

5. How do the two procedures compare?

__________________________________________________________________________

6. What patterns do you see?

__________________________________________________________________________

7. Are the two procedures related? Explain.

__________________________________________________________________________

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

23

In the Range

Grades 3 - 6

Overview

Students will interpret the rounding involved in measuring to identify the possible range of a given measurement.

Introduction

1. Have students measure the length of a table or desk in the room and record the measurement to the nearest millimeter, for example, 1357 mm.

Discuss how measurements in millimeters can be recorded as 1357 mm or as thousandths of meters, 1.357 m. Note that the measurement was rounded to 1357 mm because it fell somewhere between ½ of a millimeter less than 1357 mm (1356.5 mm) and ½ of a millimeter more than 1357 mm (1357.5 mm).

Math Concepts

• rounding whole numbers

• rounding decimals

• measurement with metric units (length, mass, capacity)

Materials

• TI-15

• pencil

• meter sticks or metric measuring tapes

• student activity

(p.27)

1356.5 1357 1357.5

2. Have students then use rounding to record the same measurement to the nearest centimeter (136 cm or 1.36 m).

3. Enter the original measurement on the calculator as 1.357 and fix the display at two decimal places.

4. Have students fix the display at one decimal place. Ask:

What does this number represent?

(The measurement rounded to the nearest tenth of a meter, or the measurement rounded to 14 decimeters.)

EXAS INSTRUMENTS INCORPORATED

³

To fix the display at 2 decimal places, press

Š ™ ®

³

Have students discuss how the display of matches their rounding of the measurement to 136 cm.

TI-15: A Guide for Teachers

.

1.36

24

In the Range

(Continued)

5. Have students fix the display to no decimal

(The

1

. Ask:

places. press Š and then “ to display

What does this number represent?

measurement rounded to the nearest meter.)

6. Introduce the entering a number on the calculator with three decimal places to represent a measurement in millimeters; for example, 2.531. Then display the number rounded to the nearest whole number (3). Show this display to students.

7. Tell students that this number represents the measurement of a length of board to the nearest meter. Ask students:

What could its measurement be if it had been measured to the nearest decimeter?

(2.5 m to 3.5 m)

8. Round the original number to the nearest tenth (2.5). Ask students:

Does this lie within the range we identified?

In the Range

game by secretly

³

To round to the nearest whole number, press

Š “ ®

³

To round to the nearest tenth, press Š ˜ ®.

.

9. Repeat for measuring to the nearest centimeter (hundredths) and millimeter (thousandths). (The range for centimeters would be 2.45 to 2.55, with

2.53 lying within that range; and the range for millimeters would be 2.525 to 2.535, with 2.531 lying within that range.)

10. Have students work in pairs to play the game and record their observations on their student activity pages.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

25

In the Range

(Continued)

Collecting and Organizing Data

As students are playing the game, focus their attention on the patterns that are developing by asking questions such as:

When you record a measurement, why is

•

rounding always involved?

When you read a measurement, what interval

•

should that measurement always indicate to you?

(½ a unit less or ½ a unit more

How would this interval look on a number line

•

(or meter stick)?

How is ½ represented in the metric system?

•

How are you deciding how to represent the

•

range of possible measurements? What patterns are you using?

)

Analyzing Data and Drawing Conclusions

To guide students in the analysis of their data, ask questions such as:

What range is indicated by every measurement?

•

What patterns did you use in identifying the

•

range of possible measurements?

How would you use these patterns to round

•

256.0295 to the nearest tenth?

Continuing the Investigation

Have students replace the units of length with units of mass (grams, centigrams) or capacity (liters, milliliters) to notice the same patterns.

Have students discuss why this decimal place-value approach with the calculator does not work for measurements in yards, feet, and inches. Have them identify what range a measurement would lie in if it was measured to the nearest yard, nearest foot, and nearest inch. (For example, 2 yards would lie between 1 yard and 18 inches and 2 yards and 18 inches.)

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

26

Name ___________________________

In the Range

Date ___________________________

Collecting and Organizing Data

Have your partner secretly enter a measurement with three decimals places into the calculator, and then fix the number to be rounded to the nearest whole number. Now look at the display and answer the following questions:

1. What is the measurement to the nearest meter? _________________

a. What could be the range of the measurement if it had been

measured to the nearest tenth of a meter (decimeters)?

_______________________________________________________________________

b. Set Š to the nearest tenth (˜).

What is the measurement to the nearest tenth? _______________

Is that within the range you identified? ______________________

2. What is the measurement to the nearest tenth of a meter? ________

a. What could be the range of the measurement if it had been

measured to the nearest hundredth of a meter (centimeters)?

_______________________________________________________________________

b. Set Š to the nearest hundredth (

™

).

What is the measurement to the nearest hundredth? __________

Is that within the range you identified? ______________________

3. What is the measurement to the nearest hundredth of a meter? ___

a. What could be the range of the measurement if it had been

measured to the nearest thousandth of a meter (millimeters)?

_______________________________________________________________________

b. Set Š to the nearest thousandth (

š

).

What is the measurement to the nearest thousandth? _________

Is that within the range you identified? ______________________

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

27

Name ___________________________

In the Range

Date ___________________________

Analyzing Data and Drawing Conclusions

Identify three measurements to the nearest millimeter that would be:

a. 10 m when rounded to the nearest meter. ___________________

b. 9.0 m when rounded to the nearest tenth of a meter (decimeter).

_______________________________________________________________________

c. 9.05 m when rounded to the nearest hundredth of a meter

(centimeter).

_______________________________________________________________________

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

28

The Value of Place Value

Grades 2 - 6

Overview

Students will build their flexibility in using numbers by exploring the connections between the number symbols and their representations with base-ten materials.

Introduction

1. Read

2. Give each group of students a large pile of units

3. Tell students you have run out of unit pieces and

4. Have students explore the answer to this

5. Have students compare their solutions with the

Counting On Frank

by Rod Clement. Discuss some other kinds of questions that a person could ask about how many objects fit in or on other objects.

(over 300) from the base-ten materials, and tell them that this is how many jelly beans fit into a jar that you filled. Ask them to count the “jelly beans,” and observe the techniques they use (counting one at a time, making groups of 10, etc.).

then ask:

How many rods (groups of 10) would I need to use to make a pile of jelly beans the same size as yours?

problem with their units or apply their knowledge of place value. Then show them how to explore the answer using the calculator.

base-ten materials to the calculator display. (They can make 31 tens rods from the 314 units, with 4 units left over.)

‡

mode to

1

– .

is used to

31

í

Materials

• TI-15

• pencil

•

• base-ten

• student

.

, meaning

Math Concepts

Grades 2 - 4

• whole number place value (through thousands)

• money

Grades 4 - 6

• decimal place value (through thousandths)

• metric units (meters, decimeters, centimeters)

³

To use the Place Value feature for this activity:

1. Press ‹

2. Press " ® to select

MAN

(Manual).

3. Press $ ® to set the

Place Value 11

–.

This lets you find out how many ones, tens, hundreds, etc., are in a number. (The mode – find what digit is in the ones, tens, hundreds, etc., place.)

³

To explore answers to this problem on the calculator:

1. Press ‹.

2. Enter the number of units (for example,

314).

3. Press Œ ’ to see the display. (Using 314, the display is there are 31 tens in

314.)

Counting on Frank

by Rod Clement

materials

activity (pages 32 and 33)

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

29

The Value of Place Value

Collecting and Organizing Data

Have students use their base-ten materials and the calculator to continue the exploration with other numbers, identifying how many hundreds and thousands (and 0.1s and 0.01s for older students). Encourage exploration with questions such as:

How many hundreds are in 120? 2478? 3056?

•

How many tens are in 120? 2478? 3056?

•

How many units (ones) are in 120? 2478?

•

3056?

What numbers can you find that have 12 units?

•

12 tens? 12 hundreds?

What numbers can you find that have 60 units?

•

60 tens? 60 hundreds?

Analyzing Data and Drawing Conclusions

Have students use the table on

Value

Student Activity page to record their findings and identify the patterns they see. To help them focus on the patterns, ask questions such as:

The Value of Place

(Continued)

³

Students can use the

11

Place Value

– .

mode to test their conjectures. For example, if they think 1602 has 160 hundreds, they enter

1602

, press Œ ‘, and

íí

16

see then use the base-ten materials to see why there are only 16 hundreds in 1602. (If students use the – mode to find what digit is in the hundreds place, they will see displayed to show that 6 is the digit in the hundreds place.

. They can

1

– .

íí

í

6

How does the number of tens in 1314 compare

•

to the number 1314? How about 567? 2457? 4089, etc.?

If you cover the digit in the units place, you see how many tens are in a number.

How does the number of hundreds in 1314

•

compare to the number 1314? How about 567? in 2457? in 4089, etc.?

If you cover the digits to the right of the hundreds place, you see how many hundreds are in a number.

How does the display on the calculator compare

•

to what you can do with the base-ten materials?

If the calculator shows 31_, for 316, I should be able to make 31 tens rods out of the 316 units I have.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

30

The Value of Place Value

Continuing the Investigation

Connect the place-value patterns to money. For example, ask students:

If each one of your “jelly beans” costs a penny,

•

how many pennies would you spend for 1,314 jelly beans?

1,314 pennies.

How many dimes (tens) would you spend?

•

131 dimes and 4 more pennies.

How many dollars (hundreds)?

•

13 dollars, plus 14 more pennies, or 1 dime and 4 pennies.

Older students can record the money (and enter it into the calculator) in decimal form, 13.14. Then they can use the calculator to connect dimes to one tenth (0.1) of a dollar ($13.14 has 131 dimes or tenths) and pennies to one hundredth (0.01) of a dollar ($13.14 has 1314 pennies or hundredths).

(Continued)

For older students, connect the place-value patterns to conversions between metric units. For example, a measurement of 324 centimeters can also be recorded as 32.4 decimeters (or rounded to 32 dm) because 1 dm = 10 cm, or it can be recorded as 3.25 meters (or rounded to 3 m), because 1 m = 100 cm.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

31

The Value of Place

Name ___________________________

Date ___________________________

Value, Part A

Collecting and Organizing Data

1. Use your base-ten materials and your calculator to explore how many tens, hundreds, and thousands are in a number. Record your observations in the table. What patterns do you see?

Number Number of

Thousands

Number of

Hundreds

Number of

Tens

Analyzing Data and Drawing Conclusions: Patterns

2. Write 5 numbers that have 15 tens.

__________________________________________________________________________

3. Write 5 numbers that have 32 hundreds.

__________________________________________________________________________

4. Write 5 numbers that have 120 tens.

__________________________________________________________________________

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

32

The Value of Place

Name ___________________________

Date ___________________________

Value, Part B

Collecting and Organizing Data

1. Use your base-ten materials and your calculator to explore how many tenths, hundredths, and thousandths are in a number. Record your observations in the table. What patterns do you see?

Number Number of

Tenths

Number of

Hundredths

Number of

Thousandths

Analyzing Data and Drawing Conclusions: Patterns

2. Write 5 numbers that have 15 tenths.

__________________________________________________________________________

3. Write 5 numbers that have 32 hundredths.

__________________________________________________________________________

4. Write 5 numbers that have 120 tenths.

__________________________________________________________________________

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

33

What’s the Problem?

Grades 2 - 5

Overview

Students will connect number sentences to problem situations and use addition, subtraction, multiplication, and division to solve the problems.

Introduction

1. On a sentence strip or on the overhead, display a number sentence such as “8 + 2 = ?” Have students brainstorm situations and related questions that this number sentence could be representing. For example, “If I bought eight postcards on my vacation and I had two postcards already at home, how many postcards do I have now?”

2. If necessary, have students act out the situation with counters and determine that the value of “?” is 10.

3. Demonstrate how to display this equation on the calculator, and how to tell the calculator what the value of ? is.

Math Concepts

• addition, subtraction

• multiplication, division (Grades 3 - 5)

• number sentences (equations)

• inequalities (Grades 3 - 5)

³

To display this equation on the calculator, put the calculator in Problem Solving mode by pressing the ‹ key. Then enter the equation

8 + 2 = ?

®

display ( how many whole number solutions there are to the equation.

To test your solution to the equation, enter the value of 10 and press

®

display

and press

. The calculator

1 SOL

. The calculator will

YES

Materials

• TI-15

• counters

• pencil

• student activity (page 37)

) tells

.

4. Now display an equation such as ? - 10 = 5. Have students brainstorm situations and related questions that this number sentence could be representing. For example, “I had some money in my pocket, and I spent 10 cents of it. I only have 5 cents left. How much money did I have in my pocket to begin with?” Have students practice the keystrokes necessary to display this equation and test the value they determine for “?”.

5. Over a period of time, continue to introduce students to different types of number sentences to explore. For example, ? - 8 < 5 (which has 13 whole number solutions) and ? x ? = 24 (which has 8 solutions of whole number factor pairs) and ? x 4 = 2 (which has no whole number solution).

EXAS INSTRUMENTS INCORPORATED

³

If an incorrect value is tested for ?, the calculator will display

NO

and provide a hint. For example, if a student tests 5 for the equation calculator displays NO, then shows and then returns to the original equation.

TI-15: A Guide for Teachers

? - 10= 5

5 - 10 < 5

, the

,

34

What’s the Problem?

Collecting and Organizing Data

As an ongoing activity, have students work in pairs and use the sheet to create problem-solving cards. Have one partner create an addition, subtraction, multiplication, or division number sentence, using the “?” and record it in the top box and on the calculator. If possible, the other partner creates a situation and question to go with the number sentence and records it in the bottom box. The two boxes can be glued or taped to opposite sides of an index card.

Have students work together with the calculator to explore how many whole number solutions the equation has and test what the solutions are. Provide ideas for exploration by asking questions such as:

What actions could be happening in your story

•

to go with addition (subtraction, multiplication, or division)?

What’s The Problem?

Student Activity

(Continued)

How could you use these counters to act out this

•

number sentence?

What could this number in the number sentence

•

represent in your story?

What could the question mark in the number

•

sentence represent in your story?

Can you make a story for a number sentence

•

that begins with a question mark?

Analyzing Data and Drawing Conclusions

To focus students’ thinking on the relationships between their stories and the numbers and operations in their number sentences, ask questions such as:

How would using a different number here

•

change your story?

How would using a greater than or less than

•

symbol instead of an equal sign in the number sentence change your story?

•

How would using a different operation in your number sentence change your story?

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

35

What’s the Problem?

Continuing the Investigation

•

Have partners create stories and trade them. Each partner can then write a number sentence to go with the other partner’s story.

•

Have students sort the number sentences they have made into categories: e.g., those with 0 whole number solutions, those with one whole number solution, those with two whole number solutions, those with infinite whole number solutions.

•

Have students try to find an equation or inequality with exactly 0 whole number solutions, exactly 1 whole number solution, exactly 2 whole number solutions, more than 5 whole number solutions, etc.

(Continued)

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

36

Name ___________________________

What’s the Problem?

Date ___________________________

Write a number sentence using an operation and the “?”

Write a story that describes a situation and asks a question that can be represented by the number sentence.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

37

How to Use

the TI.15

Display, Scrolling, Order of

Operations, Parentheses 39 Clearing and Correcting 42 Mode Menus 45 Basic Operations 48 Constant Operations 55 Whole Numbers and Decimals 63 Memory 68 Fractions 7 1 Percent 80 Pi 84 Powers and Square Roots 88 Problem Solving: Auto Mode 94 Problem Solving: Manual Mode 100 Place Value 106

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

38

Display, Scrolling, Order of Operations, and Parentheses

1

Keys

1.

2.

3.

opens a parenthetical expression.

X

You can have as many as 8 parentheses at one time.

closes a parenthetical expression.

Y

and " move the cursor left and

!

right.

and $ move the cursor up and

#

down through previous entries and results.

Notes

The examples on the transparency

•

masters assume all default settings.

The EOSTM transparency master

•

demonstrates the order in which the TI-15 completes calculations.

When using parentheses, if you

•

press

Syn Error

Operations inside parentheses are

•

performed first. Use X or Y to change the order of operations and, therefore, change the result.

Example:

The first and second lines display

•

entries up to 11 characters plus a

3

decimal point, a negative sign, and a 2-digit positive or negative exponent. Entries begin on the left and scroll to the right. An entry will always wrap at the operator.

before pressing Y,

®

is displayed.

1 + 2 x 3 = 7 (1 + 2) x 3 = 9

EXAS INSTRUMENTS INCORPORATED

Results are displayed right-

•

justified. If a whole problem will not

1

2

fit on the first line, the result will display on the second line.

TI-15: A Guide for Teachers

39

Equation Operating System

Priority Functions

EOS

1 (first)

2

3

4

5

6

7

X Y

¢

¨ ¬

M

V W

T U

¦ Ÿ

8 (last)

®

Because operations inside parentheses are performed first, you can use X

Y

to change the order of operations and, therefore, change the result.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

40

Order of Operations

1 + 2 x 3 =

Press Display

1

T

2

V

3

1Û2Ý3Ú 7

®

(1 + 2) x 3 =

Press Display

Add

T

Multiply

V

Parentheses

X Y

X V

1 3

2

T

®

Y

Å1Û2ÆÝ3Ú 9

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

41

Clearing and Correcting

2

Keys

1.

2.

turns the calculator on and off.

−

clears the last digit you entered,

w

allowing you to correct an entry without re-entering the entire number.

3.

clears the last entry, all pending

”

operations, and any error conditions. You can then enter a new number and continue your calculation.

Notes

The examples on the transparency

•

masters assume all default settings.

Pressing − and ” simultaneously

•

resets the calculator. Resetting the calculator:

−

Returns settings to their defaults.

−

Clears memory and constants.

Pressing ” does not affect the

•

mode settings, memory, or constants.

1

EXAS INSTRUMENTS INCORPORATED

3

2

TI-15: A Guide for Teachers

42

Clearing entries

1. Enter 335 + 10.

2. Clear the entry and pending

operation.

3. Enter 335 N 9.

4. Complete the calculation.

Press Display

335

T

10

335Û10á

”

(clear the entry)

á

Clear

”

335

U

9

®

Note: the history.

”

clears the screen, but not

335Ü9

335Ü9Ú 326

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

43

Correcting entry errors using

w

1. Enter 1569 + 3.

2. Change the 9 to an 8.

3. Add 3.

4. Complete the calculation.

Press Display

1569

w w w

3

T

3

1569Û3á

8

1568á

1568Û3á

Backspace

w

®

1568Û3Ú 1571

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

44

Mode Menus

Keys

See the tables on the next two pages for details about each mode setting option.

1.

displays the Calculator Mode

‡

menu, from which you can select the following options:

2.

‹ ‡

displays the Problem Solving Mode menu, from which you can select the following options:

Setting Options

3

Setting Options

Division (P)

Constants (Op)

Clear

RESET

2

.

+1 ?

Op 1 Op 2

NY

n/d

Mode

Level of difficulty

Operation

Display option

3.

displays the Fractions menu,

¢

Auto Man

1 2 3

+ – x P ?

11-. 1-.

from which you can select the following options:

Setting Options

Display

Simplify

1

Notes

The examples on the transparency

•

U n/d

Man Auto

n/d

masters assume all default settings.

You must be in Problem Solving

•

3

(‹) to see its menu when you press

. Otherwise, you will see

‡

the Calculator Mode menu.

EXAS INSTRUMENTS INCORPORATED

Press

•

Calculator Mode menu, ‹

to display the

‡

display the Problem Solving Mode menu, or

to display the

¢

Fractions Mode menu. Press after you make your selection, then press

‡

or

again to exit

¢

the menu.

TI-15: A Guide for Teachers

to

®

45

Mode Menus

(Continued)

Calculator Mode Menu

Setting Option Explanation Example

3

Division (Þ)

Constant Operations (OP)

Clear OP1 When selected, clears Op1

Reset N No; does not reset the calculator.

.

n/d Displays division results as a fraction

+1 Shows the constant operation on the

? Hides the constant operation

OP2 When selected, clears Op2

Y Yes; resets the calculator.

Displays division results as a decimal .75

display

1x5 15

15

Problem Solving Mode Menu

Setting SubMenu Option Example

3 4

Auto

Manual Display option

EXAS INSTRUMENTS INCORPORATED

Level of difficulty

Operation

(for Problem Solving Place Value only)

1 2 3

+ – x P ?

divide, find the operation)

(Displays the number of ones,

11-.

tens, hundreds, or thousands)

(Displays the digit that is in the

1-.

ones, tens, hundreds, or thousands place)

(add, subtract, multiply,

TI-15: A Guide for Teachers

1234 For ‘: 12_ _

1234 For ‘: _ 2 _ _

46

Mode Menus

(Continued)

3

Fractions Menu

Setting Option Explanation Example

Display

Simplify Man Allows manual simplification

U n/d

n/d Displays results as improper fractions

Displays results as mixed numbers

1

7

4

6

=

8

3 4

Auto Automatically simplifies to most

reduced form of fraction

3 4

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

47

Basic Operations

4

Keys

1.

2.

3.

4.

adds.

T

subtracts.

U

multiplies.

V

divides. The result may be

W

displayed as a decimal or fraction depending on the mode setting you have selected.

5.

divides a whole number by a

£

whole number and displays the result as a quotient and remainder.

6.

completes the operation.

®

7. M lets you enter a negative number.

Notes

The examples on the transparency

•

masters assume all default settings.

The result of Integer Divide

•

£

always appears as quotient and remainder (__ r __).

The maximum number of digits for

•

quotient or remainder (r) is 5. Quotient, remainder, and the character cannot total more than 10 characters.

If you use the result of integer

•

division in another calculation, only the quotient is used. The remainder is dropped.

All numbers used with

•

£

must

be positive whole numbers.

r

If you attempt to divide by 0, an

•

error message is displayed.

T, U, V, W, ®

•

, and

£

work

with the built-in constants.

5

4

3

2

1

6

7

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

48

Basic operations

Add, Subtract

2 + 54 N 6 =

Press Display

2 6

3 x 4 P 2 =

Press Display

54

T

®

U

2Û54Ü6Ú 50

T U

Multiply, Divide

V W

Equals

®

3

V

®

4

EXAS INSTRUMENTS INCORPORATED

W

2

3Ý4P2Ú 6

TI-15: A Guide for Teachers

49

Entering negative numbers

The temperature in Utah was N3° C at 6:00 a.m. By 10:00 a.m., the temperature had risen 12° C. What was the temperature at 10:00 a.m.?

Press Display

M

3

T

12

3Û12Ú 9

Ü

®

Negative

M

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

50

Division with remainders

Chris has 27 pieces of gum. He wants to share the pieces evenly among himself and 5 friends. How many pieces will each person get? How many pieces will be left over?

Press Display

27

£

6

27Þ

6Ú 4½3

®

Integer Divide

£

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

51

Division with decimal result

Set the division display option to decimal and divide 27 by 6.

Press Display

‡ ®

Ù

ê

»Ä¸

/

‡

á

27

W

6

®

27Þ

6 Ú 4Ù5

Divide

W

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

52

Division with fractional result

ê

Set the division display option to fraction and divide 27 by 6.

Press Display

‡" ®

»Ä¸ êêêê

n

P

d

n

P

d

/

‡

27

W

6

®

Ù

á

27Þ

6Ú 4 êê

Divide

W

5

¤®

10

n

P

d

5 1

4 êêê

4êê

10 2

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

53

Calculating equivalent units of time

Sara ran 2 kilometers in 450 seconds. Convert her time to minutes and seconds.

450 seconds = ? minutes

? seconds

Press Display

450

£

60

450Þ60Ú 7½30

®

Integer Divide

£

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

54

Constant Operations

Keys

5

1.

lets you define or execute

›

operation 1.

2.

lets you define or execute

œ

constant operation 2.

Notes

The examples on the transparency

•

masters assume all default settings.

The constant memory is set in

•

conjunction with › and

œ

when

you perform a calculation that uses

T, U, V, W, £

, and ¨.

The constant function works with

•

whole numbers, decimals, and fractions.

When you use › or

•

œ

, a counter appears at the left and the total appears on the second line at the right of the display. The counter shows how many times the constant has been repeated. If the number at the right exceeds 6 digits, the counter will not be shown. The counter returns to 0 after it reaches 99.

When you use

•

£

with the constant function, subsequent calculations are performed with the quotient portion of the result. The remainder is dropped.

You can clear a stored constant

•

by resetting the calculator (pressing − and

”

simultaneously) or by pressing

1

2

, pressing $ to scroll to the

‡

CLEAR menu, selecting OP1 (or OP2) and pressing

by itself does not clear the

−

. Pressing

®

constant function.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

55

Addition as “counting on”

There are 4 frogs in a pond. If 3 more frogs jump into the pond 1 at a time, how many frogs will be in the pond?

Press Display

› T

(stores

1

›

Û1

Op1

Constant

Operations

›

Add

T

operation)

4

(initialize u s i n g 4 )

›

(add 1 one at a time)

›

Op1

4

Op1

4Û1 15

Op1

5Û1 26

Op1

6Û1 37

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

56

Multiplication as “repeated addition”

Maria put new tile in her kitchen. She made 4 rows with 5 tiles in each row. Use repeated addition to find how many tiles she used. Before you begin, set the calculator to hide the constant operation.

Constant

Operations

›

Press Display

‡$"

(hide constant operation)

+1 Ã

‡

á+

› T

(store the operation)

5

›

Û5

Op1

0

0á

(initialize u s i n g 0 )

êê

¼Á

Continued

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

57

Multiplication as “repeated addition”

Continued

Press Display

›

Op1

1 5

Op1

210

Op1

315

Op1

420

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

58

Powers as “repeated multiplication”

Use this formula and repeated multiplication to find the volume of a cube with a base of 5 meters.

V = l x w x h = 5 x 5 x 5 = 5

Press Display

› V

(store the

5

›

Ý5

Op1

3

Constant

Operations

›

Multiply

V

operation)

1

(initialize u s i n g 1 )

›

Op1

1á

Op1

1Ý5 15

Op1

5Ý5 225

Op1

25Ý5 3 125

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

59

Using

¨

as a constant

Use this formula to find the volume of each cube.

V = base

Press Display

› ¨

3

›

3

Op1

É3

Constant

Operations

›

Powers

¨

2

3

4

›

Op1

2É3 18

Op1

›

3É3 127

Op1

›

4É3 164

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

60

Using OP 1 and OP 2 together

Ming received 5 stickers for each household job she completed. She gave her brother 2 stickers for helping with each job. If they completed 3 jobs, how many stickers does she have?

Press Display

› T

œ U

5

2

›

Û5

Ü2

Op1

Op1 Op2

œ

0

Op1 Op2

0á

Constant

Operations

›

œ

›

œ

›œ

EXAS INSTRUMENTS INCORPORATED

Op1 Op2

0Û5 15

Op1 Op2

5Ü2 13

Op1 Op2

8Ü2 16

Op1 Op2

11Ü2 19

TI-15: A Guide for Teachers

61

Clearing constant operations

Before entering a new operation in OP1 or OP2, you must clear the current values.

Press Display

‡

Ù ßÄ¸

ê

Þ

$ $

¼ÁÏ ¼Á2 êêêê

ç

Mode Menu

‡

®

(clears OP1)

¼ÁÏ ¼Á2 êêêê

" ®

(clears OP2)

¼ÁÏ ¼Á2

‡

(exits Mode menu)

Note: Pressing constant operations.

á

”

does not clear

ç

êêêê

ç

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

62

Whole Numbers and Decimals

7

Keys

6

1.

2.

enters a decimal point.

r

sets the number of decimal

Š

places in conjunction with the Place Value keys (3 through 9 on the illustration below). Only the displayed result is rounded; the internally stored value is not rounded. The calculated value is padded with trailing zeros if needed.

3.

Š 

rounds results to the

nearest thousand.

4. Š

rounds results to the

‘

nearest hundred.

5. Š ’ rounds results to the nearest ten.

6. Š “ rounds results to the nearest one.

7.

Š ˜

rounds results to the

nearest tenth.

8. Š

rounds results to the

™

nearest hundredth.

9. Š

rounds results to the

š

nearest thousandth.

Š r

removes the fixed-decimal

setting.

You must press Š before a Place Value key each time you want to change the number of places for rounding.

Notes

The examples on the transparency

•

masters assume all default settings.

The calculator automatically

•

2

3

4

5

6

1

8

9

EXAS INSTRUMENTS INCORPORATED

rounds the result to the number of decimal places selected. (Only the displayed value is rounded. The internally stored value is not rounded.)

TI-15: A Guide for Teachers

63

Whole Numbers and Decimals

6

Notes

•

(Continued)

All results are displayed to the fixed setting until you either clear the setting by pressing Š r or reset the calculator.

You can set 0 through 3 decimal places.

If students are puzzled when they round .555 to the nearest whole number, for example, and the result is 1, you may need to remind them of the rules of rounding.

You can use r to enter decimal numbers regardless of the fixed decimal setting.

You must press takes effect.

®

before FIX

You can apply the FIX setting to an

•

individual value or to the result of an operation.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

64

Setting the number of decimal places

Round 12.345 to the hundredth’s place, the tenth’s place, the thousandth’s place, and then cancel the Fix setting.

Press Display

12

®

r

345

12Ù345Ú

12Ù345

Fix

Š ™

12Ù345Ú 12Ù35

Fix

Š ˜

12Ù345Ú 12Ù3

Fix decimal

Š

Š š

To cancel Fix:

Š r

Fix

12Ù345Ú

12Ù345

12Ù345Ú

12Ù345

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

65

Addition with money

José bought ice cream for $3.50, cookies for $2.75, and a large soda for $.99. How much did he spend?

Press Display

Fix

Š ™ ®

3 2

r

50

r

75

r

99

T

®

3Ù50Û2Ù75 ÛÙ99Ú 7Ù24

Fix

Fix decimal

Š

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

66

Converting decimals to fractions

Convert the decimal .5 to a fraction, and then view the decimal again after the conversion.

Press Display

5

r

Ÿ

®

ÙÓÚ ØÙÓ

n

N

&

d

D

ÙÓÚ Ó

ííí

ÏØ

Fix decimal

Š

Ÿ

(Return to decimal)

ØÙÓ

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

67

Memory

Keys

7

1.

z

z ®

z T

z U

z V

z W

functions as shown below:

Stores displayed value over value in memory. Adds displayed value to memory. Subtracts displayed value from value in memory.

Multiplies displayed

value by value in memory. Divides value in memory by the displayed value.

z £

Performs integer division on value in memory using the displayed value. Only the quotient is stored and displayed.

2.

|

recalls the contents of memory to the display. When pressed twice, it clears the memory.

Notes

The examples on the transparency

•

masters assume all default settings.

Results are stored to memory and

•

not displayed. The display remains the same.

You can store integers, fractions,

•

and decimals in memory.

EXAS INSTRUMENTS INCORPORATED

•

is displayed anytime a value

M

other than 0 is in memory.

To clear the memory, press

•

|

twice.

1

2

TI-15: A Guide for Teachers

68

Using memory to add products

Hamburgers 2 $1.19 = Milk shakes 3 $1.25 = Coupon for each

milk shake 3 $.20 =

Total cost =

Press Display

2

V

1

r

19

2Ý1Ù19Ú 2Ù38

®

z ®

M

2Ý1Ù19Ú 2Ù38

3

V

1

r

25

3Ý1Ù25Ú 3Ù75

M

®

Store to Memory

z

Memory Recall

|

z T

Add milk shakes

(

to memory.)

3

V r

20

®

z U

Deduct coupon

(

from memory.)

|

Recall the total

(

cost.)

EXAS INSTRUMENTS INCORPORATED

M

3Ý1Ù25Ú 3Ù75

M

3ÝÙ20Ú 0Ù6

M

3ÝÙ20Ú 0Ù6

M

5Ù53

TI-15: A Guide for Teachers

69

Using memory to find averages

Dai has test scores of 96 and 85. He has weekly scores of 87 and 98. Find the average for each group of scores and the average of his averages together.

Press Display

96

T

85

96Û85Ú 181

®

2

W

®

181Þ2Ú 90Ù5

Store to Memory

z

Add

T

Memory Recall

|

z ®

87

T

98

®

2

W

®

T | ®

2

W

®

M

181Þ2Ú 90Ù5

M

87Û98Ú 185

M

185Þ2Ú 92Ù5

M

92Ù5Û90Ù5Ú

183

M

183Þ2Ú 91Ù5

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

70

Fractions

Keys

8

1.

displays a menu of mode

¢

settings from which you can select how the fraction results will be displayed. You select 2 items.

U n/d

(default) displays mixed

number results.

displays fraction results.

n/d

(default) displays unsimplified

Man

fraction results so you can simplify them manually (step-by-step).

Auto

displays fraction results

simplified to lowest terms.

2.

lets you enter the whole-



number part of a mixed number.

3.

lets you enter the numerator of



a fraction.

4. ¥ lets you enter the denominator of a fraction.

5.

¦

changes a mixed number to

a fraction and vice versa.

6.

simplifies a fraction using the

¤

lowest common prime factor. If you want to choose the factor (instead of letting the calculator choose it), press integer), and then press

, enter the factor (an

¤

®

. You must be in Manual mode to use this function.

7.

changes a fraction to its

Ÿ

decimal equivalent and vice versa.

3

2

6

4

EXAS INSTRUMENTS INCORPORATED

8.

displays the factor (divisor)

§

used to simplify the last fraction result. You must be in Manual

5

1

8

7

mode to use this function.

Notes

The examples on the transparency

•

masters assume all default settings.

TI-15: A Guide for Teachers

71

Fractions

Notes (continued)

8

Dividing a fraction by a fraction

•

gives fractional results regardless of the division setting (decimal or fraction).

•

The

mode settings provide 4

¢

possible display options for computational results displayed in fraction form. For example, for 6 ÷ 4, the displays would look like this:

manual simp/improper (n/d):

auto simp/improper (n/d):

manual simp/mixed number:

(U n/d)

auto simp/mixed number:

When you multiply or divide

•

fractions and decimals, the result is displayed as a decimal. A decimal cannot be converted to a fraction if the result would overflow the display.

Clearing with w in fractions

•

occurs from right bottom to left top. If you accidentally press

¥

(the denominator key) after entering the numerator, without

6 4 3

2 2

1

4

1

2

entering a numeral for the denominator first, using w will not correct that error. You will need to clear and begin the entry again.

If the decimal place is set to 0, the

•

decimal equivalent for a fraction will not be displayed.

(U n/d)

You can enter the denominator or

•

numerator first. For operations, you can enter 1 to

•

1000 for the denominator. For conversions to decimal, you can enter 1 to 100,000,000 for the denominator.

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

72

Adding mixed numbers

r

3

A baby girl weighed 4

8

pounds at

/

birth. In the next 6 months, she

3

gains 2

4

pounds. How much does

/

she weigh?

Press Display

4 3

 

T

8

¥

3

ííí

4

8

Û

Numerator Key



Denominator Key

¥

Unit Key



Mixed to Imprope

Conversion

¦

2



4

¥ ®

¦

EXAS INSTRUMENTS INCORPORATED

3



3 3 1

+

84 8

=

íííííí

2

ííí

74

57

ннннн

8

TI-15: A Guide for Teachers

73

Simplifying fractions

Method 1: The calculator chooses a common factor

18

Simplify

.

24

Press Display

18

(Enter the fraction.)



24

18

ннннн

24

¤

18 S

(Prepare to simplify.)

®

(Simplify the fraction.)

ннннн

à¾á

24

N

n

&

D

d

18 9

ннннн

à¾

íííí

24 12

Numerator Key



Denominator Key

¥

Simplify

¤

Factorial

§

(Optional: Check factor. You must be in Manual mode.)

§

(Return to the fraction.)

¤ ®

(Continue simplifying.)

EXAS INSTRUMENTS INCORPORATED

2

N

n

&

D

d

9

íííí

12

9 3

íííí

à¾

ííí

12 4

TI-15: A Guide for Teachers

74

Simplifying fractions

Method 2: You choose a common factor

18

Simplify

.

24

Press Display

18

(Enter the fraction.)



24

18

íííí

24

á

¤

18

íííí

Prepare to

(

simply.)

à¾

24

6

18

íííí

(Enter a common factor.)

à¾Ôá

24

Numerator Key



Denominator Key

¥

Simplify

¤

®

(Simplify the fraction.)

EXAS INSTRUMENTS INCORPORATED

18 3

à¾Ô

24 4

ííííííí

TI-15: A Guide for Teachers

75

Converting fractions to decimals

5

Convert the fraction

10

to a

decimal, and then view the original

Numerator Key

fraction after the conversion.

Press Display

5



10

®

55

íííí

Ú

10 10

Ÿ

(Return to fraction.)

N D

&

n d

íííí

0Ù5

n d

íííí

10



Denominator Key

¥

Fraction to

Decimal

Ÿ

5

(Return

Ÿ

to decimal.)

EXAS INSTRUMENTS INCORPORATED

0Ù5

TI-15: A Guide for Teachers

76

Converting decimals to fractions

Convert the decimal .5 to a fraction, and then view the decimal again after the conversion.

Press Display

5

r

Ÿ

®

(Return

Ù5Ú 0Ù5

N

n

&

D

d

Ù5Ú 5

íííí

10

Numerator Key



Denominator Key

¥

Fraction to

Decimal

Ÿ

to decimal.)

0Ù5

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

77

Converting between fractions and mixed numbers

6

Convert the improper fraction

4

to

Numerator Key

a mixed number.



Denominator Key

Press Display

¥

¢

¿ »Ä¸ »Д¸ кккккк

Fraction Modes

$

¢

6



®

¤ ®

¦

4

èæ êêêê

62

Ú

44

2 1

ííí

1

à¾

4 2

1

ííííííí

íííí

3

íííí

2

¢

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

78

Comparing fractions and decimals

Linda swims 20 laps in 5.72 minutes. Juan swims 20 laps in 5¾ minutes. Who swims faster? Compare the time as decimals and fractions.

To compare the times as decimals:

Press Display

5 4

 ®

3



33

=

ííí

5

ííí

5

44

Ÿ

Numerator Key



Denominator Key

¥

5Ù75

N

n

&

D

5

d

нннннн

Ÿ

75

100

Continue to compare as fractions:

5

Ÿ

r

72

®

5Ù72Ú 5Ù72

N

n

&

D

d

72

ннннн

5

100

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

79

Percent

9

Keys

1.

y

2.

ª

converts to a percent.

enters a percent.

Notes

The examples on the transparency

•

masters assume all default settings.

1

2

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

80

Converting with percent

Convert 25% to a decimal.

Press Display

25

ª ®

25ãÚ 0Ù25

25

Convert

to a percent.

100

Press Display

25

¥ y ®



100

25

íííí

100

àã 25ã

Percent

ª

Convert to

Percent

y

Convert 3 to a percent.

Press Display

3

y ®

3àã 300ã

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

81

Converting with fractions, decimals, and percent

Convert 25% to a fraction, simplify to lowest terms, and then convert the fraction to a decimal.

Press Display

25

ª ®

25ãÚ 0Ù25

N/D"n/d

Ÿ

25

нннннн

100

N/D"n/d

¤ ®

25 5

нннннн

à¾

100 20

íííí

Percent

ª

Fraction to

Decimal

Ÿ

¤ ®

Ÿ

y

N/D"n/d

5

ííí

à

¾

20 4

0Ù25

0Ù25àã 25ã

1

ííí

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

82

Calculating tips

The Chen family went to a restaurant for dinner. Their bill was $31.67. How much was the tip if they left 15% of their bill? How much was the total including the tip?

Press Display

31.67

®

31Ù67Ú 31Ù67

Fix

Š ™

31Ù67Ú 31Ù67

Percent

ª

Convert to

Percent

y

15

V ®

31.67

4.75

®

ª

Fix

31Ù67Ý15ãÚ

4Ù75

T

31Ù67Û4Ù75Ú

36Ù42

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

83

Pi

10

Keys

1.

©

enters p.

Notes

The examples on the transparency

•

masters assume all default settings.

Internally, pi is stored to 13 digits

•

(3.141592653590). Only 9 decimal places are displayed.

To convert from p to a decimal

•

value, press places are displayed.

. Nine decimal

Ÿ

1

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

84

Using pi to find circumference

Use this formula to find the amount of border you need to buy if you want to put a circular border around a tree at a distance of 3 meters from the tree.

C = 2pr = 2 x p x 3

Press Display

Pi

©

2

V ©

3

V

Ÿ

2Ýß

®

2ÝßÝ3Ú Ôß

18Ù84955592

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

85

Using pi to find area

Use this formula to find how much of the lawn would be covered by a sprinkler with a radius of 12 meters.

A = pr

2 =

p

x 12

2

12 m

Pi

©

Press Display

© V

ßÝ

12

¨

2

ЯЭПРЙР

®

ЯЭПРЙРЪS 144Я

Ÿ

452Ù3893421

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

86

Using pi to find volume

Use this formula to find how much space a ball occupies.

3

p

r

4

V =

3

5 cm

Pi

©

Press Display

4

V © V

ÒÝßÝ

5

W

¨

3

ТЭЯЭУЙС

ТЭЯЭУЙСЮС

®

ТЭЯЭУЙСЮСЪ

523Ù5987756

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

87

Powers and Square Roots

11

Keys

1.

2.

lets you specify a power for the

¨

value entered. When you press the value is displayed if it is within the range of the calculator.

calculates the square root of

¬

positive values, including fractions.

®

,

Notes

The examples on the transparency

•

masters assume all default settings.

2

EXAS INSTRUMENTS INCORPORATED

1

TI-15: A Guide for Teachers

88

Finding the area of a square

Use this formula to find the size of the tarpaulin needed to cover the entire baseball infield.

A = x

2

= 90

2

Powers

¨

Press Display

90

¨

2

ЧШЙРЪ 8100

®

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

89

Finding the square root

Use this formula to find the length of the side of a square clubhouse if 36 square meters of carpet would cover the floor.

L =

x = 36

36 m

of carpet

2

Square Root

¬

Press Display

¬

36

Y

âÅ36ÆÚ 6

®

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

90

Calculating powers

Fold a piece of paper in half, in half again, and so on until it is not possible to physically fold it in half again. How many sections are there after ten folds?

Press Display

Powers

¨

2

¨

10

®

2ÉÏØÚ 1024

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

91

Calculating negative powers

Find the standard numerals for the following powers:

-3

=

2

-3

-2 .2

(1/2)

Press Display

2

¨ M

3

=

-3

=

-3

=

2ÉÜÑÚ ШЩПРУ

®

Powers

¨

Negative

M

2

M

3

M

2

r ®

1



3

®

¨

®

¨ M

2

¨ M

3

Ü

2ЙЬСЪ ЬШЩПРУ

Ù2ÉÜÑÚ ÏÐÓ

1. ê ÉÜÑÚ Ö

2

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

92

Using powers of 10

1.3 x 103 = ?

Press Display

Powers

¨

1

r

10

1

r

10

®

3

¨

3

V

3

V

¨ M

®

1.3 x 10

3

1Щ3ЭПШЙСЪ

1300

3

L

= ?

1Щ3ЭПШЙЬСЪ

0Ù0013

EXAS INSTRUMENTS INCORPORATED

TI-15: A Guide for Teachers

93

Texas instruments TI-15 A Guide for Teachers

Specifications and Main Features

Frequently Asked Questions

User Manual

Table of Contents

About the Teacher Guide

About the TI.15

Activities

Patterns in Percent

Fraction Forms

Comparing Costs

Number Shorthand: Scientific Notation

Related Procedures

In the Range

The Value of Place Value

What’s the Problem?

Display, Scrolling, Order of Operations, and Parentheses

Equation Operating System

Order of Operations

Clearing entries

Mode Menus

Basic Operations

Entering negative numbers

Division with remainders

Division with decimal result

Division with fractional result

Calculating equivalent units of time

Constant Operations

Addition as “counting on”

Multiplication as “repeated addition”

Powers as “repeated multiplication”

Using OP 1 and OP 2 together

Clearing constant operations

Whole Numbers and Decimals

Setting the number of decimal places

Addition with money

Converting decimals to fractions

Memory

Using memory to add products

Using memory to find averages

Fractions

Adding mixed numbers

Simplifying fractions

Converting fractions to decimals

Converting decimals to fractions

Converting between fractions and mixed numbers

Comparing fractions and decimals

Percent

Converting with percent

Converting with fractions, decimals, and percent

Calculating tips

Pi

Using pi to find circumference

Using pi to find area

Using pi to find volume

Powers and Square Roots

Finding the area of a square

Finding the square root

Calculating powers

Calculating negative powers

Using powers of 10