3B Scientific Oscillation Tube User Manual [en, de, es, fr, it]

Page 1

3B SCIENTIFIC® PHYSICS

U14328 Präzisionsröhre

Bedienungsanleitung

9/03 ALF

Die Präzisionsröhre dient in Verbindung mit der Mariotte’schen Flasche U14327 zur Bestimmung des Adiabaten-Exponenten cP/cV nach Rüchardt.

1. Sicherheitshinweise

• Glasröhre vorsichtig handhaben. Keinen mechanischen Belastungen aussetzen. Bruchgefahr!

• Auf extreme Sauberkeit sowohl der Glasröhre als auch des Aluminiumzylinders achten, da schon geringste Verunreinigungen zu erhöhter Reibung führen.

• Aluminiumzylinder nicht fallen lassen. Schon geringste Verformung beeinträchtigt den Versuch.

2. Beschreibung, technische Daten

Präzisionsglasrohr bei Lieferung an beiden Enden mit Gummistopfen verschlossen und genau eingepasstem Aluminiumzylinder. Lässt man den Zylinder in das unten geschlossene senkrecht gehaltene Glasrohr gleiten, so sinkt er langsam nach unten, weil die Luft nur sehr langsam durch den engen Spalt zwischen Zylinder und Glaswand dringen kann. Dreht man das Präzisionsrohr um 180°, so verhält sich der Zylinder entsprechend, da im oberen Teil der Glasröhre beim Absinken ein Unterdruck entsteht und die Luft nur sehr langsam eindringen kann. Im dritten Fall lässt man den Zylinder in das offene Rohr fallen und verschließt es sofort. Der Zylinder wird abgebremst und schwingt einige Male auf und ab.

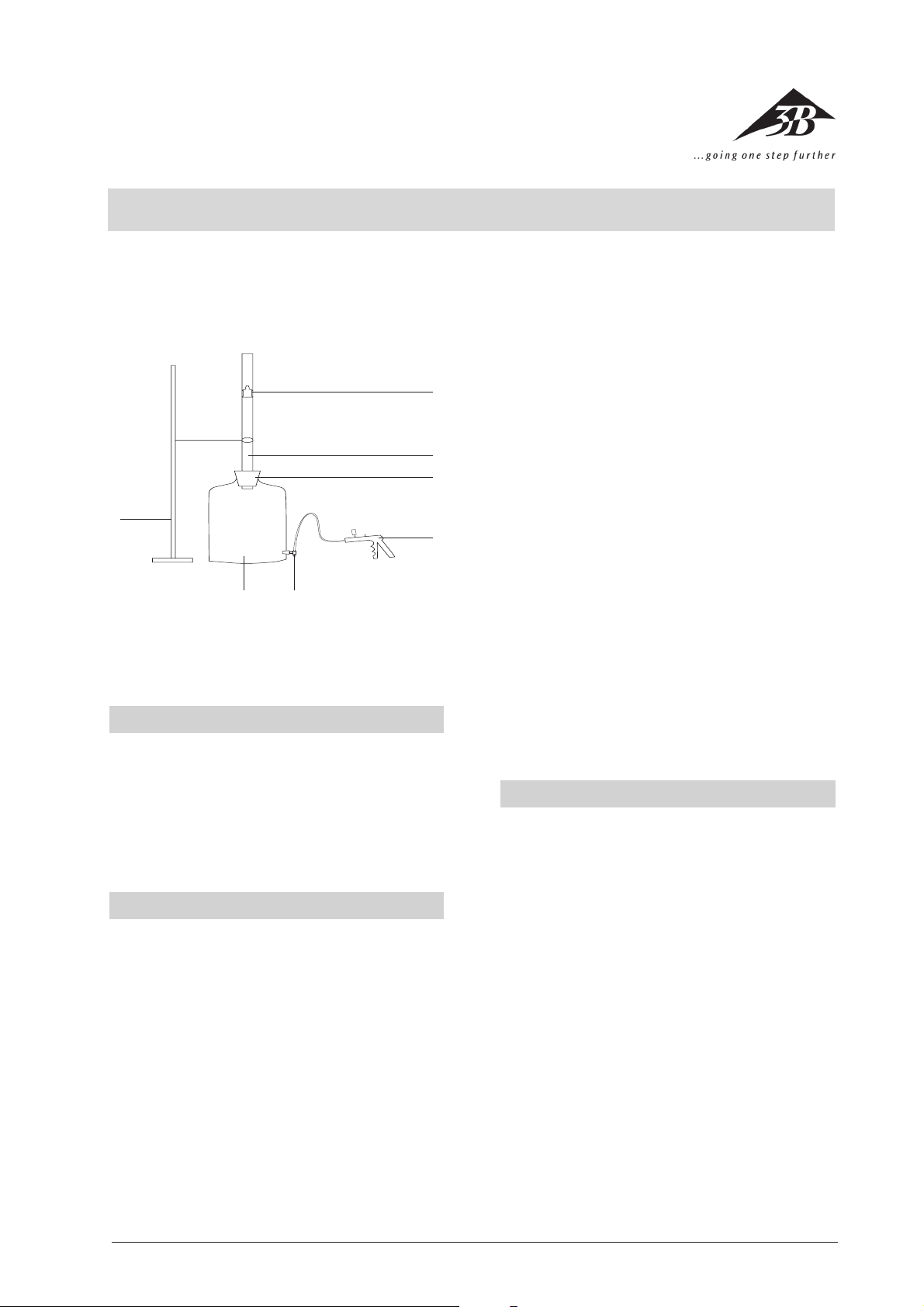
1 Stativ

2 Mariotte’sche Flasche

3 3-Wege-Hahn

4 Handpumpe

5 Stopfen

6 Präzisionsröhre

7 Aluminiumzylinder

Abmessungen: 600 mm x 16 mm Ø Aluminiumzylinder: 15,2 g

2.1 Lieferumfang

1 Präzisionsglasrohr 2 Gummistopfen 1 Aluminiumzylinder

3. Theoretische Grundlagen

Verwendete Formelzeichen:

m: Masse des Aluminiumzylinders d: Innendurchmesser des Präzisionsrohres A: Querschnittsfläche des Präzisionsrohres V: Volumen des Messgefäßes pL: Luftdruck p: Druck in der Flasche g: Erdbeschleunigung n: Molzahl R: universelle Gaskonstante (8,31451 kJ/kmol K) T: Temperatur Ts: Schwingungsdauer t: Zeit cp: spez. Wärme bei konstantem Druck cV: spez. Wärme bei konstantem Volumen

?: cP/c M: Eigenkreisfrequenz

Der Zustand einer abgeschlossenen Menge eines idealen Gases lässt sich durch die Zustandsgrößen Druck p, Volu-

innen

Page 2

men V und Temperatur T eindeutig beschreiben. Es gilt:

464

ApTmVTdp

p V = n R T (1)

führt zur periodischen Beschleunigung des Zylinders mit der Masse m. Nach dem zweiten Newtonschen Axiom gilt folgende Differentialgleichung für s(t)

Für Zustandsänderungen ohne Wärmeaustausch mit der Umgebung kann diese Gleichung in die Adiabatengleichung umgeformt werden:

p V? = const. (2)

Der Adiabatenexponent ? ist das Verhältnis der spezifischen Wärmekapazität bei konstantem Druck cp und der spezifischen Wärmekapazität bei konstantem Volumen

cV:

(3)

Setzt man die Glasröhre senkrecht in den durchbohrten Gummistopfen einer Glasflasche mit 10 l Volumen und lässt den Aluminiumzylinder in die Glasröhre gleiten, so führt er harmonische Schwingungen auf dem durch das abgeschlossene Luftvolumen gebildeten Luftpolster aus. Wenn der Druck p in der Flasche gleich der Summe aus dem durch die Zylindermasse m hervorgerufenen Druck und dem äußeren Luftdruck pL ist, befindet sich der Zylinder im Gleichgewicht:

dsdtpA

(8)

Aus (8) ergibt sich die Eigenkreisfrequenz M der harmonischen Schwingung

(9)

und daraus die Schwingungsdauer T

(10)

Für die Bestimmung des Adiabaten- Exponenten ? folgt:

(11)

4. Bedienung

(4)

Wird der Zylinder um die Strecke s aus der Gleichgewichtslage ausgelenkt, so ändert sich p um ,p und V um ,V. Der Aluminiumzylinder erfährt eine Rückstellkraft, die proportional zur Auslenkung ist. Er führt auf dem unter ihm befindlichen Luftpolster harmonische Schwingungen aus. Da der Schwingungsvorgang relativ rasch abläuft, kann man ihn durch die adiabatische Zustandsänderung beschreiben. Durch Bilden der Ableitung dp/dV von (2) und Übergang zu endlichen Änderungen ,p und ,V erhält man

∆∆

V=−

(5)

Da der Zylinder sich um die Strecke s in der Präzisionsröhre bewegt, beträgt die Änderung des Volumens

,V = As (6)

Die rücktreibende Kraft

FAp

==−

∆χ

(7)

• Luftdruck, Innendurchmesser des Präzisionsrohres, Masse des Aluminiumzylinders und Volumen des Messgefäßes bestimmen.

• Glasröhre auf die Mariotte’sche Flasche aufsetzen, senkrecht ausrichten und an einem Stativ befestigen.

• Die Mariotte’sche Flasche sollte mit einer Gummimatte oder ähnlichem ausgelegt sein, um eine Beschädigung sowohl der Flasche als auch des Zylinders zu verhindern, wenn der Zylinder in die Flasche fällt.

• Zur Vereinfachung des Versuchs ist es zu empfehlen eine Handpumpe über den 3-Wege-Hahn an die Mariotte’sche Flasche anzuschließen. Der Aluminiumzylinder kann auf diese Weise im Glasrohr hochgepumpt und entnommen werden, ohne das Glasrohr immer wieder neu ausrichten zu müssen.

• Den Aluminiumzylinder mit einem fusselfreiem Tuch und etwas Waschbenzin säubern und bei geschlossenem Hahn nicht verkantet in die Glasröhre einführen und fallen lassen. Den Zylinder nur am Handgriff anfassen, um Verunreinigungen zu vermeiden.

• Mit einer Stoppuhr die Zeit für fünf Schwingungen 10 mal messen.

• Die Zeitmessung ist zu starten, wenn der Zylinder zum ersten Mal abgebremst wird und sich an der tiefsten Stelle befindet. Gestoppt wird die Zeitmessung, wenn der Zylinder zum sechsten Mal am tiefsten Punkt angekommen ist.

Page 3

• Mittels der Handpumpe bei geöffnetem Hahn, den Zylinder nach oben pumpen. Dabei darauf achten, dass der Zylinder nicht herausfällt und beschädigt wird.

• Den Zylinder ganz aus dem Rohr entnehmen und so den herrschenden Luftdruck im System wieder herstellen. Hahn wieder schließen.

• Weitere neun Messungen durchführen und den Durchschnittswert für die Schwingungsdauer ermitteln.

• Berechnung durchführen.

Allgemeine Hinweise:

Die Qualität der Messungen hängt stark von folgenden Bedingungen ab:

• Die Präzisionsröhre muss extrem sauber sein. Gegebenenfalls das Glasrohr mit Seidenpapier reinigen.

• Der Aluminiumzylinder muss ebenfalls extrem sauber sein. Schon geringste Verunreinigungen wie Hautfettablagerungen führen zu starker Reibung. Den Zylinder deshalb vor jeder Messung mit einem fusselfreiem Tuch und etwas Waschbenzin säubern.

• Geringste Verformung des Zylinders (z.B. verursacht durch Fallenlassen) führen zu Beeinträchtigungen.

• Die Glasröhre muss senkrecht ausgerichtet sein.

• Alle Stopfen müssen luftdicht sein.

• Da die Schwingungsdauer quadratisch in das Ergebnis

einfließt, muss die Zeit sorgfältig genommen werden.

5. Messbeispiel

Volumen V: 10400 cm³ Masse des Zylinders m: 15,2 g Ø

des Rohres d: 16 mm

Innen

Luftdruck pL: 1018 mbar

Zeit t in Sekunden für 5 Schwingungen:

5,172 5,276 5,259 5,224 5,305 5,175 5,231 5,241 5,191 5,175

Summe: 52,249

Mittelwert: 5,2249

Schwingungsdauer Ts: 1,04498 s

Nach Einsetzen in die Formel (11) erhält man:

? = 1,39

Literaturwert:

? = 1,40

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Deutschland • www.3bscientific.com • Technische Änderungen vorbehalten

Page 4

3B SCIENTIFIC® PHYSICS

U14328 Precision tube

Instruction sheet

9/03 ALF

The precision tube is used together with the Marriott bottle U14327 to determine the adiabatic exponent cP/c using Rüchardt’s method.

1. Safety instructions

• Handle the glass tube carefully. Do not subject to mechanical stress or it may break.

• Make sure both glass tube and aluminium cylinder are thoroughly clean because even the slightest amount of dirt leads to increased friction.

• Do not drop the aluminium cylinder. Even the slightest deformation adversely affects the experiment.

2. Description, technical data

A precision glass tube supplied with rubber stoppers at both ends and with an aluminium cylinder precisely engineered to slip inside. If the glass tube is held vertical with the bottom end closed and the aluminium cylinder is allowed to slide down inside it, the cylinder drops very slowly since there is only a tiny gap around the sides for air to escape from underneath. If the tube is rotated by 180°, the cylinder behaves in the same manner since the pressure in the upper part of the tube is reduced as the cylinder slides down, and air can only get in to fill the space very slowly. In the third situation, the cylinder is allowed to drop into an open tube which is then immediately stoppered. The cylinder then slows and oscillates up and down a few times.

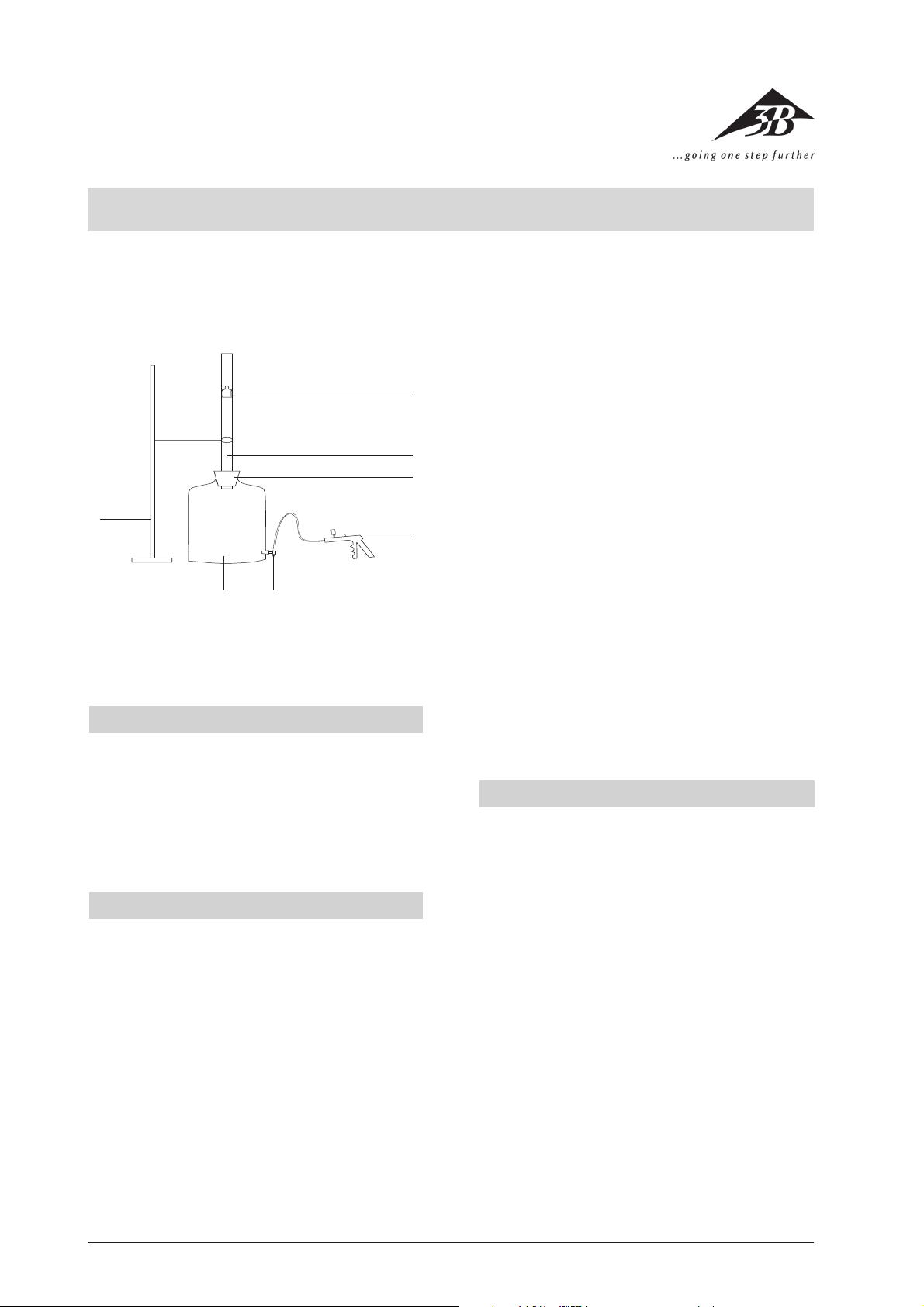
1 Stand

2 Marriott bottle

3 3-way stopcock

4 Hand pump

5 Stoppers

6 Precision tube

7 Aluminium cylinder

Dimensions: 600 mm x 16 mm Ø

Aluminium cylinder: 15.2 g

2.1 Scope of supply

1 Precision glass tube 2 Rubber stoppers 1 Aluminium cylinder

3. Theory

Symbols used in the formula:

m: Mass of aluminium cylinder d: Inside diameter of precision tube A: Cross-sectional area of precision tube V: Volume of measuring flask pL: Atmospheric pressure p: Pressure in the bottle g: Acceleration due to Earth’s gravity n: Number of moles R: Universal gas constant (8.31451 kJ/kmol K) T: Temperature Ts: Period of oscillation t: Time cp: Specific heat at constant pressure cV: Specific heat at constant volume

?: cP/c M: Natural frequency of oscillation

The state of an enclosed quantity of an ideal gas can be uniquely expressed in terms of the quantities pressure p, volume V and temperature T as follows:

inner

Page 5

p V = n R T (1)

464

ApTmVTdp

For changes in state where no exchange of heat with the environment takes place, this equation can be reduced to the adiabatic formula:

From (8) the natural frequency of oscillation is given by

(9)

p V? = const. (2)

The adiabatic exponent ? is the ratio of the specific heat at constant pressure cp to the specific heat at constant volume cV:

(3)

A stopper with a hole is put into a glass vessel with a volume of 10 l . The precision tube runs through the hole in this stopper so that it is in a vertical position. If the aluminium cylinder is allowed to drop into the tube, it bounces on the cushion of air enclosed inside the apparatus. This leads to a periodic oscillation. When the pressure p in the glass vessel is equal to the sum of the pressure due to the mass of the aluminium cylinder and the external atmospheric pressure, the cylinder is in a state of equilibrium:

(4)

If the cylinder is moved a distance s from its equilibrium position, p changes by the value ,p and V changes by ,V. A force acts on the aluminium cylinder to push it back towards equilibrium. This force is proportional to the distance s. A harmonic oscillation now begins atop the air cushion under the cylinder. Since the oscillation occurs fairly rapidly, it can be described in terms of the adiabatic change of state. By deriving dp/dV from equation (2) and assuming this also applies to the small finite changes ,p and ,V we obtain

∆∆

V=−

(5)

Since the cylinder moves along a distance s in the precision tube, the change in volume is

,V = As (6)

The restoring force

FAp

==−

∆χ

(7)

leads to the periodic acceleration of a cylinder of mass m. Newton’s second law then gives us the following differential equation for s(t)

dsdtpA

(8)

thus the period of the oscillation Ts is

(10)

Therefore, to derive the adiabatic exponent ? the following applies:

(11)

4. Operation

• Determine the atmospheric pressure, the diameter of the inside of the precision tube, the mass of the aluminium cylinder and the volume of the measuring flask.

• Place the glass tube on the Marriott bottle, make sure it is vertical and secure it to a stand.

• The Marriott bottle should have a rubber mat or similar placed inside to avoid damage to the bottle and the cylinder if the cylinder falls into the bottle.

• To simplify the experiment, it is recommended that a hand pump be attached to the Marriott bottle via the 3-way stopcock. The cylinder can then be pumped back up the tube and retrieved from the top so that it is not necessary to repeatedly set the tube up.

• The aluminium cylinder should be cleaned with a fluff-free cloth and some petroleum ether. It should not be introduced into the tube at a crooked angle and allowed to drop when the stopcock is closed. Do not touch the cylinder except by the grip to prevent it getting dirty.

• Measure the duration of 5 oscillations 10 times using a stopwatch.

• The time measurement should start at the point when the cylinder comes to a halt for the first time at its lowest point. The watch should be stopped when the cylinder reaches its lowest point for the sixth time.

• Using the hand pump with the stopcock open, pump the cylinder back to the top. Make sure that the cylinder does not fall out at the end and get damaged.

• Take the cylinder right out of the tube so that the pressure in the apparatus returns to atmospheric pressure. Close the stopcock again.

• Make the measurements another nine times and determine the average value for the time.

• Perform the calculation.

Page 6

General notes:

The accuracy of the measurements depends strongly upon the following factors:

• The precision tube must be extremely clean. If necessary, clean the tube with tissue paper.

• The aluminium cylinder must also be extremely clean. The slightest amount of dirt such as grease from fingers can lead to considerable friction. Therefore the cylinder should be cleaned before each measurement using a fluff-free cloth and some petroleum ether.

• The slightest deformation of the cylinder (e.g. due to having been dropped) adversely affects the experiment.

• The glass tube must be vertical.

• All stoppers must be airtight.

• Since the duration of the oscillation is squared in the

equation, the time does need to be measured as accurately as possible.

Atmospheric pressure pL: 1018 mbar

Time t in seconds for five oscillations:

5.172

5.276

5.259

5.224

5.305

5.175

5.231

5.241

5.191

———

5.175

Total: 52.249

Average: 5.2249

Period of oscillation Ts: 1.04498 s

5. Measurement example

Volume V: 10400 cm³ Mass of cylinder m: 15.2 g Ø

of tube d: 16 mm

inner

Substituting into equation (11) gives:

? = 1.39

From published tables:

? = 1.40

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Germany • www.3bscientific.com • Technical amendments are possible

Page 7

3B SCIENTIFIC® PHYSICS

U14328 Tube de précision

Instructions d‘utilisation

9/03 ALF

En liaison avec le flacon de Mariotte U14327, le tube de précision permet de déterminer l’exposant adiabatique

cP/cV d’après Rüchardt.

1. Consignes de sécurité

• Manipuler le tube en verre avec précaution. Ne pas l’exposer à des charges mécaniques. Risque de cassure !

• Veiller à une propreté rigoureuse tant du tube en verre que du cylindre en aluminium, car les plus petites impuretés peuvent provoquer un frottement excessif.

• Ne pas laisser tomber le cylindre en aluminium. Une très faible déformation suffit déjà pour influencer l’expérience.

2. Description, caractéristiques techniques

A sa livraison, le tube est obturé des deux côtés par des bouchons en caoutchouc et équipé d’un cylindre en aluminium exactement adapté. Lorsqu’on le laisse glisser dans le tube tenu verticalement et fermé par le bas, le cylindre descend lentement, car l’air ne peut pénétrer que très lentement à travers une fente étroite entre le cylindre et la paroi en verre. Si l’on tourne le tube à 180°, le cylindre réagit de même, car une dépression apparaît alors dans la partie supérieure du tube et l’air ne peut pénétrer que très lentement. Dans le troisième cas, on laisse le cylindre tomber dans le tube ouvert pour refermer immédiatement ce dernier. Le cylindre est

1 Pied

2 Flacon de Mariotte

3 Robinet à trois voies

4 Pompe manuelle

5 Bouchon

6 Tube de précision

7 Cylindre en aluminium

freiné et oscille plusieurs fois dans le sens vertical. Dimensions : 600 mm x Ø Cylindre en aluminium : 15,2 g

2.1 Matériel fourni

1 tube de précision en verre 2 bouchons en caoutchouc 1 cylindre en aluminium

3. Notions théoriques

Symboles utilisés dans les formules :

m: Masse du cylindre en aluminium d: Diamètre intérieur du tube de précision A: Surface de section du tube de précision V: Volume du récipient de mesure pL: Pression d’air p: Pression dans la bouteille g: Accélération de la pesanteur n: Indice molaire R: Constante universelle du gaz (8,31451 kJ/kmol K) T: Température Ts: Durée d’une oscillation t: Temps cp: Chaleur spéc. à pression constante c8: Chaleur spéc. à volume constant

?:cP/c M: Fréquence angulaire propre

L’état d’une quantité fermée d’un gaz idéal peut être décrit sans équivoque à l’aide des grandeurs d’état pres-

16 mm

int.

Page 8

sion p, volume V et température T. Règle générale :

464

ApTmVTdp

p V = n R T (1)

entraîne une accélération périodique du cylindre avec la masse m. D’après le deuxième axiome de Newton, l’équa- tion différentielle suivante s’applique à s(t) :

En cas de modification de l’état sans échange thermique avec l’environnement, cette équation peut être transformée dans l’équation adiabatique suivante :

p V? = const. (2)

L’exposant adiabatique ? est le rapport entre la capacité thermique spécifique à pression constante cp et la capacité thermique spécifique à volume constant cV :

(3)

Si l’on place le tube verticalement dans la perforation du bouchon en caoutchouc d’un flacon en verre de 10 l et qu’on fait glisser le cylindre en aluminium dans le tube, il produit des oscillations harmoniques sur le coussin d’air formé par le volume d’air fermé. Si la pression p dans le flacon est égale à la somme de la pression provoquée par la masse du cylindre m et de la pression d’air extérieure pL, le cylindre est en équilibre :

(4)

Si le cylindre est dévié de son équilibre sur une portée s, p est modifié de ,p et V de ,V. Le cylindre en aluminium subit une force de rappel proportionnelle à la déviation. Il produit des oscillations harmoniques sur le coussin d’air qui se trouve sous lui. Comme les oscillations ont lieu relativement rapidement, on peut les décrire par la modification d’état adiabatique. Par la déviation dp/dV de (2) et la transition à des modifications finies ,p et ,V, on obtient

∆∆

V=−

(5)

Comme le cylindre se déplace sur s dans le tube de précision, la modification du volume est

,V = As (6)

La force de rappel

FAp

==−

∆χ

(7)

dsdtpA

(8)

(8) permet d’obtenir la fréquence angulaire propre M de l’oscillation harmonique

(9)

et, à partir de là, la durée de l’oscillation T

(10)

Pour déterminer l’exposant adiabatique ? :

(11)

4. Manipulation

• Déterminer la pression de l’air, le diamètre intérieur du tube de précision, la masse du cylindre en aluminium et le volume du récipient de mesure.

• Placer le tube en verre sur le flacon de Mariotte, l’ajuster verticalement et le fixer sur un pied.

• Le flacon de Mariotte doit être accompagné d’un tapis en caoutchouc ou similaire, pour éviter que ni le flacon ni le cylindre ne soient endommagés si le cylindre tombe dans le flacon.

• Pour simplifier l’expérience, il est recommandé de brancher une pompe manuelle au flacon de Mariotte via un robinet à trois voies. Il est possible ainsi de remonter le cylindre en aluminium dans le tube à l’aide de la pompe et de le retirer, sans être obligé de réajuster à chaque fois le tube.

• Nettoyer le cylindre avec un chiffon non pelucheux et un peu de ligroïne ; ensuite, le robinet étant fermé, introduire le cylindre sans à-coups dans le tube et le laisser glisser. Ne tenir le cylindre qu’à la poignée, pour éviter de le salir.

• A l’aide d’un chronomètre, mesurer dix fois le temps pour cinq oscillations.

• Démarrer la mesure lorsque le cylindre est freiné pour la première fois et se trouve au point le plus bas.

Page 9

Conclure la mesure lorsque le cylindre a passé pour la sixième fois le point le plus bas.

• Avec la pompe manuelle, le robinet étant ouvert, pomper le cylindre vers le haut. Veiller à ne pas faire tomber le cylindre pour ne pas l’endommager.

• Retirer le cylindre entièrement du tube et rétablir ainsi la pression d’air dans le système. Refermer le robinet.

• Effectuer neuf autres mesures et déterminer la moyenne pour la durée de l’oscillation.

• Effectuer le calcul.

Remarques générales :

la qualité des mesures dépend fortement des conditions suivantes :

• Le tube de précision doit être absolument propre. Au besoin, le nettoyer avec du papier de soie.

• Le cylindre en aluminium doit également être absolument propre. D’infimes impuretés, telles des dépôts de gras, provoquent déjà d’importants frottements. Aussi, nettoyer le cylindre avant chaque mesure avec un chiffon non pelucheux et un peu de ligroïne.

• Une déformation infime du cylindre (due par ex. à une chute) suffit déjà pour influencer les mesures.

• Le tube en verre doit être orienté verticalement.

• Tous les bouchons doivent être hermétiques à l’air.

• Comme la durée de l’oscillation est élevée au carré,

sa mesure doit être réalisée minutieusement.

5. Exemple de mesure

Volume V : 10 400 cm³ Masse du cylindre m : 15,2 g Ø

du tube d : 16 mm

int.

Pression d’air pL: 1 018 mbar

Temps t en secondes pour 5 oscillations :

5,172 5,276 5,259 5,224 5,305 5,175 5,231 5,241 5,191

———

5,175

Somme : 52,249

Moyenne : 5,2249

Durée de l’oscillation Ts : 1,04498 s

On obtient ainsi la formule (11) suivante :

? = 1,39

Valeur relevée dans la littérature :

? = 1,40

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Allemagne • www.3bscientific.com • Sous réserve de modifications techniques

Page 10

3B SCIENTIFIC® PHYSICS

U14328 Tubo di precisione

Istruzioni per l’uso

9/03 ALF

Il tubo di precisione serve, unitamente alla bottiglia di Mariotte U14327, a determinare l’esponente adiabatico

cP/cV secondo Rüchardt.

1. Norme di sicurezza

• Maneggiare con cautela il tubo di vetro. Non sottoporlo a sollecitazioni meccaniche. Pericolo di rottura!

• Fare attenzione alla massima pulizia sia del tubo di vetro che del cilindro di alluminio, poiché anche le più piccole impurità aumentano l’attrito.

• Non fare cadere il cilindro di alluminio. Anche la più piccola deformazione compromette l’esperimento.

2. Descrizione, caratteristiche tecniche

Tubo di vetro di precisione alla consegna chiuso sulle due estremità con tappi di gomma e dotato di cilindro di alluminio perfettamente calibrato. Se si fa scorrere il cilindro nel tubo di vetro, chiuso nella parte inferiore e tenuto in posizione verticale, il cilindro scende lentamente verso il basso, poiché l’aria può penetrare solo molto lentamente attraverso la stretta fessura esistente tra cilindro e parete di vetro. Se si ruota il tubo di precisione di 180°, il cilindro si comporta in modo corrispondente, poiché nella parte superiore del tubo di vetro, in caso di abbassamento, si produce una depressione e l’aria può penetrare solo molto lentamente. Nel terzo caso, si lascia cadere il cilindro nel tubo aperto e lo si chiude subito. Il cilindro viene frenato e oscilla alcune volte in su e in giù.

1 Stativo

2 Bottiglia di Mariotte

3 Rubinetto a 3 vie

4 Pompa manuale

5 Tappo

6 Tubo di precisione

7 Cilindro di alluminio

Dimensioni: 600 mm x 16 mm Ø Cilindro di alluminio: 15,2 g

2.1 Fornitura

1 tubo di vetro di precisione 2 tappi di gomma 1 cilindro di alluminio

3. Principi teorici

Simboli delle formule utilizzati:

m: massa del cilindro di alluminio d: diametro interno del tubo di precisione A: area delle sezione trasversale del tubo di precisione V: volume del recipiente graduato pL: pressione atmosferica p: pressione nella bottiglia g: accelerazione di gravità n: numero di mole R: costante universale dei gas (8,31451 kJ/kmol K) T: temperatura Ts: periodo di oscillazione t: tempo cp: calore spec. a pressione costante cV: calore spec. a volume costante

?: cP/c M: pulsazione propria

Lo stato di una quantità definita di un gas ideale può essere descritta in modo univoco mediante le grandezze

interno

Page 11

di stato: pressione p, volume V e temperatura T. Ossia:

464

ApTmVTdp

p V = n R T (1)

seguente equazione differenziale per s(t)

dsdtpA

(8)

Per i cambiamenti di stato senza scambio di calore con l’ambiente questa equazione può essere convertita nell’equazione adiabatica:

p V? = const. (2)

L’esponente adiabatico ? è il rapporto della capacità termica specifica a pressione costante cp e della capacità termica specifica a volume costante cV:

(3)

Se si colloca il tubo di vetro in verticale sul tappo di gomma perforato di una bottiglia di vetro con un volume pari a 10 l e si fa scorrere il cilindro di alluminio nel tubo di vetro, vengono prodotte oscillazioni armoniche sul cuscino d’aria creato dal volume d’aria definito. Se la pressione p nella bottiglia è uguale alla somma della pressione determinata dalla massa del cilindro m e della pressione atmosferica esterna pL, il cilindro è in equilibrio:

(4)

Se il cilindro viene deviato durante il percorso s dalla posizione di equilibrio, allora p diventa ,p e V passa a ,V. Il cilindro di alluminio è sottoposto ad una forza di richiamo, che è proporzionale alla deviazione, e produce oscillazioni armoniche sul cuscino d’aria che si trova sotto. Poiché il processo di oscillazione è relativamente veloce, lo si può descrivere mediante il cambiamento di stato adiabatico. Mediante la creazione della derivazione dp/dV di (2) e il passaggio alle modifiche finali ,p e ,V, si ottiene

∆∆

V=−

(5)

Poiché il cilindro si muove durante il percorso s nel tubo di precisione, la modifica del volume è uguale a

,V = As (6)

La forza di richiamo

FAp

==−

∆χ

(7)

determina un’accelerazione periodica del cilindro con la massa m. In base al secondo assioma di Newton vale la

Dalla (8) si ricava la pulsazione propria Mdell’oscillazione armonica

(9)

e da ciò il periodo di oscillazione T

(10)

Per la determinazione dell’esponente adiabatico ? se- gue:

(11)

4. Comandi

• Determinare la pressione atmosferica, il diametro interno del tubo di precisione, la massa del cilindro di alluminio e il volume del recipiente graduato.

• Collocare il tubo di vetro sulla bottiglia di Mariotte, allineare in verticale e fissare ad uno stativo.

• La bottiglia di Mariotte deve essere collocata su un tappetino di gomma o simile, per impedire un danneggiamento sia della bottiglia che del cilindro, quando il cilindro cade nella bottiglia.

• Per semplificare l’esperimento, si consiglia di collegare una pompa manuale mediante il rubinetto a 3 vie alla bottiglia di Mariotte. Il cilindro di alluminio può in questo modo essere pompato in alto nel tubo di vetro ed essere estratto, senza dover sempre riallineare il tubo di vetro.

• Pulire il cilindro di alluminio con un panno che non lascia filaccia e un po’ di benzina solvente e, a rubinetto chiuso, non introdurlo e lasciarlo cadere in posizione inclinata nel tubo di vetro. Afferrare il cilindro solo sull’impugnatura, per evitare di imbrattarlo.

• Con un cronometro misurare 10 volte il tempo necessario ad eseguire cinque oscillazioni.

• La misurazione del tempo deve iniziare quando il cilindro viene frenato per la prima volta e si trova sul punto più profondo. La misurazione del tempo viene arrestata quando il cilindro è giunto per la sesta volta nel punto più profondo.

• Mediante la pompa manuale, a rubinetto aperto, pompare verso l’alto il cilindro, facendo attenzione a che il cilindro non cada e non venga così danneggiato.

Page 12

• Estrarre completamente il cilindro dal tubo e ristabilire nel sistema la pressione atmosferica dominante. Chiudere nuovamente il rubinetto.

• Eseguire nove ulteriori misurazioni e determinare il valore medio del periodo di oscillazione.

• Eseguire il calcolo.

5. Esempio di misurazione

Volume V: 10.400 cm³ Massa del cilindro m: 15,2 g

del tubo d: 16 mm

interno

Pressione atmosferica pL: 1018 mbar

Indicazioni generali:

la qualità delle misurazioni dipende in larga misura dalle condizioni che seguono.

• Il tubo di precisione deve essere estremamente pulito. Se necessario, pulire il tubo di vetro con carta seta.

• Ugualmente il cilindro di alluminio deve essere estremamente pulito. Anche le più piccole impurità, come i residui di grasso cutaneo, determinano un attrito elevato. Pertanto prima di ogni misurazione, pulire il cilindro con un panno che non lascia filaccia e un po’ di benzina solvente.

• Una piccolissima deformazione del cilindro (ad es. causata da una caduta) ne compromette il funzionamento.

• Il tubo di vetro deve essere allineato in verticale.

• Tutti i tappi devono essere a tenuta d’aria.

• Poiché il periodo d’oscillazione viene conteggiato al

quadrato nel risultato, il tempo deve essere rilavato con precisione.

Tempo t in secondi per 5 oscillazioni:

5,172 5,276 5,259 5,224 5,305 5,175 5,231 5,241 5,191

———

5,175

Somma: 52,249

Media: 5,2249

Periodo di oscillazione T

: 1,04498 s

Dopo l’inserimento nella formula (11), si ottiene:

? = 1,39

Valore di letteratura:

? = 1,40

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Germania • www.3bscientific.com • Con riserva di modifiche tecniche

Page 13

3B SCIENTIFIC® PHYSICS

U14328 Tubo de precisión

Instrucciones de uso

9/03 ALF

En conjunción con la botella de Mariotte, U14327, los tubos de precisión sirven para determinar el exponente adiabático cP/cV, según el método de Rüchardt.

1. Aviso de seguridad

• Manipular con cuidado los tubos de vidrio. Éstos no

deben someterse a ninguna carga mecánica. ¡Peligro de rompimiento!

• Se debe observar una extrema limpieza, tanto de los

tubos de vidrio como del cilindro de aluminio, puesto que incluso una mínima presencia de impurezas conduce a una elevada fricción.

• No se debe dejar caer el cilindro de aluminio. Incluso

las más pequeñas deformaciones influyen negativamente en el resultado del experimento.

2. Descripción, datos técnicos

El tubo de precisión se suministra obturado en ambos extremos por tapones de caucho y con un cilindro de aluminio exactamente ajustado. Si se deja resbalar el cilindro dentro del tubo cerrado en su extremo inferior y mantenido en posición vertical, éste se deslizará lentamente hacia abajo, debido a que el aire sólo puede penetrar muy lentamente a través de la estrecha grieta que separa el cilindro de la pared del tubo. Si se gira el tubo de precisión 180°, el cilindro se comporta correspondientemente, puesto que en el tramo superior del tubo de vidrio, durante el descenso, se origina una depresión y el aire sólo puede penetrar con mucha lentitud. En el tercer caso, se deja caer el cilindro

1 Soporte

2 Botella de Mariotte

3 Grifo de tres pasos

4 Bomba de mano

5 Tapón

6 Tubo de precisión

7 Cilindro de aluminio

dentro del tubo abierto y se lo cierra inmediatamente. El cilindro se frena y oscila varias veces hacia arriba y hacia abajo.

Dimensiones: 600 mm x 16 mm Ø Cilindro de aluminio: 15,2 g

2.1 Volumen de suministro

1 tubo de vidrio de precisión 2 tapones de caucho 1 cilindro de aluminio

3. Fundamentos teóricos

Símbolos usados en las fórmulas:

m: peso del cilindro de aluminio d: diámetro interior del tubo de precisión A: área de la sección transversal del tubo de precisión V: volumen del matraz graduado pL: presión atmosférica p: presión en la botella g: aceleración terrestre n: número de moles R: constante universal de los gases

(8,31451 kJ/kmol K)

T: temperatura Ts: duración de oscilación t: tiempo cp: calor específico ante presión constante cV: calor específico ante volumen constante

?: cP/c M: frecuencia angular propia

interior

Page 14

El estado de una determinada cantidad de gas ideal que

464

ApTmVTdp

se encuentre en un espacio cerrado, se puede describir claramente por medio de las magnitudes de estado de presión p, volumen V y temperatura T. Es válido lo siguiente:

p V = n R T (1)

conduce a aceleraciones periódicas del cilindro con el peso m. De acuerdo con el segundo axioma de Newton, para s(t), es válida la siguiente ecuación diferencial:

dsdtpA

(8)

Para los cambios de estado, sin intercambio de calor con el medio ambiente, esta fórmula se puede transformar en la ecuación adiabática:

p V? = const. (2)

El exponente adiabático ? es la relación existente entre la capacidad de calor específica ante presión constante cp y la capacidad de calor específica ante volumen constante

cV:

(3)

Si el tubo de vidrio se inserta verticalmente en el tapón perforado de una botella de gas, que tenga un volumen de 10 l, y se deja que el cilindro de aluminio se deslice dentro del tubo de vidrio, éste rebotará armoniosamente sobre el colchón neumático formado por el volumen de aire encerrado. Si la presión p, al interior de la botella, es igual a la suma de la presión provocada por el peso m del cilindro y la presión atmosférica externa pL, el cilindro se encuentra en equilibrio:

(4)

Si el cilindro se desvía del estado de equilibrio en un tramo s, entonces p varía en ,p y V en ,V. El cilindro de aluminio soporta una fuerza antagonista que es proporcional a la desviación. El cilindro oscila armoniosamente sobre el colchón neumático que se encuentra por debajo de él. Dado que el proceso de oscilación se detiene de una manera relativamente rápida, éste se puede describir por medio de las variaciones adiabáticas de estado. Al realizar la derivación dp/dV de (2) y el paso a las variaciones finales ∆p y ∆V se obtiene:

∆∆

V=−

(5)

Dado que el cilindro se mueve dentro del tubo de precisión en un tramo s, la variación del volumen es igual a:

,V = As (6)

La fuerza antagonista

FAp

==−

∆χ

(7)

A partir de (8) se obtiene la frecuencia angular propia M de la oscilación armónica:

(9)

y con ello la duración de la oscilación T

(10)

Para la determinación del exponente adiabático ? se sigue lo siguiente:

(11)

4. Servicio

• Determinar la presión atmosférica, el diámetro interno del tubo de precisión, el peso del cilindro de aluminio y el volumen del matraz graduado.

• Colocar el tubo de precisión sobre la botella de Mariotte, posicionarlo verticalmente y fijarlo a un soporte.

• La botella de Mariotte se debe guarnecer por dentro con una esterilla de goma o algún elemento similar, para prevenir un daño, tanto de la botella como del cilindro, en el caso de que el cilindro caiga y golpee el fondo de la botella.

• Para simplificar el experimento, es recomendable conectar una bomba de mano a la botella de Mariotte, por medio de un grifo de tres pasos. De esta manera, el cilindro de aluminio se puede bombear hacia arriba, dentro del tubo, y retirarlo sin necesidad de alinear nuevamente el tubo de vidrio.

• El cilindro de aluminio se debe limpiar con un paño libre de pelusas y un poco de gasolina de lavado, y se debe introducir y dejar caer dentro del tubo de vidrio, con el grifo cerrado y sin ladear. Para evitar que se ensucie, el cilindro sólo se debe tomar por la empuñadura.

• Medir diez veces con un cronómetro el tiempo necesario para que se produzcan cinco oscilaciones.

• La medición del tiempo se debe iniciar cuando el cilindro se frene por primera vez y se encuentre en la posición más profunda. La medición se debe detener cuando el cilindro haya arribado por sexta ocasión al punto más profundo.

• Provocar el ascenso del cilindro por medio de una bomba de mano, con el grifo abierto. Durante este

Page 15

proceso se debe tener cuidado de que el cilindro no caigahacia afuera y se dañe.

• Retirar completamente el cilindro del tubo para que así vuelva a actuar sobre el sistema la presión atmosférica reinante. Cerrar nuevamente el grifo.

• Realizar nuevas mediciones y determinar el valor promedio de la duración de la oscilación.

• Llevar a cabo el cálculo.

Notas generales:

La calidad de las mediciones depende, en gran medida, de las siguientes condiciones:

• El tubo de precisión debe encontrarse en un estado de extrema limpieza. Dado el caso, se debe limpiar el tubo de vidrio con papel de seda.

• De igual manera, el cilindro de aluminio debe encontrarse extremadamente limpio. Incluso las más pequeñas impurezas, tales como acumulación de grasa de la piel, producen una fuerte fricción. Por tanto, antes de cada medición, se lo debe limpiar con un paño libre de pelusas y un poco de bencina de lavado.

• Una mínima deformación del cilindro (provocada, por ejemplo, por una caída) menoscaba los resultados del experimento.

• El tubo de vidrio debe alinearse verticalmente.

• Todos los tapones deben ser herméticos.

• Dado que para obtener el resultado se toma el cua-

drado del valor de duración de oscilación, el tiempo debe registrarse cuidadosamente.

5. Ejemplo de medición

Volumen V: 10400 cm³ Peso m del cilindro: 15,2 g Ø

d del tubo: 16 mm

Interior

Presión atmosférica pL: 1018 mbar

Tiempo t en segundos para 5 oscilaciones:

5,172 5,276 5,259 5,224 5,305 5,175 5,231 5,241 5,191 5,175

Suma: 52,249

Promedio: 5,2249

Duración de oscilación Ts: 1,04498 s

Al aplicar la fórmula (11) se obtiene:

? = 1,39

Valor tomado de la literatura:

? = 1,40

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Alemania • www.3bscientific.com • Se reservan las modificaciones técnicas

Page 16

3B SCIENTIFIC® PHYSICS

U14328 Tubo de precisão

Instruções para o uso

9/03 ALF

O tubo de precisão serve, em associação com a garrafa de Mariotte U14327, para a determinação do expoente adiabático cP/cV segundo Rüchardt.

1. Indicações de segurança

• Manusear o tubo de vidro com cuidado. Não submeter a qualquer esforço mecânico. Perigo, eles podem quebrar!

• Garantir uma limpeza extrema tanto do tubo de vidro assim como do cilindro de alumínio, já que mesmo ínfimas impurezas podem levar a um maior atrito.

• Não deixar cair o cilindro de alumínio. Mesmo deformações muito pequenas podem falsear os resultados.

2. Descrição, dados técnicos

O tubo de precisão é fornecido com tampões de borracha em ambas extremidades e um cilindro de alumínio exatamente na sua medida. Caso se deixar o cilindro deslizar no tubo mantido na vertical e com a extremidade inferior tampada ele descerá lentamente, porque o ar só consegue sair lentamente pelo estreito espaço entre o cilindro e a parede do tubo de vidro. Girando-se o tubo de precisão em 180°, o cilindro comporta-se da mesma forma já que na parte superior do tubo estabelece-se baixa pressão e o ar só pode penetrar no tubo muito lentamente. No terceiro caso, deixa-se o cilindro cair no tubo aberto e tampa-se-o ime-

1 Tripé

2 Garrafa de Mariotte

3 Torneira de 3 vias

4 Bomba manual

5 Tampão

6 Tubo de precisão

7 Cilindro de alumínio

diatamente. O cilindro freia na sua queda e oscila várias vezes para cima e para baixo.

Dimensões: 600 mm x 16 mm Ø Cilindro de alumínio: 15,2 g

2.1 Fornecimento

1 Tubo de precisão 2 Tampões de borracha 1 Cilindro de alumínio

3. Fundamentos teóricos

Símbolos utilizados nas fórmulas:

m: Massa do cilindro de alumínio d: Diâmetro interno do tubo de precisão A: Superfície de corte perpendicular do tubo de precisão V: Volume do recipiente de medição pL: Pressão do ar p: Pressão na garrafa g: Aceleração da atração da terra n: Número de mol R: Constante universal do gás (8,31451 kJ/kmol K) T: Temperatura Ts: Duração da oscilação t: Tempo cp: Calor espec. a pressão constante

cV: Calor espec. a volume constante ?: cP/c

M: Freqüência natural

interno

Page 17

O estado de uma quantidade fechada de um gás ideal

464

ApTmVTdp

pode ser descrito de forma unívoca por meio das grandezas de estado pressão p, Volume V e temperatura T. É válido:

p V = n R T (1)

leva à aceleração periódica do cilindro com a massa m. Conforme ao segundo axioma de Newton é válida a seguinte equação diferencial para s(t)

dsdtpA

(8)

Para mudanças de estado sem troca de calor com o ambiente, esta equação pode ser transformada na equação adiabática:

p V? = const. (2)

O expoente adiabático ? é a relação da capacidade térmica específica com pressão constante cp e capacidade térmica específica com volume constante cV:

(3)

Colocando-se o tubo de vidro na vertical nos tampões de borracha perfurados de uma garrafa de gás com um volume de 10 l e deixando o cilindro de alumínio deslizar no tubo de vidro, este entrará em oscilação harmônica sobre o colchão de ar formado pelo volume de ar enclausurado. Quando a pressão p na garrafa iguala a soma da pressão originada pela massa do cilindro m e da pressão do ar externo pL, então o cilindro se encontra em situação de equilíbrio:

(4)

Se o cilindro for inclinado para fora do ponto de equilíbrio numa distância s, então p se transforma em ,p e V em ,V. O cilindro de alumínio é sujeito a uma força de restauração, a qual é proporcional à inclinação. Ele gera oscilações harmônicas sobre o colchão de ar que se encontra debaixo dele. Sendo que o processo oscilatório acontece muito rapidamente, é possível descreve-lo através da modificação adiabática de estado. Por cálculo da derivada dp/dV de (2) e passagem às variações finitas ,p e ,V obtêm-se

∆∆

V=−

(5)

Sendo que o cilindro se move numa distância s no tubo de precisão, a variação do volume totaliza

,V = As (6)

A força contrária

FAp

==−

∆χ

(7)

De (8) resulta a freqüência natural M das oscilações harmônicas

(9)

e daí a duração de oscilação T

(10)

Para a determinação do expoente adiabático ? segue:

(11)

4. Utilização

• Determinar a pressão, o diâmetro interno do tubo de precisão, a massa do cilindro de alumínio e o volume do recipiente de medição.

• Colocar o tubo de vidro sobre a garrafa de Mariotte, levar à posição vertical e fixa-los num tripé.

• Na garrafa de Mariotte deveria ser colocada um tapetezinho de borracha ou semelhante de forma a evitar a danificação tanto da garrafa como do cilindro quando este cair na garrafa.

• Para simplificar o ensaio, é recomendável a conexão de uma bomba manual com a garrafa de Mariotte pela torneira de 3 vias. O cilindro de alumínio pode desta forma ser elevado no tubo de vidro por bombeamento e assim ser retirado, sem que seja necessário voltar a montar o tubo de vidro para isto.

• Limpar o cilindro de alumínio com um pano que não solte fiapos e um pouco de benzina para a limpeza e logo introduzi-lo no tubo de vidro com a torneira fechada e paralelo ao tubo deixando-o cair. Só pegar no tubo pelo punho de modo a evitar depósitos de impurezas no cilindro.

• Medir 10 vezes com um cronômetro o tempo para cinco oscilações.

• A medição do tempo deve começar quando o cilindro for freiado pela primeira vez e se encontrar no ponto mais baixo. A medição do tempo deve terminar quando o cilindro tiver chegado pela sexta vez no ponto mais baixo.

• Levar o cilindro para cima bombeando por meio da bomba manual e com a torneira aberta. Ao faze-lo,

Page 18

prestar atenção para que o cilindro não caia e seja danificado.

• Retirar completamente o cilindro do tubo para assim restabelecer a pressão do ar ambiente no sistema. Voltar a fechar a torneira.

• Efetuar mais nove medições e determinar o valor médio para a duração da oscilação.

• Efetuar o cálculo.

Indicações gerais:

a qualidade das medições depende fortemente das condições seguintes:

• O tubo de precisão deve estar extremamente limpo. Caso seja necessário, limpar o tubo de vidro com papel de seda.

• O cilindro de alumínio também deve estar perfeitamente limpo. Mesmo as mais ínfimas impurezas, como depósitos de gordura dos dedos, levam a forte atrito. Por isso, limpar o cilindro com um pano que não solte fiapos e um pouco de benzina para limpeza antes de cada medição.

• Mesmo as menores deformações do cilindro (por exemplo, resultante de uma queda) prejudicam os resultados.

• O tubo de vidro deve estar colocado em posição vertical.

• Todos os tampões devem ser herméticos ao ar.

• Sendo que o tempo de oscilação integra o resultado

ao quadrado, o tempo deve ser medido com muito cuidado.

5. Exemplo de medição

Volume V: 10400 cm³ Massa m do cilindro: 15,2 g Ø

do tubo d: 16 mm

Interno

Pressão do ar pL: 1018 mbar

Tempo t em segundos para 5 oscilações:

5,172 5,276 5,259 5,224 5,305 5,175 5,231 5,241 5,191

———

5,175

Soma: 52,249

Valor médio: 5,2249

Duração da oscilação Ts: 1,04498 s

Após a aplicação da fórmula (11) obtêm-se:

? = 1,39

Valor teórico:

? = 1,40

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Alemanha • www.3bscientific.com • Sob reserva de modificações técnicas