3B Scientific Oscillation Tube User Manual

3B SCIENTIFIC® PHYSICS

U14328 Precision tube

Instruction sheet

9/03 ALF

®

1

2

The precision tube is used together with the Marriott
bottle U14327 to determine the adiabatic exponent cP/c
using Rüchardt’s method.

1. Safety instructions

• Handle the glass tube carefully. Do not subject to
mechanical stress or it may break.

• Make sure both glass tube and aluminium cylinder
are thoroughly clean because even the slightest
amount of dirt leads to increased friction.

• Do not drop the aluminium cylinder. Even the slight­est deformation adversely affects the experiment.

2. Description, technical data

A precision glass tube supplied with rubber stoppers at
both ends and with an aluminium cylinder precisely en­gineered to slip inside.
If the glass tube is held vertical with the bottom end
closed and the aluminium cylinder is allowed to slide
down inside it, the cylinder drops very slowly since there
is only a tiny gap around the sides for air to escape from
underneath. If the tube is rotated by 180°, the cylinder
behaves in the same manner since the pressure in the
upper part of the tube is reduced as the cylinder slides
down, and air can only get in to fill the space very slowly.
In the third situation, the cylinder is allowed to drop into
an open tube which is then immediately stoppered. The
cylinder then slows and oscillates up and down a few
times.

3

7

6

5

4

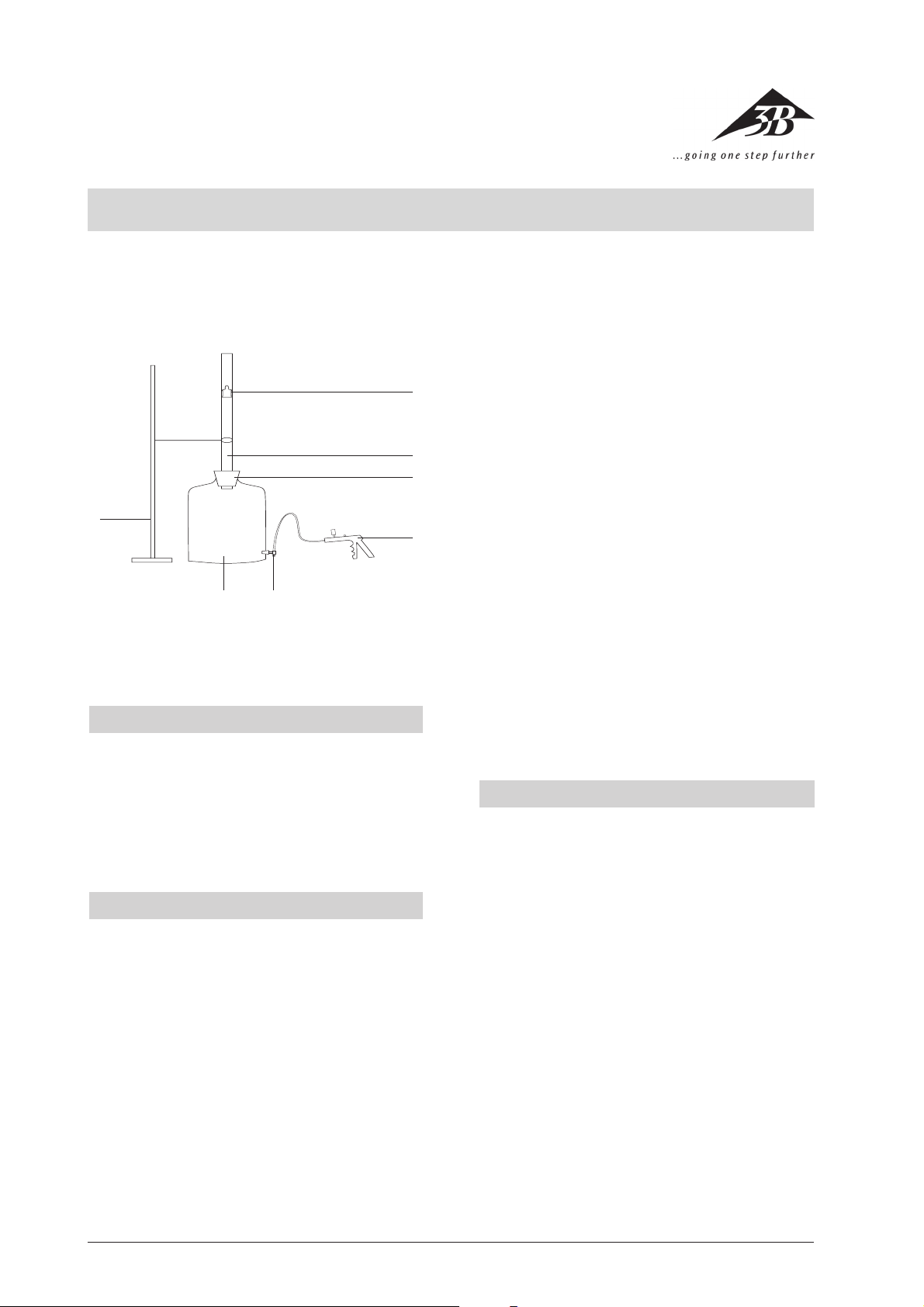
1 Stand

2 Marriott bottle

3 3-way stopcock

4 Hand pump

5 Stoppers

6 Precision tube

7 Aluminium cylinder

Dimensions: 600 mm x 16 mm Ø

V

Aluminium cylinder: 15.2 g

2.1 Scope of supply

1 Precision glass tube
2 Rubber stoppers
1 Aluminium cylinder

3. Theory

Symbols used in the formula:

m: Mass of aluminium cylinder
d: Inside diameter of precision tube
A: Cross-sectional area of precision tube
V: Volume of measuring flask
pL: Atmospheric pressure
p: Pressure in the bottle
g: Acceleration due to Earth’s gravity
n: Number of moles
R: Universal gas constant (8.31451 kJ/kmol K)
T: Temperature
Ts: Period of oscillation
t: Time
cp: Specific heat at constant pressure
cV: Specific heat at constant volume

?: cP/c
M: Natural frequency of oscillation

The state of an enclosed quantity of an ideal gas can be
uniquely expressed in terms of the quantities pressure p,
volume V and temperature T as follows:

V

inner

4

p V = n R T (1)

ω

χ

=

pA

V

2

χ

π

==

464

2

22

2

4

mV

ApTmVTdp

ss

For changes in state where no exchange of heat with the
environment takes place, this equation can be reduced
to the adiabatic formula:

From (8) the natural frequency of oscillation is given by

(9)

p V? = const. (2)

The adiabatic exponent ? is the ratio of the specific heat
at constant pressure cp to the specific heat at constant
volume cV:

c

P

χ

=

c

V

(3)

A stopper with a hole is put into a glass vessel with a
volume of 10 l . The precision tube runs through the hole
in this stopper so that it is in a vertical position. If the
aluminium cylinder is allowed to drop into the tube, it
bounces on the cushion of air enclosed inside the appa­ratus. This leads to a periodic oscillation.
When the pressure p in the glass vessel is equal to the
sum of the pressure due to the mass of the aluminium
cylinder and the external atmospheric pressure, the cyl­inder is in a state of equilibrium:

pp

mg

=+

L

A

(4)

If the cylinder is moved a distance s from its equilibrium
position, p changes by the value ,p and V changes by
,V. A force acts on the aluminium cylinder to push it
back towards equilibrium. This force is proportional to
the distance s. A harmonic oscillation now begins atop
the air cushion under the cylinder. Since the oscillation
occurs fairly rapidly, it can be described in terms of the
adiabatic change of state. By deriving dp/dV from equa­tion (2) and assuming this also applies to the small finite
changes ,p and ,V we obtain

∆∆

p

p

χ

V=−

V

(5)

Since the cylinder moves along a distance s in the preci­sion tube, the change in volume is

,V = As (6)

The restoring force

2

FAp

==−

∆χ

pA

s

V

(7)

leads to the periodic acceleration of a cylinder of mass m.
Newton’s second law then gives us the following differ­ential equation for s(t)

2

dsdtpA

2

2

+=

χ

0

s

V

(8)

thus the period of the oscillation Ts is

2

π

T

==

s

ω

mV

2

π

2

pA

χ

(10)

Therefore, to derive the adiabatic exponent ? the follow­ing applies:

(11)

4. Operation

• Determine the atmospheric pressure, the diameter
of the inside of the precision tube, the mass of the
aluminium cylinder and the volume of the measur­ing flask.

• Place the glass tube on the Marriott bottle, make sure
it is vertical and secure it to a stand.

• The Marriott bottle should have a rubber mat or simi­lar placed inside to avoid damage to the bottle and
the cylinder if the cylinder falls into the bottle.

• To simplify the experiment, it is recommended that a
hand pump be attached to the Marriott bottle via the
3-way stopcock. The cylinder can then be pumped
back up the tube and retrieved from the top so that it
is not necessary to repeatedly set the tube up.

• The aluminium cylinder should be cleaned with a
fluff-free cloth and some petroleum ether. It should
not be introduced into the tube at a crooked angle
and allowed to drop when the stopcock is closed. Do
not touch the cylinder except by the grip to prevent it
getting dirty.

• Measure the duration of 5 oscillations 10 times using
a stopwatch.

• The time measurement should start at the point when
the cylinder comes to a halt for the first time at its
lowest point. The watch should be stopped when the
cylinder reaches its lowest point for the sixth time.

• Using the hand pump with the stopcock open, pump
the cylinder back to the top. Make sure that the cylin­der does not fall out at the end and get damaged.

• Take the cylinder right out of the tube so that the
pressure in the apparatus returns to atmospheric pres­sure. Close the stopcock again.

• Make the measurements another nine times and
determine the average value for the time.

• Perform the calculation.

5