3B Scientific Inertia Balance User Manual

3B SCIENTIFIC® PHYSICS

Inertia Balance U30045

Instruction Sheet

11/08 ALF

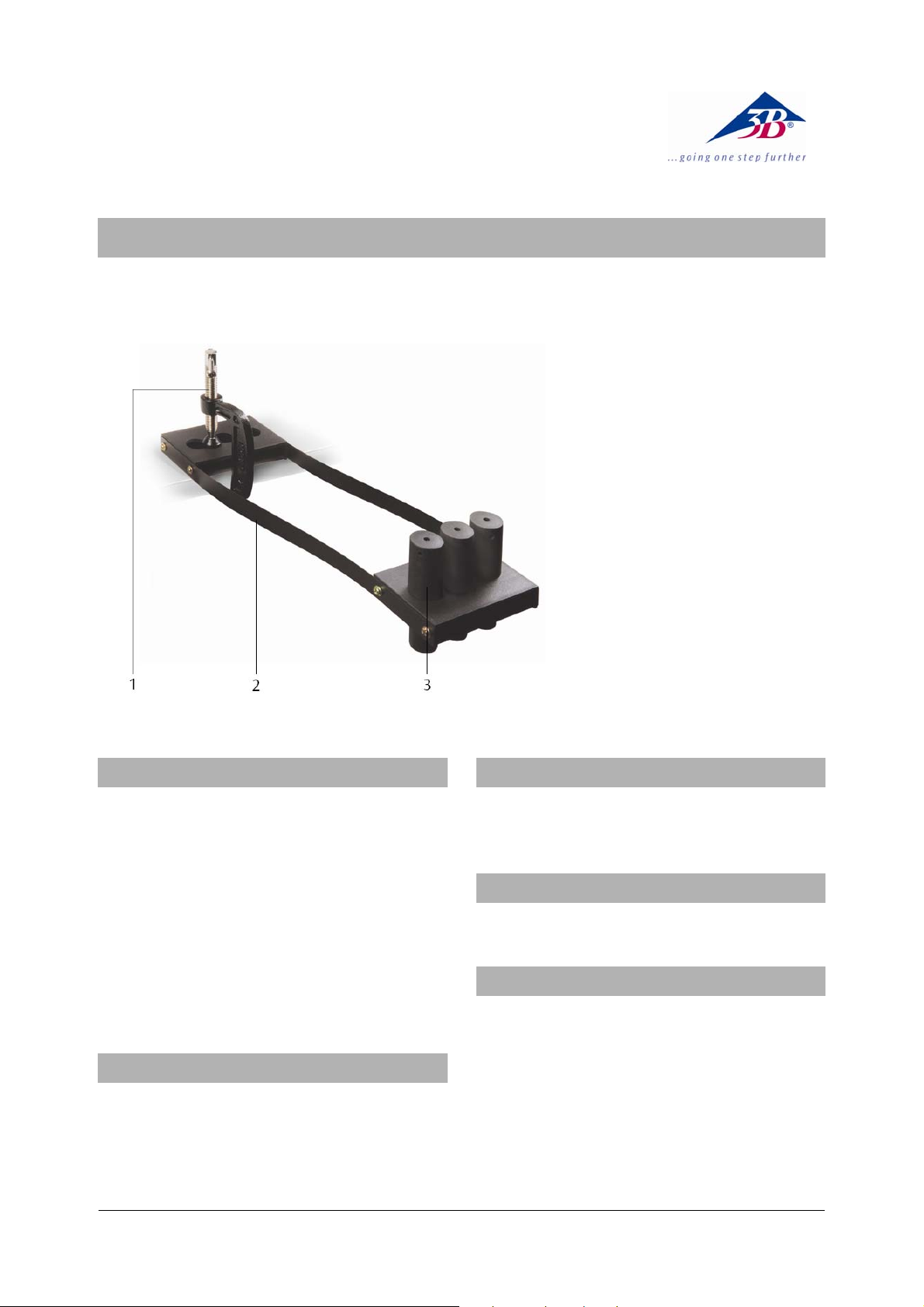
1 Table clamp
2 Steel spring strip
3 Masses

1. Description

The inertia balance allows for measurement of mass
independent of Earth’s gravitational force.

The apparatus consists of two metal trays connected
by stiff steel spring strips. One tray has 3 holes to hold
up to three masses. The other tray may be anchored
to a table edge or laboratory bench with the included
table clamp so that the stage can vibrate horizontally.
The period of the horizontal oscillations depends on
the mass on the stage.

After the apparatus is calibrated by determining the
vibration frequency for objects of known mass, it can
be used to determine the unknown masses.

2. Equipment supplied

1 Inertia balance
1 Table clamp
1 Cord, 1.85 m
3 Masses

3. Technical data

Length of steel strip: approx. 350 mm
Masses: approx. 175 g each

4. Additionally required equipment

1 Mechanical Stopwatch, 15 min U40801

5. Operation

The mass of an object is the amount of matter it
contains, while its weight depends on the
gravitational force acting on it. Most of the methods
we use to compare masses depend on the forces they
exert – in other words, they depend on their weight.

The inertia balance gives us a method of comparing
masses directly, and independently of the
gravitational forces exerted on them.

• Attach the inertia balance to a table edge.

1

• Using no mass on the stage displace the apparatus

slightly so that the stage vibrates horizontally.

• Record the period for 10 oscillations.

• Do three measuring runs, then average the

results.

The period T of one oscillation is the time taken
between the stage passing through its mid point, and
the next time it passes through the same point,
moving in the same direction.

• Repeat this process using as many different

combinations as possible of the three masses
supplied.

• Put your results into a table.

• Plot a graph of period T against mass m.

• Repeat the experiment with the unknown mass,

and use the graph to find the magnitude of this
mass.

The inertia balance is actually a compound spring
system. The period of an oscillating spring is given by
the equation

T ⋅π=

D

m

22

4 (1)

where T = period, m = mass on the spring, D = the
spring's elasticity constant.

• Plot a graph of T

• Determine the elasticity constant D from the slope

2

against mass.

of the graph.

• Calculate the unknown mass m from the equation (1).

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Germany • www.3bscientific.com

Subject to technical amendment

© Copyright 2008 3B Scientific GmbH