Page 1

3B SCIENTIFIC® PHYSICS
Trägheitswaage U30045
Bedienungsanleitung
11/08 ALF
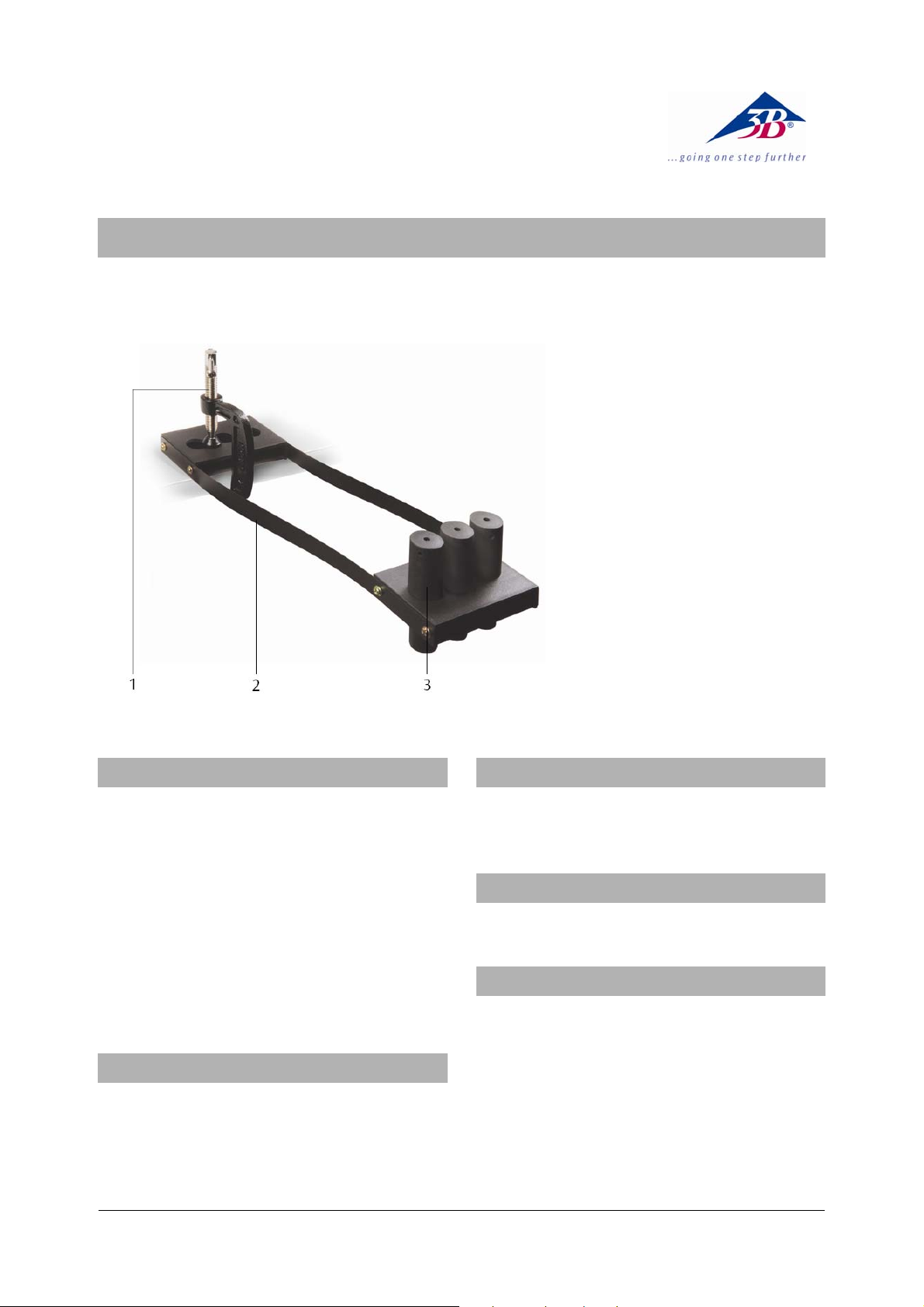
1 Tischzwinge
2 Stahlfederband
3 Massen
1. Beschreibung
Die Trägheitswaage dient zur Bestimmung der Masse
unabhängig von der Erdanziehungskraft.
Das Gerät besteht aus zwei Metallplatten, die mit
steifen Stahlfederbändern miteinander verbunden
sind. Eine Platte ist mit 3 Öffnungen zur Aufnahme
von bis zu drei Massen versehen; die andere Schale
kann mit der mitgelieferten Tischzwinge an einer
Tischkante angeklemmt werden, so dass sie horizontal
schwingen kann. Die Schwingungsperiode ist dabei
abhängig von der Masse der schwingenden Platte.
Nach Kalibrieren der Vorrichtung durch Bestimmung
der Schwingungsfrequenz von Objekten bekannter
Masse lässt sich das Gerät dazu verwenden,
unbekannte Massen zu bestimmen.
2. Lieferumfang
1 Trägheitswaage
1 Tischzwinge
1 Kabel, 1,85 m
3 Massen
3. Technische Daten
Stahlfederbandlänge: ca. 350 mm
Massen: je ca. 175 g
4. Zusätzlich erforderliche Geräte
1 Mechanische Stoppuhr, 15 min U40801
5. Bedienung
Die Masse eines Körpers ist die Menge der darin
enthaltenen Materie, während sein Gewicht von der
Gravitationskraft abhängt, die auf ihn wirkt. Die
meisten Messmethoden von Massen beruhen auf den
Kräften, die sie ausüben, d.h. sie beruhen auf ihrem
Gewicht.
Mittels der Trägheitswaage können Massen direkt
gemessen werden unabhängig von der Gravitation, die
auf sie wirkt.
• Trägheitswaage am Tisch befestigen.
• Platte ohne Masse seitlich auslenken und in
horizontale Schwingung versetzen.
1
Page 2

• Zeit für 10 Schwingungen messen.
• Messung dreimal wiederholen, dann Ergebnisse
mitteln.
Die Periodendauer T einer Schwingung ist die Zeit, die
die Platte braucht vom Durchgang durch den
Mittelpunkt ihrer Ruhelage bis zum nächsten
Durchgang durch den gleichen Punkt in der gleichen
Bewegungsrichtung.
• Experiment mit allen Kombinationen der drei
Massen wiederholen.
• Ergebnisse in eine Tabelle eintragen.
• Periodendauer T in Abhängigkeit der Masse m in
einem Koordinatensystem graphisch darstellen.
• Experiment mit der unbekannten Masse
wiederholen und mittels des Graphen die Größe
der Masse bestimmen.
Die Trägheitswaage entspricht einer schwingenden
Feder, deren Periodendauer durch die Gleichung 1
gegeben ist:
T ⋅π=
D
m
22
4 (1)
mit T = Periodendauer, m = Masse auf der Feder, D =
Elastizitätskonstante der Feder.
2
• T
in Abhängigkeit der Masse m graphisch
darstellen.
• Die Elastizitätskonstante der Feder D ergibt sich
aus der Steigung des Graphen.
• Unbekannte Masse mittels der Gleichung 1 errechnen.
3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Deutschland • www.3bscientific.com
Technische Änderungen vorbehalten
© Copyright 2008 3B Scientific GmbH
Page 3
3B SCIENTIFIC® PHYSICS
Inertia Balance U30045
Instruction Sheet
11/08 ALF
1 Table clamp
2 Steel spring strip
3 Masses
1. Description
The inertia balance allows for measurement of mass
independent of Earth’s gravitational force.
The apparatus consists of two metal trays connected
by stiff steel spring strips. One tray has 3 holes to hold
up to three masses. The other tray may be anchored
to a table edge or laboratory bench with the included
table clamp so that the stage can vibrate horizontally.
The period of the horizontal oscillations depends on
the mass on the stage.
After the apparatus is calibrated by determining the
vibration frequency for objects of known mass, it can
be used to determine the unknown masses.
2. Equipment supplied
1 Inertia balance
1 Table clamp
1 Cord, 1.85 m
3 Masses
3. Technical data
Length of steel strip: approx. 350 mm
Masses: approx. 175 g each
4. Additionally required equipment
1 Mechanical Stopwatch, 15 min U40801
5. Operation
The mass of an object is the amount of matter it
contains, while its weight depends on the
gravitational force acting on it. Most of the methods
we use to compare masses depend on the forces they
exert – in other words, they depend on their weight.
The inertia balance gives us a method of comparing
masses directly, and independently of the
gravitational forces exerted on them.
• Attach the inertia balance to a table edge.
1
Page 4

• Using no mass on the stage displace the apparatus
slightly so that the stage vibrates horizontally.
• Record the period for 10 oscillations.
• Do three measuring runs, then average the
results.
The period T of one oscillation is the time taken
between the stage passing through its mid point, and
the next time it passes through the same point,
moving in the same direction.
• Repeat this process using as many different
combinations as possible of the three masses
supplied.
• Put your results into a table.
• Plot a graph of period T against mass m.
• Repeat the experiment with the unknown mass,
and use the graph to find the magnitude of this
mass.
The inertia balance is actually a compound spring
system. The period of an oscillating spring is given by
the equation
T ⋅π=
D
m
22
4 (1)
where T = period, m = mass on the spring, D = the
spring's elasticity constant.
• Plot a graph of T
• Determine the elasticity constant D from the slope
2
against mass.
of the graph.
• Calculate the unknown mass m from the equation (1).
3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Germany • www.3bscientific.com
Subject to technical amendment
© Copyright 2008 3B Scientific GmbH
Page 5

3B SCIENTIFIC® PHYSICS
Balance d’inertie U30045
Instructions d'utilisation
11/08 ALF
1 Étau de fixation pour table
2 Bande ressort en acier
3 Masses
1. Description
La balance d’inertie permet de mesurer la masse
indépendamment de la force de gravitation de la
Terre.
L'appareil se compose de deux plateaux en métal
reliés entre par des bandes ressort en acier rigide.
L'un des plateaux dispose de 3 orifices pour y fixer
jusqu'à trois masses, le deuxième plateau pouvant
être lui fixé au coin d'une table ou sur un banc de
laboratoire à l'aide de l'étau de fixation pour table ce
qui lui permet d'osciller horizontalement. La période
d'oscillation dépendant dans ce cas de la masse du
plateau oscillant.
L'appareil une fois calibré en déterminant la
fréquence vibratoire d'objets de masse connue, il
pourra s'utiliser afin de déterminer des masses
inconnues.
2. Matériel fourni
1 balance d’inertie
1 étau de fixation pour table
1 cordon de 1,85 m
3 masses
3. Caractéristiques techniques
Longueur de la bande
ressort en acier : env. 350 mm
Masses : env. de 175 g chacune
4. Appareils supplémentaires nécessaires
1 Chronomètre mécanique, 15 min U40801
5. Manipulation
La masse d'un corps est définie comme la quantité de
matière qu'il contient, son poids dépendant par
contre de la force gravitationnelle qui agit sur ce
corps. La plupart des méthodes permettant de
mesurer les masses font appel aux forces qu'elles
exercent, c'est à dire qu'elles font appel à leur poids.
La balance d’inertie permet de mesurer directement
des masses indépendamment des forces de gravitation
auxquelles elles sont soumises.
• Fixez la balance d’inertie sur la table.
• Écartez latéralement le plateau sans masse, puis
faites-le osciller horizontalement.
• Mesurez le temps nécessaire à 10 oscillations.
1
Page 6

• Répétez vos mesures plusieurs fois, puis faites la
moyenne des résultats obtenus.
La durée de la période T d'une oscillation est le temps
nécessité par le plateau pour passer du point central
de sa position de repos jusqu'au prochain passage par
le même point et dans la même direction de
mouvement.
• Répétez l'essai expérimental en appliquant toutes
les combinaisons des trois masses.
• Portez les résultats dans un tableau.
• Représentation graphique de la durée d'une
période T en fonction de la masse m dans un
système de coordonnées.
• Répétez l'essai expérimental avec une masse
inconnue, puis déterminez la taille de la masse au
moyen du graphe.
La balance d’inertie correspond à un ressort oscillant
dont la durée de la période est obtenue par l'équation
1 :
T ⋅π=
D
m
22
4 (1)
T étant la durée de la période, m étant la masse sur le
ressort et D étant la constante d'élasticité du ressort.
• Représentation graphique T
2
en fonction de la
masse m.
• La constante d'élasticité du ressort D se calculera
à partir de la pente du graphe.
• Calculez la masse inconnue en appliquant
l'équation 1.
3B Scientific GmbH ▪ Rudorffweg 8 ▪ 21031 Hamburg ▪ Allemagne ▪ www.3bscientific.com
Sous réserve de modifications techniques
© Copyright 2008 3B Scientific GmbH
Page 7

3B SCIENTIFIC® PHYSICS
Inertia Balance U30045
Istruzioni per l'uso
11/08 ALF
1 Morsetto da tavolo
2 Nastro di acciaio
3 Masse
1. Descrizione
Inertia Balance consente di misurare una massa
indipendente dalla forza di gravità della Terra.
Il dispositivo comprende due vassoi di metallo
collegati mediante nastri elastici rigidi in acciaio. Un
vassoio presenta 3 fori su cui collocare tre masse,
mentre l’altro vassoio può essere fissato al bordo di un
tavolo o al bancone di laboratorio con l’apposito
morsetto in dotazione, in modo che possa oscillare
orizzontalmente. Il periodo di oscillazione in questo
caso dipende dalla massa della piastra oscillante.
Dopo aver calibrato il dispositivo fissando la
frequenza di vibrazione per oggetti di massa
conosciuta, è possibile utilizzarlo per determinare
masse sconosciute.
2. Fornitura
1 Inertia Balance
1 morsetto da tavolo
1 cordone, 1,85 m
3 masse
3. Dati tecnici
Lunghezza del nastro di acciaio: ca. 350 mm
Masse: ciascuno ca. 175 g
4. Altri apparecchi necessari
1 Cronometro meccanico, 15 min U40801
5. Utilizzo
La massa di un corpo è la quantità di materia ivi
contenuta, mentre il peso dipende dalla forza
gravitazionale, che agisce su di esso. La maggior parte
dei metodi di misura di masse si basano sulle forze
che esse esercitano, cioè si basano sul loro peso.
Mediante la bilancia inerziale, è possibile misurare
direttamente le masse indipendentemente dalla
gravitazione che agisce su di esse.
• Fissare la bilancia inerziale sul tavolo.
• Spostare lateralmente la piastra senza massa e
metterla in oscillazione orizzontale.
• Misurare il tempo occorrente per 10 oscillazioni.
1
Page 8

• Ripetere tre volte la misurazione, quindi calcolare
la media dei risultati.
Il periodo T di un'oscillazione è il tempo che occorre
alla piastra tra il passaggio per il centro della sua
posizione di riposo e il successivo passaggio per lo
stesso punto nella stessa direzione di movimento.
• Ripetere l'esperimento con tutte le combinazioni
delle tre masse.
• Inserire i risultati in una tabella.
• Rappresentare graficamente in un sistema di
coordinate il periodo T in funzione della massa m.
• Ripetere l'esperimento con la massa sconosciuta e
determinare mediante il grafico la grandezza
della massa.
La bilancia inerziale corrisponde a una molla
oscillante il cui periodo è dato dall'equazione 1:
T ⋅π=
D
m
22
4 (1)
con T = periodo, m = massa sulla molla, D = costante
di elasticità della molla.
• Rappresentare graficamente T
2
in funzione della
massa m .
• La costante di elasticità della molla D risulta dalla
pendenza del grafico.
• Calcolare la massa sconosciuta mediante
l'equazione 1.
3B Scientific GmbH • Rudorffweg 8 • 21031 Amburgo • Germania • www.3bscientific.com
Con riserva di modifiche tecniche
© Copyright 2008 3B Scientific GmbH
Page 9

3B SCIENTIFIC® PHYSICS
Balanza de inercia U30045
Instrucciones de uso
11/08 ALF
1 Abrazadera de mesa
2 Fleje elástico de acero rígido
3 Masas
1. Descripción
La balanza de inercia permite medir la masa
independientemente de la fuerza de gravedad
terrestre.
El aparato consta de dos bandejas de metal unidas
por flejes elásticos de acero rígido. Una bandeja tiene
3 agujeros, para sostener hasta tres pesas, y la otra se
puede suspender del borde de una mesa o banco de
laboratorio empleando la abrazadera, incluida en el
suministro, de tal forma que pueda oscilar
horitonzalmente. El período de oscilación depende de
la masa de la placa oscilante.
Después de la calibración realizada por medio de la
determinación de la frecuencia de oscilación de
objetos de masa conocida, el equipo se puede utilizar
para determinar valores de masas desconocidas.
2. Volumen de entrega
1 balanza de inercia
1 abrazadera de mesa
1 cuerda, 1,85 m
3 masas
3. Datos técnicos
Longitud del fleje de acero: aprox. 350 mm
Masas: c/u aprox. 175 g
4. Aparatos requeridos adicionalmente
1 Cronómetro mecánico, 15 min U40801
5. Manejo
La masa de un cuerpo es la cantidad de materia
contenida en él, mientras que su peso depende de la
fuerza de gravitación que actúa sobre él mismo. La
mayoría de los métodos de medición de la masa se
basan en las fuerzas que actúan sobre ellos, es decir
que se basan en el peso.
Por medio de la balanza de inercia se pueden
determinar masas directamente sin medir la fuerza de
gravitación que actúa sobre ellas.
• Se fija la balanza de inercia en el borde de una
mesa.
• La placa sin masas se desvía del reposo
lateralmente y se deja oscilar libremente.
1
Page 10

• Se mide el tiempo para 10 oscilaciones.
• Se repite tres veces la medición y se hace un
promedio de los resultados.
El período de una oscilación es el tiempo que la placa
necesita para realizar dos pasos consecutivos por la
posición de reposo en la misma dirección de
movimiento.
• Se repite el experimento con todas las
combinaciones posibles de las tres masas.
• Se anotan los resultados en una tabla.
• Se representa en un sistema de coordenadas el
período T en dependencia con la masa m.
• Se repite el experimento con una masa
desconocida y por medio de las gráficas se
determina el valor de la misma.
La balanza de inercia corresponde a un muelle
oscilante cuyo período se da por la ecuación 1:
T ⋅π=
D
m
22
4 (1)
con T = Período, m = Masa sobre el muelle, D =
Constante de elasticidad del muelle.
2
• T
se representa gráficamente en dependencia con
masa.
• La constante de elasticidad del muelle D se
obtiene de la pendiente de la gráfica.
• La masa desconocida se calcula en base a la
ecuación 1.
3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburgo • Alemania • www.3bscientific.com
Nos reservamos el derecho a cambios técnicos
© Copyright 2008 3B Scientific GmbH
Page 11

3B SCIENTIFIC® PHYSICS
Balança de inércia U30045
Manual de instruções
11/08 ALF
1 Grampo de mesa
2 Faixa de aço
3 Massas
1. Descrição
A balança de inércia permite a medição de massas
independentemente da força gravitacional da Terra.
A balança de inércia consiste em duas bandejas de
metal conectadas por faixa de molas de aço rígido.
Uma bandeja tem 3 orifícios para prender até três
massas e a outra pode ser presa na borda de uma
mesa ou de um banco de laboratório com o grampo
de mesa incluído no fornecimento, para que possa
oscilar horizontalmente. Nisto o período de oscilação
é dependente da massa da bandeja em oscilação.
Depois que o aparelho foi calibrado determinar a
freqüência de oscilação de objetos de massas
conhecidas.
2. Fornecimento
1 balança de inércia
1 grampo de mesa
1 corda, 1,85 m
3 massas
3. Dados técnicos
Comprimento da faixa de aço: aprox. 350 mm
Massas: cada uma aprox. 175 g
4. Aparelhos complementares exigidos
1 Cronômetro mecânico, 15 min U40801
5. Operação
A massa de um corpo e a quantidade de matéria
contida nele, enquanto o seu peso depende da força
de gravidade, que atua sobre ele. A maioria dos
métodos de medição de massas baseia-se nas forças
que eles exercem, é dizer, são baseados no seu peso.
Por meio da balança de inércia as massas podem ser
medidas diretamente, independente da gravitação
que atua sobre elas.
• Fixar a balança de inércia na mesa.
• Deslocar a bandeja sem as massas lateralmente e
causar uma oscilação horizontal nela.
• Medir o tempo de 10 oscilações.
1
Page 12

• Repetir a medição três vezes, depois mediar dos
resultados.
A duração do período T duma oscilação é o tempo,
que a bandeja precisa, desde a passagem a través do
ponto médio do seu equilibro até a próxima passagem
pelo mesmo ponto no mesmo sentido de movimento.
• Repetir a experiência em todas as combinações
das três massas.
• Anotar os resultados numa tabela.
• Representar graficamente num sistema de
coordenadas a duração do período T em
dependência da massa m.
• Repetir a experiência com a massa desconhecida
e determinar a grandeza da massa por meio do
gráfico.
A balança de inércia corresponde a uma mola em
oscilação, cuja duração de período é dada pela
equação 1:
m
22
T ⋅π=
4
(1)
D
com T = duração de período, m = massa sobre a
mola, D = constante de elasticidade da mola.
• Representar graficamente T
2
em dependência da
massa m.
• A constante de elasticidade da mola D resulta da
subida do gráfico.
• Calcular a massa desconhecida por meio da
equação 1.
3B Scientific GmbH ▪ Rudorffweg 8 ▪ 21031 Hamburgo ▪ Alemanha ▪ www.3bscientific.com
Sob reserva de alterações técnicas
© Copyright 2008 3B Scientific GmbH
 Loading...
Loading...