How it Works
Trane
Loading...
TRG-TRC007-EN
User Manual
82 pgs2.49 Mb0

Trane TRG-TRC007-EN User Manual

Air Conditioning Clinic
Fundamentals of HVAC Acoustics
One of the Fundamental Series
TRG-TRC007-EN
Comment Card
We want to ensure that our educational materials meet your ever-changing resource development needs. Please take a moment to comment on the effectiveness of this Air Conditioning Clinic.
Fundamentals of HVAC Acoustics
One of the Fundamental Series
TRG-TRC007-EN Content 12345678910
About me … Type of business _________________________________________________________
Give the completed card to the presenter or drop it in the mail. Thank you!
Level of detail (circle one) Too basic Just right Too difficult
Rate this clinic from 1–Needs Improvement to 10–Excellent
Booklet usefulness 12345678910
Slides/illustrations 12345678910
Presenter’s ability 12345678910
Training environment 12345678910
Other comments? _________________________________________________________ _______________________________________________________________________________ _______________________________________________________________________________
Job function _________________________________________________________ Optional: name _________________________________________________________
phone _________________________________________________________ address _________________________________________________________
Trane
An American Standard Company www.trane.com
For more information contact your local district office or e-mail us at comfort@trane.com
…
Response Card
We offer a variety of HVAC-related educational materials and technical references, as well as software tools that simplify system design/analysis and equipment selection. To receive information about any of these items, just complete this postage-paid card and drop it in the mail.
Education materials
Software tools
Periodicals
Other?
Thank you for your interest!
Air Conditioning Clinic series About me…
❏
Engineered Systems Clinic series Name _______________________________________________
❏
Trane Air Conditioning Manual Title _______________________________________________
❏
Trane Systems Manual Business type _______________________________________________
❏
Equipment Selection Phone/fax _____________________ ________________________
❏
System design & analysis E-mail address _______________________________________________
❏
Engineers Newsletter Company _______________________________________________
❏
_____________________________ Address _______________________________________________
❏
_______________________________________________ _______________________________________________
Trane
An American Standard Company www.trane.com
For more information contact your local district office or e-mail us at comfort@trane.com
Fundamentals of HVAC
Fundamentals of HVAC Acoustics
One of the Fundamental Series
A publication of Trane, an American Standard Company
Preface
Fundamentals of HVAC Acoustics
A Trane Air Conditioning Clinic
Figure 1
Trane believes that it is incumbent on manufacturers to serve the industry by regularly disseminating information gathered through laboratory research, testing programs, and field experience.
The Trane Air Conditioning Clinic series is one means of knowledge sharing. It is intended to acquaint a nontechnical audience with various fundamental aspects of heating, ventilating, and air conditioning (HVAC). We have taken special care to make the clinic as uncommercial and straightforward as possible. Illustrations of Trane products only appear in cases where they help convey the message contained in the accompanying text.
This particular clinic introduces the reader to the fundamentals of HVAC acoustics.
© 2001 American Standard Inc. All rights reserved
ii
TRG-TRC007-EN
Contents
period one Fundamentals of Sound ..................................... 1
What is Sound? ....................................................... 2
Octave Bands ......................................................... 6
Sound Power and Sound Pressure ........................... 9
period two Sound Perception and Rating Methods ...... 15
Human Ear Response ............................................ 15
Single-Number Rating Methods ............................. 17
Octave-Band Rating Method .................................. 28
period three Acoustical Analysis ............................................ 29
Setting a Design Goal ............................................ 30
Source–Path–Receiver Analysis ............................. 32
Sound-Path Modeling ............................................ 37
Terms Used in Sound-Path Modeling ..................... 41
period four Equipment Sound Rating ................................ 47
Fields of Measurement ......................................... 48
HVAC Equipment Sound Rating ............................. 54
period five Review ................................................................... 63
Quiz ......................................................................... 68
Answers ................................................................ 70
Glossary ................................................................ 71
TRG-TRC007-EN iii
iv TRG-TRC007-EN
notes
period one
Fundamentals of Sound
Fundamentals of HVAC Acoustics
period one
Fundamentals of Sound
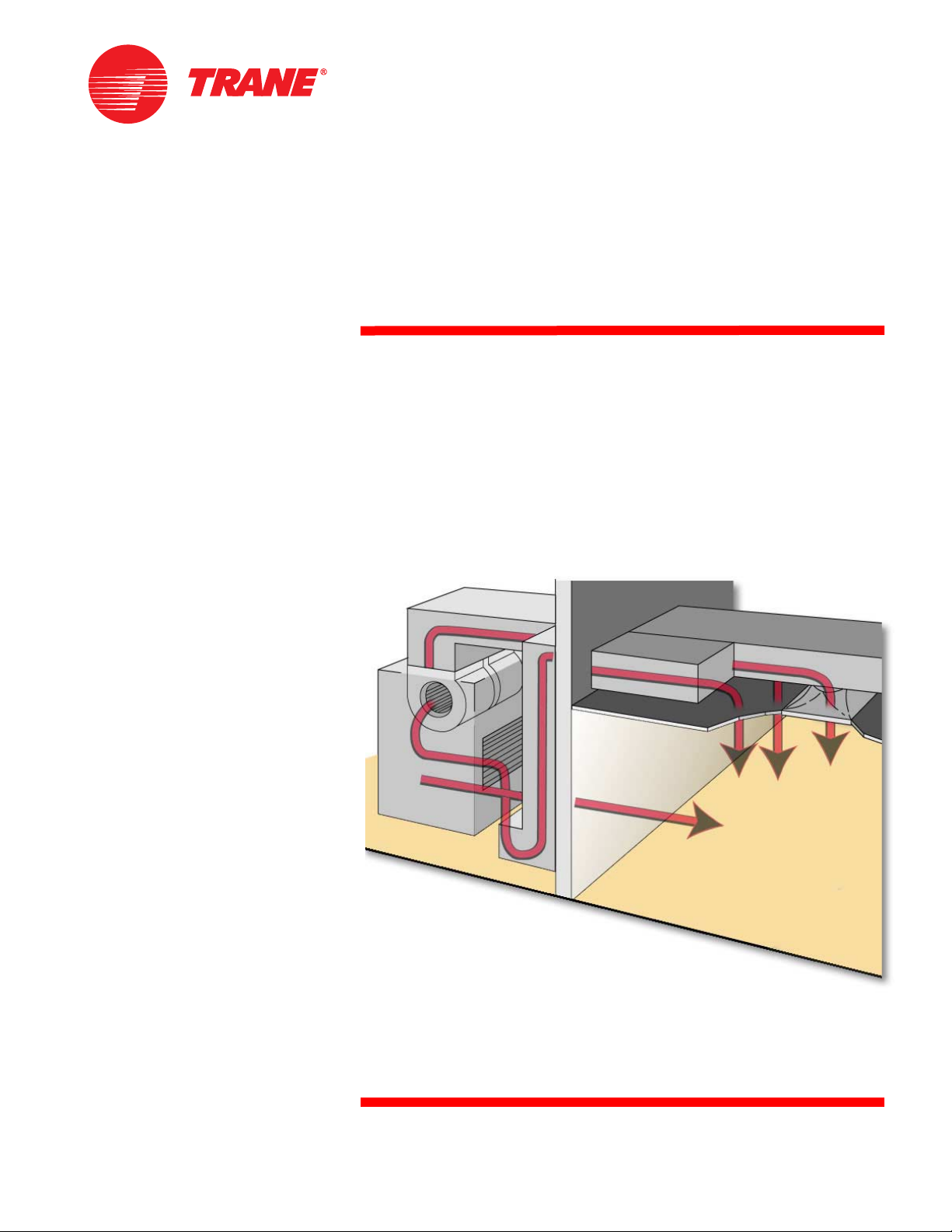
Figure 2
People have become increasingly conscious of acoustics as a component of a comfortable environment. Sound levels, both indoor and outdoor, can be affected to varying degrees by HVAC equipment and systems.
The degree to which the HVAC system affects the sound at a particular location depends on the strength of the sound source and the environmental effects on the sound as it travels from that source to the listener.
TRG-TRC007-EN 1
notes
period one
Fundamentals of Sound
What is Sound?
I Audible emissions resulting from vibration of
molecules within an elastic medium
I Generated by vibrating surface or movement
of a fluid
I In buildings, it may be airborne or structure-
borne
I Noise is unwanted sound
Figure 3
What is Sound?
Sound is the audible emissions resulting from the vibration of molecules
within an elastic medium. It is generated by either a vibrating surface or the movement of a fluid. In the context of building HVAC systems, this elastic medium can be either air or the building structure. For structurally-borne sound to become audible, however, it must first become airborne.
Noise is different than sound. Sound is always present, but is not always obtrusive. Noise is defined as unwanted sound. Generally, people object to sound when it interferes with speech, concentration, or sleep.
2 TRG-TRC007-EN
notes
period one
Fundamentals of Sound
Sound Wave and Frequency
one cycle
one cycle
cycles
cycles
seconds
seconds
amplitude
amplitude
+
+
-
-
frequency, Hz==
frequency, Hz
time
time
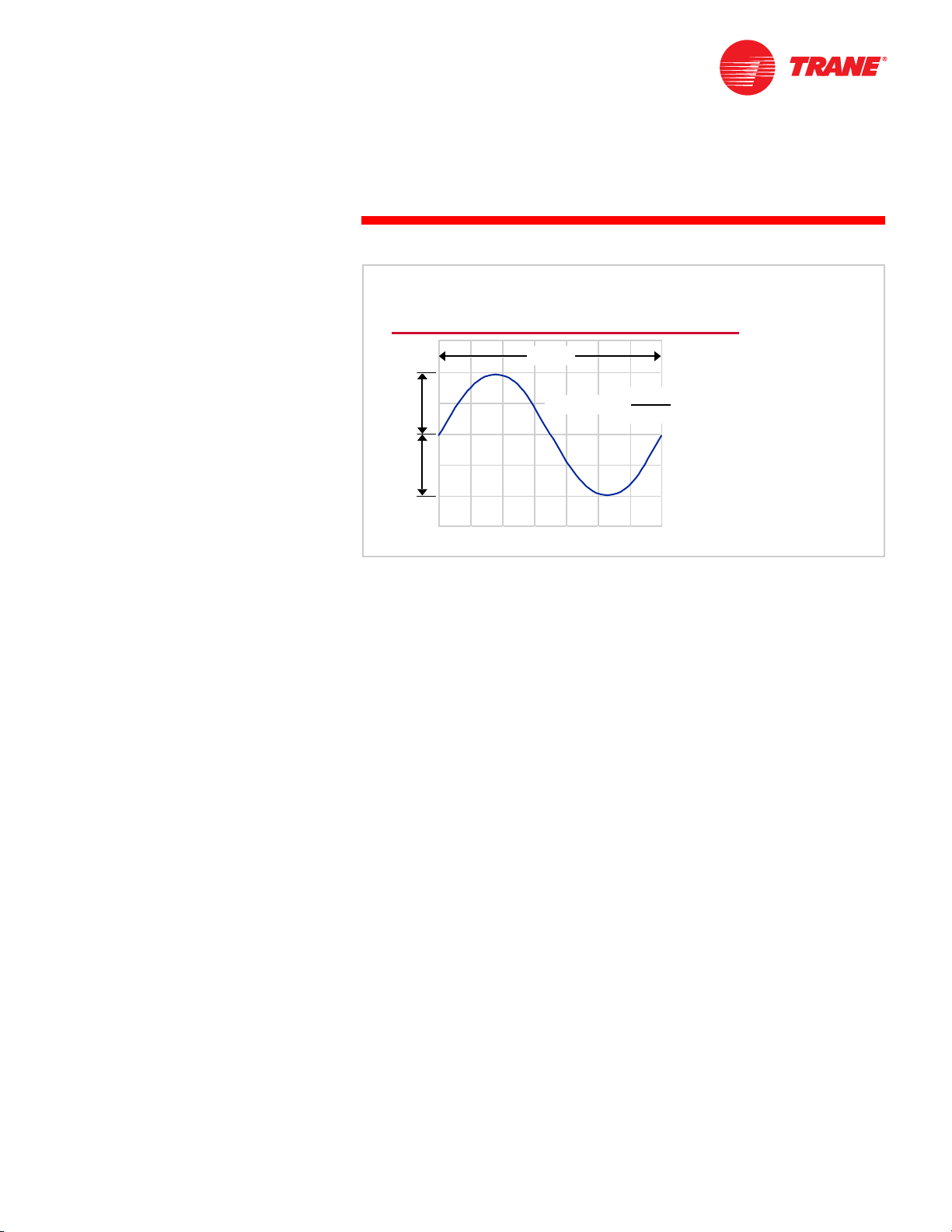
Airborne sound is transmitted away from a vibrating body through the transfer of energy from one air molecule to the next. The vibrating body alternately compresses and rarefies (expands) the air molecules. The pressure fluctuations that result from the displacement of these air molecules take the form of a harmonic, or sine, wave. The amplitude of the wave depicts pressure. The higher the amplitude, the louder the sound.
This transfer of energy takes time. Each complete sequence of motion (compression and rarefaction) constitutes a cycle, and the time required to complete one cycle is the cycle period. The frequency of the periodic motion is the number of cycles that occur in a second. The unit of measure for frequency is the hertz (Hz). One hertz is equal to one cycle per second.
cycles
frequency, Hz
The terms pitch and frequency are often (incorrectly) used interchangeably. Frequency is an objective quantity that is independent of sound-pressure level. Pitch, however, is a subjective quantity that is primarily based on frequency, but is also dependent on sound-pressure level and composition. Pitch is not measured, but is described with terms like bass, tenor, and soprano.
-----------------------
=
seconds
Figure 4
TRG-TRC007-EN 3
notes
period one
Fundamentals of Sound
Wavelength
one cycle
one cycle
speed of sound
wavelength
wavelength
The wavelength of the sound is the linear measurement of one complete cycle. The wavelength and frequency of a sound are related by using the following equation:
speed of sound
=
=
frequency
frequency
Figure 5
speed of sound
wavelength
The speed of sound transmission is a physical property of the medium. For air, the speed varies slightly with temperature change. Because the temperature range encountered in the study of HVAC acoustics is relatively small, the speed of sound can be considered a constant 1,127 ft/s (344 m/s). For example, sound traveling through the air at a frequency of 200 Hz has a wavelength of 5.6 ft (1.7 m).
wavelength
æö
wavelength
èø
---------------------------- ---------------
=
frequency
1,127 ft/s
-------------------------
= 5.6 ft=
200 Hz
344 m/s
--------------------- -
200 Hz
1.7 m==
4 TRG-TRC007-EN
notes
period one
Fundamentals of Sound
Broadband Sound
amplitude
amplitude
time
time
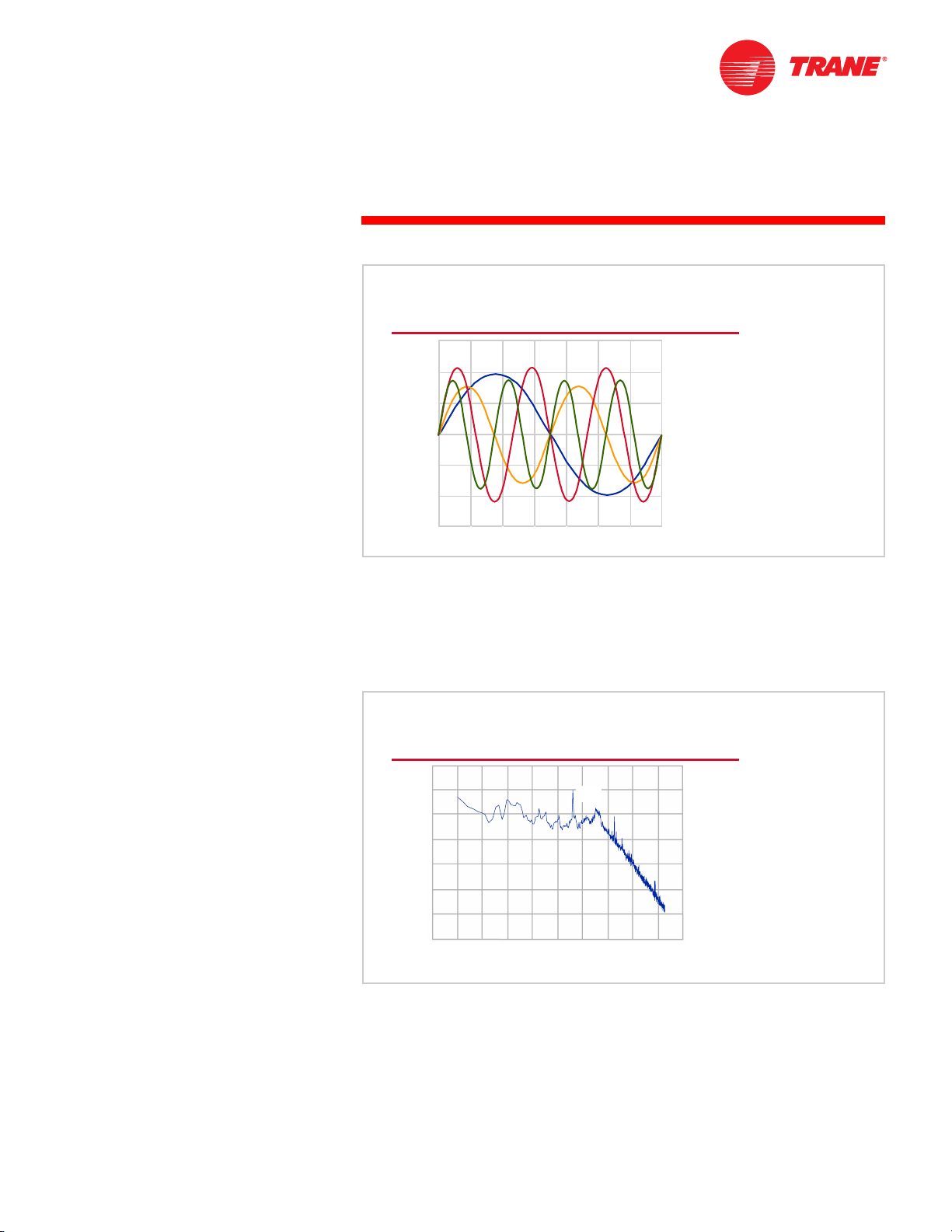
The wave form shown in Figure 5 represents sound occurring at a single frequency. This is called a pure tone.
A pure sinusoidal wave form, however, is very rare in HVAC acoustics. Typically, sounds are of a broadband nature, meaning that the sound is composed of several frequencies and amplitudes, all generated at the same time. Figure 6 represents the components of broadband sound.
Figure 6
Broadband Sound and Tones
tone
tone
amplitude
amplitude
logarithmic scale
frequency
frequency
logarithmic scale
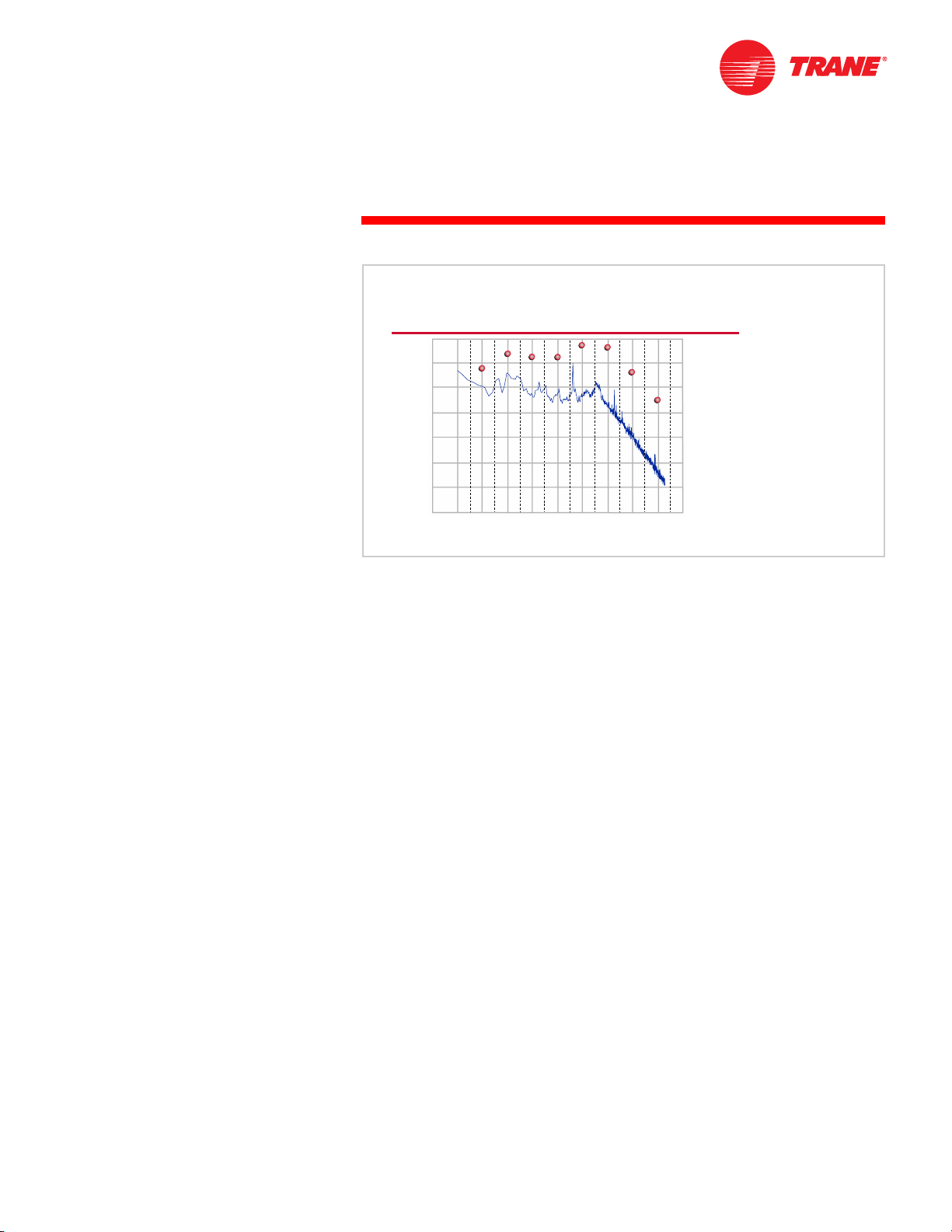
Figure 7
Alternatively, plotting the amplitude (vertical axis) of each sound wave at each frequency (horizontal axis) results in a graphic of the broadband sound that looks like this. As you can see from this example, the sound energy is greater at some frequencies than at others.
TRG-TRC007-EN 5
period one
Fundamentals of Sound
notes
Again, a pure tone has a single frequency. If a sound in a narrow band of frequencies is significantly greater than the sound at adjacent frequencies, it would be similar to a tone. Tones that stand out enough from the background sound can be objectionable. Many of the sounds generated by HVAC equipment and systems include both broadband and tonal characteristics.
Octave Bands
octave
octave
band
band
1
1
2
2
3
3
4
4
5
5
6
6
7
7
8
8
center
center
frequency (Hz)
frequency (Hz)
63
63
125
125
250
250
500
500
1,000
1,000
2,000
2,000
4,000
4,000
8,000
8,000
Octave Bands
Because sound occurs over a range of frequencies, it is considerably more difficult to measure than temperature or pressure. The sound must be measured at each frequency in order to understand how it will be perceived in a particular environment. The human ear can perceive sounds at frequencies ranging from 20 to 16,000 Hz, whereas, HVAC system designers generally focus on sounds in the frequencies between 45 and 11,200 Hz. Despite this reduced range, measuring a sound at each frequency would result in 11,156 data points.
frequency
frequency
range (Hz)
range (Hz)
45 to 90
45 to 90
90 to 180
90 to 180
180 to 355
180 to 355
355 to 710
355 to 710
710 to 1,400
710 to 1,400
1,400 to 2,800
1,400 to 2,800
2,800 to 5,600
2,800 to 5,600
5,600 to 11,200
5,600 to 11,200
Figure 8
For some types of analyses, it is advantageous to measure and display the sound at each frequency over the entire range of frequencies being studied. This is called a full-spectrum analysis and is displayed like the example shown in Figure 7.
To make the amount of data more manageable, this range of frequencies is typically divided into smaller ranges called octave bands. Each octave band is defined such that the highest frequency in the band is two times the lowest frequency. The octave band is identified by its center frequency, which is calculated by taking the square root of the product of the lowest and highest frequencies in the band.
center frequency lowest frequency × highest frequency =
The result is that this frequency range (45 to 11,200 Hz) is separated into eight octave bands with center frequencies of 63, 125, 250, 500, 1,000, 2,000, 4,000, and 8,000 Hz. For example, sounds that occur at the frequencies between 90 Hz and 180 Hz are grouped together in the 125 Hz octave band.
6 TRG-TRC007-EN
notes
period one
Fundamentals of Sound
Octave Bands
logarithmic
logarithmic
sums
sums
amplitude
amplitude
125
63
125
63
500
250
250
500
frequency, Hz
frequency, Hz
1,000
1,000
2,000
2,000
4,000
4,000
8,000
8,000
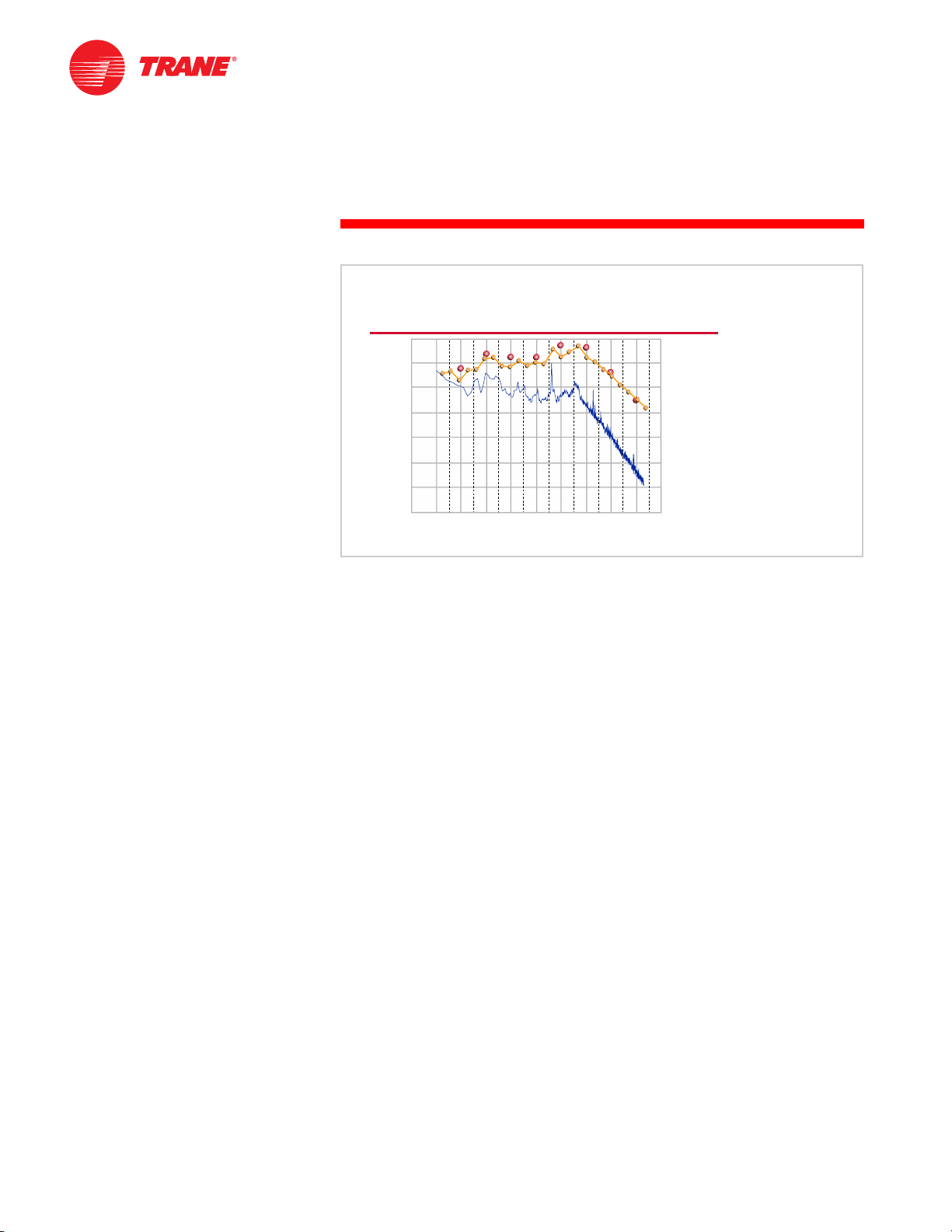
Figure 9
Octave bands compress the range of frequencies between the upper and lower ends of the band into a single value. Sound measured in an octave band is the logarithmic sum of the sound level at each of the frequencies within the band.
Unfortunately, octave bands do not indicate that the human ear hears a difference between an octave that contains a tone and one that does not, even when the overall magnitude of both octaves is identical. Therefore, the process of logarithmically summing sound measurements into octave bands, though practical, sacrifices valuable information about the “character” of the sound.
TRG-TRC007-EN 7
notes
period one
Fundamentals of Sound
One-Third Octave Bands
amplitude
amplitude
125
63
125
63
500
250
250
500
frequency, Hz
frequency, Hz
1,000
1,000
2,000
2,000
4,000
4,000
8,000
8,000
Figure 10
Middle ground between octave-band analysis and full-spectrum analysis is provided by one-third octave-band analysis. One-third octave bands divide the full octaves into thirds. The upper cutoff frequency of each third octave is greater than the lower cutoff frequency by a factor of the cube root of two (approximately 1.2599). If tones are contained in the broadband sound, they will be more readily apparent in the third octaves.
The use of octave bands is usually sufficient for rating the acoustical environment in a given space. One-third octave bands are, however, more useful for product development and troubleshooting acoustical problems.
8 TRG-TRC007-EN
notes
period one
Fundamentals of Sound
Sound Power and Sound Pressure
I Sound power
K Acoustical energy emitted by the sound source
K Unaffected by the environment
I Sound pressure
K Pressure disturbance in the atmosphere
K Affected by strength of source, surroundings, and
distance between source and receiver
Figure 11
Sound Power and Sound Pressure
Sound power and sound pressure are two distinct and commonly confused characteristics of sound. Both are generally described using the term decibel (dB), and the term “sound level” is commonly substituted for each. To understand how to measure and specify sound, however, one must first understand the difference between these two properties.
Sound power is the acoustical energy emitted by the sound source, and is expressed in terms of watts (W). It is not affected by the environment.
Sound pressure is a pressure disturbance in the atmosphere, expressed in terms of pascals (Pa), that can be measured directly. Sound pressure magnitude is influenced not only by the strength of the source, but also by the surroundings and the distance from the source to the listener. Sound pressure is what our ears hear and what sound meters measure.
While sound-producing pressure variations within the atmosphere can be measured directly, sound power cannot. It must be calculated from sound pressure, knowing both the character of the source and the modifying influences of the environment.
TRG-TRC007-EN 9
notes
period one
Fundamentals of Sound
An Analogy
I Sound power
K Correlates to bulb wattage
I Sound pressure
K Correlates to brightness
Figure 12
The following comparison of sound and light may help illustrate the distinction between these two properties. Think of sound power as the wattage rating of a light bulb. Both measure a fixed amount of energy. Whether you put a 100-watt light bulb outdoors or in a closet, it is always 100-watt light bulb and always gives off the same amount of light.
Sound pressure corresponds to the brightness, from the light emitted by the light bulb, in a particular location in the room. Both sound pressure and brightness can be measured with a meter, and the immediate surroundings influence the magnitude of each. In the case of light, brightness depends on more than the wattage of the bulb. It also depends on how far the observer is from the light bulb, the color of the room, how reflective the wall surfaces are, and whether the light bulb is covered with a shade. These other factors affect how much light reaches the receiver, but do not affect the wattage of the light bulb.
Similarly, sound pressure depends not only on the sound power emitted by the source, but also on the characteristics of the surrounding environment. These might include the distance between the sound source and the listener, whether the room is carpeted or tiled, and whether the room is furnished or bare. Just as with light, environmental factors like these affect how much sound reaches the listener.
10 TRG-TRC007-EN
notes
period one
Fundamentals of Sound
Decibel
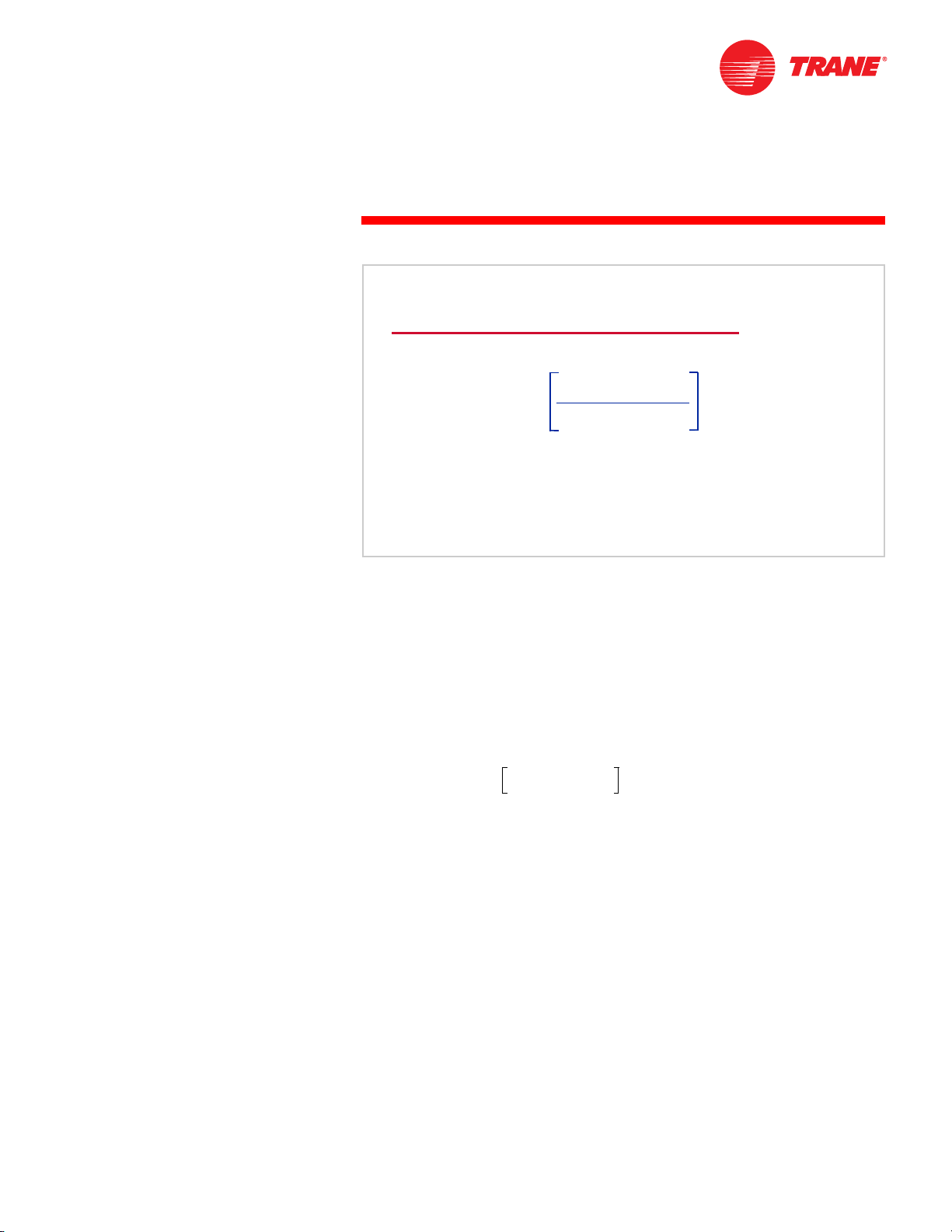
dB = 10 log
Sounds encompass a wide range of volumes, or levels. The loudest sound the human ear can hear without damage due to prolonged exposure is about 1,000,000,000 times greater than the quietest perceptible sound. A range of this magnitude makes using an arithmetic scale cumbersome, so a logarithmic scale is used instead.
The measurement of sound level is expressed in terms of decibels (dB), a dimensionless quantity. A decibel is a calculated value based on the ratio of two quantities. It is defined as ten times the logarithm to the base ten (log measured quantity divided by the reference quantity. The reference quantity must be specified to prevent confusion regarding the magnitude of the ratio.
measured value
dB 10 log
=
--------------------------- ------------------
10
reference value
measured value
10
reference value
Figure 13
) of the
10
TRG-TRC007-EN 11
notes
period one
Fundamentals of Sound
Logarithmic Scale
ratio
ratio
1
1
10
10
100
100
1,000
1,000
10,000
10,000
100,000
100,000
1,000,000
1,000,000
10,000,000
10,000,000
100,000,000
100,000,000
1,000,000,000
1,000,000,000
log
log
10
10
0
0
1
1
2
2
3
3
4
4
5
5
6
6
7
7
8
8
9
9
A logarithm is the exponent power of the base. In this case, the base is ten. For example, the log the log
of 1,000,000,000 (or 109) equals 9.
10
of 10 (or 101) equals 1, the log10 of 100 (or 102) equals 2, and
10
As mentioned earlier, the loudest sound the human ear can hear without damage due to prolonged exposure is about 1,000,000,000 times greater than the quietest perceptible sound. If we use the quietest perceptible sound as the reference value, this ratio would range from 1 to 1,000,000,000. Converting this arithmetic range to a log
scale yields a range of 0 to 9. This unitless result is
10
described in terms of bels. Multiplying by ten results in the more-commonly used broader range of 0 to 90 decibels (dB
10 ´´´´ log
10 ´´´´ log
0
0
10
10
20
20
30
30
40
40
50
50
60
60
70
70
80
80
90
90
10
10
Figure 14
).
12 TRG-TRC007-EN
notes
period one
Fundamentals of Sound
Equation for Sound Power
Lw= 10 log
When a reference value is established and placed in the denominator of the ratio, the dB can be calculated for any value entered into the numerator.
The reference value used for calculating sound-power level is 1 picowatt (pW),
-12
or 10
watts. Therefore, sound-power level (Lw) in dB is calculated using the
following equation:
L
10 log
=
W
10
sound power, watts
---------------------------- ----------------------------
10
10
-12
watts
10
-12
W
Figure 15
Equation for Sound Pressure
sound power, W
Lp= 20 log
The reference value used for calculating sound-pressure level is 20 micropascals (µPa), or 2 ×10 is calculated using the following equation:
TRG-TRC007-EN 13
10
-5
Pa. Therefore, sound-pressure level (Lp) in dB,
20 mmmmPa
Figure 16
sound pressure, mmmmPa
period one
Fundamentals of Sound
notes
sound pressure, µPa
20 log
=
L
p
--------------------------- ---------------------------- -- -
10
20 Pa
or 10 log
sound pressure, µPa
---------------------------- --------------------------- -- -
10
20 Pa
2
Again, these reference values can be considered the threshold of hearing. The multiplier 20 is used in the sound-pressure level equation instead of 10 because sound power is proportional to the square of sound pressure.
Logarithmic Addition of Decibels
5
5
4
4
3
3
2
2
1
1
0
0
add to the higher dB value
add to the higher dB value
0
0
5
5
dB difference between values being added
dB difference between values being added
50 dB + 44 dB = 51 dB
Measuring sound using a logarithmic scale means that decibel values cannot be added arithmetically. Instead, logarithmic addition must be used to add two or more sound levels. This involves converting the decibel values into ratios of sound intensity, adding these ratios, and then converting the sum back into decibels. The mathematics become rather involved—the graph in Figure 17 has been developed to simplify the procedure.
To demonstrate the use of this figure, consider the example of adding a 50 dB sound to a 44 dB sound. The difference between these two sounds is 6 dB. Therefore, 1 dB is added to the higher of the two sounds (50 plus 1) to arrive at the logarithmic sum of 51 dB.
10
10
15
15
Figure 17
Also, notice that the logarithmic sum of two sounds of equal magnitude (0 dB difference) results in a 3 dB increase. Therefore, adding two 50 dB sounds would result in a combined sound level of 53 dB.
14 TRG-TRC007-EN
notes
period two
Sound Perception and Rating Methods
Fundamentals of HVAC Acoustics
period two
Sound Perception and Rating Methods
Figure 18
The study of acoustics is affected by the response of the human ear to sound pressure. Unlike electronic sound-measuring equipment, which provides a repeatable, unbiased analysis of sound pressure, the sensitivity of the human ear varies by frequency and magnitude. Our ears are also attached to a highly arbitrary evaluation device, the brain.
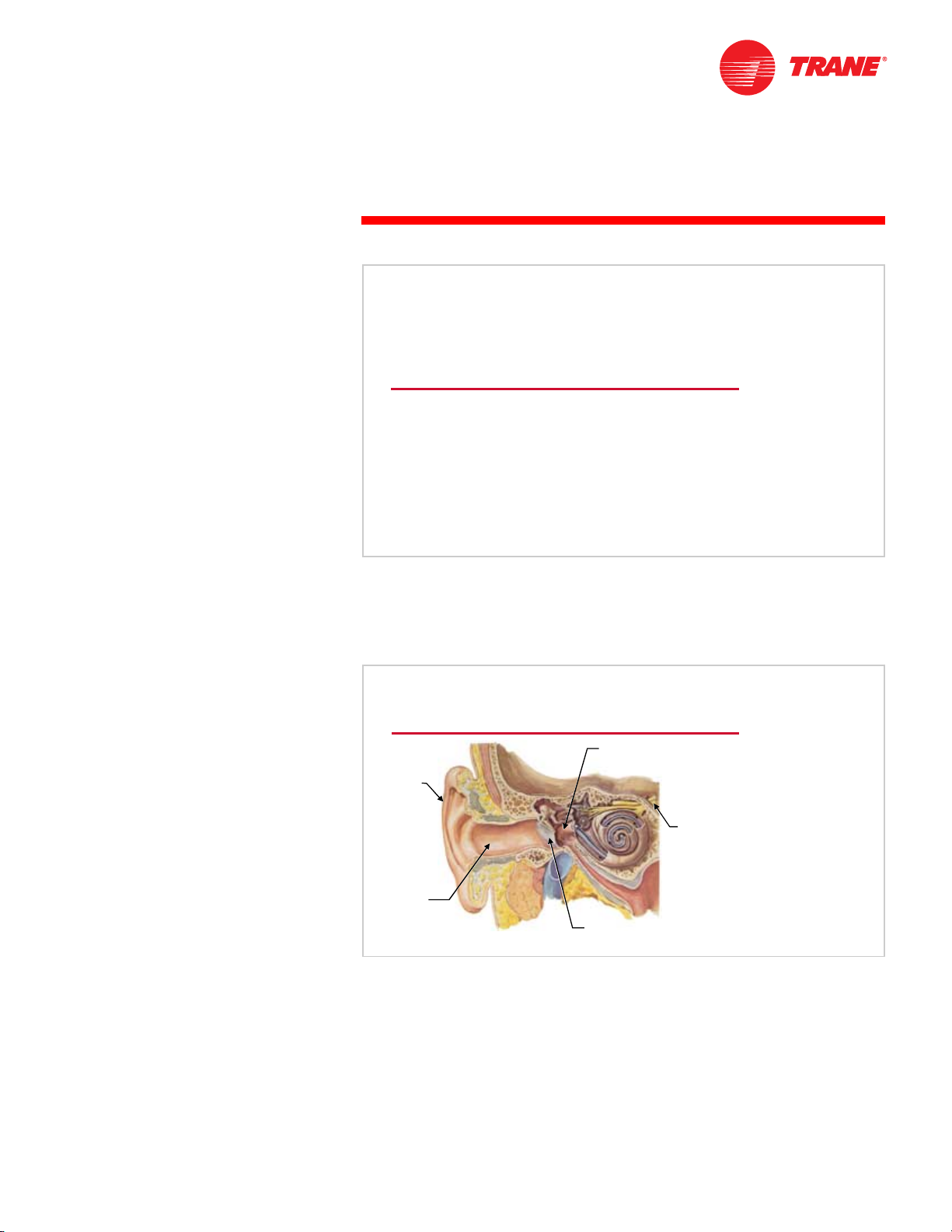
The Human Ear
middle
middle
middle
ear
ear
ear
outer
outer
outer
ear
ear
ear
nerves
nerves
nerves
to brain
to brain
to brain
auditory
auditory
auditory
canal
canal
canal
Human Ear Response
The ear acts like a microphone. Sound waves enter the auditory canal and impinge upon the ear drum, causing it to vibrate. These vibrations are ultimately transformed into impulses that travel along the auditory nerve to the brain, where they are perceived as sound. The brain then analyzes and evaluates the signal.
eardrum
eardrumeardrum
Figure 19
TRG-TRC007-EN 15
notes
period two
Sound Perception and Rating Methods
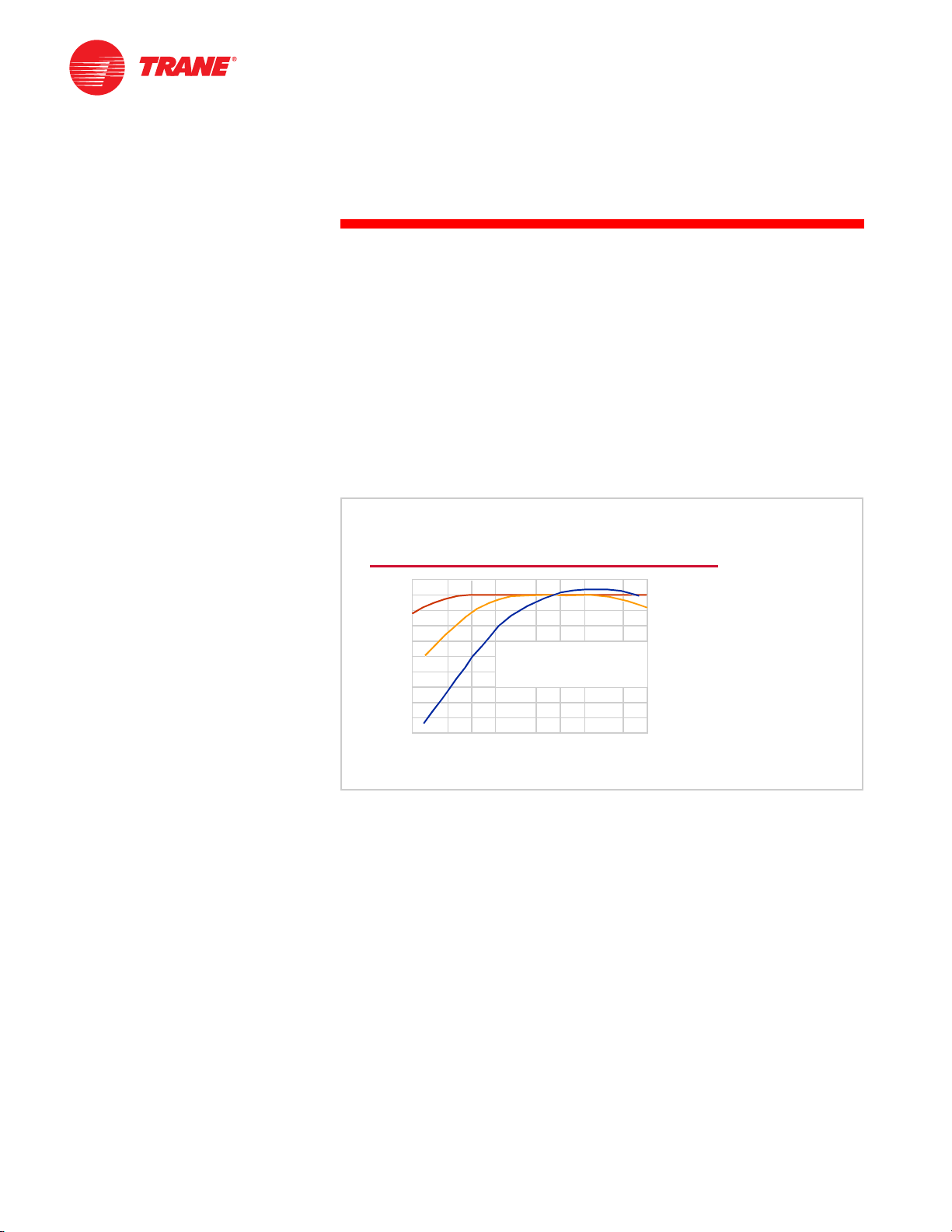
Loudness Contours
120
120
Pa
Pa
m
m
100
100
80
80
60
60
40
40
sound pressure, dB ref 20
sound pressure, dB ref 20
20
20
0
0
20
20
100
5050100
200
200
frequency, Hz
frequency, Hz
500
500
1,000
1,000
2,000
2,000
5,000
5,000
10,000
10,000
Figure 20
The sensation of loudness is principally a function of sound pressure, however, it also depends upon frequency. As a selective sensory organ, the human ear is more sensitive to high frequencies than to low frequencies. Also, the ear’s sensitivity at a particular frequency changes with sound-pressure level. Figure 20 illustrates these traits using a set of contours. Each contour approximates an equal loudness level across the frequency range shown.
For example, a 60 dB sound at a frequency of 100 Hz is perceived by the human ear to have loudness equal to a 50 dB sound at a frequency of 1,000 Hz. Also, notice that the contours slant downward as the frequency increases from 20 to 200 Hz, indicating that our ears are less sensitive to low-frequency sounds. The contours are flatter at higher decibels (> 90 dB), indicating a more uniform response to “loud” sounds across this range of frequencies.
As you can see, the human ear does not respond in a linear manner to pressure and frequency.
16 TRG-TRC007-EN
notes
period two
Sound Perception and Rating Methods
Response to Tones
Figure 21
Additionally, tones evoke a particularly strong response. Recall that a tone is a sound that occurs at a single frequency. Chalk squeaking on a blackboard, for example, produces a tone that is extremely irritating to many people.
Single-Number Rating Methods
I A-, B-, and C-weighting
I Noise criteria (NC) curves
I Room criteria (RC) curves
I Sones
I Phons
Figure 22
Single-Number Rating Methods
The human ear interprets sound in terms of loudness and pitch, while electronic sound-measuring equipment interprets sound in terms of pressure and frequency. As a result, considerable research has been done in an attempt to equate sound pressure and frequency to sound levels as they are perceived by the human ear. The goal has been to develop a system of single-number descriptors to express both the intensity and quality of a sound.
With such a system, sound targets can be established for different environments. These targets aid building designers in specifying appropriate acoustical requirements that can be substantiated through measurement. For example, a designer can specify that “the background sound level in the theater
TRG-TRC007-EN 17
period two
Sound Perception and Rating Methods
notes
shall be X,” where X is a single-number descriptor conveying the desired quality of sound.
The most frequently used single-number descriptors are the A-weighting network, noise criteria (NC), and room criteria (RC). All three share a common problem, however: they unavoidably lose valuable information about the character, or quality, of sound. Each of these descriptors is based on octave­band sound data which, as noted earlier, may already mask tones. Further, the process of converting from eight octave bands to a single number overlooks even more sound data.
Despite this shortcoming, the single-number descriptors summarized in this clinic are valuable tools for defining sound levels in a space, and are widely used to specify the acoustical requirement of a space.
A–B–C Weighting
C
C
0
0
10
--10
B
B
20
relative response, dB
relative response, dB
--20
30
--30
A
A
40
--40
20
5050100
20
frequency responses for sound
frequency responses for sound
meter weighting characteristics
meter weighting characteristics
100
200
500
500
frequency, Hz
frequency, Hz
1,000
1,000
200
2,000
2,000
5,000
5,000
10,000
10,000
Figure 23
One simple method for combining octave-band sound data into a single­number descriptor is A-, B-, or C-weighting. The weighting curves shown in Figure 23 compensate for the varying sensitivity of the human ear to different frequencies.
A-weighting, which is most appropriately used for low-volume (or quiet) sound levels, best approximates human response to sound in the range where no hearing protection is needed. B-weighting is used for medium-volume sound levels. C-weighting is used for high-volume (or loud) sound levels where the response of the ear is relatively flat.
18 TRG-TRC007-EN
Loading...
+ 57 hidden pages
Buy Points How it Works FAQ Contact Us Questions and Suggestions Privacy Policy Cookie Policy Terms of Use