Datasheet FPS Datasheet (Fairchild)

www.fairchildsemi.com

Application Note AN4140

Transformer Design Consideration for off-line Flyback Converters using Fairchild Power Switch (FPS

1. Introduction

For flyback coverters, the transformer is the most important factor that determines the performance such as the efficiency, output regulation and EMI. Contrary to the normal transformer, the flyback transformer is inherently an inductor that provides energy storage, coupling and isolation for the flyback converter. In the general transformer, the current flo ws in bot h the prim ary and se condary wi nding at the same time. However, in the flyback transformer, the current flows only in the primary winding while the energy in the core is charged and in the secondary windin g while the energy in the core is discharged. Usually gap is introduced between the core to increase the energy storage capacity.

This paper presents practical design considerations of transformers for off-line flyback converters employing Fairchild Power Switch (FPS). In order to give insight to th e reader, practical design examples are also provided.

2. General Transformer design procedure

(1) Choose the proper core Core type

for commercial SMPS (Switchied mode power supply) applications. Various ferrite cores and bobbins are shown in Figure 1. The type of the core should be chosen with regard to system requirements including number of outputs, physical height, cost and so on. Table 1 shows features and typical application of various cores.

: Ferrite is the most widely used core material

Core Features Typical Applications EEEI-Low cost Aux. power

EFD

-Low profile LCD Monitor

EPC EER -Large winding window area

-Various bobbins for multiple output

PQ -Large cross sectional area

-Relatively expensive

Table 1. Features and typical applications of various cores

Core size : Actually, the initial selection of the core is

bound to be crude since there are too many variables. One way to select the proper core is to refer to the manufacture's core selection guide. If there is no proper reference, use the table 2 as a starting point. The core recommended in table 1 is typical for the universal input range, 67kHz switching frequency and 12V singl e outp ut app lication . When th e in put voltage range is 195-265 Vac (European input range) or the switching frequency is higher than 67 kHz, a small er core can be used. For an application with low voltage and/or mul tiple outputs, usually a larger core should be used than recommended in the table.

Output Power

0-10W EI12.5

10-20W EI22 EE19 EPC19 20-30W EI25 EE22 EPC25 EER25.5 30-50W EI28

50-70W EI35 EE30 EER28L 70-100W EI40 EE35 EER35 100-150W EI50 EE40 EER40

150-200W EI60 EE50

EI core EE core EPC core EER core

EE8 EI16 EI19

EI30

EE10

EE13

EE16

EE25 EPC30 EER28

EE60

)

Battery charger

CRT monitor, C-TV DVDP, STB

EPC10 EPC13 EPC17

EER42 EER49

Figure 1. Ferrite core (TDK)

Table 2. Core quick selection table (For universal input range, fs=67kHz and 12V single output)

Rev. 1.0.0

AN4140 APPLICATION NOTE

∆

Once the core type and size are determined, the following variables are obtained from the core data sheet.

- A

: The cross-sectional area of the core (mm2)

- A

: Winding window area (mm2)

- B

: Core saturation flux density (tesla)

sat

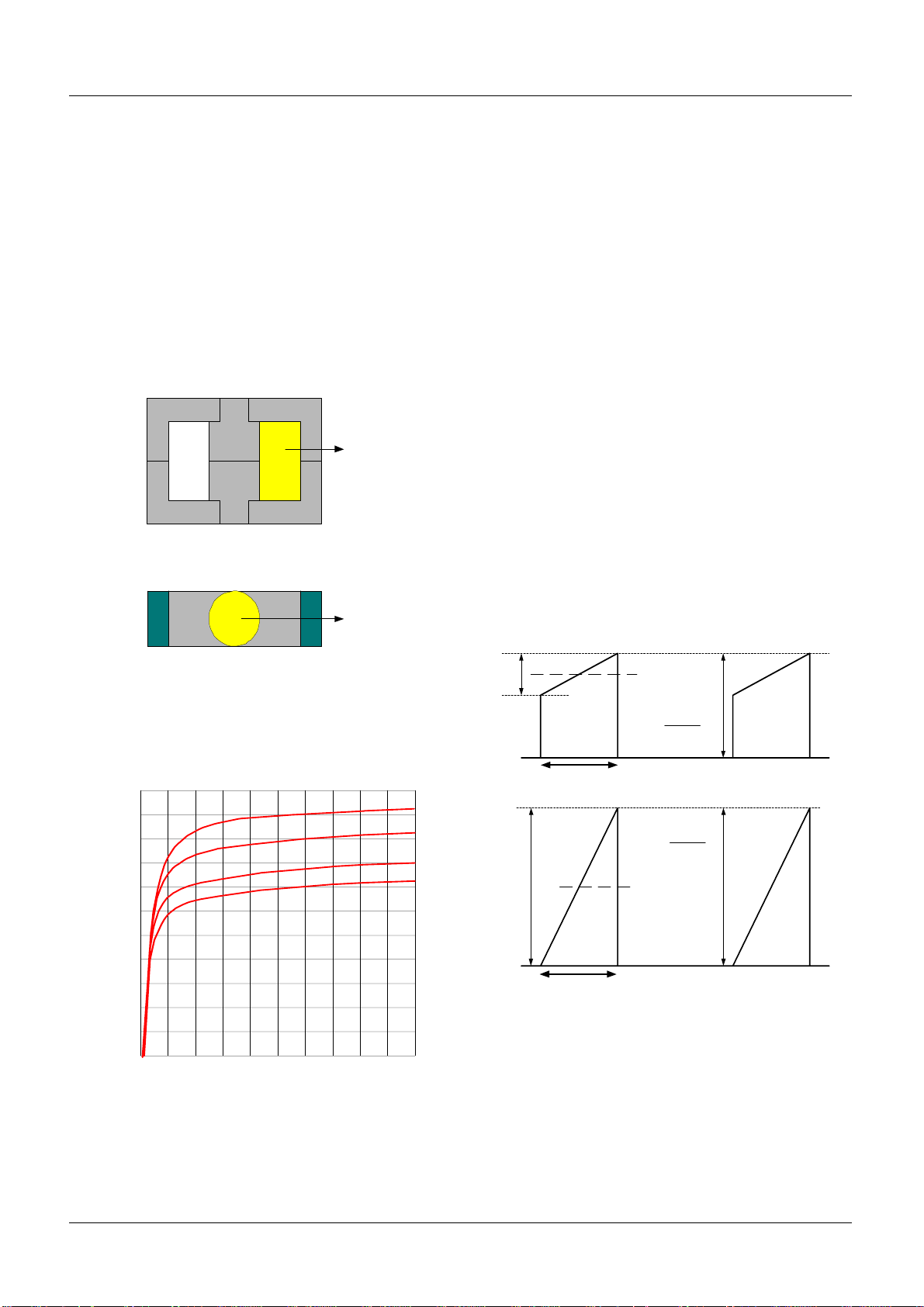
Figure 2 shows the Ae and A

of a core. The typical B-H

characteristics of ferrite core from TDK (PC40) are shown in Figure 3. Since the saturation flux density (B

) decreases as

sat

the temperature increases, the high temperature characteristics should be considered. If there is no reference data, use

=0.3~0.35 T.

sat

AwAw

AeAe

(2) Determine the primary side inductance (Lm) of the transformer

In order to determine the primary side inductance, the following variables should be determined first. (For a detailed design procedure, please refer to the application note AN4137.)

- P

: Maximum input power

- f

: Switching frequency of FPS device

min

- V

- D

- K

: Minimum DC link voltage

: Maximum duty cycle

max

: Ripple factor, which is defined at the minimum input

voltage and full load condition, as shown in Figure 4. For DCM opera tion, K

= 1 and for CCM operation KRF < 1.

The ripple factor is closely related with the transformer size and the RMS value of the MOSFET current. Even though the conduction loss in the MOSFET can be reduced through reducing the ripple factor, too small a ripple factor forces an increase in transformer size. Considering both efficiency and core size, it is reasonable to set K universal input range and K

= 0.4-0.8 for the European

= 0.3-0.5 for the

input range. Meanwhile, in the case of low power applications below 5W where size is most critical, a relatively large ripple factor is used in order to minimize the transformer size. In that case, it is typical to set K

0.7 for the universal input range and K

= 1.0 for the

= 0.5-

European input range.

Figure 2. Window Area and Cross Sectional Area

Magnetization Curves (typical)

Material :PC40

500

400

300

200

Flux density B (mT)

100

Figure 3. Typical B-H characteristics of ferrite core

(TDK/PC40)

800 1600

Magnetic field H (A/m)

25 ℃℃℃℃

60 ℃℃℃℃

100 ℃℃℃℃

120 ℃℃℃℃

peak

EDC

peak

EDC

max

Figure 4. MOSFET Drain Current and Ripple Factor (KRF)

CCM operation : KRF < 1

EDC

DCM operation : KRF =1

With the given variables, the primary side inductance, Lm is obtained as

min

()

--------------------------------------------- -= (1) 2PinfsK

⋅

max

APPLICATION NOTE AN4140

where V maximum duty cycle, P the switching frequency of the FPS device and K

min

is the minimum DC input voltage, D

is the maximum input power, fs is

max

is the

ripple factor. Once L

is determined, the maximum peak cur rent and RMS

current of the MOSFET in normal operation are obtained as

peak

rms

where I

and

()

∆

-----+= (2)

EDC

------------------------------------- -=4()

EDC

min

-----------------------------------= (5) Lmf

∆

 

max

-----

------------- -=3()

min

⋅

max

With the chosen core, the minimum number of turns for the transformer primary side to avoid the core saturation is given by

LmI

min

over

-------------------

= (6)

satAe

(turns)

(3) Determine the number of turns for each output

Figure 6 shows the simplified diagram of the transformer, whrere V by the feedback control while V

stands for the reference output th at is regulated

stands for the n-th

o(n)

output. First, determine the turns ratio (n) between the primary side

and the feedback controlled secondary side as a reference.

--------------------------

Vo1VF1+

where N

and Ns1 are the number of turns for primary side

and reference output, respectively, V and V

is the diode (DR1) forward voltage drop of the

---------= = (7) N

is the output voltage

reference output that is regulated by the feedback control. Then, determine the proper integer for N

resulting Np is larger than N

min

obtained from equation (6).

so that the

The number of turns for the other output (n-th output) is determined as

sn()

on()VFn()

---------------------------------=N V

o1VF1

⋅

turns() 8()

The number of turns for Vcc winding is determined as

where L

is the primary side inductance, I

pulse-by-pulse current limit level, A area of the core and B

is the saturation flux density in

sat

is the cross-sectional

is the FPS

over

tesla. If the pulse-by-pulse current limit lev el of FPS is larger than

the peak drain current of the power supply design, it may result in excessive transformer size since I

is used in

over

determining the minimum primary side turns as shown in equation (6). Therefore, it is required to choose a FPS with proper current limit specifications or to adjust the peak drain current close to I in Figure 5. It is reasonable to design I

considering the transient response and tolerance of I

over

by increasing the ripple factor as shown

over

peak

to be 70-80% of

over

Pulse-by-pulse current limit of FPS (I

70-80% of I

∆

Increasing ripple

factor (K

)

over

peak

Decreasing pirmary

side Inductance (L

)

over

∆

EDC

)

Vcc*V

--------------------------- -=N

where V FPS device, and V

* is the nominal value of the supply voltage of the

is the forward voltage drop of Da as

defined in Figure 6. Since V increases, it is proper to set V

o1VF1

⋅

turns() 9()

increases as the output load

* as Vcc start voltage (refer to

the data sheet) to avoid trigge ring the ov er voltage pro tectio n during normal operation.

S(n)

F(n)

R(n)

+ V

- V

O(n)

Figure 5. Adjustment peak drain current

Figure 6. Simplified diagram of the transformer

AN4140 APPLICATION NOTE

other windings can act as Faraday shields. When the primary Once the number of turns on the primary side have been determined, the gap length of the core is obtained through approximation as

=mm() 10()

G40



πA

-------------------- -



1000L



–

-----A

side winding has more than t wo layers, the innermost layer

winding should start from th e drain pin of FPS as show n in

Figure 7. This allows the winding driven by the highest

voltage to be shielded by other windings, thereby

maximizing the shielding effect.

where A

is the AL-value with no gap in nH/turns2, Ae is the

cross sectional area of the core as shown in Figure 2, L specified in equation (1) and N

is the number of turns for

the primary side of the transformer

(4) Determine the wire diameter for each winding

The wire diameter is determined based on the rms current through the wire. The current density is typically 5A/mm when the wire is long (>1m). When the wire is short with a small number of turns, a current density of 6-10 A/mm also acceptable. A void using wire with a diameter larger than 1 mm to avoid severe eddy current losses as well as to make winding easier. For high current output, it is better to use parallel windings with multiple strands of thinner wire to minimize skin effect.

3. Transformer Construction Method.

(1) Winding Sequence

(b) Vcc winding

In general, the voltage of each winding is influenced by the

voltage of the adjacent winding. The optimum placement of

the Vcc winding is determined by the over voltage pro tection

(OVP) sensitivity, the Vcc operating range and control

scheme.

-Over voltage protection (OVP) sensitivity : When the

output voltage goes above its normal operation value due to

some abnormal situation, Vcc voltage also increases. FPS

uses Vcc voltage to indirectly monitor the over voltage

situation in the secondary side. However, a RCD snubber

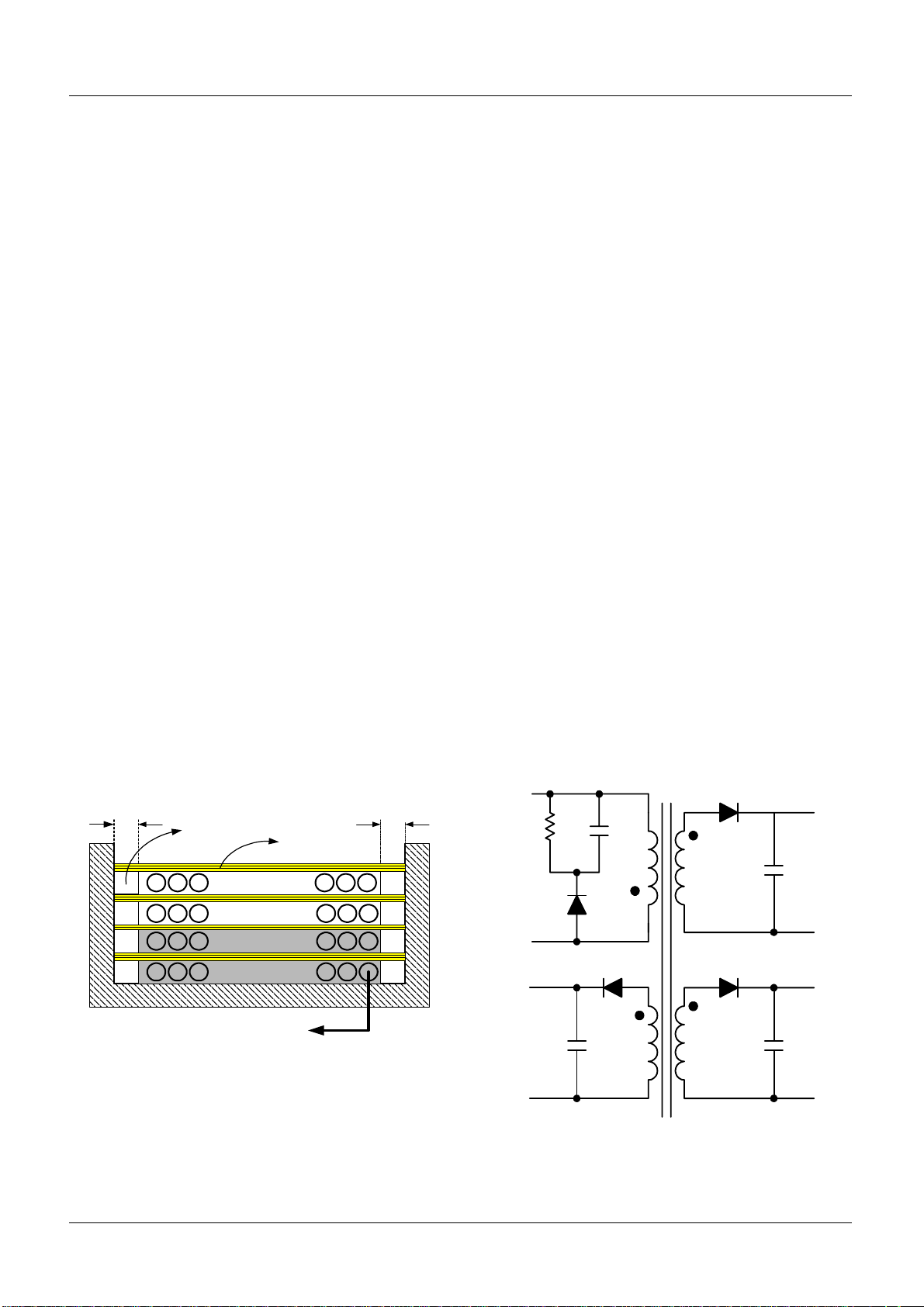
network acts as an another output as shown in Figure 8 and

Vcc voltage is also influenced by the snubber capacitor

voltage. Because the snubber voltage increases as the drain

current increases, OVP of FPS can be triggered not only by

the output over voltage condition, but also by the over load

condition.

The sensitivity of over voltage protection is closely related to

the physical distance between windings. If the Vcc winding

is close to the secondary side output winding, Vcc voltage

will change sensitively to the variation of th e output voltage.

Meanwhile, if the Vcc winding is placed close to the primar y

side winding, Vcc voltage will vary sensitively as the

snubber capacitor voltage changes.

(a) Primary winding

3mm 3mm

Barrier tape

Insulation tape

...... Na ...

.......... Ns .........

....... Np/2 .....

........ Np/2 ..... .

Bobbin

To FPS Drain pin

Figure 7. Primary side winding

It is typical to place all the primary winding or a portion of the primary winding innermost on the bobbin. This minimizes the length of wire, reducing the conduction loss in the wire. The EMI noise radiation can be reduced, since the

Figure 8. Primary side winding

APPLICATION NOTE AN4140

- Vcc operating range : As menti one d ab ove, Vcc voltage is influenced by the snubber capacitor voltage. Since the snubber capacitor voltage changes according to drain current, Vcc voltage can go above its operating range

Secondary winding

(4 turns)

triggering OVP in normal operation. In that case, Vcc winding should be placed closest to the reference output winding that is regulated by feedback control and far from the primary side winding as shown in Figure 9.

3mm 3mm

...... Na (Vcc winding) .....

..... Ns1 (Reference output) .....

........ Ns2 .......

Figure 10. Multiple parallel strands winding

.......... Np .........

.............. Np ..............

Secondary winding (3 strands, 4 turns)

.............. Np ..............

Figure 9. Winding sequence to reduce Vcc variation

- Control scheme : In the case of primary side regulation, the output voltages should follow the Vcc voltage tightly for a good output regulation. Therefore, Vcc winding should be placed close to the secondary windings to maximize the coupling of the Vcc winding with the secondary windings. Meanwhile, Vcc winding should be placed far from primary winding to minimize coupling to the primary. In the case of secondary side regulation, the Vcc winding can be placed between the primary and secondary or on the outermost position.

When it comes to a transform er with multiple outputs, the highest output power winding should be placed clos est to the primary side winding, to reduce leakage inductance and to maximize energy transfer efficiency. If a secondary side winding has relatively few turns, the winding should be spaced to traverse the entire width of the winding area for improved cou pling. Using multipl e parallel strands of wi re will also help to increase the fill factor and couplin g for the secondary wi ndings with few t urns as shown in Figu re 10. To maximize the load regulation, the winding of the output with tight regulation requirement should be placed closest to the winding of the reference output that is regulated by the feedback control.

(2) Winding method

-Stacked winding on other winding: A common technique

for winding multiple outputs with the same polarity sharing a

common ground is to stack the secondary windings instead

of winding each output winding separately, as shown in

Figure 11. This approach will improve the load regulation of

the stacked outputs and reduce the total number of secondary

turns. The windings for the lowest voltage output provide the

return and part of the winding turns for the next higher

voltage output. The turns of both the lowest output and the

next higher output provide turns for succeeding outputs. The

wire for each output must be sized to accommodate its

output current plus the sum of the output currents of all the

output stacked on top of it.

-Stacked winding on other output: If a transformer has a

very high voltage and lo w curr ent ou tput, the winding can be

stacked on the lower voltage output as shown in Figure 12.

This approach provides better regulation and reduced diode

voltage stress for the stacked output. The wire and rectifier

diode for each output must be sized to accommodate its

output current plus the sum of the output currents of all the

output stacked on top of it.

AN4140 APPLICATION NOTE

the leakage inductance is a sandwich winding as shown in

Figure 13. Se co nda ry w in din gs w i th onl y a fe w t ur ns shou ld

be spaced across the width of the bobbin window instead of

being bunched together, in order to maximize coupling to the

primary. Using multiple parallel strands of wire is an

additional technique of increasing the fill factor and coupling

of a winding with few turns as shown in Figure 10.

3mm 3mm

.............. Na ..............

.............. Np/2 ..............

.............. Ns1 ..............

Figure 11. Stacked winding on other winding

(4)

Transformer shielding

.............. Ns2 ..............

.............. Np/2 ..............

Figure 13. Sandwich winding

A major source of common mode EMI in Switched Mode

Power Supply (SMPS) is the parasi tic capacitances coupled

to the switching devices. The MOSFET drain voltage drives

capacitive current through various parasitic capacitances.

Some portion of these capacitive currents flow into the

neutral line that is connected to the earth ground and

observed as common mode noise. By using an electrostatic

separation shield between the windings (at primary winding

side, or at secondary winding side, or both), the common

mode signal is effectively "shorted" to the ground and the

capacitive current is reduced. When properly designed, such

shielding can dramatically reduce the conducted and radiated

emissions and susceptibility. By using this technique, the

Figure 12. Stacked winding on other output

size of EMI filter can be reduced. The shield can be easily

implemented using copper foil or tightly wound wire. The

shield should be virtually grounded to a quiescent point s uch

as primary side DC link, primary ground or secondary

ground.

(3)

Minimization of Leakage Inductance

The winding order in a transformer has a large effect on the leakage inductance. In a multiple output transformer, the secondary with the highest output power should be placed closest to the primary for the best coupling and lowest leakage. The most common and effective way to minimize

Figure 14 shows a shielding example, which allows the

removal of the Y-capacitor that is commonly used to reduce

common mode EMI. As can be seen, shields are used not

only on the bottom but also on the top of the primary

winding in order to cancel the coupling of parasitic capaci-

tances. Figure 15 also shows the detailed shielding

construction.

AN4140 APPLICATION NOTE

DC link

TOP

Insulation Tape Copper Foil

Shielding A

Drain

BOTTOM

Shielding B

#1 #2 #3 #4 #5

Winding Direction

3mm 3mm

.............. Na ..............

.............. Ns ............. .

Shield B

Insulation Tape

.............. Np ..............

#1 #2 #3 #4 #5

Primary Winding

Winding Directio

Copper Foil

Shield A

Figure 14. Shielding example to remove Y-capacitor

(5) Practical examples of transformer construction

As described in the above sections, there many factors that should be considered in determining the winding sequence and winding method. In this section some practical examples of transformer construction are presented to give a comprehensive understanding of practical transformer construction.

#1 #2 #3 #4 #5

Figure 15. Shielding method to remove Y-Capacitor

a) LCD monitor SMPS example

Figure 16 shows a simplified transformer schematic for typical LCD monitor SMPS. The 5V output is for the Microprocessor and 13V output is for the inverter input of LCD back light. While 5V output is regulated with the feedback control, 13V output is determined by the transformer tu rns ratio and a stacked winding is usually used to maximize the regulation.

APPLICATION NOTE AN4140

Transformer construction Example A (Figure 17) : In this example, the leakage inductance is minimized by employing a sandwich winding. The Vcc winding is placed outside to

3mm 3mm

provide shielding effect. Since the Vcc winding is placed on the top half of primary winding, the coupl ing between the Vcc winding and 5V output winding is poor, which may require a small dummy load on the 5V output to prevent UVLO (Under Voltage Lock Out) in the no load condition.

Transformer construction Example B (Figure 18)

: In this

example, the leakage inductance is larger than example A, since a sandwich winding is not used. However, the Vcc winding is tightly coupled with the 5V output winding and Vcc remains its normal operation range in the no load condition. Even though this approach can prevent U VLO in no load conditions without dummy load, the power

Ns1

Ns2

conversion efficiency might be relatively poor compared to example A due to the large leakage inductance.

Figure 18. LCD monitor SMPS transformer construction

example (B)

(13V/2.5A)

(5V/2A)

Figure 16. LCD monitor SMPS transformer example

3mm 3mm

Np/2

Ns1

(b) CRT monitor SMPS ex ample - PSR ( Primar y side

regulation)

Figure 19 shows a simplified transformer schematic for a

typical CRT monitor SMPS employing PSR (Primary side

regulation). 80V and 50V outp ut s ar e the mai n o utpu t having

high output power. Meanwhile, 5V and 6.5V outputs are

auxiliary outp ut h avi ng smal l ou tput pow er. The 80 V ou tput

winding is stacked on the 50V output to reduce the voltage

stress of the rectifier diode (D

7805

78057805

(14V)

(80V)

(50V)

Main output

with large

power

Ns2

Np/2

Figure 17. LCD monitor SMPS transformer construction example (A)

(6.5V)

Aux output

with small

power

Figure 19. CRT monitor SMPS transformer example-PSR

APPLICATION NOTE AN4140

Figure 20 shows the detailed transformer construction. In order to minimize the leakage inductance, sandwich winding is employed and the main output windings are placed closest to the primary winding. The Vcc winding is placed closest to the main output windings to provide tig ht regulations of the main output. The auxiliary output windings are placed outside of the primary winding to provide a shielding effect.

3mm 3mm

Ns4

Ns3

Np/2

Ns1

Np/2

Main output

with large

power

(80V)

Reference

7805

78057805

output

(50V)

(6.5V )

(14V)

Aux output

with small

power

Figure 20. CRT monitor SMPS transformer construction example (PSR)

Figure 21 shows a simplified transformer schematic for typical CRT monitor SMPS employing SSR (Secondary side regulation). 80V and 50V outputs are the main output having high output power. Meanwhile, 5V and 6.5V outputs are auxiliary output having small output power. The 80V output winding is stacked o n 50V ou tput to reduce t he volt age stres s of the rectifier diode (D

). Figure 22 shows the detailed transformer construction. In order to minimize the leakage inductance, a sandwich winding is employed and the main output windings are placed closest to the primary winding. The Vcc winding is placed outermost to provide a shielding effect. The auxiliary output windings are placed between windings of the main output winding to obtain bett er regulation.

Figure 21. CRT monitor SMPS transformer example-SSR

3mm 3mm

Np/2

Ns2

Ns4

Ns3

Ns1

Np/2

Figure 22. CRT monitor SMPS transformer construction example (SSR)

AN4140 APPLICATION NOTE

References

Colonel Wm. T. McLyman, Transformer and Inductor design Handbook, 2nd ed. Marcel Dekker, 1988.

Anatoly Tsaliovich, Electromagnetic shielding handbook for wired and wireless EMC application, 1998

Bruce C. Gabrielson and Mark J. Reimold, "Suppression of Powerline noise with isolation transformers", EMC expo87 San Diego, 1987.

D.Cochrane, D.Y.Chen, D. Boroyevich, "Passive cancellation of common mode noise in power electronics circuits," PESC 2001, pp.1025-1029

Otakar A. Horna, "HF Transformer with triaxial cable shielding against capacitive current", IEEE Transactions on parts, hybrids, and packaging, vol.php-7, N0.3 , Sep. 1971.

AN4140 APPLICATION NOTE

by Hang-Seok Choi / Ph. D Power Supply Group / Fairchild Semiconductor

Phone : +82-32-680-1383 Facsimile : +82-32-680-1317

E-mail : hschoi@fairchildsemi.co.kr

DISCLAIMER

FAIRCHILD SEMICONDUCTOR RESERVES THE RIGHT TO MAKE CHANGES WITHOUT FURTHER NOTICE TO ANY PRODUCTS HEREIN TO IMPROVE RELIABILITY, FUNCTION OR DESIGN. FAIRCHILD DOES NOT ASSUME ANY LIABILITY ARISING OUT OF THE APPLICATION OR USE OF ANY PRODUCT OR CIRCUIT DESCRIBED HEREIN; NEITHER DOES IT CONVEY ANY LICENSE UNDER ITS PATENT RIGHTS, NOR THE RIGHTS OF OTHERS.

LIFE SUPPORT POLICY

FAIRCHILD’S PRODUCTS ARE NOT AUTHORIZED FOR USE AS CRITICAL COMPONENTS IN LIFE SUPPORT DEVICES OR SYSTEMS WITHOUT THE EXPRESS WRITTEN APPROVAL OF THE PRESIDENT OF FAIRCHILD SEMICONDUCTOR CORPROATION. As used herein:

1. Life support devices or systems are devices or systems

which, (a) are intended for surgical implant into the body, or (b) support or sustain lif e, or (c) whose failure to perfo rm when properly used in a ccordance with instructions for us e provided in the labeling, can be reasonably expected to result in significant injury to the user.

2. A critical component is any component of a life support device or system whose failure to perform can be reasonably expected to cause the failure of t he life support device or system, or to affect its safety or effectiveness.

www.fairchildsemi.com

 2003 Fairchild Semiconductor Corporation

3/24/04 0.0m 002

Datasheet FPS Datasheet (Fairchild)

Specifications and Main Features

Frequently Asked Questions

User Manual