3B Scientific Acoustics Kit User Manual [en, de, fr, it, es]

Download

Page 1

3B SCIENTIFIC

Lehrgerät Akustik U8440012

Bedienungsanleitung

05/09 ELWE/ALF

PHYSICS

1. Beschreibung

Der Gerätesatz Lehrgerät Akustik ermöglicht die Vermittlung eines weitgehend geschlossenen Überblicks über das Themengebiet Akustik. Mit dem Lehrgerät können zahlreiche Versuche durchgeführt werden.

Versuchsbeispiele:

1. Saitentöne

2. Der reine akustische Ton

3. Schwingende Luftsäulen

4. Die offene Luftsäule

5. Die Lippenpfeife

6. Schwingende Stäbe

7. Infraschall

8. Ultraschall

9. Die Schreibstimmgabel

10. Fortschreitende Wellen

11. Dopplereffekt

12. Chladni’sche Klangfiguren

13. Glockenschwingungen

14. Stehende Wellen

15. Obertöne

16. Messung der Wellenlänge

17. Der Resonanzboden

18. Der Resonanzkasten

19. Der Kugelresonator

20. Die Saiteninstrumente und ihre Gesetze

21. Die Tonleiter auf den Saiteninstrumenten

22. Messung der Saitenspannung

23. Abhängigkeit der Tonhöhe von der Saitenspannung

24. Blasinstrumente und ihre Gesetze

25. Die C-Dur-Tonleiter und ihre Intervalle

26. Wohlklang und Missklang

27. Der G-Dur-Dreiklang

28. Der vierstimmige G-Dur-Dreiklang

29. Die Dur-Tonleiter mit beliebigem Grundton

30. Einschaltung der halben Töne

Lieferung erfolgt im Kunststofftablett mit Schaumstoffeinsatz zur schonenden Aufbewahrung der Einzelteile.

Page 2

2. Lieferumfang

1 Tablett mit Schaumstoffeinsatz für Lehrgerät "Akustik" 2 Monochord 3 Steg zum Monochord 4 Metallophon 5 Chladni-Platte 6 Stimmgabel, 1700 Hz 7 Stimmgabel, 440 Hz 8 Schreibstimmgabel, 21 Hz 9 Federwaage 10 Halteklammer 11 Tischklemme 12 Helmholtz-Resonator Ø 70 mm Ø 52 mm Ø 40 mm Ø 34 mm

13 Glasrohr für offene Luftsäule 14 Kundt’sche Röhre 15 Glasrohr für geschlossene Luftsäule 16 Stiel zu Chladni-Platte/ Glockenschale 17 Galtonpfeife 18 Schreibstift mit Halter 19 Lykopodiumpulver 20 Kunststoffklotz zu Tischklemme 21 Gummikappe 22 Glockenschale 23 Zungenpfeife 24 Lippenpfeife 25 Stahlsaite 26 Perlonsaite 27 Wellenseil 28 Abstimmschieber

Page 3

3. Technische Daten

Abmessungen: ca. 530 x 375 x 155 mm3 Masse: ca. 4,5 kg

4. Versuchsbeispiele

1. Saitentöne

• Die mäßig gespannte Saite des Monochords mit

dem Finger kräftig anzupfen.

• Dann durch Rechtsdrehen des Wirbels die

Saitenspannung erhöhen und abermals anzupfen.

Man vernimmt zuerst einen tiefen, anschließend einen höheren Ton.

Erklärung: Schwingende Saiten erzeugen durch abwechselnde Verdichtung und Verdünnung der umgebenden Luft akustische Töne. Je höher die Saitenspannung, desto schneller die Schwingung und desto höher der Ton.

2. Der reine akustische Ton

• Die Stimmgabel (440 Hz) kräftig mit dem An-

schlaghammer des Metallophons anschlagen.

Man vernimmt einen reinen akustischen Ton ganz bestimmter, unveränderlicher Tonhöhe, der langsam ausklingt.

Erklärung: Die Stimmgabel besteht aus einem U-förmig gebogenen Stahlstück, das im Scheitelpunkt (Bogen) in einen Stiel übergeht. Da die Stimmgabel nur in einer Schwingungsform zu schwingen vermag (gegenläufige Bewegung der Zinken von innen nach außen und umgekehrt) erzeugt sie einen reinen Ton unveränderlicher Höhe. Wegen der unveränderlichen Tonhöhe verwendet man die Stimmgabel zum Stimmen der Musikinstrumente.

3. Schwingende Luftsäulen

• Das Glasrohr für geschlossene Luftsäule mittels

Tischklemme, Kunststoffklotz und Halteklammer am Arbeitstisch befestigen.

• Den Abstimmschieber in das Glasrohr einfüh-

ren.

• Die Stimmgabel (440 Hz) kräftig mit dem An-

schlaghammer des Metallophons anschlagen. Durch mehr oder weniger starkes Ausziehen des Abstimmschiebers die Länge der "geschlossenen Luftsäule" verändern.

Nur in einer Stellung des Abstimmschiebers kommt die Luftsäule in starke Mitschwingung (Resonanz), in allen anderen Stellungen bleibt sie stumm. Die Resonanz ist durch eine Überhöhung der Lautstärke wahrnehmbar.

Erklärung: Geschlossene Luftsäulen kommen in Mitschwingung, wenn ihre Länge einem Viertel der erregenden. Wellenlänge entspricht. Die Stimmgabel schwingt mit 440 Schwingungen in der Sekunde. Nach der Beziehung

ndigkeitngsgeschwiFortplanzu

34000

440

eWellenläng =

s/cm

⋅

s/Schwing

cm,

⋅=

277

Frequenz

beträgt die Wellenlänge des erzeugten Tons 77,2 cm. Eine Viertelwellenlänge ist also 19,3 cm.

Der Abstand des Kolbens von der Rohröffnung beträgt im Resonanzfall 19,3 cm.

4. Die offene Luftsäule

• Den Versuch mit dem Glasrohr für offene Luft-

säule durchführen.

Die offene Luftsäule von genau der doppelten Länge der geschlossenen kommt bei Vorhalten der Stimmgabel in Mitschwingung, was durch eine Überhöhung der Lautstärke wahrnehmbar ist.

Erklärung: Offene Luftsäulen kommen in Mitschwingung, wenn ihre Länge einer halben Wellenlänge oder einem Vielfachen einer halben Wellenlänge entspricht. An den Enden der offenen Luftsäule bilden sich Schwingungsbäuche, in der Mitte ein Schwingungsknoten.

5. Die Lippenpfeife

• Die Lippenpfeife anblasen und durch Auszie-

hen des Stempels die Pfeifenlänge verändern.

Man vernimmt je nach Pfeifenlänge einen mehr oder weniger tiefen Ton charakteristischer Klangfarbe.

Erklärung: Beim Einblasen eines gleichmäßigen Luftstromes in die Mündung der Pfeife kommt die in der Pfeifenröhre eingeschlossene Luft dadurch in Schwingung, dass sich an der Lippe (Schneide) in regelmäßiger Folge Luftwirbel ablösen. Der sich ergebende Ton hängt von der Länge der Luftsäule ab. Bei der geschlossenen Pfeife entspricht die Pfeifenlänge (gemessen von der Schneidenkante bis zum Boden) im Grundton einer Viertelwellenlänge. An der Schneide bildet ich ein Bauch und am Boden ein Knoten.

6. Schwingende Stäbe

• Einige Stäbe des Metallophons mit dem beige-

gebenen Anschlaghammer anschlagen.

Beim Anschlagen der Metallstäbe entstehen wohlklingende Töne ganz charakteristischer Klangfarbe. Je kürzer der Stab, desto höher der Ton.

Erklärung: Elastische Stäbe werden zu schwingungsfähigen Systemen, wenn sie an den Punkten ihrer Schwingungsknoten aufliegen (etwa 22% der Gesamtlänge von den Enden entfernt).

Page 4

7. Infraschall

• Die Schreibstimmgabel durch gleichzeitiges

Zusammendrücken beider Zinken und plötzliches Loslassen in Schwingung versetzen.

Die Stimmgabel führt langsame, mit dem Auge noch gut wahrnehmbare Schwingungen aus. Wird sie dicht ans Ohr gehalten, so vernimmt man einen sehr tiefen (gerade noch hörbaren) Ton.

Erklärung: Die Zinken der Stimmgabel schwingen gegenläufig hin und her und erzeugen in dar umgebenden Luft Verdichtungen und Verdünnungen. Treffen diese auf das Ohr, so wird das Trommelfell in Mitschwingung versetzt. Man hört einen Ton.

Die Stimmgabel schwingt mit etwa 20 Schwingungen in der Sekunde hin und her. Der tiefste, gerade noch hörbare Ton hat etwa 16 Schwingungen in der Sekunde. Schwingungen unter 16 Hertz sind nicht mehr hörbar. Man bezeichnet sie als Infraschall (lat. infra = unterhalb).

8. Ultraschall

• Die Galtonpfeife anblasen.

Man vernimmt keinen Ton mehr sondern nur ein zischendes Geräusch.

Ergebnis: Wegen ihrer geringen Länge erzeugt die Galtonpfeife sehr hohe Töne, die für den Menschen nicht mehr hörbar sind. Man bezeichnet sie als Ultraschall (lat. ultra = über).

9. Die Schreibstimmgabel

• An den Zinken der Schreibstimmgabel den

Schreibstift befestigen.

• Die Stimmgabel durch Zusammendrücken der

Zinken in Schwingung versetzen und mit dem Schreibstift gleichmäßig über ein Blatt Papier auf einer nicht zu weichen Unterlage fahren.

Der Schreibstift zeichnet auf dem Papier eine wellenförmige Linie gleichbleibender Wellenlänge, aber abnehmender Amplitude auf.

Erklärung: Schall entsteht durch periodische Schwingung fester, flüssiger oder luftförmiger Körper. Der geometrische Ort der schwingenden Teilchen des Körpers in Abhängigkeit von der Zeit liegt auf einer Wellenlinie (Sinuslinie). Bei einmaligem Anstoß führen schwingende Körper eine "gedämpfte" Schwingung aus (stetige Abnahme der Amplitude). Erfolgt die Energiezufuhr dauernd (Dauerton einer Autohupe, dauernd angeblasene Orgelpfeife), so erhalten wir eine ungedämpfte Schwingung gleichbleibender Amplitude (= Lautstärke).

10. Fortschreitende Wellen

• Die Schlaufe des Wellenseils durch einfaches

Verknoten an einer Türklinge befestigen.

• Das Seil mäßig straff spannen und mit der

Hand eine ruckartige, seitliche Bewegung ausführen.

Vom Bewegungszentrum (Hand) ausgehend, löst sich eine Welle ab, die mit bestimmter Fortschreitungsgeschwindigkeit am Seil entlang läuft, am festen Ende reflektiert wird und wieder zum Ausgangspunkt zurückkehrt.

Erklärung: Jeder feste, flüssige und luftförmige Körper führt bei plötzlicher Erschütterung Schwingungen aus, die sich mit einer bestimmten Fortpflanzungsgeschwindigkeit im schwingenden Medium ausbreiten.

11. Dopplereffekt

• Die Leichtmetall-Stimmgabel (1700 Hz) kräftig

mit dem Anschlaghammer des Metallophons anschlagen, sie kurze Zeit still halten und sie dann in der Luft rasch hin und her schwenken.

In ruhendem Zustand erzeugt die Stimmgabel einen kräftigen Ton gleichbleibender Höhe. In bewegtem Zustand ändert sich die Tonhöhe dauernd. Erfolgt die Bewegung auf das Ohr zu, so erhöht sich der Ton, erfolgt sie vom Ohr weg, so erniedrigt er sich.

Erklärung: Durch den sich verringernden Abstand der Schallquelle vom Ohr verkürzt sich der zeitliche Abstand zweier Verdichtungen, da die 2. Verdichtung einen kürzeren Weg zum Ohr hat als die erste. Das Ohr nimmt eine höhere Frequenz wahr. Der Ton wird höher. Bei der Entfernung der Schallquelle vom Ohr werden die zeitlichen Abstände zwischen den Verdichtungen und Verdünnungen verlängert. Der Ton wird tiefer.

12. Chladni’sche Klangfiguren

• Die Chladni-Platte mittels Tischklemme und

Kunststoffklotz am Arbeitstisch befestigen. Die Platte mit Vogelsand oder ähnlichem bestreuen, so dass dieser in dünner Schicht ein Drittel der Platte bedeckt.

• Die Platte nun mit einem gut kollophonierten

Geigenbogen genau in der Mitte zwischen zwei Ecken anstreichen unter gleichzeitiger, leichter Berührung einer Ecke mit dem Finger der anderen Hand.

• Platte mehrmals kräftig anstreichen, so dass sie

in lebhafte, gut hörbare Schwingung gerät.

Beim Anstreichen der Platte vernimmt man einen ganz bestimmten akustischen Ton. Die Sandkörner geraten an manchen Stellen in lebhafte Mitschwingung, tanzen auf der Plattenoberfläche auf und nieder und lagern sich in eigenartigen Klangfiguren auf der Oberfläche ab.

Erklärung: Auf der Platte bilden sich "stehende Wellen" aus. Die Platte schwingt beim Anstreichen nicht einfach als Ganzes auf und nieder sondern

Page 5

⋅

gerät an bestimmten Stellen (den Bäuchen) in Schwingung, während sie an anderen Stellen (den Knoten) völlig ruht. Durch Berührung der Platte an einer Ecke wurde an dieser Stelle ein Knoten erzwungen.

13. Glockenschwingungen

• Die Glockenschale mit der Öffnung nach oben

mittels Tischklemme und Kunststoffklotz am Arbeitstisch befestigen.

• Den Rand der Glocke an verschiedenen Stellen

mit dem Anschlaghammer anschlagen (alternativ mit dem Geigenbogen anstreichen).

Die Tonhöhe hängt von der Anschlagstelle ab. Es ist ohne weiteres möglich Unterschiede von einem ganzen Ton zu erhalten. Wird die Glocke an bestimmten Stellen angeschlagen, so werden beide Töne angeregt und man erhält die bekannten „Schwebungen“ (periodisches An- und Abschwellen der Lautstärke in mehr oder weniger rascher Folge).

Erklärung: Glocken sind verformte schwingende Platten. Die Obertöne sind meist nicht harmonisch zum Grundton. Auch Glocken teilen sich durch Knotenlinien in einzelne schwingende Abteilungen.

14. Stehende Wellen

• Die Schlaufe des Wellenseils durch einfaches

Verknoten an einer Türklinge befestigen.

• Das Seil mäßig straff spannen und mit der

Hand langsame, kreisförmige Bewegungen durchführen.

• Dann das Seil straffer spannen und die Kreis-

bewegungen schneller werden lassen.

Bei langsamer Bewegung entstehen an den Enden des Seils jeweils Knoten und in der Mitte ein Bauch. Bei schnellerer Bewegung entstehen 3 Knoten und 2 Bäuche und bei noch schnellerer Bewegung 4 Knoten und 3 Bäuche.

Erklärung: Durch die Reflexion an der Tür bilden sich stehende Wellen aus. Wegen der Trägheit des Auges sieht. man die ursprüngliche und die reflektierte Welle scheinbar gleichzeitig.

In der Grundschwingung schwingt das Seil in seiner ganzen Länge in Form einer Halbwelle auf und nieder. In der Mitte ist ein Bauch, an beiden Enden sind Knoten. In der 1. Oberschwingung (Oktave) schwingt das Seil in Form einer ganzen Welle (2 Bäuche und 3 Knoten). In der 2. Oberschwingung haben wir 3 Bäuche und 4 Knoten usw.

15. Obertöne

• Die Lippenpfeife mit dem Mund zuerst

schwach und dann sehr stark anblasen.

Man vernimmt zuerst den Grundton und bei stärkerem Anblasen einen wesentlich höheren Ton.

Erklärung: Bei der gedackten Pfeife müssen sich stehende Wellen stets in der Weise ausbilden, dass sich am Boden ein Knoten und an der Schneide ein Bauch befindet. Dies ist der Fall, wenn die Länge der Pfeife genau 1/4 Wellenlänge entspricht. Es ist aber auch der Fall, wenn die Entfernung der Öffnung vom Boden 3/4, 5/4, 7/4 usw. der Wellenlängen beträgt.

Neben dem Grundton entstehen also auch sämtliche ungeradzahligen Obertöne der harmonischen Tonreihe in mehr oder weniger starkem Maße.

Nur dem mehr oder weniger starken Auftreten von Obertönen ist es zuzuschreiben (und zu verdanken), dass jedes Musikinstrument eine ganz charakteristische Klangfarbe hat.

16. Messung der Wellenlänge

• Das Ende der genau 45 cm langen Kundt’schen

Röhre mit der Gummikappe verschließen und

mit einem Teelöffel eine kleine Menge Lykopodiumpulver in die schräg gehaltene Röhre füllen, so dass sich eine nicht zu große Menge, gleichmäßig verteilt, als feines gelbes Band in der Röhre befindet.

• Glasrohr mittels Halteklammer, Tischklemme

und Plastikklotz am Arbeitstisch befestigen.

• Die Stimmgabel (1700 Hz) an einem Hammer-

stiel sehr kräftig anschlagen und einen Zinken breitseits dicht vor die Rohröffnung halten. Diese Schallanregung eventuell mehrmals wiederholen!

Das Lykopodiumpulver kommt an den Schwingungsbäuchen in lebhafte Mitschwingung, während es an den Knoten völlig in Ruhe bleibt. Die Pulverteilchen fallen auf den Grund des Rohres und bilden dort periodische Anhäufungen, die sich längs der Rohrachse 4 1/2 Mal wiederholen.

Erklärung: Die Leichtmetall-Stimmgabel hat eine Frequenz von 1700 Schwingungen in der Sekunde. Nach der einfachen Beziehung

keiteschwindiglgSchal

340

1700

eWellenläng =

s/m

⋅

⋅=

Frequenz

beträgt die zugehörige Wellenlänge 20 cm. In eine: Röhre von 45 cm Länge "passen" also 4½ Halbwellen oder 2 volle und eine Viertelwellenlänge, wie der Versuch es zeigte. An der Öffnung der Röhre ist immer ein Bauch, am Boden immer ein Knoten.

Page 6

17. Der Resonanzboden

• Die Stimmgabel a’ = 440 Hertz kräftig mit dem

Anschlaghammer des Metallophons anschlagen und mit ihrem Stiel auf die Tischplatte stellen.

Der in freier Luft kaum hörbare Ton der Stimmgabel wird durch das Aufstellen auf die Tischplatte so sehr verstärkt, dass er nunmehr im ganzen Raum deutlich vernehmbar ist.

Erklärung: Durch den auf- und abschwingenden Stiel der Stimmgabel wird die Tischplatte in Mitschwingung versetzt. Da die wirksame Tischfläche wesentlich größer ist als die der Stimmgabel, wird die Lautstärke des Tons erheblich verstärkt.

18. Der Resonanzkasten

• Die Stimmgabel a' = 440 Hertz kräftig anschla-

gen und mit ihrem Stiel auf den Resonanzkas-

ten des Monochords stellen. Es tritt eine bedeutende Verstärkung des Tons ein. Erklärung: Wie bei Versuch 17.

19. Der Kugelresonator

• Die Helmholtz-Resonatoren der Reihe nach mit

der kleinen Spitze ans Ohr halten. Man vernimmt einen Ton, der umso tiefer ist je

größer der Durchmesser des Resonators ist. Erklärung: Jeder gleichwie gestaltete Hohlraum

(Röhre, Hohlkugel) hat eine ganz bestimmte, nahezu obertonfreie Grundschwingung Diese Grundschwingung kann man erregen, wenn der Hohlraum an seiner Öffnung angeblasen oder auch nur mit dem Fingerknöchel gegen den Hohlraum geklopft wird. Die Eigenschwingung wird aber auch in erster Linie dann erregt, wenn im umgebenden Lärm Töne enthalten sind, die mit der Grundschwingung des Resonators übereinstimmen. So kann man mit dem Kugelresonator ein Klanggemisch auf seinen Gehalt an Teiltönen prüfen. Herrscht in einem Raum absolute Stille, so bleibt der Resonator stumm.

20. Die Saiteninstrumente und ihre Gesetze

• Den Quersteg hochkant unter die Saite des

Monochords schieben so dass die rechte Kante

genau mit der Zahl 20 der Maßskala zusam-

menfällt und die 40 cm lange Saite in zwei

gleich lange Abschnitte von je 20 cm Länge un-

terteilt wird.

• Die halbe Saitenlänge durch Anziehen des

Wirbels auf die Stimmgabel (440 Hz) a’ (Kam-

merton) abstimmen.

• Durch Anzupfen oder besser Anstreichen der

Saite die Tonhöhen bei 40 cm, 20 cm, 10 cm

und 5 cm Saitenlänge vergleichen.

Bei 20 cm Saitenlänge erhält man den Kammerton a’ = 440 Hertz, bei 40 cm Saitenlänge den um eine Oktave tieferen Ton a = 220 Hertz, bei 10 cm Saitenlänge den um eine Oktave höheren Ton a’’ = 880 Hertz und bei 5 cm Saitenlänge den um 2 Oktaven höheren Ton a’’’ = 1760 Hertz.

Erklärung: Bei der doppelten Saitenlänge erhält man einen um eine Oktave tieferen Ton, bei der halben Saitenlänger die 1. und bei 1/4 Saitenlänge die 2. Oktave. Die Frequenzen von Saiten verhalten sich umgekehrt wie ihre Längen.

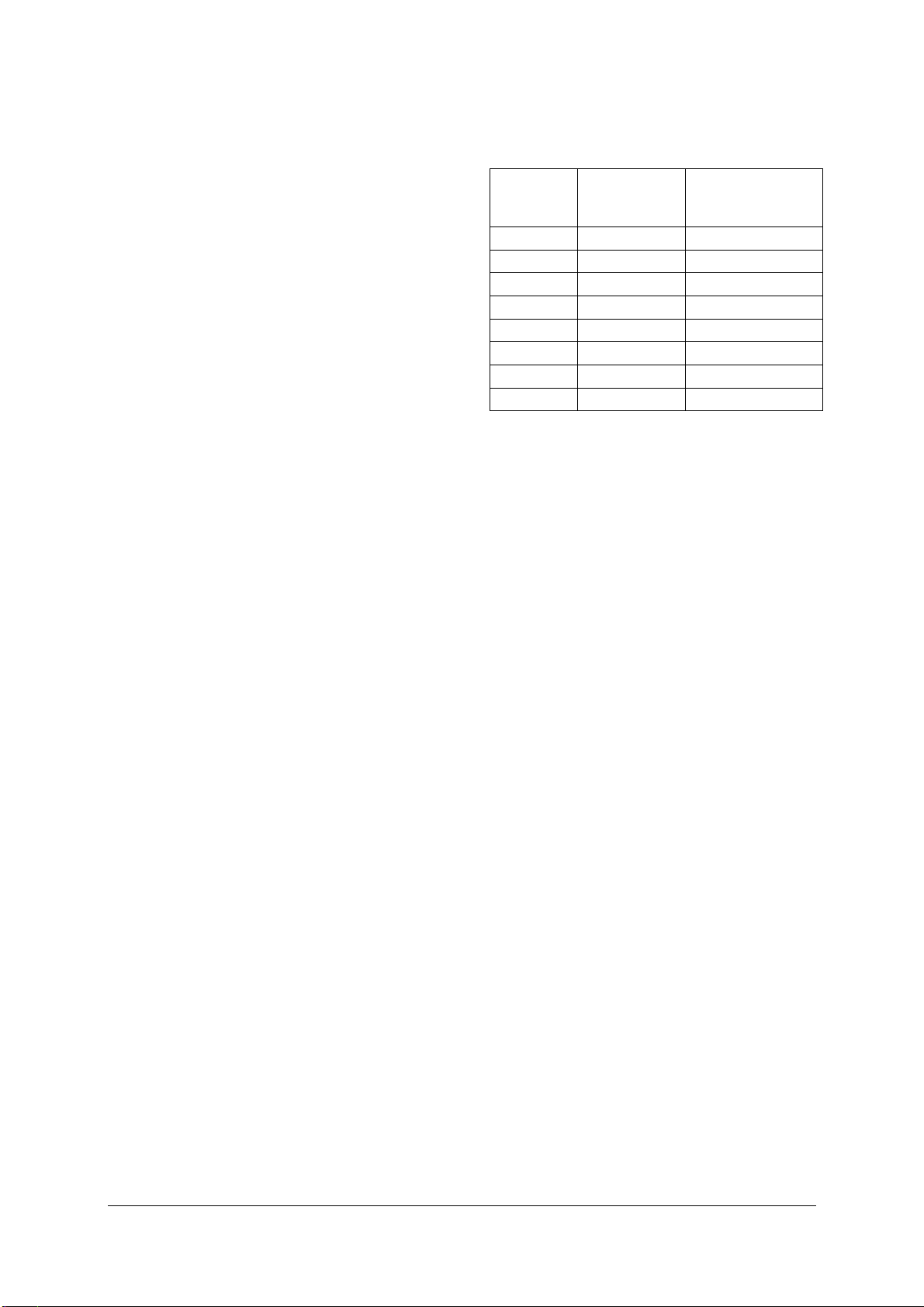
21. Die Tonleiter auf den Saiteninstrumenten

• Auf dem Monochord durch Verschieben des

Quersteges die dem menschlichen Ohr einge-

prägte

Tonleiter spielen und jeweils die Längen des schwingenden Saitenstückes und das Verhältnis des schwingenden Saitenstückes zur Gesamtlänge der Saite (40 cm) ermitteln.

Ton Saitenlänge Längenverhältnis

c 40 cm 1 d 35,55 cm 8/9 e 32 cm 4/5 f 30 cm 3/4 g 26,66 cm 2/3 a 24 cm 3/5 h 21,33 cm 8/15

c’ 20 cm 1/2

Erklärung: Die Saite muss halb so lang sein, wenn, unter sonst gleichen Bedingungen wie Saitenspannung, Saitendicke usw., die Oktave erreicht werden soll. Bei den übrigen Tönen der Tonleiter ergeben sich für das Verhältnis der schwingenden Saitenlängen zur ganzen Saitenlänge einfachste Verhältniszahlen. Je kleiner diese Zahlen sind, desto besser ist der Wohlklang. (Oktave 1:2, Quinte c/g 2:3 usw.)

22. Messung der Saitenspannung

• Die Federwaage auf das Monochord aufstecken

und das Ende der Perlonsaite in den Schlitz der Federwaage einhängen.

• Durch Anziehen des Wirbels die Saite

unter Verwendung der Stimmgabel a’ = 440 Hertz auf den Kammerto n absti mmen.

• Mit der Federwaage die Saitenspannung

bestimmen.

Die Saitenspannung beträgt bei der Perlonsaite 5,5 kg.

Page 7

23. Abhängigkeit der Tonhöhe von der Saitenspannung

Ein Ergebnis von Versuch 22 war, dass, um den Kammerton zu erhalten, die Perlensaite mit 5,5 kg gespannt werden muss. Wie hoch ist die Saitenspannung bei dem um eine Oktave tiefer liegenden Ton a (220 Hertz)?

• Den Wirbel lockern, bis der Ton a ertönt.

• Zur Kontrolle den Quersteg unter die Maßzahl

20 (halbe Saitenlänge) setzen und die halb e Saitenlänge wieder auf den Kammerton abstimmen. Die ganze Saite schwingt dann mit

der halben Frequenz. Die Saitenspannung geht auf 1,4 kg zurück. Erklärung: Die Frequenz einer Saite ist proportional

zur Quadratwurzel aus dem spannenden Gewicht. Wenn die Kraft, welche die Saite spannt, 4x, 9x, 16x so groß ist, erhöht sich die Frequenz um das 2-, 3und 4-fache. 1/4 von 5,5 ist (rund) 1,4, wie gemessen wurde.

24. Blasinstrumente und ihre Gesetze

• Die Lippenpfeife mit dem Mund anblasen und

durch mehr oder weniger starkes Ausziehen

des Bodens die wirksame Länge der Pfeife ver-

ändern. Bei kleiner Pfeifenlänge erhält man hohe, bei gro-

ßer tiefere Töne. Erklärung: Beim Einblasen eines schwachen Luft-

stromes bilden sich stehende Wellen aus, wobei die Pfeifenlänge einer Viertelwellenlänge entspricht. Beim Einblasen eines stärkeren Luftstromes entstehen Obertöne, deren Frequenz ein ungeradzahliges Vielfaches des Grundtones ist.

Bei der offenen Pfeife ist die Grundschwingung doppelt so groß wie bei der geschlossenen.

25. Die C-Dur-Tonleiter und ihre Intervalle

• Zur Bestimmung der Intervalle wird jeweils die

höhere Frequenz durch die nächst niedrigere

geteilt. Für das Intervall d/c =1188/1056 ist der gemeinsa-

me Teiler 132 man erhält also 9/8, 10/9, 16/15, 9/8, 10/9, 9/8 und 16/15.

Erklärung: Die Intervalle (Zwischenräume) der einzelnen Töne der Tonleiter sind nicht gleich groß. Man unterscheidet große ganze (9/8), kleine ganze (10/9) und halbe Tonschritte (16/15).

26. Wohlklang und Missklang

• Auf der Zungenpfeife die verschiedensten Zu-

sammenklänge anblasen. Ausgesprochene Wohlklänge (Konsonanzen) erhält

man bei der Oktave, der Quinte, der Quarte, der großen und kleinen Terz. Missklänge (Dissonanzen) sind die Sekunde und die Septime, sowie der Zu-

sammenklang unmittelbar nebeneinander liegender Töne.

27. Der G-Dur-Dreiklang

• Auf der Zungenpfeife die Töne g e d gleichzei-

tig anblasen.

Man vernimmt einen besonders wohlklingenden Zusammenklang, den man als den G-Dur-Dreiklang bezeichnet.

Erklärung: Sollen mehrere Töne einen wohlklingenden Zusammenklang, eine Konsonanz, bilden, so müssen sie es paarweise tun. Der G-DurDreiklang setzt sich aus der großen Terz und der kleinen Terz zusammen. Die Frequenzen der Töne g h d stehen untereinander in einem besonders einfachen Verhältnis und zwar 4:5:6.

Um dieses Zahlenverhältnis zu erhalten, müssen die auf der Zungenpfeife angegebenen Grundfrequenzen jeweils durch 6 geteilt werden. (Um die physikalisch korrekten Frequenzen zu erhalten, müssen die aufgedruckten Grundfrequenzen mit 33 multipliziert werden).

Zwischen Zungenpfeife und Metallophon kann zudem fertigungsbedingt eine Abweichung der Stimmung hörbar sein.

28. Der vierstimmige G-Dur-Dreiklang

• Den G-Dur-Dreiklang durch die Oktave g' er-

gänzen. Also gleichzeitig g h d’ g’ spielen.

Man erhält den besonders vollen und wohlklingenden "vierstimmigen G-Dur-Dreiklang".

Erklärung: Im vierstimmigen Dreiklang sind folgende Konsonanzen enthalten:

Die Oktave 1:2 Die Quinte 2:3 Die große Terz 4:5 Die kleine Terz 5:6

29. Die Dur-Tonleiter mit beliebigem Grundton

• Auf dem Metallophon zuerst die C-Dur-

Tonleiter, beginnend mit c, anschließend die G-Dur-Tonleiter, beginnend mit g, spielen.

Die C-Dur-Tonleiter von c' bis c" erklingt klangrein. Bei der mit g' beginnenden G-Dur-Tonleiter tritt bei f" ein schwerer Fehler auf. Der Ton ist um einen halben Tonschritt zu tief.

Erklärung: Nach Versuch 25 müssen bei jeder Tonleiter folgende Intervalle auftreten:

9/8, 10/9 16/15, 9/8, 10/9, 9/8, 16/15. Bei der Tonfolge g'...g" stehen auf der Grundplatte

des Metallophons aber folgende Intervalle: 10/9, 9/8, 16/15

, 9/8, 10/9, 16/15, 9/8

Die unterstrichenen Intervalle sind richtig, die übrigen mehr oder weniger falsch.

Page 8

Die Intervalle 9/8 und 10/9 liegen einander aber so nahe, dass sie nur schwer voneinander zu unterscheiden sind, daher ist der Fehler von g' bis h' belanglos. Schwerwiegend dagegen ist der Fehler zwischen e" und f". Hier beträgt das Intervall 16/15, während es in Wirklichkeit 9/8 betragen sollte. Man hört daher f" um einen halben Ton zu tief.

30. Einschaltung der Halbtonschritte

• Auf der Zungenpfeife die Tonleiter von g' bis g"

spielen, nachdem vorher sicher gestellt wurde,

dass der Ton a' der Zungenpfeife tatsächlich

auf den Kammerton abgestimmt ist. Dazu

Stimmgabel anschlagen und vergleichen. Auf der Zungenpfeife ertönt die G-Dur-Tonleiter

völlig klangrein. Erklärung: Anstelle des Tones f' ist ein neuer Ton,

das fis' eingeschaltet, der so berechnet ist, dass das Intervall zwischen e’ und fis’ 9/8 und das zwischen fis’’ und g’’ 16/15 beträgt. Dies geschieht dadurch, dass man die Frequenz des f durch Multiplikation mit 25/24 erhöht.

Die durch Erhöhung der Töne hervorgerufenen neuen Töne heißen: cis, dis, eis, fis, gis, ais, his.

Die Erhöhung wird in der Notenschrift durch ein vorgesetztes Kreuz bezeichnet.

Die um einen halben Ton niedrigeren Töne erhält man durch Multiplikation des höheren Tones mit 24/25. Diese Töne werden in der Notenschrift durch ein vorgesetztes b gekennzeichnet. Sie heißen: ces, des, es, fes, ges, as, b.

Mit nur geringen Fehlern werden auf dem Klavier die Töne cis und des usw. einander gleichgesetzt.

Elwe Didactic GmbH • Steinfelsstr. 6 • 08248 Klingenthal • Deutschland • www.elwedidactic.com

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Deutschland • www.3bscientific.com

Technische Änderungen vorbehalten

Page 9

3B SCIENTIFIC

Instruction sheet

05/09 ELWE/ALF

PHYSICS

Acoustics Kit U8440012

1. Description

This set of apparatus makes it possible to impart an extensive and well-rounded overview on the topic of acoustics. The set can be used for conducting numerous experiments.

Sample experiments:

1. String tones

2. Pure acoustic tones

3. Vibrating air columns

4. Open air column

5. Whistle

6. Vibrating rods

7. Infrasound

8. Ultrasound

9. Tuning fork with plotter pen

10. Progressive waves

11. Doppler effect

12. Chladni figures

13. Chimes

14. Standing waves

15. Overtones

16. Measurement of wavelength

17. Soundboard

18. Resonator box

19. Spherical cavity resonator

20. Stringed instruments and the laws they obey

21. Scales on stringed instruments

22. Measurement of string tension

23. Relation between pitch and string tension

24. Wind instruments and the laws they obey

25. C major scale and its intervals

26. Harmony and dissonance

27. G major triad

28. Four-part G major chord

29. Major scales in an arbitrary key

30. Introduction of semitones

The set is supplied in a plastic tray with a foam insert that facilitates safe storage of the individual components.

Page 10

2. Contents

1 Trays with foam inserts for acoustics kit 2 Monochord 3 Bridge for monochord 4 Metallophone 5 Chladni plate 6 Tuning fork, 1700 Hz 7 Tuning fork, 440 Hz 8 Tuning fork with plotter pen, 21 Hz 9 Spring balance 10 Retaining clip 11 Table clamp 12 Helmholtz resonators 70 mm dia. 52 mm dia.v 40 mm dia. 34 mm dia. 13 Glass tube for open air column 14 Kundt’s tube 15 Glass tube for closed air column 16 Rod for Chladni plate/bell dome

17 Galton whistle 18 Plotter pen with holder 19 Lycopodium powder 20 Plastic block for clamp 21 Rubber top 22 Bell dome 23 Reed pipe 24 Whistle 25 Steel string 26 Nylon string 27 Resonance rope 28 Plunger

Page 11

3. Technical data

Dimensions: 530 x 375 x 155 mm3 approx. Weight: 4.5 kg approx.

4. Sample experiments

1. String tones

• Pluck the monochord string hard when it is

moderately taut.

• Subsequently increase the tension on the string

by turning the peg to the right. Pluck the string

again. At first, a low tone is heard. As the string is tight-

ened the tone gets higher. Reasons: vibrating strings generate acoustic tones

by inducing alternating compression and rarefaction of the surrounding air. The greater the tension in the string, the faster the vibrations are and the higher the tone.

2. Pure acoustic tones

• Hit the 440 Hz tuning fork hard with the metal-

lophone beater. A pure acoustic tone of a very specific, unchanging

pitch can be heard. This tone dies away very slowly. Reasons: a tuning fork consists of a U-shaped steel

piece which merges into the stem at its vertex. As the tuning fork only vibrates in one oscillation mode (with both prongs either both moving apart or both moving towards one another), it produces a pure tone of an unchanging pitch. Owing to its property of producing a constant pitch, tuning forks are used for tuning musical instruments.

3. Vibrating air columns

• Attach the glass tube for demonstrating a

closed air column by means of the table clamp,

plastic block and retaining clip.

• Insert the tuning plunger into the glass tube.

• Hit the 440 Hz tuning fork hard with the metal-

lophone beater. By pulling out the plunger to a

greater or lesser degree it is possible to alter

the length of the closed air column. There is only one plunger position at which the air

column resonates strongly. At any other position there is no sound. Resonance can be detected by the increase in sound volume.

Reasons: a closed air column starts resonating when its length corresponds to one quarter of the excitation wavelength. The tuning fork vibrates with a frequency of 440 vibrations per second. Applying the following equation:

Wavekength =

⋅

scm

/34000

pagationSpeedofpro

Frequency

sfreqExciting

/440

⋅=

2.77

the wavelength of the tone produced is 77.2 cm. One quarter of this wavelength is therefore

19.3 cm. The distance between the plunger and the opening

at the end of the tube is 19.3 cm when resonance occurs.

4. Open air column

• Conduct the same experiment with an open air

column (14).

The open air column, which is exactly double the length of the closed air column, starts resonating when the tuning fork is brought into its vicinity, as can be heard by means of the increased volume.

Reasons: an open air column starts resonating when its length is half that of the wavelength or multiples of that length. Antinodes are formed at the ends of the open air column and a node at the middle.

5. Whistle

• Blow the whistle and change its length by

gradually drawing out the plunger.

Depending on the length of the whistle, its note gets higher or lower but the character or timbre of the note remains the same.

Reasons: blowing a uniform air stream into the opening of a whistle causes the air trapped in the pipe to vibrate and eddies then occur at regular intervals air the air passes over the blade. The resulting tone depends on the length of the air column. In the case of a closed air column, the length of the whistle (measured from the edge of the blade to the base of the whistle) corresponds to a quarter wavelength of the base tone. A node is formed at the blade of the whistle and an antinode is formed at the end of the pipe

6. Vibrating bars

• Use the striking hammer supplied to strike

several bars of the metallophone. When the metal bars are struck, they produce a distinct, melodious note, each of which has a similar timbre. The shorter the length of the bar, the higher the tone.

Reasons: elastic rods form systems capable of oscillating if they are resting upon a point where a node is formed (about 22% of the total length between the two ends).

Page 12

7. Infrasound

• Without the plotter pen attached, make the

tuning fork (21 Hz) vibrate by pressing its

prongs together and suddenly releasing them. The tuning fork produces slow vibrations that can

be perceived by the naked eye. When held close to the ear, a very deep (barely audible) tone can be heard.

Reasons: the prongs of the tuning fork vibrate in opposite directions and give rise to compressions and rarefactions in the surrounding air. When this reaches the ear, it makes the eardrum vibrate. A tone is thus perceived.

The tuning fork vibrates at approximately 20 vibrations per second. The lowest note that can be perceived by human hearing has a frequency of approximately 16 vibrations per second. Vibrations below 16 Hz are not audible to the human ear. The sound produced by these vibrations is called infrasound. (Latin: infra = below).

8. Ultrasound

• Blow the Galton whistle.

No sound can be heard, simply a hiss. Reasons: owing to its short length, the Galton whis-

tle produces very high tones which are not audible to the human ear. This phenomenon is called ultrasound. (Latin: ultra = above).

9. Tuning fork with plotter pen

• Attach the pen (8) to the prongs of the tuning

fork (21 Hz).

• Make the tuning fork vibrate by pressing the

prongs together and move a sheet of paper as

uniformly as possible under the pen so that the

motion is plotted onto it. Make sure that the

surface on which the paper rests is not too soft. The pen traces a wavy line of a constant wave-

length but decreasing amplitude on the paper. Reasons: sound is produced by harmonic oscilla-

tions of solids, liquids or gases. The locus of the oscillating particles of the body in relation to the time traces a sine curve. When struck once, vibrating bodies exhibit a “damped” oscillation (continuous decrease in amplitude). If the supply of energy is uninterrupted (constant sound of a car horn, constant blowing of an organ pipe), the result is an undamped oscillation of constant amplitude (loudness or volume).

10. Progressive waves

• Make a simple knot in the resonance rope and

attach it by the loop to the handle of a door.

• Make the wire moderately taut and jerk it

suddenly to the side.

From the centre of motion (the hand), a wave is produced which runs along the wire with an increasing velocity, gets reflected at the fixed end and returns to the point of origin.

Reasons: every solid, liquid and gas produces vibrations when disturbed suddenly. These vibrations spread through a medium with a definite propagation velocity.

11. Doppler effect

• Strike the light-metal tuning fork (1700 Hz)

hard with the metallophone beater. Hold it still for a short while and then rapidly move it to and fro through the air.

In a state of rest, the tuning fork produces a clear tone of uniform pitch. In a state of motion, the pitch constantly changes. If the tuning fork is moved towards the ear, the pitch rises, and if it is moved away from the ear, the pitch decreases.

Reasons: when the distance between the source of sound and the ear is decreasing, the time interval between two compressions also decreases as a second compression has to travel a shorter distance to reach the ear compared to the first. The ear registers a higher frequency. The tone thus gets higher. When the source of sound is moved away from the ear, the intervals between compressions and rarefactions get longer. The tone thus becomes deeper.

12. Chladni figures

• Use the table clamp and plastic block to attach

the Chladni plate to the workbench. Scatter some bird sand or a similar material onto the plate. Allow it to spread in a thin layer so as to cover a third of the plate.

• With one hand, bow the plate exactly half way

between two corners with a good violin bow, simultaneously touching one other corner lightly with the finger of your other hand.

• Bow several strokes across the plate, preferably

quite forcefully so that the vibrations of the plate are vigorous and well audible.

When the plate is being bowed, a very distinct acoustic tone can be heard. At certain points, the grains of sand experience lively resonance and begin to bounce up and down on the surface of the plate, accumulating in unusual figures on the surface.

Reasons: “standing waves” are formed on the plate. When bowed, the plate does not vibrate uniformly across its surface. At certain points (antinodes), the plate begins to vibrate, whereas it is in a state of complete rest at other points (nodes). By touching the plate at one corner, the point is forced into being a node.

Page 13

13. Chimes

⋅

• Secure the bell dome to the bench with its

open end facing upwards using the table

clamp and plastic block.

• Strike the edge of the bell at different points

with a hammer. (Alternatively, the edges can

also be bowed with a violin bow.) The pitch depends on the point at which the bell

has been struck. It is easily possible to obtain differences of a whole tone. If the bell is struck at definite points, both tones are excited and the result is a familiar “beating” (periodic increase and decrease in volume at varying speeds).

Reasons: bells are curved vibrating plates. The overtones are mostly not in harmony with the fundamental tone. Bells too exhibit specific vibrating regions while they are chiming

14. Standing waves

• Make a simple knot in the resonance wire and

attach it by the loop to the handle of a door.

• Make the wire moderately taut and gently

move it round in circles.

• Now make the wire tighter and spin it faster.

When moved gently, nodes arise at both ends of the wire and an antinode is created in the middle of the wire. When moved faster, three nodes and two antinodes are formed, and when moved even faster, four nodes and three antinodes are formed.

Reasons: owing to the reflection at the door handle, standing waves are formed. Due to persistence of vision, the original and reflected waves appear to be simultaneous. In its fundamental mode, the whole of the wire vibrates in one length, thus describing one half-wave. One antinode is observed in the middle of the wire with nodes at both ends. In the case of a first harmonic (octave), the wire vibrates describes the form of a complete wave (two antinodes and three nodes); for the second harmonic, there are three antinodes and 4 nodes; and so on.

15. Overtones

• First blow the whistle gently, then blow it very

hard. Initially, a fundamental tone is heard. When the

whistle is blown hard, a much higher tone can be heard.

Reasons: since the whistle is closed at one end standing waves are always formed with a node at the base and an antinode at the blade opening. This is the case when the length of the whistle is exactly 1/4 of the wavelength. It is also the case if the distance of the opening from the base is 3/4, 5/4, 7/4, etc. of the wavelength.

Apart from the fundamental tone, all the possible odd overtones or harmonics from the harmonic series are produced at varying degrees of intensity.

The fact that every musical instrument has a very characteristic timbre can be attributed solely to the presence of individual harmonics of this kind appearing to a greater or lesser degree.

16. Measurement of wavelength

• Seal off the end of the 45-cm glass tube (21)

with the rubber cap and, holding the tube at an angle, put a small quantity of lycopodium powder into the tube using a teaspoon. Carefully spread a moderate quantity of the powder uniformly to form a fine yellow strip in the tube.

• Attach the glass tube by means of the retaining

clip, table clamp and plastic block.

• Strike the tuning fork (1700 Hz) hard on the

handle of the hammer and hold one prong directly alongside the opening of the tube. If necessary, repeat this acoustic excitation several times.

At the antinodes, the lycopodium powder begins to resonate strongly, whereas it is absolutely static at the nodes. The powder particles fall to the base of the tube and form periodic clusters that repeat 4½ times along the axis of the tube.

Reasons: the light-metal tuning fork has a frequency of 1700 vibrations per second. According to the following equation:

Wavelength =

⋅

/340

1700

Speed

Frequenncy

⋅=

2.0

The corresponding wavelength is 20 cm. Thus, 4½ half-waves or 2 full waves and one quarter wave can “fit” in a 45-cm-long tube, as demonstrated in the experiment. At the opening of the tube, there is always an antinode and there is always a node at the base of the tube.

17. Soundboard

• Hit the tuning fork that produces the note

a’ = 440 Hertz hard using the metallophone beater and push the stem down onto the table top.

Simply by holding it on the table, the barely audible tone produced by the tuning fork is amplified to such an extent that it is now clearly heard throughout the room.

Reasons: owing to the rising and falling vibrations in the shaft of the tuning fork, the surface of the table begins to resonate. Since the effective table surface is much larger than the tuning fork, the loudness of the tone is considerably intensified.

Page 14

18. Resonator box

• Strike the A tuning fork (440 Hz) nice and hard

and place its stem on the resonator box of the

monochord. There is a significant amplification of the tone.

Reasons: as explained in experiment 17.

19. Spherical cavity resonator

• One by one, bring the narrow tip of each of the

Helmholtz resonators close to your ear. You hear a tone which gets deeper as the diameter

of the resonator becomes greater. Reasons: every hollow space, regardless of its

shape, e.g. pipes, hollow spheres, has a very specific resonant frequency which is almost lacking overtones. This harmonic can be produced by blowing air across the opening of the hollow space or simply by tapping the hollow space with your knuckles. However, natural resonance is also created if the surrounding noise possesses tones which match the harmonic of the resonator. In this way, the spherical cavity resonator can be used to identify individual components of a mixed sound. If the room is absolutely quiet, the resonator remains silent.

20. String instruments and the laws they obey

• Insert the bridge vertically below the string of

the monochord so that its right edge exactly

coincides with the number 20 on the scale and

the 40-cm string is divided into two equal sec-

tions of 20 cm each.

• By tightening the peg, tune half the length of

the string to match the A tuning fork (440 Hz)

(standard pitch).

• By plucking, or preferably by bowing the

string, compare the pitch for string lengths of

40 cm, 20 cm, 10 cm and 5 cm. For a string length of 20 cm, the note matches the

standard concert pitch A' = 440 Hz. For a string length of 40 cm, the pitch is one octave lower at A = 220 Hz. For length 10 cm, the pitch is one octave higher A’’ = 880 Hz. Finally, when the length of the string is 5 cm, the pitch is two octaves higher A’’’ = 1760 Hz.

Reasons: when the string is twice as long, the pitch is lowered by one octave. When string length is half the length it is one octave higher and when the length of the string is reduced to a quarter, the note rises to the second octave. The frequency of a string vibration is inversely proportional to the string's length.

21. Scales on stringed instruments

• By moving the bridge, play the musical scale

that is tuneful to the human ear. In each case,

calculate the ratio of the vibrating section of

the string to the total length of the string (40 cm).

Tone String length Ratio of the string

length to the total

length of the string

C 40 cm 1

D 35.55 cm 8/9

E 32 cm 4/5 F 30 cm 3/4

G 26.66 cm 2/3

A 24 cm 3/5 B 21.33 cm 8/15 C’ 20 cm 1/2

Reasons: under consistent conditions (e.g. string length, string thickness, etc.), the sound is an octave higher when the string length is halved. In the case of the other tones on the musical scale, the relation between the vibrating section of the string’s length and its total length also forms simple ratios. The smaller the ratio, the more pleasing the harmony (octave 1:2, fifth C/G 2:3, etc.).

22. Measurement of string tension

• Attach the spring balance onto the monochord

and insert the end of the nylon string into the eye of the spring balance.

• Pull the peg and, using the A' tuning

fork (440 Hz), tune the string to standard pitch.

• Use the spring balance to determine the ten-

sion of the string.

The string tension in the case of a nylon string is

5.5 kg.

23. Relation between pitch and string tension

One of the results of experiment 22 was that in order to obtain a standard pitch, the tension on the nylon string needs to be 5.5 kg. How much tension should be applied in order to obtain a pitch that is one octave lower (A = 200 Hz)?

• Loosen the peg till you hear the pitch of A.

• To make sure this is right, place the bridge

under the string at 20 cm on the scale (i.e. half the total length of the string) and tune this half-of the string to standard pitch. Removing the bridge, the whole string will vibrate at half

the frequency. The string tension has been reduced to 1.4 kg. Reasons: the frequency of the string is proportional

to the square root of the tension. If the tensile force on the string is higher by a multiple of 4, 9, 16, etc., the frequency is increased two-fold, three-

Page 15

fold, four-fold, etc. As measured earlier, 1/4 of 5.5 is 1.4 (rounded up).

24. Wind instruments and the laws they obey

• Blow the whistle. You can change the effective

length of the whistle by moving the plunger.

When the length is short, the whistle produces a high tone and when it is longer, it produces a lower tone.

Reasons: when a weak air current passes through the whistle, standing waves are produced. In this case, the length of the whistle corresponds to a quarter wave length. When a strong air current passes through the whistle, overtones are produced whose frequency is an odd multiple of the fundamental tone.

In the case of an open whistle, the first harmonic is twice the frequency of that for a closed whistle.

25. C major scale and its intervals

• To determine the intervals, the higher fre-

quency is divided by the lower frequency.

For the interval D/C = 1188/1056, the common divisor is 132. We thus get ratios of 9/8, 10/9, 16/15, 9/8, 10/9, 9/8 and 16/15.

Reasons: the intervals between the individual tones of a musical scale are not equal. Intervals can be distinguished into the major tone (9/8), minor tone (10/9) and half-tone (16/15).

26. Harmony and dissonance

• Play all possible combinations on the reed

pipe.

Pleasing harmonies (consonances) are produced at the octave, the fifth note, the fourth, the major third and minor third. Discordant notes (dissonances) emerge between the second and seventh notes. The combination of tones produced by two neighbouring tones is also called dissonance.

27. G major triad

• Simultaneously blow notes G, B and D on the

reed pipe.

A highly melodious combination is heard. This combination of notes is termed the G major triad.

Reasons: consonance is produced if several notes produce a melodious combination of pairs. The G major triad is formed as a combination of the major third and the minor third. The frequencies of the notes G, Band D have a very simple ratio to one another, viz. 4:5:6.

In order to derive this ratio, the fundamental frequencies specified on the reed pipe should each be divided by 6.

(To obtain a physically correct frequency, the fundamental frequencies printed on the pipe need to be multiplied by 33).

It is also possible for the tuning of the reed pipe and metallophone to differ audibly due to manufacturing processes.

28. Four-part G major chord

• Add to the G major triad the G’ octave as well.

To achieve this, simultaneously play G, B, D

and G’. The result is a full and melodious “four-part G

major chord”. Reasons: a four-part major chord features the fol-

lowing consonances: Octave 1:2 Fifth 2:3 Major third 4:5 Minor third 5:6

29. Major scales in an arbitrary key

• First play the C major scale on the metallo-

phone. Begin with C. Subsequently play a simi-

lar scale starting from G. A C major scale from C’ to C’’ sounds pleasantly

consonant. If you try to play a similar scale starting at G’, though, there is a definite dissonance at F’’. The note is a semitone too low.

Reasons: according to experiment 25, the following intervals must be exhibited in every scale:

9/8, 10/9 16/15, 9/8, 10/9, 9/8, 16/15 For the sequence of notes G’…G’’, however, the

following intervals are specified on the base plate of the metallophone:

10/9, 9/8, 16/15

, 9/8, 10/9, 16/15, 9/8

The underlined intervals are correct, the others are incorrect in this sense.

The intervals 9/8 and 10/9 are so close to one another that it is extremely difficult to distinguish between them. Hence, the divergence from the ideal between G’ and B’ is irrelevant. However, the “imperfection’ between E’’ and F’’ is easily noticeable. In this case, an interval of 16/15 occurs instead of 9/8. The F’ note is therefore a semitone too deep.

30. Producing half-tones

• On the reed pipe, play the scale from G’ to G’’

making sure that the A’ note of the reed pipe is

genuinely tuned to standard pitch. Use the

tuning fork to compare the pitch. A G major scale on the reed pipe is pleasantly con-

sonant. Reasons: instead of the F’ note, a completely new

note, F#, is introduced. The interval between F’

Page 16

and F#’ is 9/8 and the interval between F#’’ and G’ is 16/15. This is achieved by taking the frequency of the F note and increasing it by multiplying it by 25/24.

The new notes produced by sharpening the tones are called C#, D#, F#, G# and A#. (E# and B# are equivalent to F and C respectively).

This sharpening is denoted in musical notation by a sharp sign appearing on the clave before the note.

Flat notes, which are a semitone lower than the conventional notes are produced by multiplying the latter by 24/25. These notes are denoted in musical notation by a flat sign preceding the note on the clave. The new flat notes are called Db, Eb, Gb, Ab and Bb.

In the tempered scale used on a piano, the notes C# and Db, D# and Eb, F# and Gb etc. respectively are played using the same key, since in each case they are close enough to being identical.

Elwe Didactic GmbH Steinfelsstr. 6 08248 Klingenthal Germany www.elwedidactic.com

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburg • Germany • www.3bscientific.com

Subject to technical amendments

Page 17

3B SCIENTIFIC

Ensemble Acoustique U8440012

Instructions d'utilisation

05/09 ELWE/ALF

PHYSICS

1. Description

Le jeu d’appareils de l’uníté didacticielle Acoustique permet l’enseignement de connaissances générales complètes sur le thème de l’acoustique. L’unité didacticielle permet de réaliser de nombreuses expériences.

Exemples d’applications :

1. Sons de cordes

2. Le pur son acoustique

3. Colonnes d’air vibrantes

4. Colonne d’air ouverte

5. Sifflet à bec

6. Barres vibrantes

7. Infrasons

8. Ultrasons

9. Diapason avec pointe d’enregistrement

10. Ondes progressives

11. Effet Doppler

12. Figures de Chladni

13. Oscillations de cloches

14. Ondes stationnaires

15. Sons harmoniques

16. Mesure de la longueur d’onde

17. Fond de résonance

18. Caisse de résonance

19. Résonateur sphérique

20. Principes des instruments à cordes

21. Gammes des instruments à cordes

22. Mesure de la tension des cordes

23. Rapport entre lhauteur du son et tension de

corde

24. Principes des instruments à vent

25. La gamme en do majeur et ses intervalles

26. Consonnance et dissonance

27. Accord parfait en sol majeur

28. Accord parfait en sol majeur à quatre voix

29. Gamme majeure avec n’importe quel son

fondamental

30. Interposition des demi-tons

Livraison sur un plateau en plastique équipé d’inserts en mousse protégeant chacune des pièces pendant leur stockage.

Page 18

2. Fournitures

1 Plateau avec insert en mousse pour ensemble

acoustique

2 Sonomètre 3 Barrettes pour sonomètre 4 Métallophone 5 Plaque de Chladni 6 Diapason, 1700 Hz 7 Diapason, 440 Hz 8 Diapason avec pointe d’enregistrement, 21 Hz 9 Balance à ressort

10 Clip de fixation

11 Pince étau de table 12 Résonateurs de Helmholtz Ø 70 mm Ø 52 mm Ø 40 mm Ø 34 mm 13 Tube de verre pour colonne d’air ouverte 14 Tube de Kundt

15 Tube de verre pour colonne d’air fermée 16 Tige pour plaque de Chladni / dôme de cloche 17 Sifflet de Galton 18 Crayon avec support 19 Poudre de lycopode 20 Bloc en matière plastique pour pince étau de

table 21 Capuchon en caoutchouc 22 Dôme de cloche 23 Tuyau à anche 24 Sifflet à bec 25 Cordes en acier 26 Cordes en perlon 27 Corde vibrante 28 Curseur de syntonisation

Page 19

⋅

3. Caractéristiques techniques

Dimensions : env. 530 x 375 x 155 mm3 Masse : env. 4.5 kg

4. Exemples d’expériences

1. Sons de cordes

• Pincer vigoureusement avec le doigt la corde

moyennement tendue du sonomètre.

• Augmenter la tension de la corde en tournant

la cheville à droite et pincer à nouveau cette dernière.

On perçoit tout d’abord un son grave puis un son plus aigu.

Explication : les cordes vibrantes produisent des sons acoustiques du fait de la compression et de la raréfaction alternatives de l’air ambiant. Plus la corde est tendue plus l’oscillation est rapide et plus le son est aigu.

2. Le pur son acoustique

• Faites fortement vibrer le diapason (440 Hz) en

utilisant le marteau du métallophone.

On perçoit un son acoustique pur de hauteur de son spécifique et invariable qui s’atténue lentement.

Explication : le diapason est composé d’un morceau d’acier courbé en U relié à une tige au point culminant (coude). Etant donné que le diapason ne vibre que selon un certain mode d’oscillations (mouvement contraire des branches de l’intérieur vers l’extérieur et inversement), il produit un son pur de hauteur invariable. On utilise le diapason pour accorder les instruments de musique en raison de cette hauteur de son constante.

3. Colonnes d’air vibrantes

• Fixez le tube de verre pour la colonne d'air

fermée sur la table de travail en utilisant la pince étau de table, le bloc en matière plastique ainsi que le clip de fixation.

• Introduisez le curseur de syntonisation dans le

tube de verre.

• Faites fortement vibrer le diapason (440 Hz) en

utilisant le marteau du métallophone. Modifiez la longueur de la « colonne d'air fermée » en étirant plus ou moins fortement le curseur de syntonisation.

La colonne d'air ne commence à résonner (phénomène de résonance) que pour une seule position du curseur de syntonisation, elle demeure muette dans toutes les autres positions prises par ce curseur. La résonance est perceptible par une croissance de l'intensité sonore.

Explication : les colonnes d’air fermées commencent à osciller lorsque leur longueur correspond à un quart de la longueur d’onde d’excitation. Le diapason vibre à un rythme de 440 vibrations par seconde. Selon l’équation

Longueurd' onde

34000 cm / s

⋅

440 Oscilations / s

Vitessede propagation

Frécuence

⋅

77,2 cm

la longueur d’onde du son produit est de 77,2 cm. Le quart de la longueur d’onde correspond donc à 19,3 cm.

En présence d'un phénomène de résonance, la distance entre le piston et l'ouverture du tube est de 19,3 cm.

4. Colonne d’air ouverte

• Réaliser l’expérience avec le tube de verre (14)

à colonne d’air ouverte. La colonne d’air ouverte dont la longueur est

exactement le double de celle de la colonne fermée commence également à osciller lorsqu’on tient un diapason à son ouverture, ce qui est perceptible par une croissance de l'intensité sonore.

Explication : les colonnes d’air commencent à osciller lorsque leur longueur correspond à une demi-longueur d’onde ou à un multiple de demilongueur d’onde. Des ventres d’oscillation se forment aux extrémités de la colonne d’air ouverte tandis qu’un noeud d’oscillation apparaît au milieu.

5. Sifflet à bec

• Souffler dans le sifflet à bec et modifier la

longueur du sifflet en sortant le piston. Suivant la longueur du piston, on perçoit un son

plus ou moins grave au timbre caractéristique. Explication : en soufflant un courant d’air régulier

dans l’ouverture du sifflet, l’air enfermé dans le corps du sifflet se met à vibrer de telle sorte que des tourbillons d’air se détachent du bec (biseau) à intervalles réguliers. Le son produit dépend de la longueur de la colonne d’air. Pour le sifflet fermé, la longueur de sifflet (mesurée à partir du bord du biseau jusqu’au fond) correspond au son fondamental d’un quart de longueur d’onde. Sur l’arête se forme une surface ventrale et sur le fond un nœud.

6. Barres vibrantes

• Frapper quelques barres du métallophone au

moyen du marteau de percussion fourni. La percussion des barres métalliques au moyen du

marteau génère des sons harmonieux au timbre très caractéristique. Plus la barre est courte, plus le son est aigu.

Page 20

Explication : des barres élastiques se transforment en systèmes mécaniques pouvant vibrer si elles reposent sur les points de leurs nœuds vibratoires (à une distance des extrémités égale à environ 22 % de la longueur totale).

7. Infrason

• Faire osciller le diapason à pointe

d’enregistrement en exerçant une pression simultanée sur les deux branches et en les relâchant ensuite brusquement.

Le diapason exécute des oscillations lentes qui sont encore bien perceptibles à l’œil. En collant le diapason à l’oreille, on perçoit un son très grave (encore à peine perceptible).

Explication : les branches du diapason vibrent en sens opposé dans un va-et-vient et compriment et raréfient l’air environnant Si ces compressions et raréfactions tombent dans l’oreille, le tympan se met à vibrer en même temps. On entend alors un son.

Le diapason oscille à raison d’environ 20 oscillations à la seconde. Le son le plus grave qui puissent encore être perçu possède environ 16 oscillations à la seconde. Les vibrations inférieures à 16 Hertz ne sont plus perceptibles à l’ouïe. On les désigne sous le terme d’infrasons (en latin infra signifie en-dessous).

8. Ultrason

• Souffler dans le sifflet de Galton.

On ne perçoit plus aucun son mais seulement un bruit comparable à un sifflement.

Résultat : en raison de sa longueur réduite, le sifflet de Galton produit des sons très aigus qui ne sont plus perceptibles pour l’oreille humaine. On les désigne sous le terme d’ultrasons (en latin ultra signifie au-dessus).

9. Diapason avec pointe d’enregistrement

• Fixer le crayon aux branches du diapason à

pointe d’enregistrement.

• Faire osciller le diapason en pressant les

branches l’une contre l’autre et déplacer le crayon régulièrement sur une feuille de papier posée sur un support suffisamment rigide.

Le crayon dessine sur le papier une ligne ondulée constituée de longueurs d’ondes constantes mais d’amplitude décroissante.

Explication : le son est généré par l’oscillation périodique de corps solides, liquides ou gazeux. Le lieu géométrique des particules vibrantes du corps en fonction du temps se situe sur une ligne ondulée (la ligne sinusoïde). En cas de choc unique, les corps vibrants exécutent une oscillation « amortie » (diminution constante de l’amplitude). Si l’alimentation en énergie est constante (tonalité

continue d’un klaxon, tuyau d’orge constamment alimenté en air), nous obtenons une oscillation non amortie d’amplitude constantes (= puissance sonore).

10. Ondes progressives

• Nouer la boucle de la corde vibrante à une

poignée de porte.

• Tendre moyennement la corde et exécuter un

mouvement latéral par à-coups avec la main. Un ondulation est générée à partir du centre du

mouvement (la main) qui parcourt la corde avec une certaine vitesse de progression, se réfléchit à l’extrémité fixe et revient au point de départ.

Explication : tout corps solide, liquide et gazeux génère des oscillations lors d’une secousse soudaine ; ces oscillations se propagent sur le support vibrant avec une certaine vitesse de propagation.

11. Effet Doppler

• Frapper le diapason en métal léger (1700 Hz)

en utilisant le marteau du métallophone, le

maintenir brièvement au repos et l’agiter de

part et d’autre ensuite rapidement dans l’air. Lorsqu’il est au repos, le diapason produit un son

fort d’une hauteur constante. Lorsqu’il se trouve en mouvement, la hauteur du son varie constamment. Si l’on effectue le mouvement en le rapprochant de l’oreille, le son augmente, si le mouvement est éloigné de l’oreille, le son diminue.

Explication : la diminution de la distance de la source sonore par rapport à l’oreille raccourcit l’intervalle temporel entre deux compressions étant donné que la 2

ème

compression effectue un parcours plus court vers l’oreille que la première. L’oreille perçoit une fréquence plus élevée. Le son devient plus aigu. Si l’on éloigne la source sonore de l’oreille, les intervalles de temps entre les compressions et les raréfactions sont prolongés. Le son devient plus grave.

12. Figures de Chladni

• Fixer la plaque de Chladni au moyen de la

pince-étau et du bloc en matière plastique au plan de travail. Répartir du sable anisé ou un matériau similaire sur la plaque, de façon à ce que celui-ci recouvre un tiers de la plaque d’une fine couche.

• Faire glisser un archet de violon bien enduit de

colophane exactement au milieu de deux extrémités en touchant régulièrement et légèrement une extrémité avec le doigt de l’autre main.

• Faire glisser l’archet plusieurs fois avec vigueur

sur la plaque de manière à ce que celle-ci

Page 21

commence à produire de fortes vibrations bien perceptibles.

Lorsque l’archet glisse sur la plaque, on perçoit un son acoustique très particulier. A certains endroits, les grains de sable se mettent également à vibrer fortement et sautent à la surface de la plaque pour ensuite former des figures sonores étranges à la surface.

Explication : des « ondes statiques » se forment sur la plaque. La plaque ne vibre pas simplement dans son ensemble au contact de l’archet mais se met à vibrer à certains endroits (les ventres d’oscillation) alors qu’à d’autres (les nœuds), elle est complètement inerte. Le contact de la plaque à une extrémité a provoqué un nœud à cet endroit.

13. Vibrations de cloches

• Fixez le dôme de cloche avec son ouverture

dirigée vers le haut sur la table de travail en utilisant la pince étau de table ainsi que le bloc en matière plastique.

• Frapper sur le bord de la cloche à divers

endroits avec le marteau (vous pouvez aussi utiliser l’archet de violon à cet effet).

La hauteur du son dépend du point d’impact. Il est tout à fait possible d’obtenir des différences pour un son entier. Si on frappe sur la cloche à certains endroits, les deux sons sont activés et l’on obtient le « battement » bien connu (croissance et décroissance périodique de l’intensité sonore dans une succession plus ou moins rapide).

Explication : les cloches sont des plaques vibrantes déformées. Les sons harmoniques ne sont la plupart du temps pas en harmonie avec le son fondamental. Les cloches se répartissent elles aussi en différentes zones d’oscillations séparées par des lignes de nœuds.

14. Ondes stationnaires

• Nouer la boucle de la corde vibrante à une

poignée de porte.

• Tendre moyennement la corde et exécuter des

mouvements lents circulaires avec la main.

• Augmenter ensuite la tension de la corde et

augmenter la vitesse des mouvements circulaires.

Les mouvements lents provoquent l’apparition de nœuds aux extrémités de la corde et d’un ventre d’oscillation au milieu. Les mouvements plus rapides génèrent trois nœuds et 2 ventres d’oscillation et lorsqu’ils sont encore accélérés 4 nœuds et 3 ventres d’oscillation.

Explication : la réflexion sur la porte entraîne la formation d’ondes statiques. L’inertie de l’oeil fait que celui-ci perçoit apparemment l’onde source et l’onde réfléchie simultanément.

Dans la composante fondamentale, la corde oscille sur toute sa longueur sous la forme d’une demionde. Un ventre d’oscillation se trouve au milieu et des nœuds aux deux extrémités. Dans la 1

ère

harmonique (octave), la corde vibre sous la forme d’une onde entière (2 ventres d’oscillation et 3 nœuds). Dans la 2

ème

harmonique, nous avons 3

ventres d’oscillation et 4 nœuds, etc.

15. Sons harmoniques

• Souffler dans le sifflet à bec avec la bouche,

d’abord faiblement puis fortement.

On perçoit tout d’abord le son fondamental, puis un son nettement plus aigu lorsque l’on souffle plus fort.

Explication : pour ce sifflet couvert, des ondes statiques doivent se former en permanence de façon à ce que un nœud apparaisse au fond et un ventre d’oscillation sur l’arête du bec. C’est le cas lorsque la longueur du sifflet correspond exactement à 1/4 de longueur d’onde. C’est également le cas lorsque la distance de l’ouverture par rapport au fond correspond à 3/4, 5/4, 7/4, etc. des longueurs d’ondes.

Outre le son fondamental, un grand nombre de sons harmoniques de nombre impair de la suite sonore harmonique sont donc également produits de manière plus ou moins accentuée.

Chaque instrument de musique ne doit son timbre tout à fait caractéristique qu’à l’apparition plus ou moins prononcée de sons harmoniques.

16. Mesure de la longueur d’onde

• Obturer l’extrêmité du tube de verre dont la

longueur exacte est de 45 cm avec le capuchon en caoutchouc et remplir le tube d’une petite quantité de poudre de lycopode au moyen d’une cuiller à café en maintenant le tube incliné, de façon à ce que la poudre soit bien répartie en petite quantité et forme une fine bande jaune à l’intérieur du tube.

• Fixez le tube de verre sur la table de travail en

utilisant le clip de fixation, la pince étau de table ainsi que le bloc en matière plastique.

• Frapper très fortement le diapason (1700 Hz)

sur un manche de marteau et tenir le bord d’une branche tout près de l’ouverture du tube. Reproduire éventuellement plusieurs fois ce son.

La poudre de lycopode se met à vibrer vigoureusement près des ventres d’oscillation alors qu’elle demeure complètement inerte aux nœuds. Les particules de poudre tombent au fond du tube et forment à cet endroit des amoncellements périodiques qui se répètent 4,5 fois le long de l’axe du tube.

Page 22

Explication : le diapason en métal léger possède une fréquence de 1700 vibrations à la seconde. Selon l’équation simple

Longueurd' onde

s/m

⋅

340

⋅

1700

Vitessedu son

récuence

⋅=

la longueur d’onde correspondante est de 20 cm. Dans un tube de 45 cm de long, il est donc possible « d’emmagasiner » 4½ demi-ondes ou 2 ondes pleines et un quart d’onde, comme l’a montré l’expérience. On obtient toujours un ventre d’oscillation à l’ouverture du tube et un nœud au fond.

17. Fond de résonance

• Faites fortement vibrer le diapason a’ = 440

Hertz en utilisant le marteau du métallophone et placez-le sur le plateau de table en utilisant sa tige.

Le son du diapason à peine perceptible à l’air libre est à ce point amplifié lorsque le diapason est posé sur la table qu’on peut à présent l’entendre clairement dans toute la pièce.

Explication : entraînée par les vibrations amplifiées et désamplifiées du manche, la table se met à vibrer. Etant donné que la surface effective de la table est sensiblement plus grande que celle du diapason, l’intensité du son s’accroît considérablement.

18. Caisse de résonance

• Frapper avec force le diapason a’ = 440 Hertz

et le poser sur le manche sur la caisse de

résonnance du sonomètre.. On obtient une amplification significative du son. Explication : même explication que pour

l’expérience 17.

19. Résonateur sphérique

• Tenir les résonateurs de Helmholtz l’un après

l’autre avec la petite pointe contre l’oreille. On perçoit un son dont la gravité est

proportionnelle à la taille du diamètre du résonateur.

Explication : chaque cavité quelle qu’elle soit (tube, sphère creuse) possède une certaine composante fondamentale, quasiment exempte de sons harmoniques. Cette composante fondamentale peut être activée en soufflant dans la cavité par l’ouverture de cette dernière ou en frappant seulement avec le doigt replié contre la cavité. L’oscillation propre n’est cependant en premier lieu également activée que lorsque le bruit environnant contient des sons qui sont en harmonie avec la composante fondamentale du

résonateur. Le résonateur sphérique permet donc de vérifier le contenu en sons partiels d’un son. Lorsqu’un silence absolu règne dans une pièce, le résonateur demeure muet.

20. Principes des instruments à cordes

• Glisser la barrette sur chant sous la corde du

sonomètre de façon à ce que le bord droit corresponde exactement au chiffre 20 de l’échelle de mesure et que la corde de 40 cm soit divisée en deux sections d’une longueur égale à 20 cm chacune.

• Accorder la moitié de la longueur de la corde

au diapason (440 Hz) a’ (la du diapason) en tournant la cheville.

• Comparer les hauteurs du son à 40 cm, 20 cm,

10 cm et 5 cm de longueur de corde en pinçant la corde ou mieux, en utilisant un archet.

A une longueur de corde de 20 cm, on obtient le ton-étalon a’ = 440 Hertz, à 40 cm, le ton a = 220 Hertz, plus grave d’un octave, à 10 cm le ton a’’ = 880 Hertz plus haut d’un octave et à une longueur de corde de 5 cm, le ton a’’’ = 1760 Hertz, plus aigu de 2 octaves..

Explication : pour une longueur de corde double, on obtient un ton plus grave d’une octave, pour une demi-longueur de corde la 1 un quart de longueur de corde, la 2

ère

octave et pour

ème

octave. Les fréquences des cordes ont un comportement inversement proportionnel à celui de leurs longueurs.

21. Gammes des instruments à cordes

• Jouer la gamme connue à l’oreille humaine sur

le sonomètre en déplaçant la barrette et calculer les longueurs respectives de la section de corde vibrante et le rapport de cette section à la longueur totale de la corde (40 cm).

Ton Longueur de

corde

Rapport de

longueur Do 40 cm 1 Ré 35,55 cm 8/9 Mi 32 cm 4/5

Fa 30 cm 3/4

Sol 26,66 cm 2/3

La 24 cm 3/5

Si 21,33 cm 8/15

Do’ 20 cm 1/2

Explication : la longueur de la corde doit être réduite de moitié si l’on veut atteindre l’octave sous les mêmes conditions (tension, épaisseur de la corde, etc.). Pour les autres tons de la gamme, on obtient des indices très simples exprimant le

Page 23

rapport entre les longueurs de cordes vibrantes et la longueur total de la corde. Plus ces chiffres sont petits, plus le son est mélodieux. (Octave 1:2, Quinte Do/sol 2:3 etc.)

22. Mesure de la tension des cordes

• Placer la balance à ressort sur le sonomètre et

suspendre l’extrêmité de la corde en perlon dans la fente de la balance.

• Accorder la corde en utilisant le

diapason a’ = 440 Hertz au ton-étalon en vissant la cheville.

• Déterminer la tension de la corde avec la

balance à ressort.

La tension de la corde est de 5,5 kg pour la corde en perlon.

23. Rapport entre la hauteur du son et la tension de la corde

L’expérience 22 a montré que pour obtenir le tonétalon, il fallait tendre la corde en perlon avec 5,5 kg. Quelle est la tension d’une corde pour un ton La plus grave d’un octave (220 hertz) ?

• Dévisser la cheville jusqu’à ce que le ton se

produise.

• Placer la barrette sous le chiffre 20 (demi-

longueur de corde) pour vérifier et accorder à nouveau la demi-longueur de corde au tonétalon (la du diapason). La corde entière vibre

alors à la mi-fréquence. La tension de la corde est passée à 1,4 kg. Explication : la fréquence d’une corde est

proportionnelle à la racine carrée du poids de tension. Si la force qui tend la corde est multipliée par 4, 9, 16, la fréquence augmente du double, du triple et du quadruple. 1/4 de 5,5 est (environ) égal à 1,4, comme l’indique la mesure prise.

24. Principes des instruments à vent

• Souffler dans le sifflet à bec avec la bouche et

modifier la longueur effective du sifflet en en

tirant plus ou moins le fond. Pour une faible longueur de sifflet, on obtient des

sons aigus et pour une longueur plus importante des sons plus graves.

Explication : si le flux d’air est faible, des ondes statiques se forment, la longueur du sifflet correspondant alors à une longueur de quart d’onde. Si le flux d’air est plus important, on obtient des sons harmoniques dont la fréquence est un multiple impair du son fondamental.

Si le sifflet est ouvert, la composante fondamentale est deux fois plus importante que celle du sifflet fermé.

25. La gamme en do majeur et ses intervalles

• Pour déterminer les intervalles, il faut diviser

la fréquence la plus élevée par la fréquence la plus basse la plus proche.

Pour l’intervalle la/do =1188/1056, le dénominateur commun est 132, on obtient donc 9/8, 10/9, 16/15, 9/8, 10/9, 9/8 et 16/15.

Explication : les intervalles de chaque ton de la gamme ne sont pas de taille identique. Nous faisons une distinction entre de grands intervalles composés de tons entiers (9/8), entre de petits intervalles composés de tons entiers (10/9) et entre des intervalles de demi-tons (16/15).

26. Consonnance et dissonance

• Jouer les différents accords sur le sifflet à bec.

On obtient de vraies consonnances pour l’octave, la quinte, la quarte, la tierce majeure et la tierce mineure. Les dissonances correspondent à la seconde et la septième ainsi qu’à l’accord des tons directement voisins.

27. Accord parfait en sol majeur

• Produire simultanément les tons sol mir ré sur

le sifflet à bec.

On perçoit un accord d’une consonnance particulière que l’on désigne par accord parfait en sol majeur.

Explication : lorsque plusieurs tons sont supposés former une consonnance, ils doivent le faire par paire. L’accord parfait en sol majeur est composé d’une tierce majeure et d’une tierce mineure. Le rapport entre les fréquences des tons sol mi ré est un rapport très simple, à savoir : 4:5:6.

Pour obtenir ce rapport numérique, il faut que les fréquences fondamentales indiquées sur le sifflet à bec soient respectivement divisées par 6.

(Les fréquences de base imprimées ont à être multipliées par 33 afin d'obtenir fréquences physiquement correctes).

Pour des raisons de fabrication, le tuyau à anche et le métallophone peuvent en outre se différencier par un écart de gamme audible.

28. Accord parfait en sol majeur à quatre voix

• Ajouter l’octave sol’ à l’accord parfait en sol

majeur. Jouer donc simultanément sol si do’ sol’.

On obtient « un accord parfait en sol majeur à quatre voix » particulièrement complet et consonnant.

Explication : L’accord parfait à quatre voix contient les consonnances suivantes :

l’octave 1:2 la quinte 2:3 la tierce majeure 4:5 la tierce mineure 5:6

Page 24

29. Gamme majeure avec n’importe quel son

fondamental

• Sur le métallophone, jouer d’abord la gamme

en do majeur en commençant par do et

ensuite la gamme en sol majeur en

commençant par sol. La gamme en do majeur de do’ à do’’ possède un

son pur. Une grave erreur apparaît à fa’ dans la gamme en do majeur commençant par do’. Le ton est d’un demi-ton trop grave.

Explication : selon l’expérience n° 25, pour chaque gamme, il faut avoir les intervalles suivants :

9/8, 10/9 16/15, 9/8, 10/9, 9/8, 16/15. La succession de tons sol’…sol’’ sur la plaque du

métallophone a cependant les intervalles suivants : 10/9, 9/8, 16/15

, 9/8, 10/9, 16/15, 9/8

Les intervalles soulignés sont corrects, les autres plus ou moins erronés.

Les intervalles 9/8 et 10/9 sont juxtaposés mais trop près, de telle sorte qu’ils se distinguent à peine l’un de l’autre, ce qui explique que l’erreur de sol’ à si' est peu importante. Par contre, l’erreur entre mi’’ et fa’’ est grave. L’intervalle est ici de 16/15, alors qu’en réalité, il devrait être de 9/8. C’est la raison pour laquelle on entend un fa’’ d’un demi-ton trop grave.

30. Jouer des intervalles de demi-tons

• Jouer la gamme de sol’ à sol’’ sur le sifflet à bec

après s’être assure que le ton la’ du sifflet est bien accordé avec le la du diapason. Pour cela, faire vibrer le diapason et effectuer la comparaison.

La gamme en sol majeur jouée sur le sifflet est entièrement pure.

Explication : A la place du ton fa’, un nouveau ton est interposé, le fa dièse’ qui est calculé de manière à ce que l’intervalle entre mi’ et fis’ soit de 9/8 et celui entre fa dièse’’ et sol’’ de 16/15. Ceci est dû au fait que l’on augmente la fréquence du fa en la multipliant par 25/24.

Les nouveaux tons résultant de l’augmentation des tons sont appelés : do dièse, ré dièse, mi dièse, fa dièse, sol dièse, la dièse, si dièse

On signale cette augmentation par l’ajout d’une croix placée en avant sur la partition.

Les tons plus bas d’un demi-ton sont obtenus en multipliant le ton plus élevé par 24/25. Ces tons sont signalés sur la partition par l’ajout d’un b placé en avant. On les appelle : do bémol, ré bémol, mi bémol, fa bémol, sol bémol, la bémol, si bémol.

Les tons do dièse et do bémol, etc. sont assimilés sur le piano avec un nombre restreint d’erreurs.

Elwe Didactic GmbH ▪ Steinfelsstr. 6 ▪ 08248 Klingenthal ▪ Allemagne ▪ www.elwedidactic.com

3B Scientific GmbH ▪ Rudorffweg 8 ▪ 21031 Hambourg ▪ Allemagne ▪ www.3bscientific.com

Sous réserve de modifications technique

Page 25

Fisica 3B Scientific®

Apparecchio didattico per acustica U8440012

Istruzioni per l'uso

05/09 ELWE/ALF

1. Descrizione

Il kit “Apparecchio didattico per acustica” consente di organizzare una vasta panoramica sull’argomento acustica realizzando numerosi esperimenti.

Esempi di esperimenti:

1. Note di una corda

2. La nota acustica pura

3. Colonnine d’aria oscillanti

4. La colonnina d’aria aperta

5. La canna labiale

6. Aste oscillanti

7. Infrasuoni

8. Ultrasuoni

9. Il diapason con punta scrivente

10. Onde progressive

11. Effetto Doppler

12. Figure sonore di Chladni

13. Oscillazioni delle campane

14. Onde stazionarie

15. Armoniche superiori

16. Misurazione della lunghezza d’onda

17. Il fondo di risonanza

18. La cassa di risonanza

19. Il risuonatore sferico

20. Gli strumenti a corda e le loro leggi

21. Le scale sugli strumenti a corda

22. Misurazione della tensione della corda

23. Dipendenza dell’altezza della nota dalla tensione della corda

24. Gli strumenti a fiato e le loro leggi

25. La scala di do maggiore e i suoi intervalli

26. Armonia e dissonanza

27. L’accordo di terza di sol maggiore

28. L’accordo di terza di sol maggiore a quattro voci

29. La scala maggiore con qualsiasi tonica

30. Inserimento dei mezzi toni

Il kit è fornito in una confezione in plastica con inserti in espanso per conservare con cura i singoli componenti.

Page 26

2. Fornitura

1 Confezioni con inserto in espanso il kit

“Apparecchio didattico per acustica” 2 Monocordo 3 Asta per monocordo 4 Metallofono 5 Piastra di Chladni 6 Diapason, 1700 Hz 7 Diapason, 440 Hz 8 Diapason con punto scrivente, 21 Hz 9 Bilancia a molla 10 Morsetto di supporto 11 Morsetto da tavolo 12 Risuonatori di Helmholtz Ø 70 mm Ø 52 mm Ø 40 mm Ø 34 mm 13 Tubo di vetro per colonnina d’aria aperta

14 Tubo di Kundt 15 Tubo di vetro per colonnina d’aria chiusa

16 Asta per piastra di Chladni / calotta della

campana 17 Fischietto di Galton 18 Penna per scrittura con supporto 19 Polvere di licopodio 20 Blocco di plastica per morsetto da tavolo 21 Membrana di gomma 22 Calotta della campana 23 Canna ad ancia 24 Canna labiale 25 Corda di acciaio 26 Corda in perlon 27 Corda per onde 28 Pistone

Page 27

3. Dati tecnici

Dimensioni: ca. 530 x 375 x 155 mm3 Peso: ca. 4,5 kg

4. Esempi di esperimenti

1. Note di una corda

• Pizzicare con forza con il dito la corda

moderatamente tesa del monocordo.

• Quindi aumentare la tensione della corda

ruotando il cavicchio verso destra e pizzicarla di nuovo.

Si percepisce prima una nota bassa, quindi una nota più alta.

Spiegazione: le corde oscillanti producono note acustiche provocando delle compressioni ed espansioni alternate dell’aria circostante. All’aumentare della tensione della corda cresce la velocità di oscillazione, e quindi la nota risulta più alta.

2. La nota acustica pura

• Colpire con forza il diapason (440 Hz) con il

martelletto del metallofono.

Si percepisce una nota acustica pura con altezza del suono ben definita e invariabile, che si smorza lentamente.

Spiegazione: il diapason è costituito da un elemento d’acciaio piegato a forma di U che i corrispondenza della sommità (arco) si trasforma in un’asta. Potendo oscillare secondo una sola forma di oscillazione (movimento opposto dei rebbi dall’interno verso l’esterno e viceversa), il diapason produce una nota pura di altezza invariabile. A causa dell’altezza invariabile della nota prodotta, il diapason è utilizzato per accordare gli strumenti musicali.

3. Colonnine d’aria oscillanti

• Fissare il tubo di vetro per colonnina d’aria

chiusa mediante il morsetto da tavolo, il blocco di plastica e il morsetto di supporto sul tavolo da lavoro.

• Introdurre il pistone nel tubo di vetro.

• Colpire con forza il diapason (440 Hz) con il

martelletto del metallofono. Variare la lunghezza della “colonnina d’aria chiusa” estraendo di più o di meno il pistone.

La colonnina d’aria oscilla (risonanza) in una sola posizione del pistone, in tutte le altre posizioni rimane silenziosa. La risonanza è percepibile grazie all‘aumento del volume.

Spiegazione: le colonnine d’aria chiuse entrano in oscillazione quando la loro lunghezza corrisponde

a un quarto della. lunghezza d’onda da eccitare. Il diapason compie 440 oscillazioni al secondo. In base alla relazione

ondalunghezzad ='

ionedipropagazvelocità

frequenza

scm

⋅

/34000

⋅

la lunghezza d’onda della nota prodotta è pari a 77,2 cm. Pertanto la lunghezza di quarto d’onda è pari a 19,3 cm.

La distanza del pistone dall’apertura del tubo in caso di risonanza è pari a 19,3 cm.

4. La colonnina d’aria aperta

• Eseguire l’esperimento con il tubo di vetro (14)

con la colonnina d’aria aperta. Quando si posiziona il diapason, la colonnina

d’aria aperta entra in oscillazione ad una lunghezza pari esattamente al doppio di quella chiusa, il che è percepibile grazie all'aumento del volume.

Spiegazione: le colonnine d’aria aperte entrano in oscillazione quando la loro lunghezza corrisponde a mezza lunghezza d’onda o a un multiplo di mezza lunghezza d’onda. Alle estremità della colonnina d’aria aperta si formano dei ventri di oscillazione, al centro un nodo di oscillazione.

5. La canna labiale

• Soffiare nella canna labiale e variarne la

lunghezza estraendo il pistone. A seconda della lunghezza della canna si

percepisce una nota più o meno profonda con un colore del suono caratteristico.

Spiegazione: soffiando un flusso d’aria uniforme nella bocca della canna l’aria in essa contenuta entra in oscillazione perché sul bordo (tagliente) si producono in successione regolare dei vortici d’aria. La nota prodotta dipende dalla lunghezza della colonnina d’aria. Con la canna chiusa, la lunghezza della canna (misurata dal bordo del tagliente al fondo) corrisponde alla tonica di una lunghezza di quarto d’onda. In corrispondenza del tagliente si crea un ventre e sul fondo un nodo.

6. Aste oscillanti

• Percuotere alcune aste del metallofono con il

martelletto in dotazione. Percuotendo le aste metalliche si producono delle

note armoniche con colore del suono molto caratteristico. Più corta è l’asta più alta risulta la nota.

Spiegazione: Le aste elastiche diventano sistemi in grado di oscillare, se appoggiano sui punti dei loro

soscill

/440

⋅=

2,77

Page 28

nodi di oscillazione (circa il 22% dell’intera lunghezza tra le estremità).

7. Infrasuoni

• Far entrare in oscillazione il diapason con

punta scrivente premendo contemporaneamente i due rebbi uno verso l’altro e lasciandoli andare all’improvviso.

Il diapason esegue delle oscillazioni lente ma ben distinguibili a occhio nudo. Avvicinandolo all’orecchio, si percepisce un suono molto basso (ma ancora udibile).

Spiegazione: i rebbi del diapason oscillano su e giù in senso opposto, producendo delle compressioni ed espansioni dell’aria circostante che, quando arrivano all’orecchio, determinano un’oscillazione del timpano: si sente un suono.

Il diapason compie circa 20 oscillazioni al secondo. La nota bassa ma ancora udibile ha circa 16 oscillazioni al secondo. Le oscillazioni inferiori a 16 Hertz non sono più udibili e sono definite infrasuoni (lat. infra = sotto).

8. Ultrasuoni

• Soffiare nel fischietto di Galton.

Non si percepisce più un suono, ma solo un sibilo. Risultato: a causa della sua lunghezza ridotta, il

fischietto di Galton produce suoni troppo alti, non più udibili dall’uomo. Questi suoni vengono definiti ultrasuoni (lat. ultra = sopra).

9. Il diapason con punta scrivente

• Fissare la penna per scrittura ai rebbi del

diapason con punta scrivente.

• Far entrare in oscillazione il diapason

premendo i rebbi l’uno contro l’altro, quindi passare uniformemente con la penna per scrittura su un foglio di carta posto su un piano d’appoggio non troppo morbido.

La penna di scrittura disegna sul foglio una linea ondulata con lunghezza d’onda costante, ma ampiezza calante.

Spiegazione: l’oscillazione periodica di corpi solidi, liquidi o aeriformi produce un suono. La posizione geometrica delle particelle oscillanti del corpo in funzione del tempo è situata su una linea ondulata (sinusoide). Nel caso di un unico urto i corpi oscillanti eseguono un'oscillazione "smorzata" (diminuzione costante dell’ampiezza). Se l’apporto di energia è continuo (suono prolungato di un clacson, canna d’organo suonata in modo prolungato) si ottiene un’oscillazione non smorzata di ampiezza costante (= volume).

10. Onde progressive

• Fissare il cappio della corda per onde al

campanello di una porta con un semplice nodo.

• Tendere moderatamente la corda e con la

mano effettuare un brusco movimento laterale. Un’onda parte dal centro del movimento (la mano),

scorre lungo la corda con una determinata velocità di propagazione, viene riflessa in corrispondenza dell’estremità fissa e torna al punto di partenza.

Spiegazione: se scosso all’improvviso, ogni corpo solido, fluido o aeriforme compie delle oscillazioni che si propagano nel mezzo oscillante con una determinata velocità di propagazione.

11. Effetto Doppler

• Battere con forza il diapason in metallo

leggero (1700 Hz) con il martelletto del

metallofono, tenerlo fermo per breve tempo e

quindi muoverlo rapidamente su e giù in aria. Quando è fermo, il diapason produce una forte

nota di altezza costante. Mentre si muove, l’altezza della nota varia continuamente. Muovendo il diapason verso l’orecchio la nota si alza, allontanandolo dall’orecchio si abbassa.

Spiegazione: al diminuire della distanza tra la sorgente sonora e l’orecchio l’intervallo temporale tra le due compressioni diminuisce, perché per raggiungere l’orecchio la seconda compressione compie un percorso più breve della prima. L’orecchio percepisce una frequenza superiore e la nota diventa più alta. Allontanando la sorgente sonora dall’orecchio l’intervallo temporale tra le compressioni e le espansioni si allunga: la nota diventa più bassa.

12. Figure sonore di Chladni

• Fissare la piastra di Chladni al tavolo di lavoro

utilizzando il morsetto da tavolo e blocco di

plastica. Coprire un terzo della piastra con uno

strato sottile di sabbietta per uccelli o simile.

• Ora sfregare la piastra con un archetto da

violino ben spalmato di colofonia proprio al

centro tra due angoli e contemporaneamente

toccare leggermente un angolo col dito

dell'altra mano.

• Sfregare più volte con forza la piastra in modo

che produca un’oscillazione intensa e ben

udibile. Sfregando la piastra si percepisce una nota acustico

ben definita. In alcuni punti i granelli di sabbia oscillano intensamente, danzando su e giù sulla piastra e raccogliendosi in peculiari figure sonore sulla sua superficie.

Page 29

Spiegazione: sulla piastra si formano delle "onde stazionarie". Quando la si sfrega, la piastra non oscilla uniformemente in alto e in basso: in determinati punti (i ventri) entra in oscillazione, mentre in altri (i nodi) rimane assolutamente ferma. Toccando la piastra su un angolo, in tale punto si produce forzatamente un nodo.

13. Oscillazioni delle campane

• Fissare la calotta della campana nel morsetto

da tavolo con l’apertura rivolta verso l’alto.

• Percuotere in diversi punti il bordo della

campana con il martelletto (in alternativa sfregarlo con l’archetto da violino).

L’altezza della nota dipende dal punto in cui si percuote il bordo. Si possono certamente ottenere differenze di un’intera nota. Percuotendo la campana in determinati punti si eccitano entrambe le note ottenendo le famose “fluttuazioni” (aumento e diminuzione periodica del volume in una successione più o meno rapida).

Spiegazione: le campane sono piastre oscillanti forgiate. Nella maggior parte dei casi le armoniche superiori non sono armoniche rispetto alla tonica. Anche le campane sono divise in singole sezioni oscillanti mediante linee nodali.

14. Onde stazionarie

• Fissare il cappio della corda per onde al

campanello di una porta con un semplice nodo.

• Tendere moderatamente la corda ed eseguire

dei lenti movimenti circolari con la mano.

• Quindi tendere maggiormente la corda ed

eseguire dei movimenti circolari più veloci.

Col movimento lento si formano dei nodi in corrispondenza delle estremità della corda e un ventre al centro. Col movimento più veloce si formano 3 nodi e 2 ventri, aumentando ancora la velocità del movimento otteniamo 4 nodi e 3 ventri.

Spiegazione: con la riflessione sulla porta si creano delle onde stazionarie. A causa dell’inerzia dell’occhio sembra di vedere contemporaneamente l’onda originaria e l’onda riflessa.

Nella prima armonica la corda oscilla su e giù per la sua intera lunghezza sotto forma di una semionda. Al centro c’è un ventre, alle due estremità i nodi. Nella prima armonica (ottava) la corda oscilla sotto forma di un’onda intera (2 ventri e 3 nodi). Nella seconda armonica abbiamo 3 ventri e 4 nodi e via di seguito.

15. Armoniche superiori

• Soffiare con la bocca nella canna labiale

dapprima debolmente e poi con molta forza.

Inizialmente si percepisce la tonica, soffiando più forte una nota nettamente più alta.

Spiegazione: con la canna chiusa, per avere un nodo sul fondo e un ventre sul tagliente si devono sempre formare delle onde stazionarie. È il caso in cui la lunghezza della canna corrisponde esattamente a 1/4 della lunghezza d’onda. Ma è anche il caso in cui la distanza tra l’apertura e il fondo è pari a 3/4, 5/4, 7/4 ecc. delle lunghezze d’onda.

Oltre alla tonica, quindi, si formano anche in misura più o meno forte tutte le armoniche dispari della serie di note armonica.

Il fatto che ogni strumento musicale abbia un suo colore di suono caratteristico va attribuito (e si deve) solo al verificarsi di armoniche superiori più o meno forti.

16. Misurazione della lunghezza d’onda

• Chiudere l’estremità del tubo di vetro lungo

esattamente 45 cm con la membrana di

gomma e, mantenendolo inclinato, con un

cucchiaino da tè versare nel tubo una piccola

quantità di polvere di licopodio in modo che

nel tubo si formi una sottile fascia gialla

uniformemente distribuita.

• Fissare il tubo in vetro al tavolo di lavoro

tramite un morsetto di supporto, un morsetto

da tavolo e un blocco in plastica.

• Battere con molta forza il diapason (1700 Hz)

sul manico di un martello e tenere un rebbio

sul fianco proprio davanti all’apertura del tubo.

Eventualmente ripetere più volte l’operazione! La polvere di licopodio oscilla intensamente in

corrispondenza dei ventri di oscillazione, mentre nei nodi resta completamente ferma. Le particelle di polvere cadono sul fondo del tubo formandovi cumuli periodici che si ripetono 4 volte e ½ lungo l’asse del tubo.

Spiegazione: il diapason in metallo leggero ha una frequenza di 1700 oscillazioni al secondo. In base alla facile relazione

ondalunghezzad ='

lsuonovelocitàde

frequenza

s/m

⋅

340

⋅

1700

la relativa lunghezza d’onda è pari a 20 cm. In un tubo lungo 45 cm, quindi, "ci stanno" 4 semionde e ½ o 2 lunghezze d’onda piene e una lunghezza di quarto d’onda, come ha mostrato l’esperimento. In corrispondenza dell’apertura del tubo c’è sempre un ventre, sul fondo sempre un nodo.

⋅=

Page 30

17. Il fondo di risonanza

• Battere con forza il diapason la’ = 440 Hertz

con il martelletto del metallofono e posizionarlo con l’asta sul piano del tavolo.

Il suono del diapason, udibile a malapena in aria, viene talmente intensificato quando lo si colloca sul tavolo da risultare chiaramente percepibile in tutta la stanza.

Spiegazione: oscillando su e giù, l’asta del diapason fa entrare in oscillazione il piano del tavolo. Poiché la superficie efficace del tavolo è molto maggiore di quella del diapason, il volume del suono è sensibilmente amplificato.

18. La cassa di risonanza

• Percuotere con forza il diapason la' = 440

Hertz e collocarlo con l’asta sulla cassa di risonanza del monocordo.

Si verifica una significativa amplificazione del suono.

Spiegazione: come per l’esperimento 17.

19. Il risuonatore sferico

• Avvicinare uno dopo l’altro i risuonatori di

Helmholtz all’orecchio dalla parte dell’estremità più piccola.

Si percepisce una nota più bassa al crescere del diametro del risuonatore.

Spiegazione: ogni cavità avente la medesima struttura (tubo, sfera cava) possiede una prima armonica esattamente definita, quasi priva di armoniche superiori. La prima armonica può essere eccitata soffiando nell’apertura della cavità oppure anche solo picchiando con una nocca contro la cavità stessa. Ma l’oscillazione propria viene eccitata anche se il rumore circostante contiene suoni corrispondenti alla prima armonica del risuonatore. Il risuonatore sferico consente quindi di verificare il contenuto di suoni parziali in una combinazione di suoni. Se in una stanza c’è silenzio assoluto, il risuonatore resta silenzioso.

20. Gli strumenti a corda e le loro leggi

• Spostare l’asta trasversale di taglio sotto la fune

del monocordo in modo che il bordo destro coincida esattamente con il numero 20 della scala di misura e la corda lunga 40 cm risulti suddivisa in due sezioni uguali lunghe 20 cm ciascuna.

• Accordare la mezza lunghezza della corda sul

diapason (440 Hz) la’ (la normale) serrando il cavicchio.

• Pizzicando o, meglio, sfregando la corda

confrontare l’altezza del suono a 40 cm, 20 cm, 10 cm e 5 cm.

Con la corda lunga 20 cm si ottiene il la normale la’ = 440 Hertz, con la corda lunga 40 cm la nota più bassa di un’ottava la = 220 Hertz, con la corda lunga 10 cm la nota più alta di un’ottava la’’ = 880 Hertz e con la corda lunga 5 cm la nota più alta di due ottave la’’’ = 1760 Hertz.

Spiegazione: con la corda di lunghezza doppia si ottiene una nota più bassa di un’ottava, con la corda lunga la metà la prima ottava e con la corda lunga 1/4 la seconda ottava. Le frequenze della corda hanno un comportamento opposto rispetto alle lunghezze.

21. Le scale sugli strumenti a corda

• Spostando l’asta trasversale, suonare sul

monocordo la scala impressa nell’orecchio

umano e determinare le lunghezze della parte

di corda che oscilla e il rapporto tra parte

oscillante e lunghezza totale della corda (40 cm).

Nota Lunghezza

della corda

Rapporto tra le

lunghezze

do 40 cm 1

re 35,55 cm 8/9

mi 32 cm 4/5

fa 30 cm 3/4

sol 26,66 cm 2/3

la 24 cm 3/5

si 21,33 cm 8/15

do’ 20 cm 1/2

Spiegazione: a parità di condizioni quali tensione e spessore, per ottenere l'ottava la corda deve essere lunga la metà. Con le altre note della scala per il rapporto tra le parti oscillanti e la lunghezza totale della corda si ottengono indici molto semplici. Più piccoli sono gli indici, migliore è l’armonia. (ottava 1:2, quinta do/sol 2:3 ecc.)

22. Misurazione della tensione della corda

• Inserire la bilancia a molla nel monocordo e

agganciare l’estremità della corda in perlon

alla fessura della bilancia a molla.

• Serrando il cavicchio accordare la

corda sul la normale utilizzando il

diapason la’ = 440 Hertz.

• Determinare la tensione della corda con la

bilancia a molla. La tensione della corda in perlon è pari a 5,5 kg.

23. Dipendenza dell’altezza della nota dalla

tensione della corda

L’22 aveva mostrato che per ottenere il la normale la corda in perlon deve presentare una tensione di 5,5 kg.

Page 31

Qual è la tensione della corda della nota la (220 Hertz) più bassa di un’ottava?

• Allentare il cavicchio fino a ottenere la nota la.

• Per controllo, posizionare l’asta trasversale sul

valore 20 (mezza lunghezza della corda) e accordare nuovamente la mezza lunghezza della corda sul la normale. Tutta la corda

oscilla con una frequenza dimezzata. La tensione della corda diminuisce a 1,4 kg. Spiegazione: la frequenza di una corda è

proporzionale alla radice quadrata del peso che la tende. Se la forza che tende la corda aumenta di 4, 9 o 16 volte, la frequenza risulta doppia, tripla o quadrupla. 1/4 di 5,5 è (circa) 1,4, come la misura effettuata.

24. Gli strumenti a fiato e le loro leggi

• Suonare con la bocca la canna labiale e

variarne la lunghezza efficace del piffero

estraendo più o meno il fondo. Le lunghezze minori della canna producono note

alte, quelle maggiori note più basse. Spiegazione: soffiando un debole flusso d’aria si

formano onde stazionarie e la lunghezza della canna corrisponde a una lunghezza di quarto d’onda. Soffiando un flusso d’aria più intenso si producono armoniche superiori, la cui frequenza è un multiplo dispari della tonica.

Con la canna aperta la prima armonica è alta quanto l’armonica con la canna chiusa.

25. La scala di do maggiore e i suoi intervalli

• Per determinare gli intervalli si divide la

frequenza superiore per quella subito inferiore. Per l’intervallo re/do = 1188/1056 il divisore

comune è 132, quindi si ottiene 9/8, 10/9, 16/15, 9/8, 10/9, 9/8 e 16/15.

Spiegazione: gli intervalli delle singole note della scala non hanno la stessa grandezza. Si distingue tra note intere maggiori (9/8), note intere minori (10/9) e mezze note (16/15).

26. Armonia e dissonanza

• Eseguire i diversi accordi con la canna ad ancia.

Si ottengono armonie particolari (consonanze) con l’ottava, la quinta, la quarta, la terza maggiore e minore. Sono dissonanze la seconda e la settima e gli accordi di note immediatamente successive.

27. L’accordo di terza di sol maggiore

• Suonare contemporaneamente le note sol e re

con la canna ad ancia. Si percepisce un accordo particolarmente armonico,

chiamato accordo di terza di sol maggiore.

Spiegazione: per costituire un accordo armonico, una consonanza, più note devono farlo a due a due. L’accordo di terza di sol maggiore è composto dalla terza maggiore e dalla terza minore. Le frequenze delle note sol, si e re sono legate da un rapporto particolarmente semplice, ovvero 4:5:6.

Per ottenere questo rapporto numerico le frequenze fondamentali indicate nella canna ad ancia devono essere divise per 6. (Per ottenere dal punto di vista matematico la corretta frequenza, le frequenze fondamentali stampate devono essere moltiplicate per 33.)

Tra la canna ad ancia e il metallofono è possibile percepire una deviazione dell’accordatura intrinseca alla produzione.

28. L’accordo di terza di sol maggiore a quattro voci

• Integrare l’accordo di terza di sol maggiore

con l’ottava sol', ovvero suonare contemporaneamente sol, si, re’ e sol’.

Si ottiene un accordo particolarmente pieno e armonico: l’“accordo di terza di sol maggiore a quattro voci”.

Spiegazione: l’accordo di terza a quattro voci contiene le seguenti consonanze:

l’ottava 1:2 la quinta 2:3 la terza maggiore 4:5 la terza minore 5:6

29. La scala maggiore con qualsiasi tonica

• Suonare sul metallofono prima la scala di do

maggiore iniziando col do, quindi la scala di sol maggiore iniziando col sol.

La scala di do maggiore da do' a do" produce un suono puro. Nella scala di sol maggiore che inizia col sol' si verifica un errore grave in corrispondenza del fa". La nota è troppo bassa di mezzo tono.

Spiegazione: in base all’esperimento 25, per ogni scala devono presentarsi i seguenti intervalli:

9/8, 10/9 16/15, 9/8, 10/9, 9/8, 16/15. Ma nella sequenza sol'...sol" sulla piastra di base

del metallofono ci sono i seguenti intervalli: 10/9, 9/8, 16/15

, 9/8, 10/9, 16/15, 9/8

Gli intervalli sottolineati sono corretti, gli altri sono più o meno errati.

Gli intervalli 9/8 e 10/9 però sono così vicini da essere difficilmente distinguibili l'uno dall'altro, quindi l'errore da sol' a si' è irrilevante. Al contrario, l’errore tra mi" e fa" è grave: l'intervallo è pari a 16/15, mentre in realtà dovrebbe essere 9/8. Quindi si sente un fa" più basso di mezzo tono.

Page 32

30. Inserimento dei mezzi toni

• Con la canna ad ancia suonare la scala da sol' a

sol" dopo essersi assicurati che la nota la' sia effettivamente accordata sul la normale. A tal fine percuotere il diapason e confrontare le note.

La canna ad ancia produce una scala di sol maggiore assolutamente pura.

Spiegazione: al posto della nota fa' si è inserita una nuova nota, il fa diesis', calcolata in questo modo: l’intervallo tra mi’ e fa diesis’ è 9/8 e l’intervallo tra fa diesis’’ e sol’’ è 16/15. Ciò avviene moltiplicando per 25/24 la frequenza del fa.

Le nuove note ottenute aumentando le vecchie si chiamano do diesis, re diesis, mi diesis, fa diesis, sol diesis, la diesis, si diesis.

Nella notazione, l’aumento si indica anteponendo il simbolo

Le note più basse di mezzo tono si ottengono moltiplicando la nota più alta per 24/25. Nella notazione sono indicate anteponendo il simbolo b e si chiamano do bemolle, re bemolle, mi bemolle, fa bemolle, sol bemolle, la bemolle e si bemolle.

Con pochi errori sul pianoforte le note do diesis e re bemolle ecc. sono unificate.

Elwe Didactic GmbH • Steinfelsstr. 6 • 08248 Klingenthal • Germania • www.elwedidactic.com

3B Scientific GmbH • Rudorffweg 8 • 21031 Amburgo • Germania • www.3bscientific.com

Con riserva di modifiche tecniche

Page 33

3B SCIENTIFIC

Aparato didáctico Acústica U8440012

Instrucciones de uso

05/09 ELWE/ALF

PHYSICS

1. Descripción

El juego de aparatos didácticos de acústica hace posible la enseñanza del campo temático “Acústica“ como una unidad completa cerrada. Con este juego de aparatos es posible realizar una amplia variedad de experimentos.

Ejemplos de experimentos:

1. Tonos de cuerdas

2. El tono acústico puro

3. Columnas de aire vibrantes

4. La columna de aire abierta

5. Flauta labial

6. Varillas vibrantes

7. Infrasonido

8. Ultrasonido

9. El diapasón de registro

10. Ondas progresivas

11. El efecto Doppler

12. Figuras de Chladni

13. Oscilaciones de campana

14. Ondas estacionarias

15. Tonos armónicos

16. Medición de la longitud de onda

17. Fondo de resonancia

18. La caja de resonancia

19. El resonador volumétrico

20. Los Instrumentos de cuerdas y sus leyes

21. Las escala musical de los instrumentos de

cuerda

22. Medición de la tensión de la cuerda

23. La dependencia de l’altura del tono con la

tensión de la cuerda

24. Instrumentos de viento y sus leyes

25. La escala musical Do-Mayor y sus intervalos

26. La consonancia y disonancia

27. El tritonio G mayor

28. El tritono G mayor de cuatro voces

29. La escala musical mayor con tono fundamental

arbitrario

30. La introducción de tonos medios

La entrega se hace en un panel de plástico con inserto de gomaespuma para el almacenamiento cuidadoso de las componentes.

Page 34

2. Volumen de suministro

1 Panel con inserto de gomaespuma para

aparato didáctico „Acústica“

2 Monocordio 3 Puente para monocordio 4 Marimba 5 Placa de Chladni 6 Diapasón, 1700 Hz 7 Diapasón, 440 Hz 8 Diapasón de registro, 21 Hz 9 Balanza de muelle 10 Abrazadera soporte 11 Pinza de mesa 12 Resonador de Helmholtz Ø 70 mm Ø 52 mm Ø 40 mm Ø 34 mm 13 Tubo de vidrio para columna de aire abierta 14 Tubo de Kundt 15 Tubo de vidrio para columna de aire cerrada

16 Mango para placa de Chladni/ Copa vibradora 17 Silbato e Galton 18 Lápiz registrador 19 Polvo de licopodio

20 Bloque de plástico para la pinza de mesa

21 Tapa de goma 22 Copa vibradora 23 Flauta de lengüeta 24 Flauta labial 25 Cuerda de acero 26 Cuerda de perlón 27 Cuerda de ondas 28 Barra deslizables de sintonización

Page 35

3. Datos técnicos

Dimensiones: aprox.530 x 375 x 155

Masa: aprox. 4,5 kg

4. Ejemplos de experimentos

1. Tonos de cuerdas

• Se tira fuertemente con el dedo de la cuerda

del monocordio levemente tensa.

• Girando la clavija hacia la derecha se aumenta

la tensión de la cuerda y al mismo tiempo se

tira de ella con el dedo. Se escucha al principio un tono bajo y a

continuación uno más alto. Explicación: Cuerdas vibrantes producen tonos

acústicos por medio de compresión de descompresión del aire a su alrededor. Mientras mayor sea la tensión de la cuerda más rápida será la vibración y por lo tanto más alto será el tono generado.

2. El tono acústico puro

• Se golpea fuertemente el diapasón (440 Hz)

con el martillo de tocar la marimba. Se escucha un tono acústico puro de una altura

determinada constante que se extingue lentamente.

Explicación: El diapasón se compone de una pieza de acero doblada en forma de U, que en la curva pasa a un mango. Como el diapasón sólo puede vibrar en una sola frecuencia de vibración, se produce un tono puro de altura constante (movimientos de las horquillas contrarios entre sí, de afuera hacia dentro y viceversa). Debido al tono constante del diapasón, se usa este normalmente para afinar instrumentos musicales

3. Columnas de aire vibrantes

• El tubo de vidrio para columnas de aire

cerradas se fija en la mesa de trabajo

utilizando la pinza de mesa, el bloque de

plástico y la abrazadera soporte

• Se introduce el deslizador de sintonia en el

tubo de vidrio.

• Se golpea fuertemente el diapasón (400 Hz)

con el martillo de tocar la marimba. Sacando

fuertemente más o menos el deslizador de

sintonía, se varía la longitud de la “la columna

de aire encerrada“. Sólo en una posición del deslizador de sintonia

entra la columna de aire en oscilación de simpatía fuerte (resonancia), en cualquier otra posición permanece muda. La resonancia se puede percibir por el fuerte aumento del volumen sonoro.

Explicación: Las columnas de aire cerradas pueden entrar en resonancia cuando su longitud corresponde a un cuarto de la longitud de onda excitante. Cuando el diapasón vibra con 440 oscilaciones en un segundo

npropagació_de_Velocidad

440

34000

⋅

onda_de_Longitud =

⋅

s/cm

s/esOscilacion

277

⋅=

cm,

Frecuencia

la longitud de onda del tono producido es 77,2 cm. Un cuarto de longitud de onda es de 19,3 cm.

La distancia del émbolo desde la apertura del tubo es de 19,3 cm en caso de la resonancia.

4. La columna de aire abierta

• Se realiza el experimento con el tubo de vidrio

(14), con la columna de aire abierta.

La columna de aire abierta de una longitud igual al doble de la longitud de la columna de aire cerrada entra en resonancia al acercar un diapasón, lo cual se percibe por el fuerte aumento del volumen sonoro.

Explicación: Columnas de aire abiertas pueden entrar en resonancia cuando su longitud corresponde a la mitad de la longitud de onda o a un múltiplo de ellas. En los extremos de la columna abierta siempre se tiene una amplitud máxima y en el centro un nodo de oscilación.

5. La flauta labial

• Se sopla la flauta labial y sacando el émbolo se

cambia la longitud de la flauta..

Dependiendo de la longitud de la flauta se percibe un tono mayor o menor que el timbre característico.

Explicación: Al soplar en la flauta una corriente de aire constante, el aire encerrado en la boquilla entra en vibración originando una secuencia de remolinos de vientos regulares en el filo del labio. El tono que se origina depende da la longitud de la columna de aire. En la flauta cerrada, en el tono fundamental, la longitud de la misma corresponde a un cuarto de longitud de onda (medido desde el fondo hasta el filo del labio). En el labio se origina un máximo de amplitud mientras en el fondo un nodo.

6. Varillas vibradoras

• Unas de las barristas metálicas de la marimba

(22) se golpean con el martillo entregado..

Al golpear las barristas metálicas se escuchan tonos agradables de un timbre característico determinado. Mientras más corta sea la barrita metálica más alto es el tono.

Explicación: Barras elásticas se convierten en sistemas capaces de oscilar cuando descansan en

Page 36

sus nudos de oscilación (aprox. al 22% de la longitud total, desde uno de los extremos).

7. Infrasolido

• El diapasón de registro se deja vibrar

presionando lateralmente al mismo tiempo

ambos lados de la horquilla y dejándolas libres

al mismo tiempo.. El diapasón produce una vibración lenta

observable bien a simple vista. Si se acerca este al oído de percibe un tono bajo y levemente auditivo. Explicación: Las horquillas del diapasón oscilan una contra la otra y producen compresiones y descompresiones en el aire alrededor. Si las mismas llegan a oído, se produce una resonancia del tímpano. Se alcanza a escuchar un tono. El diapasón vibra con una 20 vibraciones en un segundo. El tono más bajo apenas perceptible al oído es de 16 vibraciones en un segundo. Vibraciones por debajo de los 16 Hercios ya no son audibles Estas se denominan bajo el nombre de infrasonido.

8. Ultrasonido

• Se sopla en el silbato de Galton.

No se percibe ningún tono claro sino un ruido en forma de silbido.

Resultado: Debido a su corta longitud el silbato de Galton produce unos tonos muy altos, los cuales no son audibles para los humanos. Estos se denominan ultrasonidos.

9. El diapasón de registro

• Se fijan en la horquilla del diapasón de registro

un lápiz de escritura.

• Se aprietan lateralmente las horquillas del

diapasón para que entren en vibración y al

mismo tiempo se desplaza uniformemente el

lápiz de escritura sobre una hoja de papel

colocado sobre una base no muy blanda. El lápiz de escritura describe sobre el papel una

línea en forma de onda de longitud de onda constante pero con amplitud decreciente. Explicación: El sonido se origina por oscilaciones periódicas de cuerpos rígidos, líquidos o gaseosos. El lugar de las partículas oscilantes del cuerpo se mueve sobre una línea ondulada en dependencia con el tiempo (línea senoidal). Si se golpea una sola vez los cuerpos oscilantes realizan una oscilación „amortiguada“ (disminución constante de la amplitud). Si la entrada de energía es constante (tono permanente de una bocina de automóvil, sople constante de una flauta de órgano), se obtiene una oscilación no amortiguada de amplitud constante (sonoridad).

10. Ondas progresivas

• Haciendo un nudo sencillo se fija el lazo de la

cuerda ondas en el picaporte de una puerta.

• Se tensa la cuerda levemente y se ejecuta en

ella con la mano un movimiento lateral de vaivén brusco.

Partiendo del centro del movimiento (la mano) se expande una ola (un pulso), que se mueve a lo largo de la cuerda con una velocidad pe propagación determinada, se refleja en el extremo y retorna al punto inicial. Explicación: Todo cuerpo rígido, líquido o gaseoso, produce vibraciones en caso de una sacudida repentina, las cuales se expenden con una velocidad determinada en el medio oscilante.

11. Efecto Doppler

• El diapasón de metal liviano (1700 Hz) se

golpea fuertemente con el martillo de tocar la marimba y luego se mantiene fijo por un tiempo y después se hacen con él movimientos de vaivén.

En el estado de reposo el diapasón produce un tono fuerte de altura constante. Al mover el diapasón en vaivén la altura del tono cambia constantemente. Si el movimiento es de acercamiento al oído, el tono del sonido aumenta y al alejarse el tono baja. Explicación: Al reducirse la distancia de la fuente de sonido al oído se reducen así los intervalos de tiempo entre dos compresiones, porque la segunda compresión tiene que recorrer una distancia más corta hasta el oído. El oído percibe una frecuencia más alta. El tono se hace mayor. Al retirarse la fuente de sonido del oído los intervalos entre las compresiones y las descompresiones se hace mayor y por ello el tono se hace más bajo.

12. Figuras de Chladni

• La placa de Chladni se fija en la mesa de

trabajo por medio de la pinza de mesa y el bloque de plástico. La placa se cubre de arena fina hasta que se tenga una capa fina en una tercera parte de la placa.

• La placa se roza con un arco de violín, bien

cubierto de colofonio, exactamente en el centro entre dos esquinas tocando levemente una de las esquins con un dedo de la otra mano.

• Se roza la placa varias veces fuertemente hasta

que ésta entre en una vibración intensa bien audible.

Al rozar la placa se percibe un tono acústico bien determinado. Los granos de arena entran en una resonancia fuerte y bailan en determinados lugares de la placa formando una figura peculiar sobre la superficie de la placa.

Page 37

Explicación: Sobre la placa se originas „ondas estacionarias“. La placa no vibra hacia arriba y abajo como un todo al ser rozada con el arco sino que lo hace en determinadas posiciones (en los vientres o amplitudes máximas) mientras que en otros permanece en reposo (nodos de oscilación). Al tocar la placa en una esquina se ha forzado un nodo en este punto.

13. Oscilaciones de campana

• La campana se fija en la mesa de trabajo con

la apertura hacia arriba, utilizando la pinza de

mesa y el bloque de plástico.

• El borde de la campana se golpea con el

martillo en diferentes posiciones (también se

puede rozar con un arco de violín) La altura del tono depende de la posición en que

se golpee. Es muy fácil obtener diferencias de hasta un tono completo. Si la campana de golpea en determinadas posiciones se pueden excitar ambos tonos y así se obtienen las conocidas "pulsaciones“ (aumentos y disminuciones periódicas de la altura del sonido en una secuencia más o menos rápida).

Explicación: Las campanas se pueden considerar como placas oscilantes deformadas. Los armónicos superiores no son armónicos con respecto al tono fundamental. Las campanas también se dividen en secciones oscilantes separadas por las líneas de nodos.

14. Ondas estacionarias

• Se fija con un nudo sencillo el lazo de la

cuerda de ondas en el picaporte de una

puerta.

• La cuerda se debe tensar levemente y se hacen

movimientos circulares lentos con la mano.

• Luego se hace más tensa la cuerda y los

movimientos circulares se hacen más rápido. Con el movimiento lento se origina un nodo en los

extremos de la cuerda y en el centro un vientre. Con un movimiento más rápido se obtienen 3 nodos y 2 vientres y con un movimiento más rápido de tienen 4 nodos y 3 vientres.

Explicación: Por reflexión en el picaporte de la puerta se originan ondas estacionarias. Por la inercia del ojo se observan al mismo tiempo onda original y la reflejada.

En la oscilación fundamental la cuerda oscila en toda su longitud en forma de media longitud de onda. En el centro un vientre y en los extremos nodos. En el primer armónico superior (octava) la cuerda oscila como una longitud de onda completa (2 vientres y 4 nodos). En el segundo armónico superior se tiene 3 vientres y 4 nodos y así sucesivamente.

15. Armónicos superiores

• Se sopla con la boca en la flauta labial,

primero débilmente y luego fuertemente.

Se percibe primero en tono fundamental y al soplar más fuertemente se escucha un tono mucho más alto.

Explicación: En la flauta cerrada las ondas estacionarias se deben formar de tal forma que en el fondo siempre se tenga un nodo y en el filo del labio un vientre. Este es el caso cuando la longitud de la flauta es ¼ de la longitud de onda. El mismo caso se tiene cuando la distancia entre la apertura y el fondo 3/4 5/4, 7/4 de la longitud de onda.

Junto al tono fundamental se generan también los armónicos superiores impares de la escala musical, más o menos con la misma intensidad..

Sólo a la aparición más o menos fuerte de los armónicos superiores se le atribuye (y agradece) el timbre muy característico de cada instrumento musical.

16. Medición de la longitud de onda

• El extremo del tubo de vidrio (21) de una

longitud exacta de 45 cm se cierra con una tapa de goma. Se toma una cantidad de polvo de licopodio en una cucharilla de té y manteniendo el tubo inclinado se llena este de tal forma que a lo largo del tubo se reparta el licopodio regularmente.

• El tubo de vidrio se fija en la mesa de trabajo

por medio de la abrazadera de soporte, la pinza de mesa y el bloque de plástico.

• El diapasón (10) se golpea fuertemente contra

un mango de martillo y una de las horquillas se coloca en su parte ancha frente a la apertura del tubo. Esta excitación del sonido se deberá posiblemente repetir varias veces.

En los vientres de la oscilación el polvo de licopodio entra fuertemente en resonancia; mientras que en los nodos permanece en reposo. Las partículas del polvo se reparten a lo largo del tubo en acumulaciones periódicas que repiten en el eje del tubo en 4 / ½ veces..

Explicación: El diapasón de metal liviano tiene una frecuencia de 1700 oscilaciones por segundo. De acuerdo con la relación sencilla:

sonido_del_Velocidad

340

1700

onda_de_Longitud =

s/m

⋅

⋅=

Frecuencia

La correspondiente longitud de onda es de 20 cm. En un tubo de 45 cm de longitud caben 41/2 semiondas o dos ondas completas y un cuarto de onda, como se puede ver en el experimento. En la apertura del tubo siempre se tiene un vientre y en el fondo un nodo.

Page 38

17. Fondo de resonancia

• Se golpea fuertemente el diapasón a’ = 440 Hz

con el martillo de tocar la marimba y el

extremo de su mango se pone en contacto

vertical con el tablero de la mesa. El tono del diapasón poco perceptible en el aire

libre se intensifica al ponerlo en contacto con el tablero de la mesa, así que es posible escucharlo claramente en todo el espacio

Explicación: Por las oscilaciones del mango del diapasón el tablero de la mesa entra en resonancia. Como la superficie activa de la mesa es mucho mayor que la del diapasón, la altura de sonido es amplificada considerablemente.

18. Caja de resonancia

• El diapasón de “la’

= 440” Hercios se golpea fuertemente y su mango se pone en contacto con la caja de resonancia del monocordio.

Se percibe una fuerte amplificación del tono. Explicación: Ver experimento 17.

19. Resonador volumétrico

• La punta de cada uno de los resonadores de

Helmholtz se acercan una tras la otro al oído.

Se percibe un tono que es menor mientras mayor sea el diámetro del resonador.

Explicación: Cada cavidad (tubo, esfera hueca), independientemente de cómo se sostenga, tiene una frecuencia fundamental casi libre de armónicos superiores. Esta oscilación fundamental se puede excitar cuando se sopla sobre la apertura de la cavidad o cuando se golpea con un dedo sobre la superficie de la cavidad. La oscilación propia se excita principalmente cuando el ruido del ambiente lleva tonos que concuerdan con la oscilación propia del resonador. Así que con el resonador esférico o volumétrico es posible analizar una mezcla de tonos y observar si se tiene tonos parciales. Si el espacio está totalmente en silencio, el resonador permanece mudo.

20. Los instrumentos de cuerdas y sus leyes

• El puente se enclava de pie bajo la cuerda el

monocordio de tal forma que el borde se encuentre en el número 20 de la escala de medida y la cuerda de 40 cm se encuentre dividida en dos partes iguales de 20 cm..

• Apretando la clavija se afina la longitud media

de la cuerda hasta la frecuencia del diapasón

“la

” (diapasón normal).

• Tirando o mejor rozan la cuerda se comparan

las alturas de tono para 40 cm, 20 cm, 10 cm y 5 cm de longitud de cuerda.

Con una longitud de cuerda de 20 cm se obtiene la frecuencia del diapasón normal la

= 440 Hz, con

40 cm de longitud de cuerda se obtiene una octava más baja fa = 220 Hz, con 10 cm un tono de una octava más alta la dos octavas más altas, el tono la

= 880 Hz y con 5 cm se tienen

= 1760 Hz.

Explicación: Al doblar la longitud de la cuerda se obtiene un tono una octava más baja, para la mitad de la longitud de la cuerda se obtiene la 1ª octava para ¼ de cuerda la 2ª. Octava. Las frecuencias de las cuerdas se comportan inversamente proporcional a sus longitudes.

21. La escala musical en los instrumentos de cuerdas

• Cambiando la posición del puente se toca en el

monocordio la escala musical acostumbrada a ser oída por las personas y se determina cada vez la longitud de la cuerda oscilante y la relación entre ella y la longitud total de la cuerda de 40 cm.

Tono Longitud de

cuerda

Relación de

longitudes

do 40 cm 1

re 35,55 cm 8/9

mi 32 cm 4/5

fa 30 cm 3/4

sol 26,66 cm 2/3

la 24 cm 3/5

si 21,33 cm 8/15

do1 20 cm 1/2

Explicación: La longitud de la cuerda debe ser la mitad cuando se ha de lograr la octava manteniendo las otras condiciones iguales, es decir: la tensión de la cuerda, el espesor etc. Para los otros tonos de la escala musical se obtiene que la relación de las cuerdas oscilantes con respecto a la longitud total se comporta como una relación sencilla de números enteros. Mientras menores son los números, mejor es el timbre (Octava 1:2, Quinta do/sol 2:3 etc.).

22. Medición de la tensión de la cuerda

• Se fija en el monocordio la balanza de muelle

y se cuelga en la ranura de ella el extremo de la cuerda de perlón.

• Tirando de la clavija se afina la cuerda

al tono de diapasón normal tomando como base el diapasón de la

• Con la balanza de muelle se determina la

’

= 440 Hz.

tensión de las cuerdas.

La tensión de la cuerda de perlón es de 5,5 kg.

Page 39

23. Dependencia de l’altura del tono con la tensión de la cuerda.

Un resultado del experimento 22 es que para obtener el tono de diapasón normal la tensión de la cuerda de perlón debe ser de 5,5 kg. ¿Cuál será la tensión de la cuerda para obtener el tono “la” (220 Hz) en una octava más baja?

• Se afloja la clavija hasta escuchar el tono “la”

• Para controlar se coloca el puente en la

posición 20 de la escala de medida y se afina la mitad de la cuerda al tono de diapasón normal. La cuerda completa vibra con la mitad

de la frecuencia. La tensión de la cuerda se fija en 1,4 kg. Explicación: La frecuencia de una cuerda es

proporcional a la raíz cuadrada de la tensión en la cuerda. Cuando la fuerza de tensión de la cuerda se multiplica por 4, 9, 16, la frecuencia aumenta en 2, 3, 4 veces: ¼ de 5,5 es aprox. 1,4.

24. Instrumentos de viento y sus leyes.

• La flauta labial se sopla con la boca y tirando

del fondo más menos fuertemente se cambia

la longitud efectiva de la flauta. Con longitudes de flautas pequeñas se tienen tonos

altos con largas tonos bajos. Explicación: Al soplar una corriente de aire tenue

se producen ondas estacionarias, siendo la longitud de la flauta un cuarto de la longitud de onda. Al soplar fuertemente se produce una corriente de aire más fuerte dando lugar a la producción de armónicos superiores cuyas frecuencias son múltiplos impares del tono fundamental..

Con la flauta abierta el tono fundamental es el doble del correspondiente con la flauta cerrada.

25. La escala musical Do-mayor y sus intervalos

• Para determinar los intervalos se divide cada

vez la frecuencia mayor por la más baja

contigua. Para el intervalo re/do = 1188/1056 el divisor

común es 132, es decir que se obtiene 9/8, 10/9, 16/15, 9/8, 10/9, 9/8 y 16/15.

Explicación: Los intervalos (espacios intermedios) entre los tonos de la escala musical no son iguales. Se diferencia entre pasos de tonos completos grandes (9/8) pasos de tonos completos pequeños (10/9) y pasos de semitonos (16/15).

26. Consonancia y disonancia

• Se soplan en la flauta lingual los tonos

compuestos más diferentes. Tonos armónicos agradables (consonantes) se

obtienen con la octava, la quinta. La cuarta y con las terceras grandes y pequeña. Tonos disonantes

(disonancias) son la segunda y la séptima y así como tonos compuestos por tonos directamente aledaños..

27. El tritono de Sol-mayor

• Se soplan en la flauta lingual al mismo tiempo

los tonos “sol – mi – re”.

Este se percibe o escucha como un tono compuesto especialmente agradable, el cual se denomina “tritono sol mayor”.

Explicación: Si varios tonos conforman un tono compuesto especialmente agradable al oído, una consonancia, estos se deben tocar de par en par. El tritono “sol mayor” se compone de la tercia pequeña y la tercia grande. Las frecuencias de los tonos sol, si, re están entre sí en una relación numérica muy sencilla 4:5:6.

Para lograr esta relación numérica se deben dividir por 6 las frecuencias fundamentales indicadas en la flauta lingual.

(Para obtener las frecuencias correctas desde un punto de vista físico, las frecuencias básicas impresas se deben multiplicar por 33).

Entre la flauta labial y la marimba puede además ser perceptible una desviación en la concordancia debida posiblemente a condiciones de fabricación.

28. El tritono Sol-mayor a cuatro voces.

• El tritono Sol-mayor se complementa con la

octava sol tiempo “sol, si, do

. Es decir que se tocan al mismo

sol1”.

Se obtiene así “tritono-sol-mayor” muy agradable al oído.

Explicación: En el tritono a cuatro voces se encuentran las siguientes consonancias:

La octava 1:2 La quinta 2:3 La tercera grande 4:5 La tercer pequeña 5:6

29. La escala musical “Mayor” con cualquier tono fundamental

• Se toca en la marimba la escala musical “Do-

mayor” iniciando con “do” y luego la “Solmayor” iniciando con “sol”.

La escala musical “Do-Mayor” de do suena con un tono puro. La escala musical Solmayor que se inicia con sol fuerte en “fa

”. El tono es un tono medio muy bajo.

muestra un error

hasta do2

Explicación: Según el experimento 25 en todas las escalas musicales deben aparecen los siguientes intervalos:

9/8, 10/9 16/15, 9/8, 10/9, 9/8, 16/15.

Page 40

En la placa fundamental de la marimba en la secuencia tónica o escala musical “sol

… sol2” se

tienen los siguientes intervalos: 10/9, 9/8, 16/15

, 9/8, 10/9, 16/15, 9/8

Los intervalos subrayados son correctos, los otros son más o menos falsos.

Los intervalos 9/8 y 10/9 se encuentran muy contiguos, de tal forma que es muy difícil diferenciarlos, por ello el error de sol es importante. Mucho más grave eS el error entre

y fa2. El intervalo aquí es de 16/15, mientras en

la realidad debería de ser 9/8. Por lo tanto fa

hasta si1 no

escucha un tono medio mucho más bajo.

30. Se introducen los pasos de semitonos

• Se toca en la flauta labial la escala musical de

sol

hasta sol2 después de estar seguro de que

el tono la

de la flauta labial se ha afina al diapasón normal. Para ello se golpea el diapasón y se compara.

En la flauta labial se escucha claramente la escala musical de “sol-mayor”.

Explicación: En lugar del tono fa nuevo tono, el fa# forma que el intervalo entre mi de 9/8 y el de fa#

, que se calcula de tal

y sol2 16/15. Esto sucede

se introduce un

y fa#1 sea

aumentado la frecuencia de fa multiplicando por 25/24..

Los nuevos tonos generados por el aumento de los tonos básicos se denominan: do#, re#, mi#, fa#, sol#, la#, si#.

El aumento se indica por medio de una cruz en las notas escritas..

Las notas reducidas en un medio tono se obtienen multiplicando el tono más alto por 24/25. En la escritura de notas se caracterizan por una “b” anticipada a la nota. Estas se llaman: b-do, b-re, bmi, b-fa, b-sol, b-la, b-si.

En el piano se igualan con un pequeño error los tonos do# y b-re.

Elwe Didactic GmbH • Steinfelsstr. 6 • 08248 Klingenthal • Alemania • www.elwedidactic.com

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburgo • Alemania • www.3bscientific.com

Se reservan las modificaciones técnicas

Page 41

3B SCIENTIFIC

Aparelho didático para acústica U8440012

Instruções para o uso

05/09 ELWE/ALF

FÍSICA

1. Descrição

O jogo de aparelhos didáticos para acústica permite uma apresentação de uma visão geral bastante completa da área temática da acústica. Com o aparelho didático podem ser realizadas numerosas experiências.

Exemplos de experiência:

1. Sons de cordas

2. O som acusticamente puro

3. Colunas de ar vibrantes

4. A coluna de ar aberta

5. O apito de boca

6. As varas vibrantes

7. Infra-som

8. Ultrasom

9. O diapasão registrador

10. Ondas em propagação

11. Efeito Doppler

12. Figuras sonoras de Chladni

13. Vibração de sinos

14. Ondas estacionárias

15. Sobretons

16. Medição de longitude de onda

17. O fundo de ressonância

18. A caixa de ressonância

19. O ressonador esférico

20. Os instrumentos de cordas e as suas leis

21. A escala musical nos instrumentos de cordas

22. Medição da tensão das cordas

23. Dependência da altura do tom da tensão das cordas

24. Instrumentos de sopro e suas leis

25. A escala do dó maior e seus intervalos

26. Tom harmônico e tom desarmônico

27. A tríade sol maior

28. A tríade sol maior de quatro vozes

29. A escala maior com tons fundamentais à escolha

30. Associação de semitons

O fornecimento é feito numa placa de plástico com amortecimento de espuma para o transporte seguro de cada parte.

Page 42

2. Fornecimento

1 Tablete com espuma protetora para o aparelho

didático "Acústica" 2 Monocórdio 3 Ponte para Monocórdio 4 Metalofone 5 Placa de Chladni 6 Diapasão, 1700 Hz 7 Diapasão, 440 Hz 8 Diapasão marcador, 21 Hz 9 Balança de mola 10 Pinça suporte 11 Pinça de mesa 12 Ressonador de Helmholtz Ø 70 mm Ø 52 mm Ø 40 mm Ø 34 mm 13 Tubo de vidro para

a coluna de ar aberta

14 Tubo de Kundt 15 Tubo de vidro para

a coluna de ar fechada

16 Vara para placa de Chladni / sino em concha 17 Apito de Galton 18 Caneta com suporte 19 Pó de Licopódio 20 Talho de plástico para a pinça de mesa 21 Capa de borracha 22 Sino em concha 23 Apito de língua 24 Apito de boca 25 Corda de aço 26 Corda de perlon 27 Corda de ondas 28 Ajuste deslizável

Page 43

⋅

3. Dados técnicos

Dimensões: aprox. 530 x 375 x 155 mm3 Massa: aprox. 4,5 kg

4. Exemplos de experiências

1. Tons de cordas

• Puxar com força a corda medianamente tensa

do monocórdio com os dedos.

• Logo, aumentar a tensão da corda girando a

borboleta e puxar numerosas vezes. Primeiro ouve-se um som baixo e logo um tom

mais alto. Explicação: cordas em vibração produzem sons

acústicos por densificação e rarefação alternada do ar ambiente. Quanto maior a tensão da corda, mais rápida é a vibração e mais alto é o tom.

2. O som acusticamente puro

• Bater com força o diapasão (440 Hz) com o

martelinho de tocar do metalofone. Ouve-se um som acusticamente puro com altura

definida e inalterada de tom que se esvanece lentamente.

Explicação: O diapasão consiste num pedaço de metal dobrado em forma de U, no ápice do arco do qual se origina uma vara. Sendo que o diapasão só vibra de uma forma (movimentos contrários das pontas de dentro para fora e inversamente) ele produz um tom puro de altura constante. Por causa da constância da altura tonal do diapasão ele é usado para afinar instrumentos musicais.

3. Colunas de ar vibrantes

• Fixar o tubo de vidro para a coluna de ar

fechada por meio da pinça de mesa, talho de

plástico e pinças de suporte, na mesa de

trabalho.

• Introduzir o ajuste deslizável no tubo de vidro.

• Bater com força o diapasão (440 Hz) com o

martelinho de tocar do metalofone. Alterar o

comprimento da “coluna de ar fechada” por

meio da extração mais ou menos forte do

ajuste deslizável. Somente numa posição do ajuste deslizável a

coluna de ar chega a uma forte oscilação compartilhada (Ressonância), em todas as outras posições ela fica muda. A ressonância é perceptível através de uma superelevação do volume de som.

Explicação: colunas de ar fechadas vibram quando o seu comprimento corresponde à quarta parte do comprimento da onda excitadora. O diapasão vibra a 440 vibrações por segundo. Conforme a relação

Comprimento de onda

34000 cm / s

⋅

440 Oscilação / s

Velocidade de propagação

77,2 cm

⋅

Freqüência

O comprimento de onda do som obtido é de 77,2 cm. Uma quarta parte do comprimento de onda é assim igual a 19,3 cm.

No caso da ressonância, a distância do pistão da apertura do tubo é de 19,3 cm.

4. A coluna de ar aberta

• Executar a experiência com o tubo de vidro

com a coluna de ar aberta.

A coluna de ar com um comprimento de exatamente o dobro da fechada começa a vibrar quando se mantém o diapasão frente à abertura, o que é perceptível através de uma superelevação do volume de som.

Explicação: colunas de ar abertas vibram quando o seu comprimento equivale à metade do comprimento de onda ou à multiplicação da metade do comprimento de onda. Nas pontas da coluna de ar aberta formam-se picos de vibração, no seu meio surgem nós de vibração.

5. O apito de boca

• Soprar no apito de boca e alterar o

comprimento do apito puxando o pistão.

Conforme o comprimento do apito ouve-se um tom mais ou menos baixo com uma coloração tonal característica.

Explicação: ao soprar uma corrente de ar regular na boca do apito, o ar que se encontra preso no apito começa a vibrar, de modo que no lábio formam-se a intervalos regulares rodamoinhos de ar. O tom resultante depende da altura da coluna de ar. No caso do apito fechado o comprimento do apito (medido da boca o fundo) equivale no tom fundamental a um quarto do comprimento de onda. Na boca forma-se um pico e no fundo um nó.

6. Varas vibrantes

• Bater em algumas varas do metalofone com o

martelo incluído no fornecimento.

Ao bater nas varas de metal surgem sons agradáveis com uma coloração tonal característica. Quanto mais curta a vara, mais alto o tom.

Explicação: As varas elásticas se convertem em sistemas capazes de vibrar, quando estão deitados sobre os pontos dos seus nós vibratórios (aproximadamente 22% do comprimento total distanciado das extremidades).

Page 44

7. Infra-som

• Levar o diapasão marcador a vibrar apertando

os dois braços ao mesmo tempo e soltando

repentinamente. O diapasão executa vibrações lentas, fáceis de

observar a olho nu. Se ele for aproximado à orelha, ouve-se um tom muito baixo (apenas perceptível).

Explicação: os braços do diapasão vibram em direções contrárias de um lado para o outro e produzem densificações e rarefações do ar do ambiente. Quando estas atingem o tímpano este é levado a vibrar. Ouve-se um tom.

O diapasão vibra a aproximadamente 20 vibrações por segundo de um lado para o outro. O tom mais baixo, ainda audível comporta aproximadamente 16 oscilações por segundo. Vibrações abaixo de 16 Hertz já não são audíveis. Elas são chamadas de infra-som (do latim infra = abaixo).

8. Ultra-som

• Soprar no apito de Galton.

Já não se ouve tom algum, só um ruído agudo. Resultado: por causa da sua curta longitude de

onda, o apito de Galton produz tons muito altos que já não são audíveis pelo ouvido humano. Estes são chamados de ultra-som (do latim ultra = acima).

9. O diapasão registrador

• Fixar a caneta nos braços do diapasão

marcador.

• Levar o diapasão a vibrar apertando os braços

e deslocá-lo com a caneta sobre uma folha de

papel sobre uma superfície que não seja mole

demais. A caneta desenha linhas em forma de ondas do

mesmo comprimento de onda, mas de amplitude decrescente sobre o papel.

Explicação: o som é produzido pela vibração periódica de corpos sólidos, líquidos ou gasosos. O local geométrico das partículas vibrantes do corpo em relação ao tempo se encontra numa linha ondular (linha de seno). Ao serem batidos uma vez, os corpos em vibração produzem uma vibração "amortecida" (redução constante da amplitude). Se o aporte de energia for constante (som constante de uma buzina, órgão musical soprado constantemente), então se obtém uma vibração não amortecida de mesma amplitude (= volume).

10. Ondas em propagação

• Fixar o laço da corda de ondas numa maçaneta

porta fazendo simplesmente um nó.

• Esticar a corda medianamente e executar um

movimento brusco puxando lateralmente com

a mão.

A partir do centro do movimento (a mão) surge uma onda com velocidade de propagação específica ao longo da corda, que se reflete na ponta fixa e logo volta para o ponto de partida.

Explicação: todo corpo sólido, líquido ou gasoso produz vibrações ao ser sacudido bruscamente, as quais se propagam no meio vibrante com uma velocidade de propagação específica.

11. Efeito Doppler

• Bater firmemente com o diapasão de metal

leve (1700 Hz) com o martelinho de tocar do metalofone, mante-lo brevemente em repouso e logo sacudi-lo no ar rapidamente de um lado para outro.

Em estado de repouso um diapasão produz um som forte de altura tonal constante. Em movimento, a altura do tom altera-se constantemente. Se o som se dirige à orelha, o tom aumenta, se ele se distancia da orelha, o tom abaixa.

Explicação: por causa da distância decrescente da orelha, a distância temporal entre duas densificações é reduzida, já que a segunda densificação tem um caminho mais curto para atingir a orelha do que a primeira. A orelha percebe uma freqüência mais alta. O tom sobe. Ao distanciar-se a fonte de som da orelha, a distância temporal entre densificação e rarefação é prolongada. O tom torna-se mais baixo.

12. Figuras sonoras de Chladni

• Fixar a placa de Chladni na mesa por meio da

pinça de mesa e o talho de plástico. Distribuir areia ou semelhante na placa de modo que esta esteja coberta de uma fina camada na terça parte de sua superfície.

• Friccionar o arco de violino bem preparado

com breu exatamente no meio entre duas pontas enquanto ao mesmo tempo toca-se levemente uma ponta com o dedo da outra mão.

• Friccionar o arco várias vezes firmemente na

placa de modo que ela inicie uma vibração intensa e audível.

Ao friccionar o arco na placa ouve-se um tom acústico bem definido. Os grãos de areia começam a vibrar intensamente em certas partes, dançam pulando sobre a superfície da placa e se acumulam formando estranhas figuras sonoras na superfície.

Explicação: sobre a placa formam-se "ondas estacionárias". Ao ser excitada a placa não oscila para baixo e para cima na sua integridade, ela só vibra em certos pontos (nos picos), enquanto em outros lugares (nós) ela fica em repouso total. Ao tocar uma das pontas da placa foram forçados nós.

Page 45

13. Vibração de sinos

⋅

• Fixar o sino em concha com a apertura para

acima por meio da pinça de mesa e talho de

plástico, na mesa de trabalho.

• Bater na beira do sino com o martelo em

diferentes lugares (alternativamente, passar o

arco). A altura do tom depende do lugar onde foi batido.

É facilmente possível de se obter diferenças de um tom inteiro. Se o sino for excitado em várias partes, então se formam ambos os tons e obtêm-se as conhecidas "flutuações" (aumento e redução periódicos do volume em seqüências mais ou menos rápidas).

Explicação: os sinos são placas vibrantes deformadas. Os tons mais altos geralmente não harmonizam com o tom fundamental. Também os sinos dividem-se em diferentes partes vibrantes separadas.

14. Ondas estacionárias

• Fixar o laço da corda de ondas numa maçaneta

porta fazendo simplesmente um nó.

• Esticar a corda medianamente e executar um

movimento lento e circular.

• Logo esticar mais a corda e acelerar o

movimento em círculos. Com movimento lento formam-se nós em cada

ponta e no meio um pico. Com o movimento mais rápido surgem 3 nós e 2 picos e acelerando-se mais surgem 4 nós e 3 picos.

Explicação: através da reflexão na porta forma-se uma onda estacionária. Por causa da lentidão do olho parece que a onda original e a refletida são simultâneas.

Na vibração básica a corda vibra em todo o seu comprimento na forma de uma meia onda para cima e para baixo. No meio encontra-se o pico, em ambas as pontas estão os nós. No primeiro nível de vibração (oitava) a corda vibra na forma de uma onda completa (2 picos e 3 nós). No segundo nível temos 3 picos e 4 nós, etc.

15. Sobretons

• Soprar no apito e boca com os lábios, primeiro

levemente, logo com muita força. Primeiro ouve-se um tom fundamental e ao soprar

com mais força ouve-se um tom muito mais alto. Explicação: no caso do apito de boca, as ondas

estacionárias devem formar-se de modo que se formem um nó no fundo e um pico no bico. Isto acontece quando o comprimento do apito corresponde exatamente a 1/4 do comprimento de onda. Também é o caso quando a distância da abertura ao fundo é equivalente a 3/4, 5/4, 7/4 e assim por diante do comprimento de onda.

Além do tom fundamental surgem, portanto também diversos sobretons impares da série tonal harmônica em maior ou menor medida.

É só por causa (e graças ao) do surgimento dos sobretons que cada instrumento musical tem ma coloração tonal bem específica.

16. Medição do comprimento de onda

• Tampar a ponta do tubo de vidro de vidro de

exatamente 45 cm com a tampa de borracha e introduzir com uma colher de chá uma pequena quantidade de pó de licopódio no tubo mantido em posição inclinada, de forma que uma quantidade não muito grande de pó se distribua de modo regular para que se forme uma fina tira amarelada no tubo.

• Fixar o tubo de vidro por meio da pinça de

suporte, pinça de mesa e talho de plástico na mesa de trabalho.

• Bater firmemente com o diapasão (1700 Hz) no

cabo de um martelo e manter um dos braços com o lado largo bem perto da abertura do tubo. Repetir essa excitação sonora eventualmente várias vezes!

O pó de licopódio inicia uma forte vibração nos picos de vibração enquanto que nos nós este fica totalmente em repouso. As partículas de pó caem no fundo do tubo e formam acumulações periódicas que se repetem no comprimento do tubo 4 1/2 vezes.

Explicação: o diapasão de metal leve tem uma freqüência de 1700 vibrações por segundo. Conforme a relação simples

Comprimento de onda

340

1700

s/m

⋅=

⋅

Velocidade do som

Freqüência

o comprimento de onda correspondente é de 20 cm. Num tubo de 45 cm de comprimento "cabem", portanto 4½ meias ondas ou 2 completas, como demonstrado na experiência. Na abertura do tubo sempre se encontra um pico, no fundo sempre se encontra um nó.

17. O fundo de ressonância

• Bater fortemente o diapasão a’ = 440 Hertz

com o martelinho de tocar do metalofone e posicionar-lo com o seu cabo sobre a superfície da mesa. O tom do diapasão apenas audível no ar livre é tão amplificado que ele agora é claramente perceptível no espaço inteiro. Explicação: por causa do movimento vibratório da vara do diapasão, o tampo da mesa também começa a vibrar. Sendo que superfície efetiva da mesa é muito maior que o diapasão, o volume do som é ampliado fortemente.

Page 46

18. A caixa de ressonância

• Bater com firmeza com o diapasão de lá' = 440

Hertz no talho de plástico (19) da pinça de

mesa e colocá-lo na caixa de ressonância do

monocórdio com a sua vara. Ocorre um aumento significativo do tom. Explicação: idêntica à experiência 17.

19. O ressonador esférico

• Manter os ressonadores de Helmholtz na

seqüência com ponta pequena perto da orelha. Ouve-se um tom que fica mais baixo conforme

aumenta o diâmetro do ressonador. Explicação: todo corpo oco, seja qual for a sua

forma (tubo, esfera oca) possui uma vibração fundamental bem específica, praticamente livre de sobretons. Esta vibração fundamental pode ser excitada se sopra na abertura do corpo oco ou mesmo só batendo com a articulação do dedo no corpo oco. Porém a vibração própria só é excitada quando se encontram no ruído de ambiente tons que coincidam com a vibração fundamental do ressonador. Assim, pode-se analisar o conteúdo em diferentes semitons de uma mistura de sons. Se no ambiente reina o silêncio total, então o ressonador fica mudo.

20. Os instrumentos de corda e as suas leis

• Inserir a ponte perpendicularmente sob a

corda do monocórdio de modo que a quina

direita coincida exatamente com o número 20

da escala e a corda de 40 cm esteja dividida

em dois segmentos iguais de cada um 20 cm

de comprimento.

• Afinar o meio comprimento da corda

apertando a borboleta no diapasão (440 Hz) lá'

(lá fundamental).

• Comparar os tons originados puxando, ou

melhor, friccionando a corda nos pontos de 40

cm, 20 cm, 10 cm e 5 cm de comprimento. Com 20 cm de comprimento de corda obtém-se o

lá fundamental = 440 Hertz, com 40 cm de comprimento de corda obtém-se o tom mais baixo de uma oitava lá = 220 Hertz, com 10 cm de comprimento de corda obtém-se o tom mais alto de uma oitava lá'’ = 880 Hertz com 5 cm de comprimento de corda obtém-se o tom mais alto de 2 oitavas lá'’’ = 1760 Hertz.

Explicação: com o dobro de comprimento de corda obtém-se um tom mais baixo de uma oitava, com a metade do comprimento de corda obtém-se a primeira oitava e com ¼ do comprimento de corda a segunda oitava. As freqüências das cordas são inversamente proporcionais ao seu comprimento.

21. A escala musical nos instrumentos de corda

• Tocar a escala tonal habitual para o ouvido

humano no monocórdio mudando a ponte de posição e medindo a cada vez o comprimento do segmento de corda em vibração e determinar a relação com o comprimento total da corda (40 cm).

Tom Compr. corda Relação compr.

dó 40 cm 1

ré 35,55 cm 8/9

mi 32 cm 4/5

fá 30 cm 3/4

sol 26,66 cm 2/3

lá 24 cm 3/5

si 21,33 cm 8/15

dó’ 20 cm 1/2

Explicação: a corda deve ter a metade do comprimento quando se queira obter a oitava sob condições idênticas de tensão da corda, espessura da corda, etc. Para os outros tons da escala tonal resultam coeficientes da maior simplicidade para a relação entre o comprimento do segmento em vibração e o comprimento total da corda. Quanto menor são esses números, melhor é o som. (Oitava 1:2, Quinta lá/sol 2:3, etc.)

22. Medição da tensão da corda

• Colocar a balança de mola no monocórdio e

encaixar a ponta da corda de perlon na fenda da balança de mola.

• Afinar a corda no lá fundamental

esticando-a com a borboleta e utilizando o diapasão lá' = 440 Hertz.

• Determinar a tensão da corda com a balança

de mola.

A tensão da corda no caso do perlon é de 5,5 kg.

23. Dependência da altura do tom da tensão das cordas

Um dos resultados da experiência 22 era que para se obter o lá fundamental, a corda de perlon deve ter 5,5 kg de tensão. Qual é a tensão da corda no caso do tom lá (220 Hertz) de uma oitava mais abaixo (220 Hertz)?

• Soltar a borboleta até obter o tom lá.

• Para efeitos de controle colocar a ponte na

marca de 20 (meio comprimento de corda) e afinar o meio comprimento de corda no lá. A corda toda vibra então em meia freqüência.

A tensão da corda cai para 1,4 kg.

Page 47

Explicação: a freqüência de uma corda é proporcional à raiz quadrada do peso da tensão. Quando a força que tensa a corda é 4x, 9x, 16x maior, a freqüência aumenta em 2, 3 e 4 vezes. 1/4 de 5,5 é (aprox.) 1,4, como foi medido.

24. Os instrumentos de sopro e as suas leis

• Soprar no apito de boca com a boca e alterar o

comprimento efetivo do apito movendo mais ou menos o seu fundo.

No caso de pequenos comprimentos de apito, obtêm-se tons altos, no caso de maiores comprimentos obtêm-se tons mais baixos.

Explicação: ao soprar uma corrente de ar fraca formam-se ondas estacionárias, sendo que o comprimento do apito equivale a uma quarta parte do comprimento das ondas. Ao sopra-se uma corrente mais forte surgem sobretons, cuja freqüência é um múltiplo ímpar do tom fundamental.

No caso do apito aberto, a vibração fundamental é duas vezes maior do que no caso do apito fechado.

25. A escala dó maior e os seus intervalos

• Para determinar os intervalos dividem-se cada

freqüência mais alta pela próxima mais baixa.

Para o intervalo ré/dó = 1188/1056, o denominador comum é 132, pelo que se obtém então 9/8, 10/9, 16/15, 9/8, 10/9, 9/8 e 16/15.

Explicação: os intervalos de cada tom da escala tonal não são do mesmo tamanho. Diferenciam-se grandes inteiros (9/8), pequenos inteiros (10/9) e passos de semitons (16/15).

26. Som harmônico e som desarmônico

• Soprar diferentes combinações de sons com o

apito de língua.

Obtêm-se consonâncias claras com a oitava, a quinta e a quarta, e com as terças menor e maior. Dissonantes são a segunda e a sétima, assim como a harmonia de tons imediatamente seguidos.

27. A tríade sol maior

• Soprar simultaneamente os tons sol e ré no

apito de língua.

Ouve-se uma harmonia particularmente consonante, conhecida como a tríade sol maior.

Explicação: caso vários tons formem uma consonância harmônica, estes têm que o fazer em pares. A tríade sol maior é formada pela conjunção duas terças maior e menor. As freqüências dos tons sol, si, ré, encontram-se numa relação particularmente simples, ou seja, 4:5:6.

Para se obter essas relações numéricas, devem-se dividir as freqüências fundamentais indicadas no apito de língua a cada vez por 6.

(Para manter as fisicamente corretas freqüências, as freqüências básicas impressas têm que ser multiplicadas por 33).

Entre o apito de língua e o metalofone dependendo do caso pode-se ouvir, além disso, um desvio da afinação.

28. A tríade sol maior de quatro vozes

• Completar a tríade sol maior de quatro vozes

com a oitava sol'. Ou seja, tocar sol, si, ré', e sol'.

Obtém-se assim o som particularmente cheio e harmonioso da "tríade sol maior a quatro vozes".

Explicação: na tríade a quatro vozes encontram-se as seguintes consonâncias:

A oitava 1:2 A quinta 2:3 A terça maior 4:5 A terça menor 5:6

29. A escala tonal maior com tom aleatoriamente escolhido

• Tocar no metalofone primeiro a escala dó

maior começando com dó, e depois tocar a escala tonal sol maior, começando com sol.

A escala tonal dó maior de dó' a dó'' soa acusticamente pura. Na escala sol maior iniciando com sol' surge um erro grave ao se chegar a fá''. O tom é baixo demais em um semitom.

Explicação: segundo a experiência 25 os seguintes intervalos devem surgir em todas as escalas:

9/8, 10/9 16/15, 9/8, 10/9, 9/8, 16/15. Na seqüência tonal sol’... sol" encontram-se porém

os seguintes intervalos: 10/9, 9/8, 16/15

, 9/8, 10/9, 16/15, 9/8

Os intervalos sublinhados são corretos, os outros mais ou menos errados.

Os intervalos 9/8 e 10/9 estão tão perto um do outro que eles só podem ser diferenciados com dificuldade, é por isso que o erro entre sol' e si' é irrelevante. O erro entre mi" e fá" é bem mais grave. Aqui, intervalo é de 16/15, enquanto que na realidade ele deveria ser de 9/8. Por isso ouve-se o fá" com um semitom abaixo do ideal.

30. Intervenção dos passos de semitons

• Tocar a escala de sol' a sol" no apito de língua,

depois de ter garantido que o tom lá' do apito de boca esteja afinado com o tom fundamental. Para tal, bater com o diapasão e comparar.

A escala sol maior toca em total pureza no apito de língua.

Explicação: em vez do tom fá' associa-se um novo tom, o fá' sustenido, o qual é calculado de modo

Page 48

que o intervalo entre mi’ e fá’ sustenido 9/8 e entre o fá'' sustenido e o sol’’ é de16/15. Isto ocorre porque a freqüência do fá é elevada por multiplicação por 25/24.

Os novos sons que surgem através da elevação dos tons chamam-se: dó sustenido, ré sustenido, mi sustenido, fá sustenido, sol sustenido, lá sustenido, si sustenido.

A elevação é indicada nas partituras por uma cruz na frente.

Os tons mais baixos de um semitom são obtidos pela multiplicação do tom mais alto por 24/25. Estes tons são indicados nas partituras por um b na frente. Eles se chamam: dó bemol, ré bemol, mi bemol, fá bemol, sol bemol, lá bemol, bemol.

No teclado do piano os tons dó bemol e ré bemol, etc., são reproduzidos com mínimo de erro.

Elwe Didactic GmbH • Steinfelsstr. 6 • 08248 Klingenthal • Alemanha • www.elwedidactic.com

3B Scientific GmbH • Rudorffweg 8 • 21031 Hamburgo • Alemanha • www.3bscientific.com

Sob reserva de alterações técnicas

3B Scientific Acoustics Kit User Manual [en, de, fr, it, es]

Specifications and Main Features

Frequently Asked Questions

User Manual