RaneNote linkwtiz riley DATASHEETS
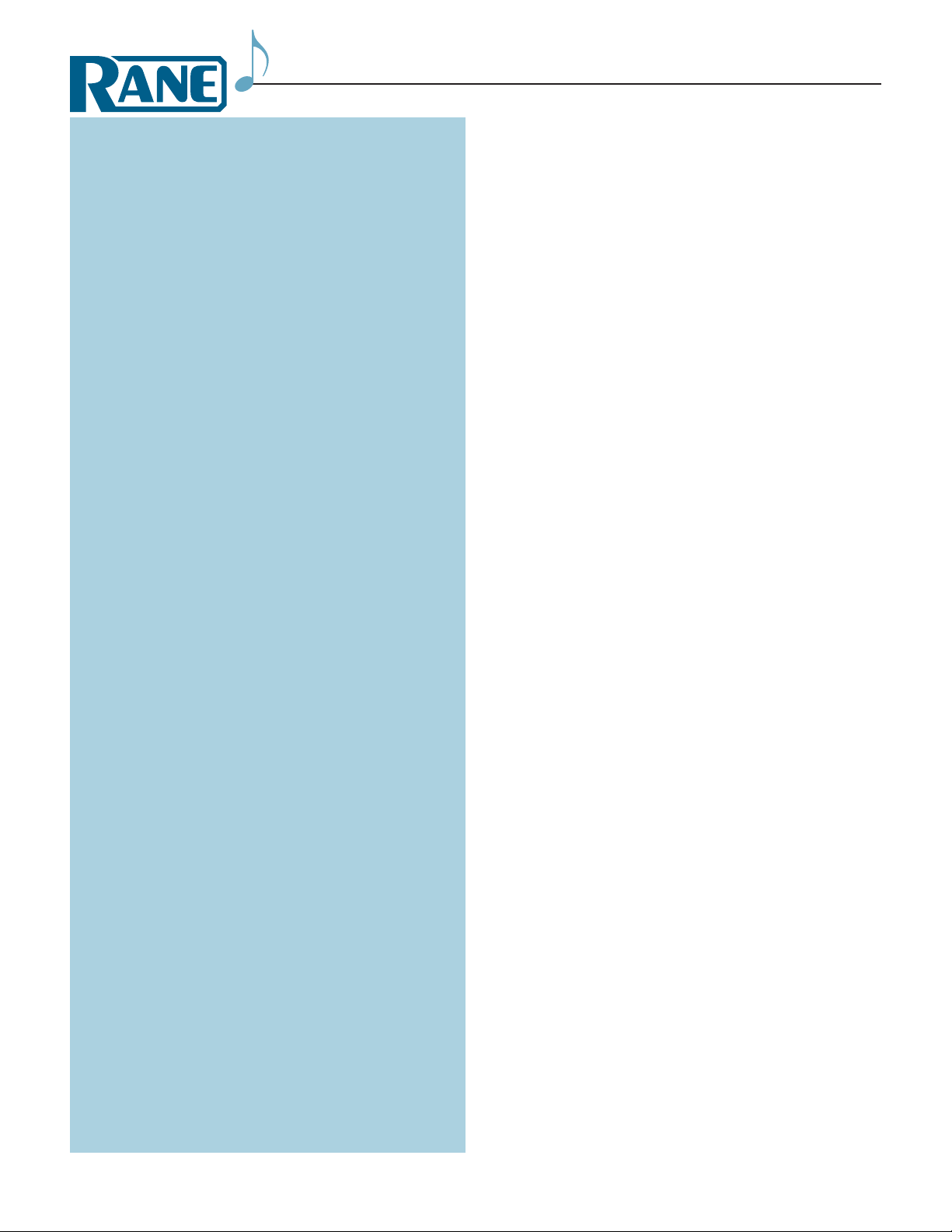
RaneNote
LINKWITZ-RILEY CROSSOVERS: A PRIMER
Linkwitz-Riley Crossovers:
A Primer
• Linkwitz-Riley Background
• 1st-Order Crossover Networks
• Butterworth Crossovers 2nd to 4th-Order
•
Linkwitz-Riley Crossovers
• Phase, Transient & Power Responses
2nd to 8th-Order
Introduction
In 1976, Siegfried Linkwitz published his famous paper
[1] on active crossovers for non-coincident drivers. In
it, he credited Russ Riley (a co-worker and friend) with
contributing the idea that cascaded Butterworth filters
met all Linkwitz’s crossover requirements. eir efforts
became known as the Linkwitz-Riley (LR) crossover
alignment. In 1983, the first commercially available
Linkwitz-Riley active crossovers appeared from Sundholm and Rane.
Today, the de facto standard for professional audio
active crossovers is the 4th-order Linkwitz-Riley (LR-4)
design. Offering in-phase outputs and steep 24 dB/octave slopes, the LR-4 alignment gives users the necessary tool to scale the next step toward the elusive goal
of perfect sound. And many DSP crossovers offer an
8th-order Linkwitz-Riley (LR-8) option.
Before exploring the math and electronics of LR
designs, it is instructional to review just what Linkwitz-Riley alignments are, and how they differ from
traditional Butterworth designs.
Dennis Bohn
Rane Corporation
RaneNote 160
© 2005 Rane Corporation
Linkwitz-Riley-1

Linkwitz-Riley Crossovers: Background
Siegfried Linkwitz and Russ Riley, then two HewlettPackard R&D engineers, wrote the aforementioned paper describing a better mousetrap in crossover design.
Largely ignored (or unread) for several years, it eventually received the attention it deserved. Typical of truly
useful technical papers, it is very straightforward and
unassuming: a product of careful analytical attention
to details, with a wonderfully simple solution.
It is seldom whether to cross over, but rather, how to
cross over. Over the years active crossovers proliferated
at a rate equal to the proverbial lucky charm.
In 1983, a 4th-order state variable active filter [2]
was developed by Rane Corporation to implement the
Linkwitz-Riley alignment for crossover coefficients and
now forms the heart of many analog active crossover
designs.
A Perfect Crossover
Mother Nature gets the blame. Another universe,
another system of physics, and the quest for a perfect
crossover might not be so difficult. But we exist here
and must make the best of what we have. And what we
have is the physics of sound, and of electromagnetic
transformation systems that obey these physics.
A perfect crossover, in essence, is no crossover at
all. It would be one driver that could reproduce all
frequencies equally well. Since we cannot have that,
second best would be multiple speakers, along the same
axis, with sound being emitted from the same point,
i.e., a coaxial speaker that has no time shift between
drivers. is gets closer to being possible, but still is
elusive. ird best, and this is where we really begin,
are multiple drivers mounted one above the other with
no time shift, i.e., non-coincident drivers adjusted
front-to-rear to compensate for their different points of
sound propagation. Each driver would be fed only the
frequencies it is capable of reproducing. e frequency
dividing network would be, in reality, a frequency gate.
It would have no phase shift or time delay. Its amplitude response would be absolutely flat and its roll-off
characteristics would be the proverbial brick wall.
(Brings a tear to your eye, doesn’t it?)
DSP digital technology makes such a crossover possible, but not at analog prices demanded by most working musicians.
Linkwitz-Riley Crossover
What distinguishes the Linkwitz-Riley crossover design from others is its perfect combined radiation pattern of the two drivers at the crossover point. Stanley
P. Lipshitz [3] coined the term “lobing error” to describe this crossover characteristic. It derives from the
examination of the acoustic output plots (at crossover)
of the combined radiation pattern of the two drivers
(see Figures 1 & 2). If it is not perfect the pattern forms
a lobe that exhibits an off-axis frequency dependent tilt
with amplitude peaking.
Interpretation of Figure 1 is not particularly obvious. Let’s back up a minute and add some more details.
For simplicity, only a two way system is being modeled.
e two drivers are mounted along the vertical center
of the enclosure (there is no side-to-side displacement,
i.e., one driver is mounted on top of the other.) All
front-to-back time delay between drivers is corrected.
e figure shown is a polar plot of the sideview, i.e., the
angles are vertical angles.
It is only the vertical displacement sound field that is
at issue here. All of the popular crossover types (constant voltage [4], Butterworth all-pass [5], etc.) are well
behaved along the horizontal on-axis plane. To illustrate the geometry involved here, imagine attaching
a string to the speaker at the mid-point between the
drivers. Position the speaker such that the mid-point is
exactly at ear level. Now pull the string taut and hold
it up to your nose (go on, no one’s looking). e string
should be parallel to the floor. Holding the string tight,
move to the left and right: this is the horizontal onaxis plane. Along this listening plane, all of the classic crossover designs exhibit no problems. It is when
you lower or raise your head below or above this plane
that the problems arise. is is the crux of Siegfried
Linkwitz’s contribution to crossover design. After all
these years and as hard as it is to believe, he was the
first person to publish an analysis of what happens offaxis with non-coincident drivers (not-coaxial). (Others
may have done it before, but it was never made public
record.)
Figure 1a represents a side view of the combined
acoustic radiation pattern of the two drivers emitting
the same single frequency. at is, a plot of what is
going on at the single crossover frequency all along the
vertical plane. e pattern shown is for the popular 18
dB/octave Butterworth all-pass design with a crossover
frequency of 1700 Hz and drivers mounted 7 inches
apart1.
Linkwitz-Riley-2
1. On-Axis
3. Canc
ellation Axis
2. P
eak
ing Axis
3. Cancellation Axis
Combined Acoustic
Radiation Pattern
Time Corrected Sound
Propagation Plane
+3 dB
Peaking
3
.
C
a
n
c
e
l
l
a
t
i
o
n
A
x
i
s
2
.
P
e
a
k
i
n
g Axis
+3
0
Amplitude (dB)
1700 Hz
Frequency (Hz)
1. On-Axis
On-Axis
Cancellation Axis
Cancellation Axi
s
Combined Acoustic
Radiation Pattern
Time Corrected Sound
Propagation Plane
0 dB
No Peaking
Figure 1a.
crossover.
Figure 1b. Butterworth all-pass design radiation pattern at
Figure 2. Linkwitz-Riley radiation response at crossover.
What is seen is a series of peaking and cancellation
nodes. Back to the string: holding it taut again and
parallel to the floor puts you on-axis. Figure 1a tells us
that the magnitude of the emitted 1700 Hz tone will
be 0 dB (a nominal reference point). As you lower your
head, the tone increases in loudness until a 3 dB peak is
reached at 15 degrees below parallel. Raising your head
above the on-axis line causes a reduction in magnitude
until 15 degrees is reached where there is a complete
cancellation of the tone. ere is another cancellation
axis located 49 degrees below the on-axis. Figure 1b
depicts the frequency response of the three axes for
reference.
For a constant voltage design, the response looks
worse, having a 6 dB peaking axis located at -20 degrees and the cancellation axes at +10 and -56 degrees,
respectively. e peaking axis tilts toward the lagging
driver in both cases, due to phase shift between the
two crossover outputs.
e cancellation nodes are not due to the crossover
design, they are due to the vertically displaced drivers. (e crossover design controls where cancellation
nodes occur, not that they occur.) e fact that the
Linkwitz-Riley-3
On Axis
Ca
ncellation Axis
Pe
ak
ing Axis
Cancellation Axis
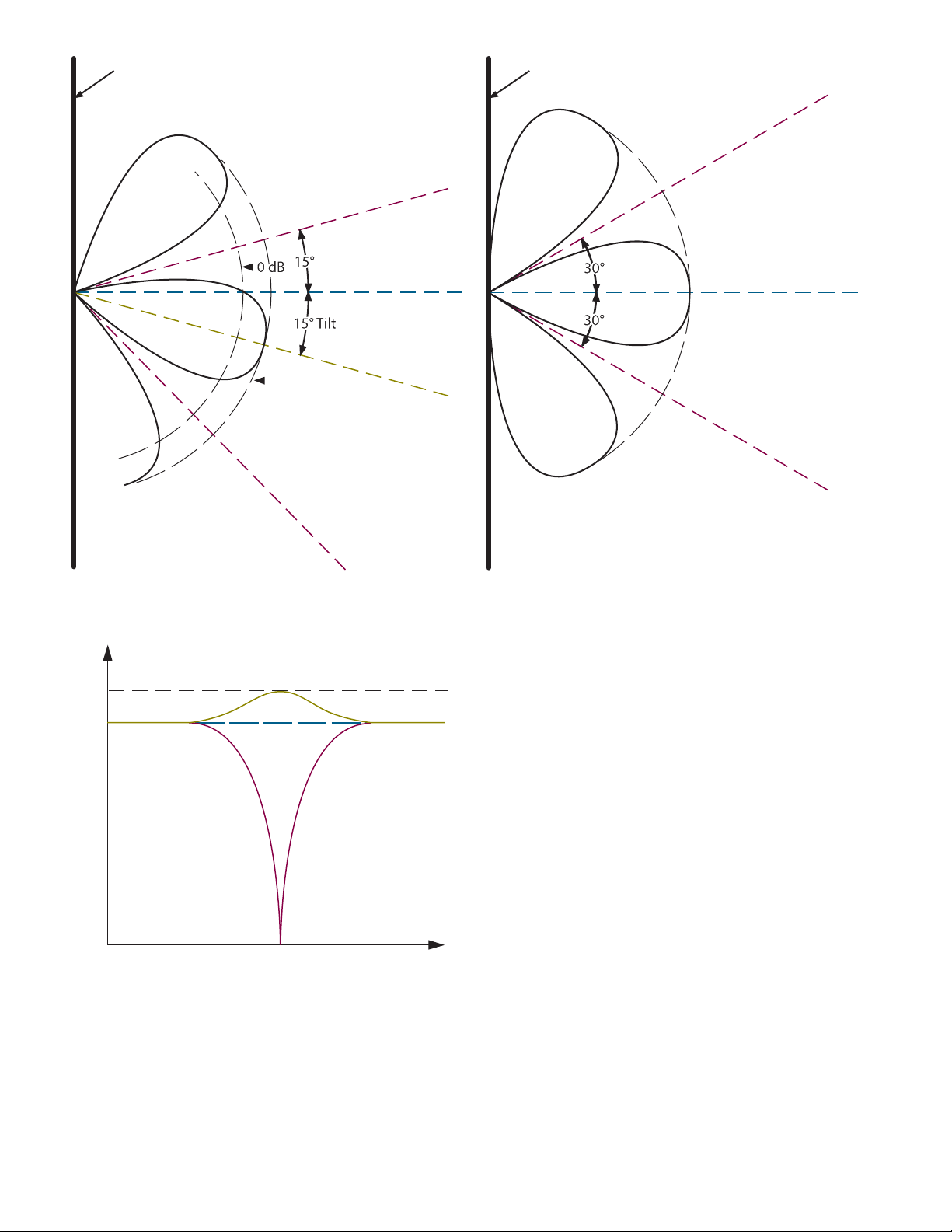
Figure 3. Butterworth all-pass crossover stage-audience relationship.
On Axis
Ca
nc
e
llat
ion Axis
Cancellation Axis
Figure 4. Linkwitz-Riley crossover stage-audience relationship.
drivers are not coaxial means that any vertical deviation from the on-axis line results in a slight, but very
significant difference in path lengths to the listener.
is difference in distance traveled is effectively a
phase shift between the drivers. And this causes cancellation nodes — the greater the distance between
drivers, the more nodes.
In distinct contrast to these examples is Figure
2, where the combined response of a Linkwitz-Riley
crossover design is shown. ere is no tilt and no peaking — just a perfect response whose only limitation is
the dispersion characteristics of the drivers. e main
contributor to this ideal response is the in-phase relationship between the crossover outputs.
Linkwitz-Riley-4
Two of the cancellation nodes are still present, but
are well defined and always symmetrical about the
on-axis plane. eir location changes with crossover
frequency and driver mounting geometry (distance
between drivers). With the other designs, the peaking
and cancellation axes change with frequency and driver
spacing.
Let’s drop the string and move out into the audience
to see how these cancellation and peaking nodes affect
things. Figure 3 shows a terribly simplified, but not too
inaccurate stage-audience relationship with the characteristics of Figure 1 added.
e band is cooking and then comes to a musical
break. All eyes are on the flautist, who immediately goes
into her world-famous 1700 Hz solo. So what happens?
e people in the middle hear it sweet, while those up
front are blown out of their seats, and those in the back
are wondering what the hell all the fuss is!
Figure 4 shows the identical situation but with the
Linkwitz-Riley characteristics of Figure 2 added. Now
the people in the middle still hear everything sweet,
but those up front are not blown away, and those in the
back understand the fuss!
I think you get the point.
Now let’s get real. I mean really real. e system isn’t
two way, it is four way. ere isn’t one enclosure, there
are sixteen. No way are the drivers 7 inches apart — try
27 inches. And time corrected? Fuhgeddaboudit.
Can you even begin to imagine what the vertical
off-axis response will look like with classic crossover
designs? e further apart the drivers are, the greater
the number of peaks and cancellations, resulting in a
multi-lobe radiation pattern. Each crossover frequency
has its own set of patterns, complicated by each enclosure contributing even more patterns. And so on.
(For large driver spacing the Linkwitz-Riley design
has as many lobes as other designs, except that the
peaks are always 0 dB, and the main lobe is always
on-axis.)
Note that all this is dealing with the direct sound
field, no multiple secondary arrivals or room interference or reverberation times are being considered. Is it
any wonder that when you move your real-time analyzer microphone three feet you get a totally different
response?
Now let me state clearly that using a Linkwitz-Riley
crossover will not solve all these problems. But it will
go a long way toward that goal.
-3
0
Amplitude (dB)
Frequency (Hz)
2
4
d
B
/
o
c
t
1
8
d
B
/
o
c
t
12 dB / oc
t
-6
-10
-20
-30
-40
f
o
2f
o
f
o
/2
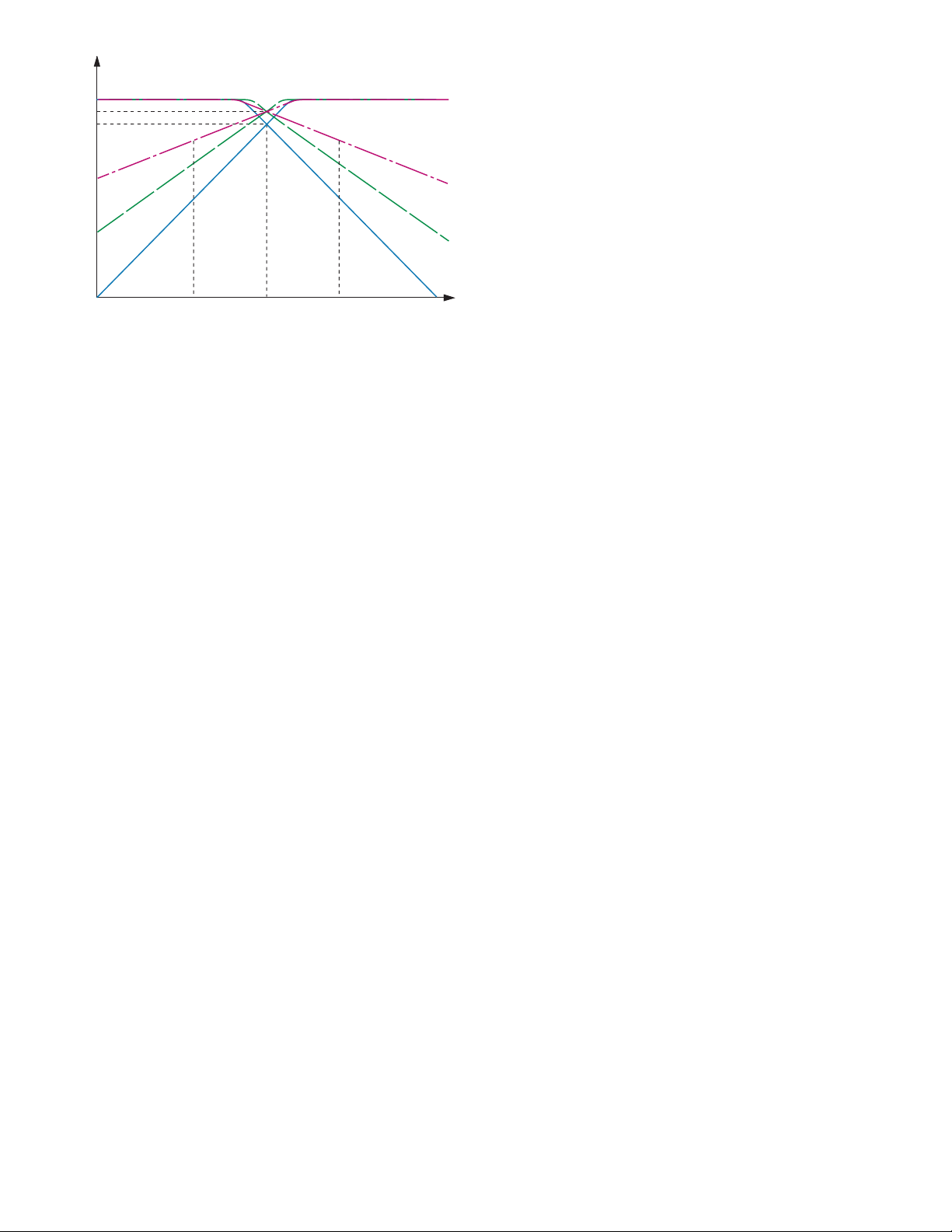
Figure 5. Frequency response of 4th-order Linkwitz-Riley crossover.
witz-Riley alignment is the rolloff rate of 24 dB/octave
(Figure 5). With such a sharp drop-off, drivers can
operate closer to their theoretical crossover points
without the induced distortion normally caused by
frequencies lying outside their capabilities. Frequencies just one octave away from the crossover point are
already attenuated by 24 dB (a factor or about 1/16).
e importance of sharp cutoff rate and in-phase frequency response of the crossover circuitry cannot be
over-stressed in contributing to smooth overall system
response.
Linkwitz-Riley crossover characteristics summary:
1. Absolutely flat amplitude response throughout the
2. e acoustic sum of the two driver responses is unity
3. Zero phase difference between drivers at crossover.
4. e low pass and high pass outputs are everywhere
5. All drivers are always wired the same (in phase).
this is, indeed, the perfect crossover. But such is not so.
e wrinkle involves what is known as “linear phase.”
e other outstanding characteristic of the Link-
passband with a steep 24 dB/octave rolloff rate after
the crossover point.
at crossover. (Amplitude response of each is -6 dB
at crossover, i.e., there is no peaking in the summed
acoustic output.)
(Lobing error equals zero, i.e., no tilt to the polar radiation pattern.) In addition, the phase difference of
zero degrees through crossover places the lobe of the
summed acoustic output on axis at all frequencies.
in phase. (is guarantees symmetry of the polar
response about the crossover point.)
A casual reading of the above list may suggest that
A Linkwitz-Riley crossover alignment is not linear
phase: meaning that the amount of phase shift is a
function of frequency. Or, put into time domain terms,
the amount of time delay through the filter is not
constant for all frequencies, which means that some
frequencies are delayed more than others. (In technical
terms, the network has a frequency-dependent group
delay, but with a gradually changing characteristic.)
Is this a problem? Specifically, is this an audible
“problem?” In a word, no.
Much research has been done on this question [69] with approximately the same conclusions: given a
slowly changing non-linear phase system, the audible
results are so minimal as to be nonexistent; especially
in the face of all of the other system nonlinearities.
And with real-world music sources (remember music?),
it is not audible at all.
State-Variable Solution
One of the many attractions of the Linkwitz-Riley design is its utter simplicity, requiring only two standard
2nd-order Butterworth filters in series. e complexities occur when adjustable crossover frequencies are
required.
After examining and rejecting all of the standard
approaches to accomplish this task, Rane developed
a 4th-order state-variable filter specifically for implementing the Linkwitz-Riley crossover. e state-variable topology was chosen over other designs for the
following reasons:
1. It provides simultaneous high-pass and low-pass out-
puts that are always at exactly the same frequency.
2. Changing frequencies can be done simultaneously
on the high-pass and low-pass outputs without any
changes in amplitude or Q (quality factor).
3. e sensitivities of the filter are very low. (Sensitivity
is a measure of the effects of non-ideal components
on an otherwise, ideal response.)
4. It offers the most cost-effective way to implement
two 4th-order responses with continuously variable
crossover frequencies.
Linkwitz-Riley-5
 Loading...
Loading...