PASCO OS-8459 User Manual

Download

Instruction Manual with

Experiment Guide and

Teachers’ Notes

012-09655A

Beginning Optics System

OS-8459

Optics Bench

Basic Optics System Table of Contents

Contents

Introduction . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 3

About the Equipment . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 4

About the Experiments. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 4

Experiment 1: Color Addition. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 7

Experiment 2: Prism. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 9

Experiment 3: Reflection . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 11

Experiment 4: Snell’s Law . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 13

Experiment 5: Total Internal Reflection . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 15

Experiment 6: Convex and Concave Lenses. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 17

Experiment 7: Hollow Lens . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 19

Experiment 8: Lensmaker’s Equation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 21

Experiment 9: Apparent Depth. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 23

Experiment 10: Focal Length and Magnification of a Thin Lens . . . . . . . . . . . . . . . . . . 27

Experiment 11: Telescope. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 31

Experiment 12: Microscope . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 35

Experiment 13: Shadows. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 39

Telescope and Microscope Test Pattern . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 41

Teacher’s Guide. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 43

Technical Support . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 51

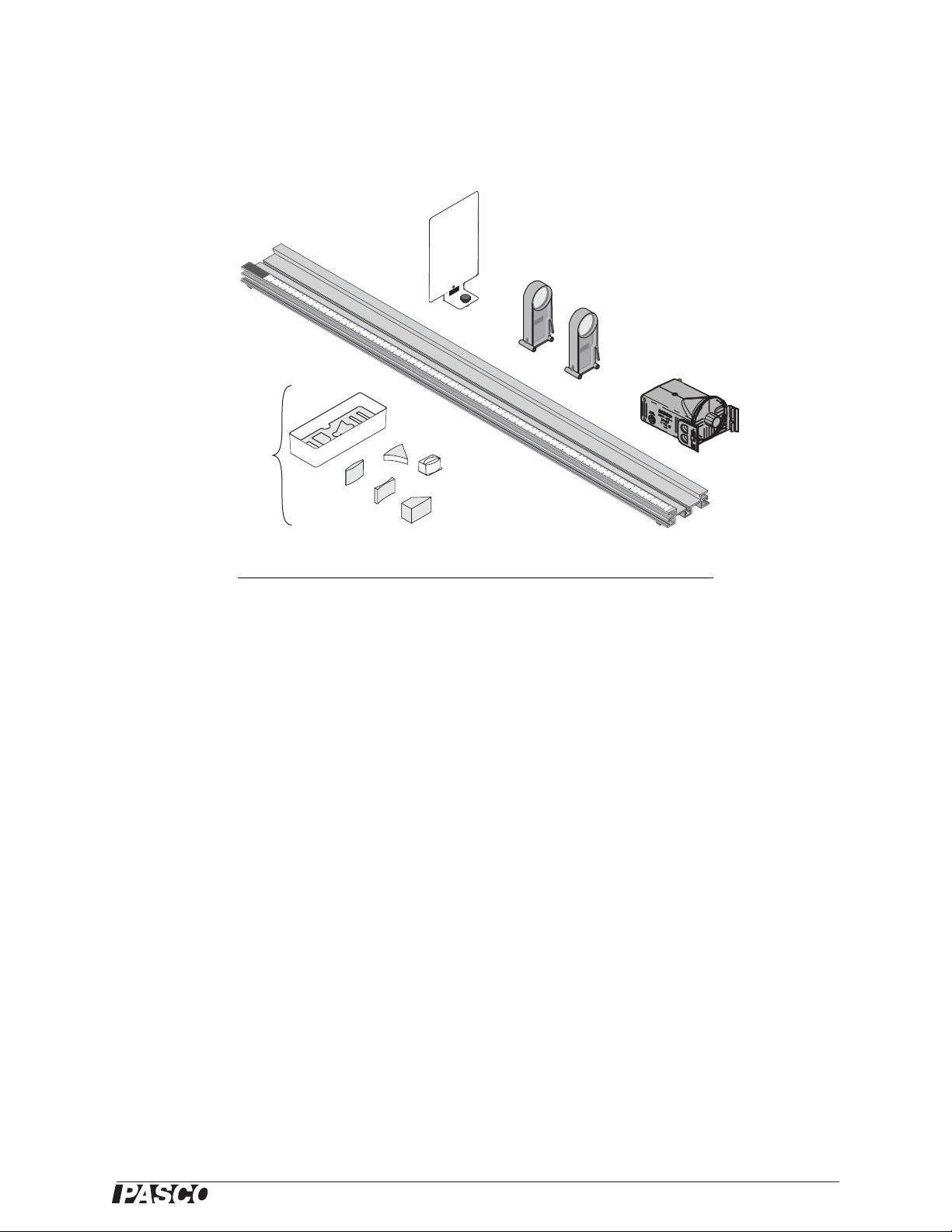
Beginning Optics System

OS-8459

Optics Bench

Included Equipment Part Number

1. 1.2 m Optics Bench OS-8508

2. Viewing Screen OS-8467

3. +100 mm Mounted Lens 003-07204

4. +200 mm Mounted Lens 003-07205

5. Light Source OS-8470

6. Ray Optics Kit with: OS-8516A a. Storage Box/Water Tank 740-177 b. Mirror 636-05100 c. Hollow Lens OS-8511 d. Convex Lens 636-05501 e. Concave Lens 636-05502 f. Acrylic Rhombus 636-05611

Introduction

The PASCO Beginning Optics System contains the optics components you will need for a variety of experiments and demonstrations. This manual includes student instructions and teacher’s notes for 13 typical experiments.

For an even greater variety, you can expand the system with any of the Beginning Optics kits and components available from PASCO, including lasers, polarizers, diffraction slits, and light sensors. See the PASCO Physics catalog or visit www.pasco.com for details.

Beginning Optics System About the Equipment

About the Equipment

For detailed information on the Light Source and Ray Optics Kit, see the instruction sheets included with those components.

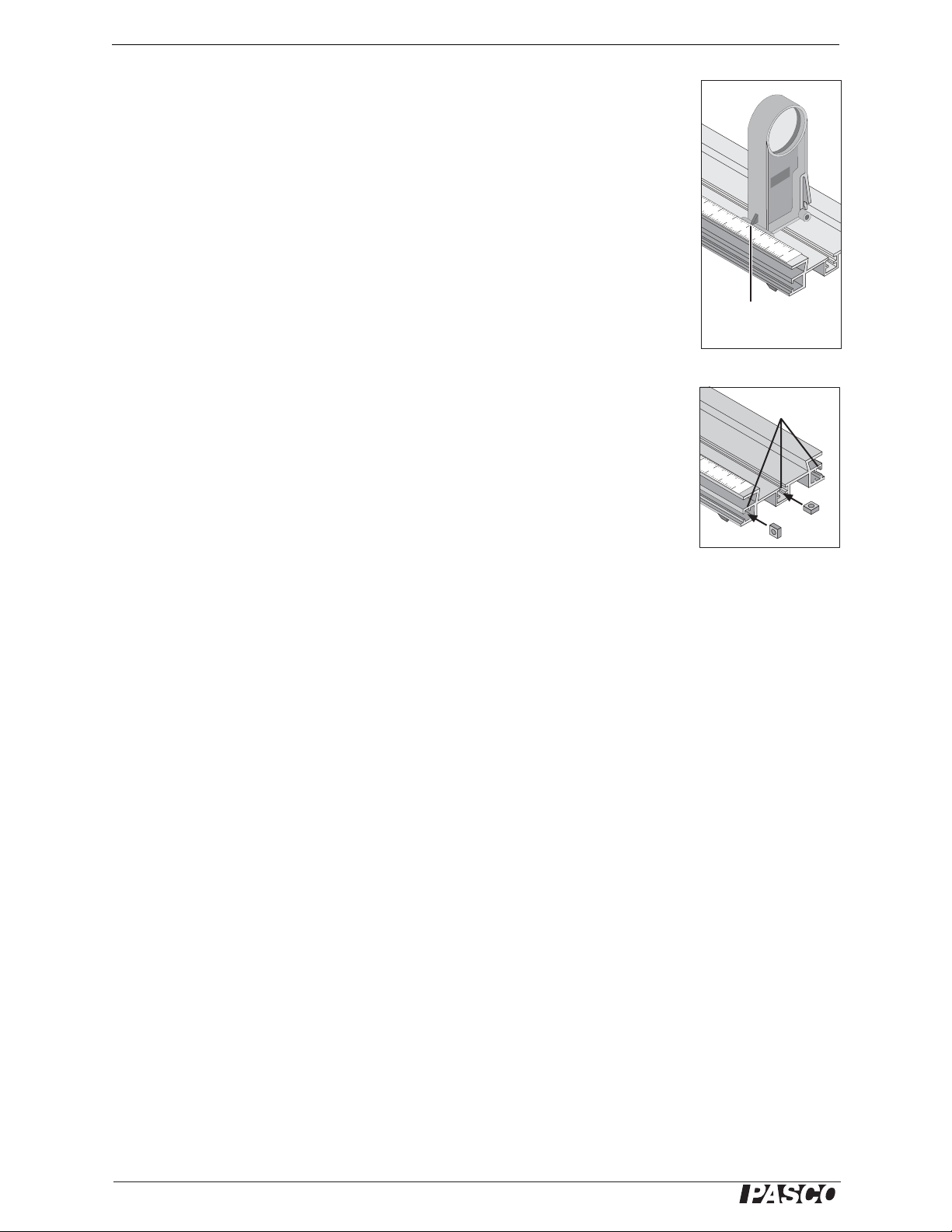
Optics Bench Basic Optics components, such as mounted lenses and the adjustable lens holder, snap into the wide centra l channel of the optics bench. Place the base of the component on the bench and push down firmly to snap it in place. To move it, squeeze the tab on base and slide it along the bench.

Components that include a square bolt and a thumb screw are designed to be fasted to the T -slots on the sides and center of the bench. Slide the bolt into the T -slot, insert the thumb screw through the component’s mounting hold, thread the screw into the bolt and tighten it down.

Use the metric scale on the bench to measure the positions of components.

metric scale for measuring component positions

Light Source The included light source can be used on a tabletop or mounted on the bench. It functions as a bright point source, an illuminated crossed-arrow object, a primary-color source, and a ray box with up to five parallel rays.

Mounted Lenses The Beginning Optics System includes two lenses mounted in holders. Use them on the optics bench with the light source, viewing screen, and other Basic Optics components.

Viewing Screen Mount the screen on the bench to view real images formed by lenses.

Ray Optics Kit The ray optics kit is a set of optics components designed for use with the light source in ray-box mode. To make the rays easy to see and trace, use the ray optics components on a white sheet of paper on a flat table top. The transparent storage box doubles as a water tank for studying lenses under water.

About the Experiments

The experiment instructions on the following pages are arranged and categorized according to which components of the Beginning Optics System they use. See the table at the top of each experiment for a detailed list of required equipment. T eachers’ notes, including typical data and answers to questions, can be found starting on page 43.

T-slots

The experiments that call for the light source work best in a dimly lit room.

Ray Optics Kit Experiments These experiments use the Ray Optics Kit, the Light Source (in ray-box mode), and may require blank white paper, a ruler, protractor, and drawing compass.

1. Color Addition (p age 7): Explore the results of mixing colored ligh t and illumi-

nating colored ink with colored light.

2. Prism (page 9): Show how a prism separates white light into its component col-

ors and show that different colors are refracted at different angles through a prism.

3. Reflection (page 11): Show how rays are reflected from plane, concave, and con-

vex mirrors.

Model No. OS-8459 About the Experiments

4. Snell’s Law (page 13): Determine the index of refraction of acrylic by measuring

angles of incidence and refraction of a ray passing through the rhombus.

5. Total Internal Reflection (page 15): Determine the critical angle at which total

internal reflection occurs in the rhombus.

6. Convex and Concave Lenses (page 17): Use ray tracing to determine the focal

lengths of lenses.

7. Hollow Lens (page 19): Use the hollow lens and water to expl ore how the prop-

erties of a lens are related to its shape, its index of refraction, and the index of refraction of the surrounding medium.

8. Lensmaker’s Equation (page 21): Determine the focal length of a concave lens

by measuring its radius of curvature.

9. Apparent Depth (page 23): Measu re the apparent dep th of the rhombu s and

determine its index of refraction by comparing the apparent depth to the actual thickness.

Optics Bench Experiments These experiments use the Optics Bench, Mounted Lenses, and Viewing Screen. Experiments 10 and 13 also use the Light Source.

10. Focal Length and Magnification of a Thin Lens (page 27): Determine the

focal length of a converging lens by forming an image on the viewing screen.

11. Telescope (page 31): Construct a telescope and determine its magnification.

12. Microscope (page 35): Construct a microscope and determine its magnification.

13. Shadows (page 39): Show the um bra and the penum bra of a shadow.

Beginning Optics System About the Experiments

Model No. OS-8459 Experiment 1: Color Addition

Experiment 1: Color Addition

Required Equipment from Beginning Optics System

Light Source Convex Lens from Ray Optics Kit

Other Required Equipment

Red, blue, and black pens Blank white paper

Purpose

In Part 1 of this experiment, you will discover the results of

Light source

mixing red, green, and blue light in different combinations. In Part 2, you will compare the appearance of red, blue, and black ink illuminated by red and blue light.

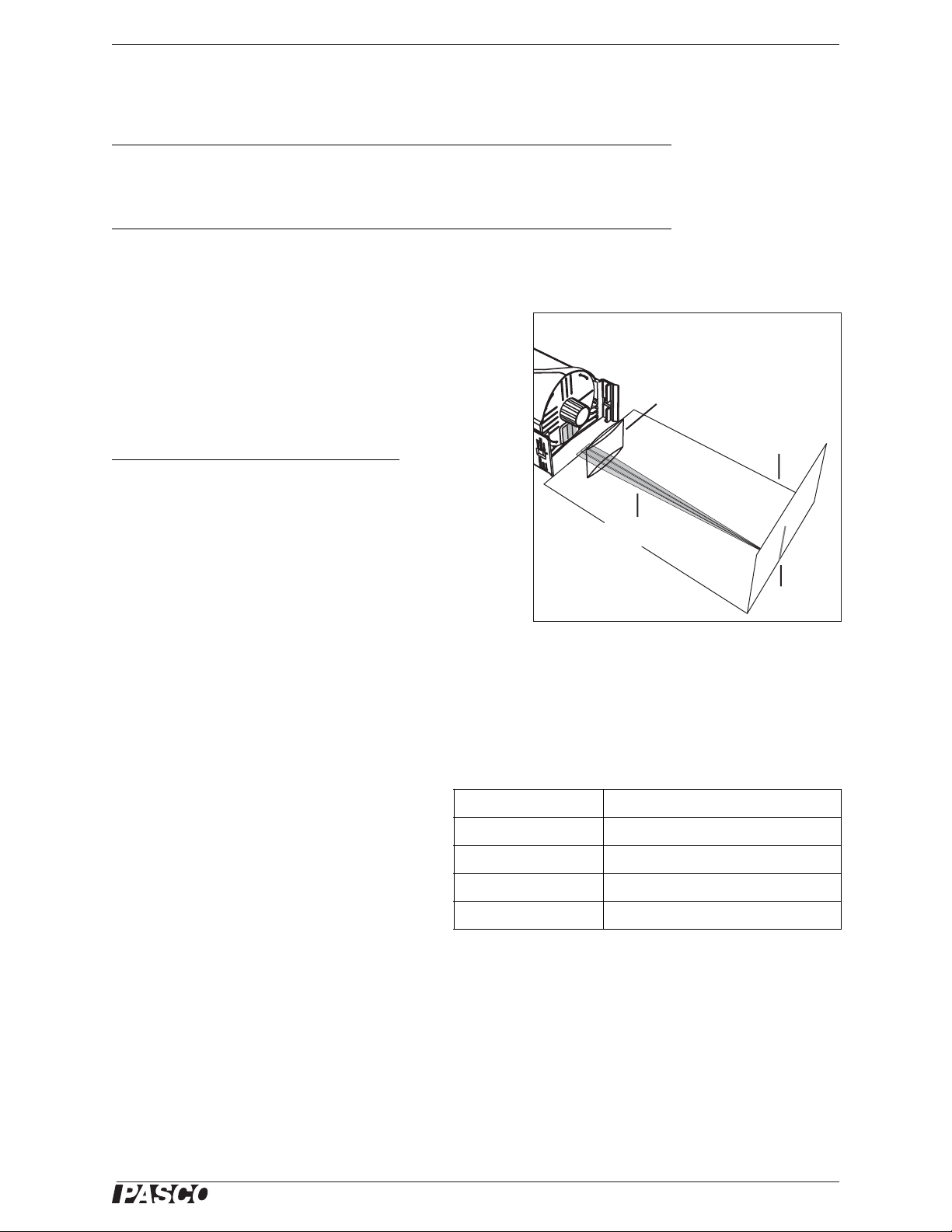
Part 1: Addition of Colored Light Procedure

1. Turn the wheel on the light source to select the red,

green, and blue color bars. Fold a blank, white sheet of paper, as shown in Figure 1.1. Lay the paper on a flat surface and put the light source on it so that the colored rays are projected along the horizontal part of the paper and onto the vertical part.

2. Place the convex lens near the ray box so it focuses the rays and causes them to

cross at the vertical part of the paper.

Note: The lens has one flat edge. Place the flat edge on the paper so the lens stands stably without rocking.

3. What is the resulting color where the three

colors come together? Record your observation in Table 1.1.

4. Now block th e green ray with a pencil.

What color results from adding red and blue light? Record the result in Table 1.1.

red + blue + green red + blue red + green

Table 1.1: Results of Colored Light Addition

Colors Added Resulting Color

Convex lens

Folded paper

Red, green, and blue rays

Combined colors

Figure 1.1: Color addition

5. Block each color in succession to see the

green + blue

addition of the other two colors and complete Table 1.1.

Questions

1. Is mixing colored light the same as mixing colored paint? Explain.

2. White lig ht is said to be the mixture of all colors. In this experiment, did mixing

red, green, and blue light result in white? Explain.

Beginning Optics System Experiment 1: Color Addition

Part 2: Observing Colored Ink Under Colored Light Procedure

1. While you lo ok away, have your partner draw two lines—one red and one

black—on a sheet of white paper. One of the lines should be labeled A, and the other B, but you should not know which is which.

Before you look at the paper, have your partner turn off the room lights and cover the red and green bars so the paper is illuminated only with blue light.

Now look. What colors do the two lines appear to be? Do they appear to be different colors? Record your observations in Table 1.2.

Finally, observe the lines under white light and record their actual colors in Table

1.2.

2. Repeat step 1, but this time have your partner draw lines using blue and black ink

(labeled C and D), and observe them under red light.

3. For Trial 2, switch roles and repeat steps 1 and 2 with the your partner observing

lines that you have drawn. Record the results in Table 1.2. (For this trial, you may try to trick your partner by drawing both lines the same color—both red or both black, for instance.)

Table 1.2: Colored Ink Observed Under Colored Light

Trial 1: Name of observer: ______________________________________

Color of Light Line Apparent Color of Ink Do they look different? Actual Color of Ink

Blue Light

Red Light

Trial 2: Name of observer: ______________________________________

Color of Light Line Apparent Color of Ink Do they look different? Actual Color of Ink

Blue Light

Red Light

A B C D

4. Look a red line and black lines under red light. Which line is easier to see?

_________________________

Questions

1. What makes red ink appear red? When red ink is illumined by blue light, is most

of the light absorbed or reflected?

2. When illum ined with red light, why is red ink on white paper more difficult to

see than black ink?

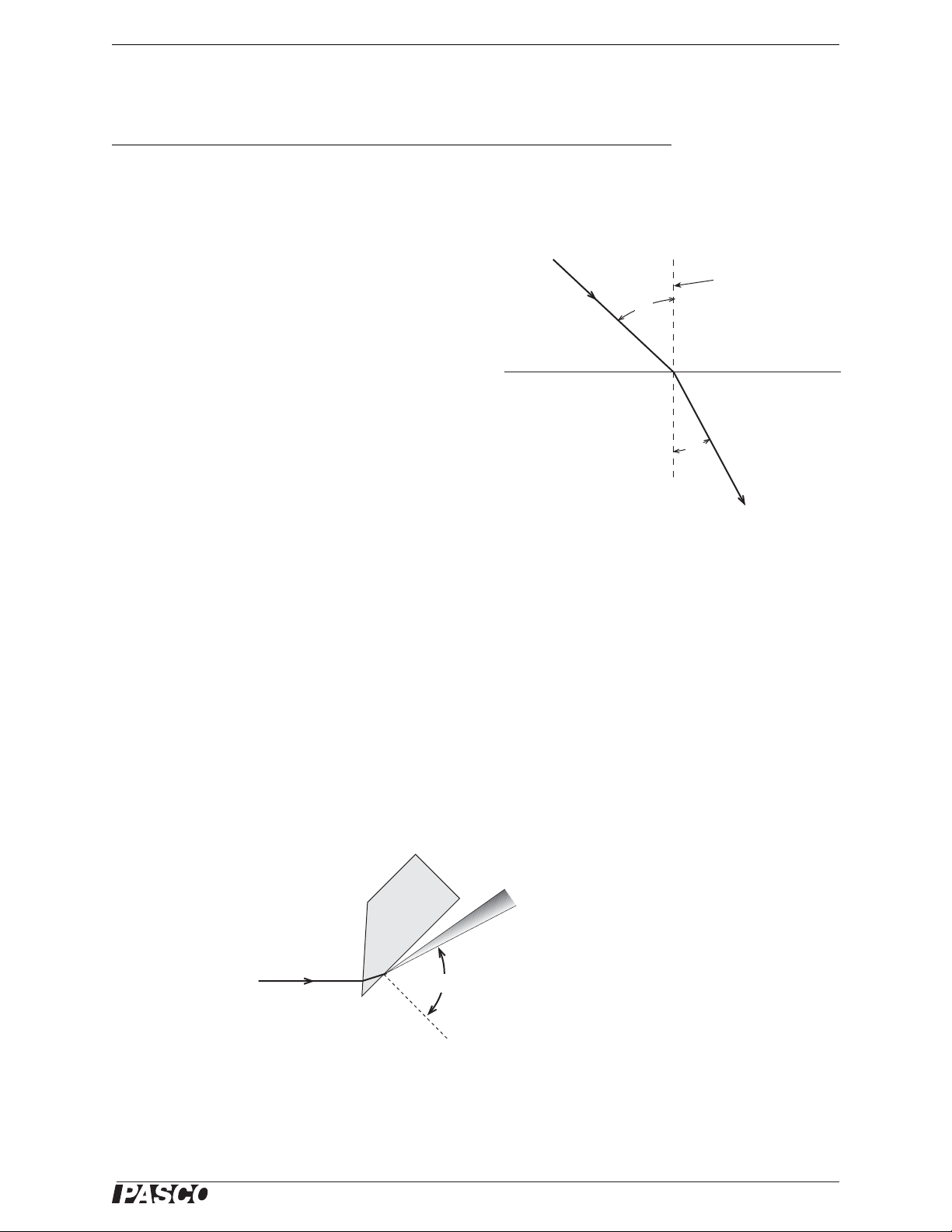
Model No. OS-8459 Experiment 2: Prism

Experiment 2: Prism

Required Equipment from Beginning Optics System

Light Source Rhombus from Ray Optics Kit Blank white paper

Purpose

Incident ray

The purpose of this experiment is to show how a prism separates white light into its component colors and to show that different colors are refracted at different angles through a prism.

Theory

When a monochromatic light ray crosses from one medium (such as air) to another (such as acrylic), it is refracted. According to Snell’s Law,

sin θ1 = n2sin θ

Figure 2.1: Refraction of Light

the angle of refraction (θ2) depends on the angle of incidence (θ1) and the indices of refraction of both media (n

and n2), as shown in Figure 2.1. Because the index of

refraction for light varies with the frequency of the light, white light that enters the material (at an angle other than 0°) will separate into its component colors as each frequency is bent a different amount.

The rhombus is made of acrylic which has an index of refraction of 1.497 for light of wavelength 486 nm in a vacuum (blue light), 1.491 for wavelength 589 nm (yellow), and 1.489 for wavelength 651 nm (red). In general for visible light, index of refraction increases with increasing frequency.

Normal to surface

Surface

Refracted ray

> n

)

Procedure

1. Place the light source in ray-box mode on a sheet of blank white paper. Turn the

wheel to select a single white ray.

Color

spectrum

Single white ray

Normal to surface

Figure 2.2

2. Position the rhombus as shown in Figure 2.2. The acute-angled end of the rhom-

bus is used as a prism in this experiment. Keep the ray near the point of the rhombus for maximum transmission of the light.

Beginning Optics System Experiment 2: Prism

3. Rotate the rhombus until the angle (θ) of the emerging ray is as large as possible

and the ray separates into colors.

(a) What colors do you see? In what order are they?

(b) Which color is refracted at the largest angle?

dependence of the index of refraction for acrylic, which color is predicted to refract at the largest angle?

4. Without repositioning the light source, turn the wheel to select the three primary

color rays. The colored rays should enter rhombus at the same angle that the white ray did. Do the colored rays emerge from the rhombus parallel to each other? Why or why not?

Model No. OS-8459 Experiment 3: Reflection

Experiment 3: Reflection

Required Equipment from Beginning Optics System

Light Source Mirror from Ray Optics Kit

Other Required Equipment

Drawing compass Protractor Metric ruler White paper

Purpose

In this experiment, you will study how rays are reflected from different types of mirrors. You will measure the focal length and determine the radius of curvature of a concave mirror and a convex mirror.

Part 1: Plane Mirror Procedure

1. Place the light source in ray-box mode on a blank sheet of

white paper. Turn the wheel to select a single ray.

2. Place the mirror on the paper. Position the plane (flat) surface

of the mirror in the path of the incident ray at an angle that allows you to clearly see the incident and reflected rays.

3. On the paper, trace and label the surface of the plane mirror

and the incident and reflected rays. Indicate the incoming and the outgoing rays with arrows in the appropriate directions.

4. Remove the light source and mirror from the paper. On the

paper, draw the normal to the surface (as in Figure 3.1).

5. Measure the angle of incidence and the angle of reflection. Measure these angles

from the normal. Record the angles in the first row Table 3.1.

6. Repeat steps 1–5 with a different angle of incidence. Repeat the procedure again

to complete Table 3.1 with three different angles of incidence.

Table 3.1: Plane Mirror Results

Normal to

surface

Incident ray

Reflected ray

Figure 3.1

Angle of Incidence Angle of Reflection

7. Turn the wheel on the light source to select the three primary color rays. Shine

the colored rays at an angle to the plane mirror. Mark the position of the surface of the plane mirror and trace the incident and reflected rays. Indicate the colors of

Beginning Optics System Experiment 3: Reflection

the incoming and the outgoing rays and mark them with arrows in the appropriate directions.

Questions

1. What is the relationship between the angles of incidence and reflection?

2. Are the three colored rays reversed left-to-right by the plane mirror?

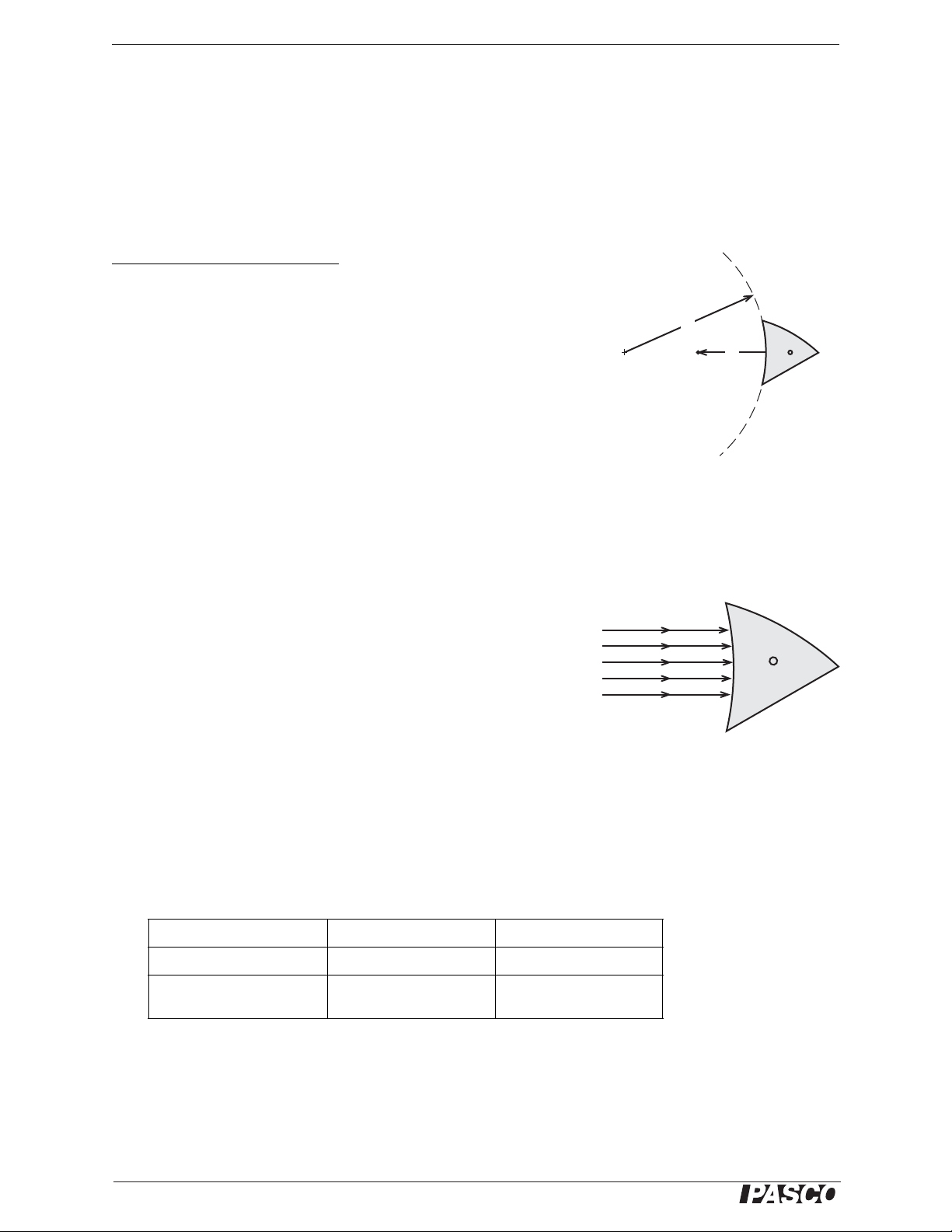
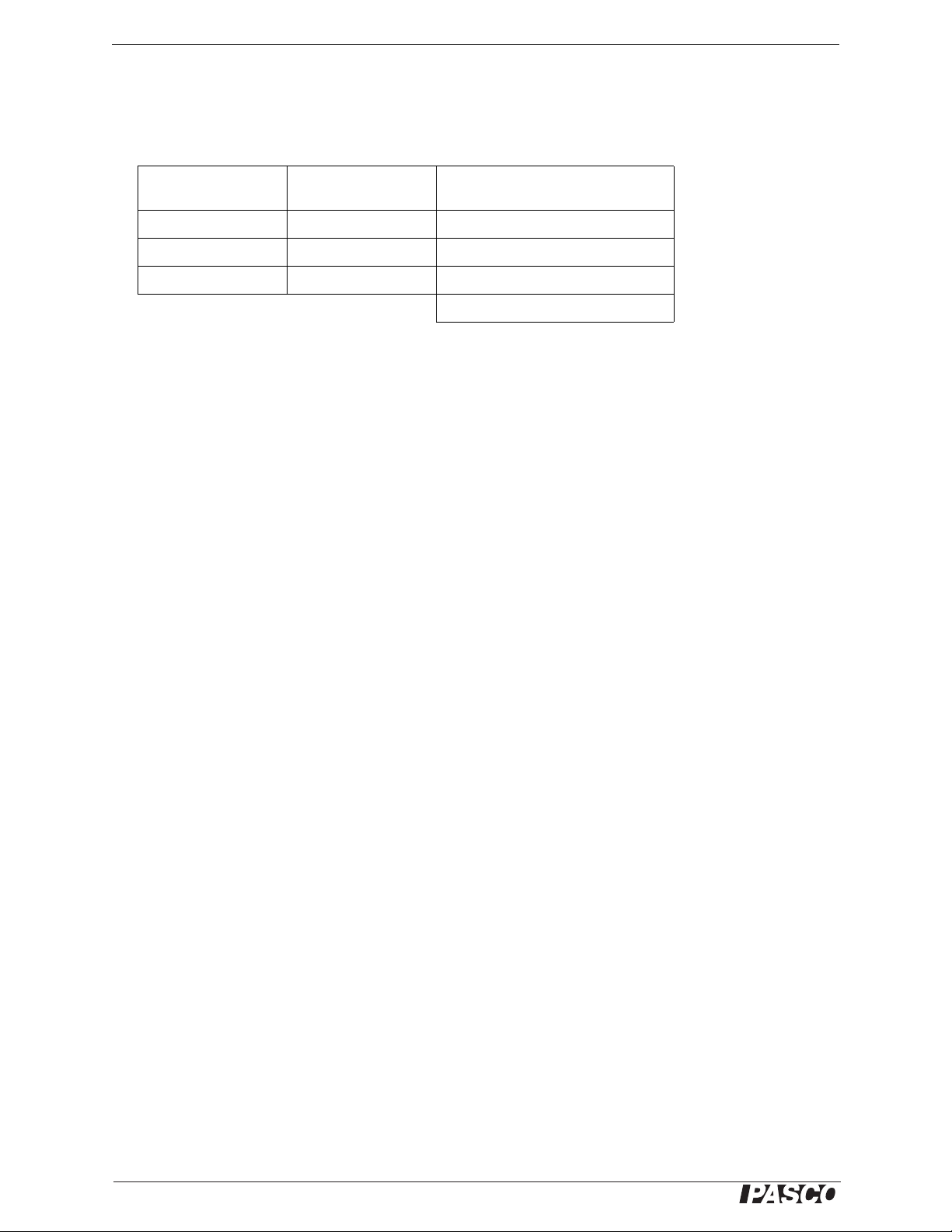
Part 2: Cylindrical Mirrors Theory

mirror

A concave cylindrical mirror focuses incoming parallel rays at its focal point. The focal length ( f) is the distance from the focal point to the center of the mirror surface. The radius of curvature (R) of the mirror is twice the focal length. See Figure 3.2.

focal point

Procedure

1. Turn the wheel on the light source to select five parallel rays. Shine

the rays straight into the concave mirror so that the light is reflected back toward the ray box (see Figure 3.3). Trace the surface of the mirror and the incident and reflected rays. Indicate the incoming and the outgoing rays with arrows in the appropriate directions. (You can now remove the light source and mirror from the paper.)

2. The place where the five reflected rays cross each other is the focal

point of the mirror. Mark the focal point.

Incident rays

3. Measure the focal length from the center of the concave mirror sur-

face (where the middle ray hit the mirror) to the focal point. Record the result in Table 3.2.

4. Use a compass to draw a circle that matches the curvature of the

mirror (you will have to make several tries with the compass set to different widths before you find the right one). Measure the radius of curvature and record it in Table 3.2.

5. Repeat steps 1–4 for the convex mirror. Note that in step 3, the reflected rays will

diverge, and they will not cross. Use a ruler to extend the reflected rays back behind the mirror’s surface. The focal point is where these extended rays cross.

Table 3.2: Cylindrical Mirror Results

Figure 3.2

Figure 3.3

Concave Mirror Convex Mirror

Focal Length Radius of Curvature

(determined using compass)

Questions

1. What is the relationship between the focal length of a cylindrical mirror and its

radius of curvature? Do your results confirm your answer?

2. What is the radius of curvature of a plane mirror?

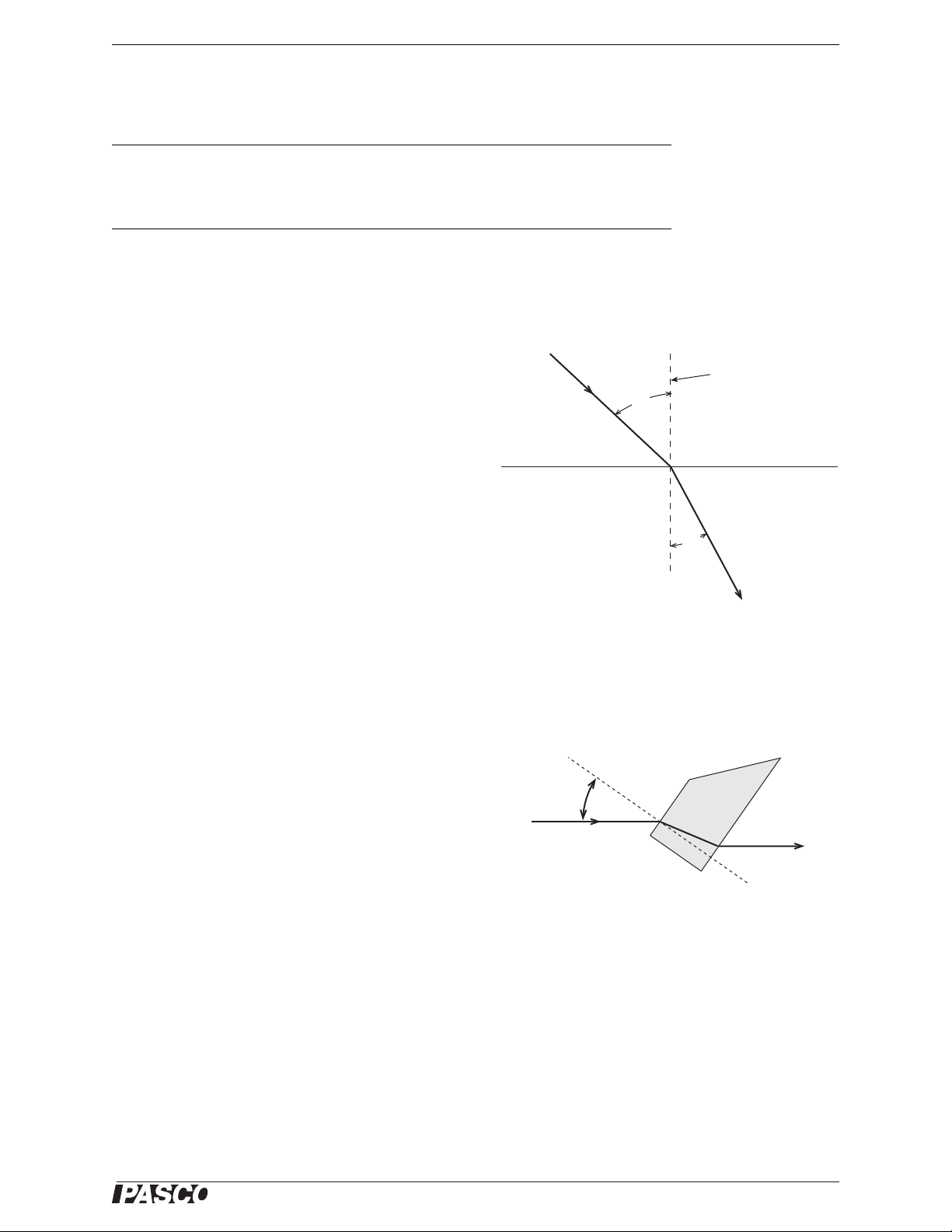
Model No. OS-8459 Experiment 4: Snell’s Law

Experiment 4: Snell’s Law

Required Equipment from Beginning Optics System

Light Source Rhombus from Ray Optics Kit

Other Required Equipment

Protractor White paper

Purpose

The purpose of this experiment is to determine the index of refraction of the acrylic rhombus. For rays entering the rhombus, you will measure the angles of incidence and refraction and use Snell’s Law to calculate the index of refraction.

Theory

For light crossing the boundary between two transparent materials, Snell’s Law states

sin θ1 = n2sin θ

where θ1 is the angle of incidence, θ2 is the angle of refraction, and n

and n2 are the respective indices of

refraction of the materials (see Figure 4.1).

Procedure

1. Place the light source in ray-box mode on a sheet of

white paper. Turn the wheel to select a single ray.

2. Place the rhombus on the paper and position it so

the ray passes through the parallel sides as shown in Figure 4.2.

Incident ray

Figure 4.1

Normal to surface

Surface

Refracted ray

> n

)

3. Mark the position of the parallel surfaces of the

Figure 4.2

rhombus and trace the incident and transmitted rays. Indicate the incoming and the outgoing rays with arrows in the appropriate directions. Carefully mark where the rays enter and leave the rhombus.

4. Remove the rhombus and draw a line on the paper connecting the points where

the rays entered and left the rhombus. This line represents the ray inside the rhombus.

5. Choose either the point where the ray enters the rhombus or the point where the

ray leaves the rhombus. At this point, draw the normal to the surface.

6. Measure the angle of incidence (θ

) and the angle of refraction with a protractor.

Both of these angles should be measured from the normal. Record the angles in the first row of Table 4.1.

Beginning Optics System Experiment 4: Snell’s Law

7. On a new sheet of paper, repeat steps 2–6 with a different angle of incidence.

Repeat these steps again with a third angle of incidence. The first two columns of Table 4.1 should now be filled.

Table 4.1: Data and Results

Angle of Incidence Angle of Refraction Calculated index of refraction of

acrylic

Average:

Analysis

1. For each row of Table 4.1, use Snell’s Law to calculate the index of refraction,

assuming the index of refraction of air is 1.0.

2. Average the three values of the index of refraction. Compare the average to the

accepted value (n = 1.5) by calculating the percent difference.

Question

What is the angle of the ray that leaves the rhombus relative to the ray that enters it?

Model No. OS-8459 Experiment 5: Total Internal Reflection

Experiment 5: Total Internal Reflection

Required Equipment from Beginning Optics System

Light Source Rhombus from Ray Optics Kit

Other Required Equipment

Protractor White paper

Purpose

In this experiment, you will determine the critical angle at which total internal reflection occurs in the acrylic rhombus and confirm your result using Snell’s Law.

Theory

For light crossing the boundary between two transparent materials, Snell’s Law states

sin θ1 = n2sin θ

where θ1 is the angle of incidence, θ2 is the angle of refraction, and n

and n2 are the respective indices of

refraction of the materials (see Figure 5.1).

In this experiment, you will study a ray as it passes out of the rhombus, from acrylic (n =1.5) to air (n

If the incident angle (θ angle (θ

), there is no refracted ray and total internal

reflection occurs. If θ

) is 90°, as in Figure 5.2.

ray (θ

) is greater than the critical

= θc, the angle of the refracted

In this case, Snell’s Law states:

n sin θc = 1 sin 90°

Solving for the sine of critical angle gives:

sin θ

-- -=

air

=1).

Incident ray

= 1

air

Figure 5.1

90°

Reflected ray

Surface

Refracted ray

> n

Reflected ray

Refracted ray

)

Figure 5.2

Beginning Optics System Experiment 5: Total Internal Reflection

point

Re po

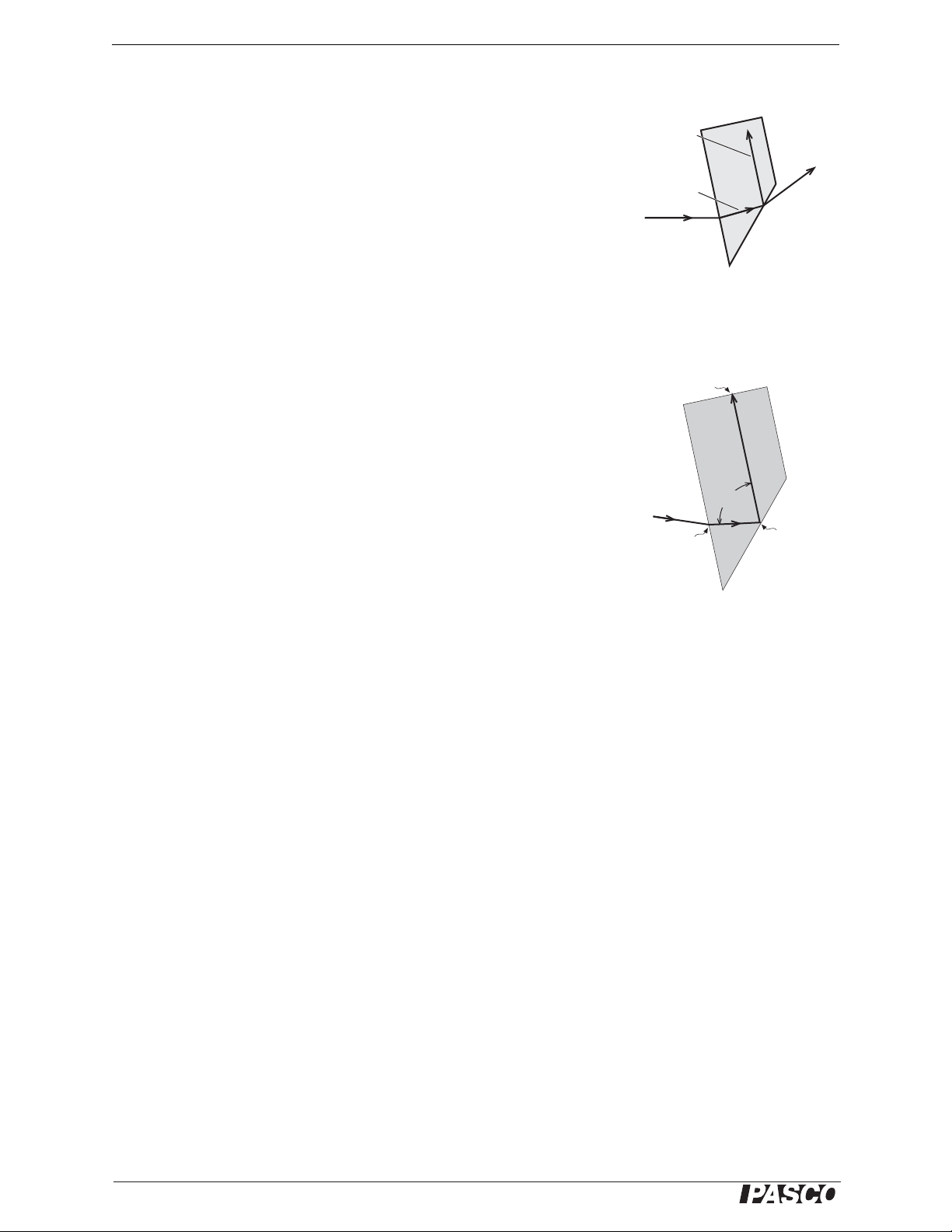
Procedure

1. Place the light source in ray-box mode on a sheet of white paper. Turn the

wheel to select a single ray.

2. Position the rhombus as shown in Figure 5.3, with the ray entering the

rhombus at least 2 cm from the tip.

3. Rotate

the rhombus until the emerging ray just barely disappears. Just as

it disappears, the ray separates into colors. The rhombus is correctly positioned if the red has just disappeared.

4. Mark the surfaces of the rhombus. Mark exactly the point on the surface

where the ray is internally reflected. Also mark the entrance point of the incident ray and the exit point of the reflected ray.

5. Remove the rhombus and draw the rays that are incident upon and

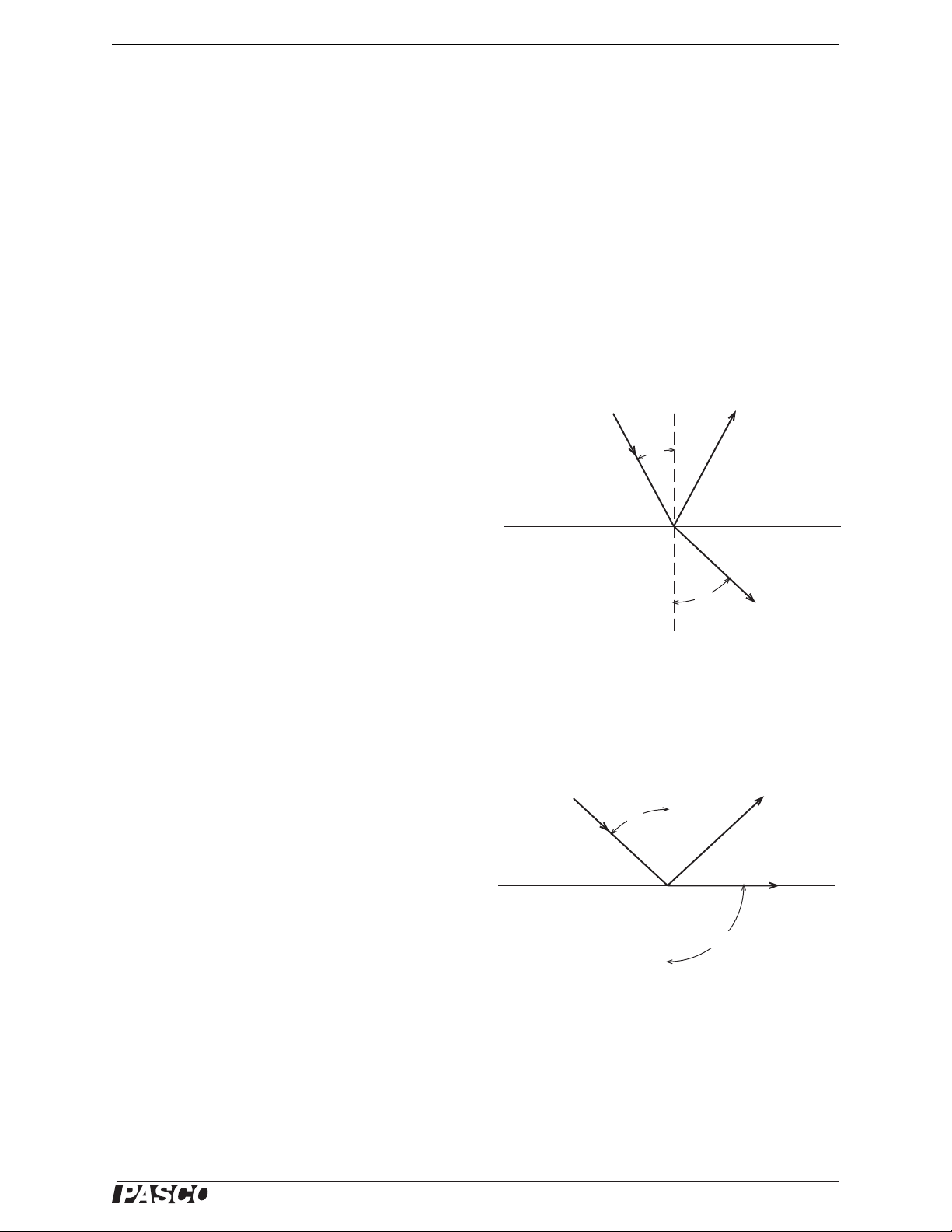
reflected from the inside surface of the rhombus. See Figure 5.4. Measure the angle between these rays using a protractor. (Extend these rays to make the protractor easier to use.) Note that this angle is twice the critical angle because the angle of incidence equals the angle of reflection. Record the critical angle here:

= _______ (experimental)

Reflected

ray

Incident

ray

Exit point

Entrance point

Figure 5.3

Refracted Ray

Reflection point

6. Calculate the critical angle using Snell’s Law and the given index of

refraction for Acrylic (n = 1.5). Record the theoretical value here:

= _______ (theoretical)

7. Calculate the percent difference between the measured and theoretical values:

% difference = _______

Questions

1. How does the brightness of the internally reflected ray change when the incident

angle changes from less than θ

2. Is the critical angle greater for red light or violet light? What does this tell you

about the index of refraction?

to greater than θc?

Figure 5.4

Model No. OS-8459 Experiment 6: Convex and Con cave Lenses

Experiment 6: Convex and Concave Lenses

Required Equipment from Beginning Optics System

Light Source Convex Lens from Ray Optics Kit Concave Lens from Ray Optics Kit

Other Required Equipment

Metric ruler

Purpose

In this experiment, you will explore the difference between convex and concave lenses and determine their focal lengths.

Theory

When parallel light rays pass through a thin lens, they emerge either converging or diverging. The point where the converging rays (or their extensions) cross is the focal point of the lens. The focal length of the lens is the distance from the center of the lens to the focal point. If the rays diverge, the focal length is negative.

Procedure

1. Place the light source in ray-box mode on a white sheet of paper. Turn the wheel

to select three parallel rays. Shine the rays straight into the convex lens (see Figure 6.1).

Note: The lenses used in this experiment have one flat edge. Place the flat edge on the paper so the lens stands stably without rocking.

2. Trace around the surface of the lens and trace the incident and transmitted rays.

Indicate the incoming and the outgoing rays with arrows in the appropriate directions.

3. The point where the outgoing rays cross is the focal point of the lens. Measure

the focal length from the center of the lens to the focal point. Record the result in Table 6.1.

Table 6.1: Results

Convex Lens Concave Lens

Focal Length

4. Repeat the procedure with the concave lens. Note that in step 3, the rays leaving

the lens are diverging and do not cross. Use a ruler to extend the outgoing rays straight back through the lens. The focal point is where these extended rays cross. (Remember to record the focal length as a negative number.)

Incoming rays

Convex lens

Figure 6.1

Beginning Optics System Experiment 6: Convex and Concave Lenses

5. Nest the convex and concave lenses together and place them in the path of the

parallel rays (see Figure 6.2). Trace the rays. Are the outgoing rays converging, diverging or parallel? What does this tell you about the relationship between the focal lengths of these two lenses?

6. Slide the convex and concave lenses apart by a few centimeters and observe the

effect. Then reverse the order of the lenses. T race at leas t one pattern of this type. What is the effect of changing the distance between the lenses? What is the effect of reversing their positions?

Figure 6.2

Model No. OS-8459 Experiment 7: Hollow Lens

Experiment 7: Hollow Lens

Required Equipment from Beginning Optics System

Light Source Hollow Lens from Ray Optics Kit Box from Ray Optics Kit (with lenses and foam insert removed)

Other Equipment

Water Paper towels White paper Double-sided adhesive tape Eye-dropper (optional, for removing water from the hollow lens)

Purpose

In this experiment you will explore how the properties of a lens are related to its shape, its index of refraction, and the index of refraction of the surrounding medium.

Background

A conventional lens is made of a material whose index of refraction is higher than that of the surrounding medium. For instance, the lenses in a pair of eyeglasses are usually made from glass or plastic with an index of refraction of 1.5 or higher, while the air surrounding the lenses has an index of refraction of 1.0. However, a lens can also have a lower index of refraction than the surrounding medium, as is the case when a hollow lens is “filled with air” and surrounded by water. (The index of refraction of water is about 1.3.)

The hollow lens in this experiment has three sections: a plano-concave section and two plano-convex sections. W e will refer to these as sections 1, 2, and 3 (see Figure 7.1).

You will determine whether each section acts as a converging or diverging lens when it is a) filled with water and surrounded by air and b) filled with air and surrounded by water.

Procedure

1. Before you test the hollow lens, make some predictions: For every configuration

in Table 7.1, predict whether incoming parallel rays will converge or diverge after passing through the lens. Record your predictions in the table.

Figure 7.1: The hollow lens

2. Place the light source in ray-box mode on a white sheet of paper. Turn the wheel

to select five parallel rays.

3. Fill section 1 with water and place the lens in front of the light source so the par-

allel rays enter it through the flat side. Do the rays converge or diverge after passing through the lens? Record your observation in T able 7.1.

Beginning Optics Syste m Experiment 7: Hollow Lens

Repeat this step with water in different section of the lens to complete the first four rows of Table 7.1.

Table 7.1: Predictions and Observations

Lens

surrounded by:

Air

Water

Section 1

filled with:

Water Air Air

Air Water Air Air Air Water

Water Air Water

Air Water Water Water Air Water Water Water Air

Section 2

filled with:

Section 3

filled with:

Prediction

(converging or diverging)

4. Dry the bottom of the hollow lens. Use double-sided adhesive tape to stick it to

the inside bottom of the transparent ray-optics box as shown in Figure 7.2. Cut a strip of white paper about 5 cm × 15 cm; tape it to the inside bottom of the box as shown. Position the light source outside of the box so that the rays enter the hollow lens through the flat side.

Box

Hollow lens

Incident rays

Observation

(converging or diverging)

Strip of paper (5 cm x 15 cm)

Figure 7.2: Hollow lens set up for testing surrounded by water

5. Fill the box with water to just below the top of the lens. Fill sections 2 and 3 of

the lens with water (leaving section 1 “filled” with air). Record your observation in Table 7.1.

Repeat this step with air in different section of the lens to complete Table 7.1.

Questions

1. Under what conditions is a plano-convex lens converging? Under what condi-

tions is it diverging?

2. If a plano-concave lens of an unknown material is a diverging lens when sur-

rounded by air, is it possible to know whether the lens will be converging or diverging when placed in water? Explain.

Model No. OS-8459 Experiment 8: Lensmaker’s Equation

Experiment 8: Lensmaker’s Equation

Required Equipment from Beginning Optics System

Light Source Concave Lens from Ray Optics Kit

Other Required Equipment

Metric ruler

Purpose

In this experiment you will determine the focal length of a concave lens in two ways: a) by direct measurement using ray tracing and b) by measuring the radius of curvature and using the lensmaker’s equation.

Theory

The lensmaker’s equation is used to calculate the focal length (in air or a vacuum), f, of a lens based on the radii of curvature of its surfaces (R refraction (n) of the lens material:

and R2) and the index of

(eq. 8.1)

-- - n 1–()

⎛⎞

----- -

----- -–

⎜⎟

⎝⎠

In this notation, R is positive for a convex surface (as viewed from outside the lens) and R is negative for a concave surface (as in Figure 7.1).

Double

Concave

Lens

Figure 8.1

Procedure

1. Place the light source in ray-box mode on a white sheet of paper. Turn the wheel

to select three parallel rays. Shine the rays straight into the convex lens (see Figure 8.2).

Note: The lens has one flat edge. Place the flat edge on the paper so the lens stands stably without rocking.

Incoming rays

Concave lens

Figure 8.2

Beginning Optics System Experiment 8: Lensmaker’s Equation

2. Trace around the surface of the lens and trace the incident and transmitted rays.

Indicate the incoming and the outgoing rays with arrows in the appropriate directions.

3. Remove the lens. To measure the focal length, use a ruler to extend the outgoing

diverging rays straight back through the lens. The focal point is where these extended rays cross. Measure the distance from the center of the lens to the focal point. Record the result as a negative value:

f = _______________ (measured directly)

4. To determine the radius of curvature, put the concave lens back in the path

of the rays and observe the faint reflected rays off the first surface of the lens. The front of the lens can be treated as a concave mirror having a

1/2 R

radius of curvature equal to twice the focal length of the effective mirror (see Figure 8.3).

Trace the surface of the lens and mark the point where the central ray hits the surface. Block the central ray and mark the point where the two outer rays cross. Measure the distance from the lens surface to the point where the reflected rays cross. The radius of curvature is twice this distance. Record the radius of curvature:

R = _______________

5. For this lens, it is not necessary to measure the curvature of both sides because

they are equal (R

= R2 = R). Calculate the focal length of the lens using the lens-

maker’s equation (Equation 8.1). The index of refraction is 1.5 for the acrylic lens. Remember that a concave surface has a negative radius of curvature.

f = __________ _____ (calculated)

6. Calculate the percent difference between the two values of f from step 3 and

step 5:

% difference = _______________

Concave lens

Figure 8.3: Reflected rays from

the lens surface

Model No. OS-8459 Experiment 9: Apparent Depth

Experiment 9: Apparent Depth

Required Equipment from Beginning Optics System

Light Source Rhombus from Ray Optics Kit Convex Lens from Ray Optics Kit Mirror from Ray Optics Kit (used to block rays)

Other Required Equipment

Metric ruler White paper Very sharp pencil

Purpose

In this experiment, you will use two different methods to measure the apparent depth of the acrylic rhombus. You will also determine the index of refraction of acrylic by comparing the apparent depth to the actual depth.

Theory

Light rays originating from the bottom surface of a block of transparent material refract at the top surface as the rays emerge from the material into the air (see Figure 9.1). When viewed from above, the apparent depth, d, of the bottom sur-

air

n > 1

= 1

face of the block is less than the actual thickness, t, of the block. The apparent depth is given by

(eq. 9.1) d = t/n

where n is the index of refraction of the material.

Part 1: Parallax Method Background

Place this page flat on the table in front of you. Hold a pencil horizontally a few centimeters above the paper. With one eye closed or covered, look down at the pencil and move your head side to side (without moving the pencil). Notice how the pencil appears to move relative to the words printed on the paper; this phenomenon is known as parallax. Now hold the tip of the pencil on the paper and check for parallax. When there is no parallax between to objects, they are at the same distance from you.

Figure 9.1

top

bottom

Procedure

1. Place a blank sheet of paper flat on the table. Use a straight edge and pencil to

draw a vertical line on the paper. Place the rhombus on the paper over the line as shown in Figure 9.2.

Beginning Optics System Experiment 9: Apparent Depth

Paper

Rhombus

Line

Figure 9.2

2. With both eyes, look down through the top of the rhombus. Does the line viewed

through the rhombus appear to be closer? Close or cover one eye, and move your head side to side. Do you see parallax between the line viewed through the rhombus and the line viewed directly?

3. In this step, you will hold a pencil near the rhombus to determine the position of

the apparent line. When the pencil and the apparent line are at the same distance from your eye, there will be no parallax between them.

While looking down through the rhombus (with one eye), hold a very sharp pencil as shown in Figure 9.3 so it appears to be lined up with the line inside the rhombus. Move your head left and right to check for parallax. Move the pencil up or down and check again. When there is no parallax, mark that point. (Hold the rhombus with your free hand, press the pencil tip gently against the side of the rhombus and twist the pencil to make a light mark. Erase the mark after you have finished this experiment.)

Analysis

Look

down

Move eye

side to side

Hold pencil

still

Figure 9.3

1. Measure the distance from the top of the rhombus to your pencil mark. Record

this apparent depth, d, in the first row of Table 9.1.

2. Measure the thickness, t, of the rhombus and record it in Table 9.1.

3. Use Equation 9.1 to calculate the index of refraction and record your result in

Table 9.1.

Table 9.1: Results

dtn

Part 1: Parallax method Part 2: Ray-tracing method

Part 2: Ray-tracing Method Procedure

1. Place the light source in ray-box mode on a white sheet of paper. Turn the wheel

to select five parallel rays. Shine the rays straight into the convex lens. Place the mirror on its edge between the ray box and the lens so that it blocks the middle three rays, leaving only the outside two rays (as in Figure 9.4, but do not put the rhombus there yet).

Note: The lens has one flat edge. Place the flat edge on the paper so the lens stands stably without rocking.

Model No. OS-8459 Experiment 9: Apparent Depth

2. Mark the place on the paper where the two rays cross each other.

3. Position the rhombus as shown in Figure 9.4. The “bottom” surface of the

rhombus must be exactly at the point where the two rays cross. The crossed rays simulate rays that originate at an object on the “bottom” of the block.

4. Trace the rhombus and trace the rays diverging from the “top” surface.

5. Remove the rhombus and light source. Trace the diverging rays back into the

rhombus. The point where these rays cross (inside the rhombus) is the apparent position of the “bottom” of the rhombus when viewed through the “top”.

Analysis

1. Measure the apparent depth, d, and record it in Table 9.1.

2. Use Equation 9.1 to calculate the index of refraction and record your result

in Table 9.1.

Questions

1. Of the two methods that you used to determine d, which one is more precise?

Explain.

2. The accepted value of the index of refraction of acrylic is n = 1.49. What was the

percent difference between the accepted value and each of your two results?

top surface

bottom surface

Convex lens

Mirror on edge

Figure 9.4

Beginning Optics System Experiment 9: Apparent Depth

Model No. OS-8459 Experiment 10: Focal Length and Magnification of a Thin Lens

Experiment 10: Focal Length and Magnification of a Thin Lens

Required Equipment from Beginning Optics System

Light Source Bench Converging lens of unknown focal length Screen

Other Equipment

Metric ruler Optics Caliper (optional, for measuring image sizes), PASCO part OS-8468

Instructors: see note on page 46.

Purpose

The purpose of this experiment is to determine the focal length of a thin lens, and to measure the magnification for a certain combination of object and image distances.

Theory

For a thin lens:

(eq. 10.1)

where f is focal length, d

is the distance between the object and the lens, and di is the

-- -

distance between the image and the lens. By measuring d

-----

----+=

and di the focal length can

be determined.

Magnification, M, is the ratio of image size to object size. If the image is inverted, M is negative.

Part I: Object at Infinity

In this part, you will determine the focal length of the lens by making a single mea-

surement of d

with .

∞≅

Procedure

1. Hold the lens in on e hand and the screen in the other hand. Focus the image of a

distant bright object (such as a window or lamp across the room) on the screen.

2. Have your partner measure th e distance from the lens to the screen. This is the

image distance, d

= _______________

Analysis

1. As do approaches infinity, what does 1/do approach?

Beginning Optics System Experiment 10: Focal Length and Magnification of a Thin Lens

2. Use the Thin Lens Formula (Equation 10.1) to calculate the focal length.

f = _______________

Part II: Object Closer Than Infinity

In this part, you will determine the focal length by measuring several pairs of object and image distances and plotting 1/d

Light source Lens

versus 1/di.

1 m

Figure 10.1

Screen

Procedure

1. Place the light source and the screen on the optics bench 1 m apart with the light

source’s crossed arrow object toward the screen. Place the lens between them (see Figure 10.1).

2. Starting with the lens close to the screen, slide the lens away from the screen to a

position where a clear image of the crossed-arrow object is formed on the screen. Measure the image distance and the object distance. Record these measurements (and all measurements from the following steps) in Table 10.1.

3. Measure the object size and the image size for this position of the lens.

4. Without moving the screen or the light source, move the lens to a second position

where the image is in focus. Measure the image distance and the object distance.

5. Measure the object size and im age size for this position also. Note that you will

not see the entire crossed-arrow pattern. Instead, measure the image and object sizes as the distance between two index marks on the pattern (see Figure 10.2 for example).

6. Repeat steps 2 and 4 with lig ht source-to-screen distances of 90 cm, 80 cm, 70

cm, 60 cm, and 50 cm. For each light source-to-screen distance, find two lens positions where clear images are formed. (You don’t need to measure image and object sizes.).

Analysis Part A: Focal Length

1. Calculate 1/do and 1/di for all 12 rows in Table 10.1.

Measure object or image

size between two

pattern features

2. Plot 1/d

versus 1/di and find the best-fit line (linear fit). This will give a straight

line with the x- and y-intercepts equal to 1/f. Record the intercepts (including units) here:

y-intercept = 1/f = _______________

x-intercept = 1/f = _______________

Note: You can plot the data and find the best-fit line by hand on paper or on a computer.

Figure 10.2

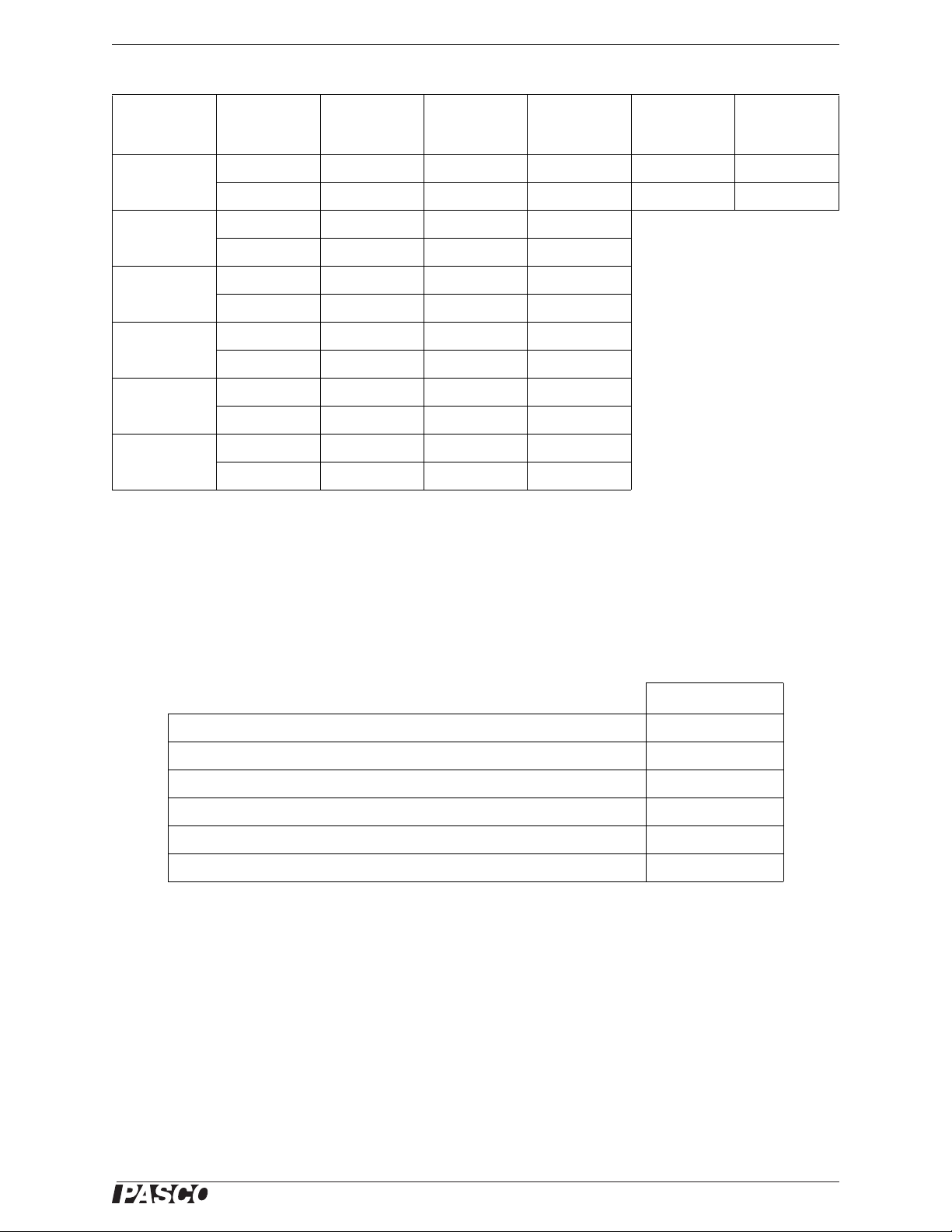
Model No. OS-8459 Experiment 10: Focal Length and Magnification of a Thin Lens

Table 10.1: Image and Object Distances

Distance from

light source to

screen

100 cm

90 cm

80 cm

70 cm

60 cm

50 cm

1/d

Image Size Object Size

3. For each intercept, calculate a value of f and record it in Table 10.2.

4. Find the percent difference between these two values of f and record them in

Table 10.2.

5. Average these two values of f. Find the percent difference between this average

and the focal length that you found in Part I. Record these data in Table 10.2.

Table 10.2: Focal Length

Result from x-intercept Result from y-intercept % difference between results from intercepts Average of results from intercepts Result from Part I % difference between Average of results from intercepts and result from Part I

Analysis Part B: Magnification

1. For the first two data points only (the first two lines of Table 10.2), use image and

object distances to calculate the magnification, M, at each position of the lens. Record the results in Table 10.3.

(eq. 10.2)

⎛⎞

-----

–=

⎜⎟

⎝⎠

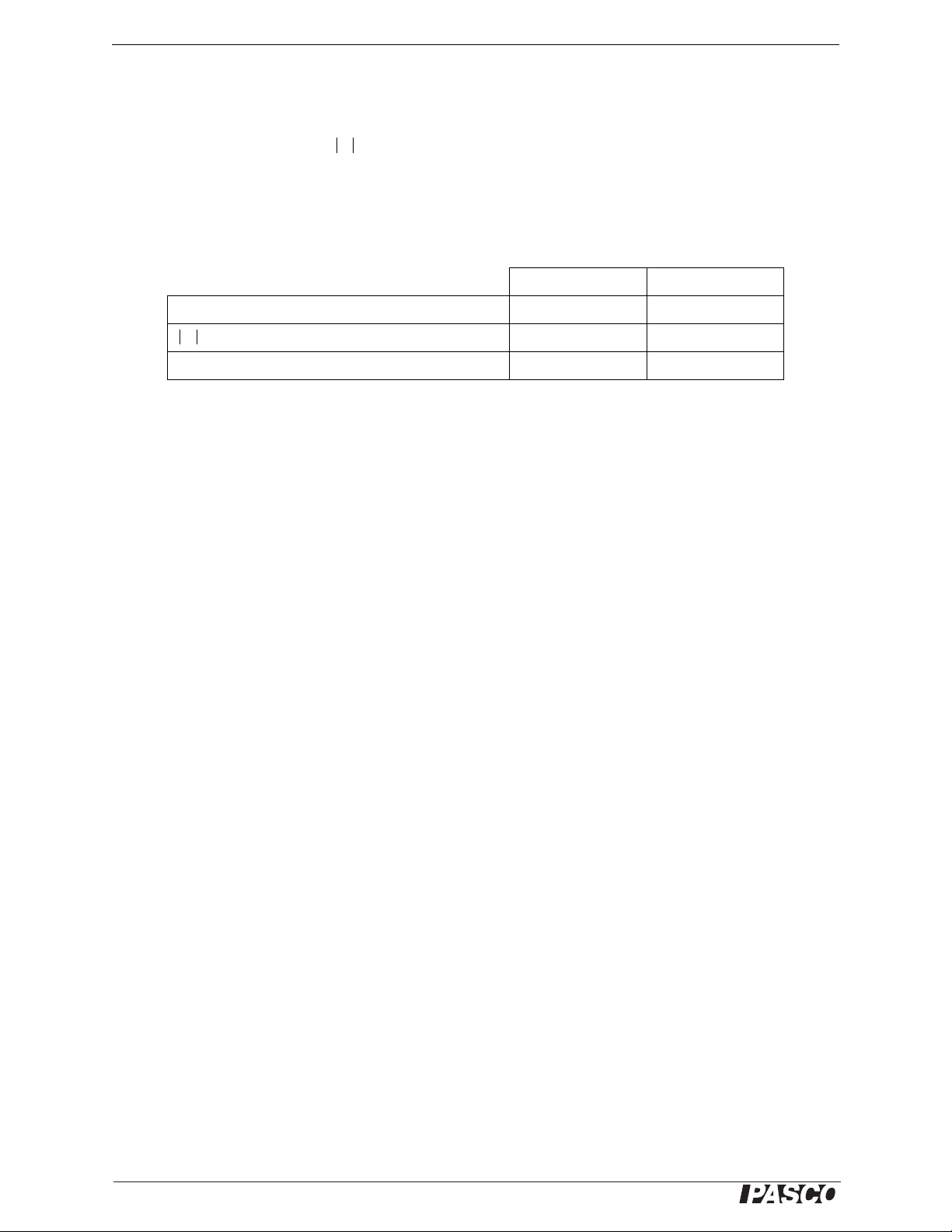
Beginning Optics System Experiment 10: Focal Length and Magnification of a Thin Lens

2. Calculate the absolute value of M (for each of the two lens positions) using your

measurements of the image size and object size. Record the results in Table 10.3.

(eq. 10.3)

image size

------------------------ -= object size

3. Calculate the percent differences between the absolute values of M found using

the two methods. Record the results in Table 10.3.

Table 10.3: Magnification

Point 1 Point 2

calculated from image and object distances

calculated from image and object sizes

% difference

QUESTIONS

1. Is the image formed by the lens upright or inverted?

2. Is the image real or virtual? Ho w do you know?

3. Explain why, for a given screen-to-object distance, there are two lens positions

where a clear image forms.

4. By looking at the image, how can you tell that the magnification is negative?

5. You made three separate determinations of f (by measuring it directly with a dis-

tant object, from the x-intercept of your graph, and from the y-intercept). Where these three values equal? If they were not, what might account for the variation?

Model No. OS-8459 Experiment 11: Telescope

Experiment 11: Telescope

Required Equipment from Beginning Optics System

Bench 2 Convex Lenses (+100 mm and +200 mm) Screen Paper grid pattern (see page 41), or a 14 × 16 grid of 1 cm squares

Purpose

In this experiment, you will construct a telescope and determine its magnification.

Theory

-d

Object

Image

Lens

+200 mm

Figure 11.1

An astronomical telescope consists of two convex lenses. The astronomical telescope in this experiment will form an image in the same place as the object (see Figure

11.1).

The lenses are thin compared to the other distances involved, which allows the Thin Lens Formula to be used:

(eq. 11.1)

where f is focal length, d

-- -

is the distance between the object and the lens, and di is the

-----

----+=

distance between the image and the lens.

The magnification, M, of a two-lens system is equal to the product of the magnifications of the individual lenses:

di1–

–

(eq. 11.2)

1M2

⎛⎞

----------

⎜⎟

⎝⎠

⎛⎞

----------

⎜⎟ ⎝⎠

Eye

Lens

+100 mm

Set Up

1. Tape the paper grid pattern to the screen to serve as the object.

2. The +200 m m lens is the obj ective lens (the one closer to the object). The +100

mm lens is the eyepiece lens (the one closer to the eye). Place the lenses near one

Beginning Optics System Experiment 11: Telescope

end of the optics bench and place the screen on the other end (see Figure 11.2). Their exact positions do not matter yet.

Screen

Figure 11.2

Procedure

1. Put your eye close to the eyepiece lens and look through both lenses at the grid

pattern on the screen. Move the objective lens to bring the image into focus.

Screen

Objective

lens

Figure 11.3

Eyepiece

lens

+200 mm

objective lens

+100 mm eyepiece lens

Right eye

Left eye

2. In this step, you will adjust your telescope to make the image occur in the

same place as the object. T o do this , you will look at both image and object at the same time and judge their relative positions by moving your head side to side. If the image and object are not in the same place, then they will appear to move relative to each other. This effect is known as parallax.

Open both eyes. Look with one eye through the lenses at the image and with the other eye past the lenses at the object (see Figure 11.3). The lines of the image (solid lines shown in Figure 11.4) will be superimposed on the lines of the object (shown as dotted lines in Figure 11.4). Move your head left and right or up and down by about a centimeter. As you move your head, the lines of the image may move relative to the lines of the object due to the parallax. Adjust the eyepiece lens to eliminate parallax. Do not move the objective lens. When there is no parallax, the lines in the center of the lens appear to be stuck to the object lines.

Note: You will probably have to adjust the eyepiece lens by no more than a few centimeters.

3. Record the position s of the lenses and screen in Table 11.1.

4. Estim a te the mag nification of your telescope by counting the number of object

squares that lie along one side of one image square. To do this, you must view the image through the telescope with one eye while looking directly at the object with the other eye. Remember that magnification is negative for an inverted image. Record the observed magnification in Table 11.1.

Lens Holder

Figure 11.4

Analysis

T o calculate the magnification, complete the following steps and record the results in Table 11. 1:

Model No. OS-8459 Experiment 11: Telescope

1. Measure do1, the distance from the object (paper pat-

tern on screen) to the objective lens.

2. Determine d

, the distance from the eyepiece lens to

the image. Since the image is in the plane of the object, this is equal to the distance between the eyepiece lens and the object (screen). Remember that the image distance for a virtual image is negative.

3. Calculate d

using do1 and the focal length of the

objective lens in the Thin Lens Formula (Equation

11.1).

4. Calculate d

by subtracting di1 from the distance

between the lenses.

5. Calculate the mag nificati on using Equation 11.2.

6. Calculate the percent difference between the calcu-

lated magnification and the observed value.

Questions

1. Is the image inverted or upright?

Table 11.1: Results

Position of Objective Lens Position of Eyepiece Lens Position of Screen Observed magnification

Calculated Magnification Percent Difference

2. Is the image that you see through the telescope real or virtual?

Further Study

Image Formed by the Objective Lens

Where is the image formed by the objective lens? Is it real or virtual? Use a desk lamp to brightly illuminate the paper grid (or replace the screen with the light source’s crossed-arrow object). Hold a sheet of paper vertically where you think the image is. Do you see the image? Is it inverted or upright? Remove the sheet of paper and hold a pencil in the same place. Look through eyepiece lens; you will see two images, one of the pencil and one of the grid pattern. Are both images inverted? Use parallax to determine the location of the pencil image.

Object at Infinity

Remove the screen and look through the lenses at a distant object. Adjust the distance between the lenses to focus the telescope. Estimate the observed magnification.

Now calculated the magnification by taking the ratio of the focal lengths of the lenses. Compare the calculated magnification to the observed magnification.

How is the distance between the lenses related to their focal lengths?

Beginning Optics System Experiment 11: Telescope

Model No. OS-8459 Experiment 12: Microscope

Experiment 12: Microscope

Required Equipment from Beginning Optics System

Bench 2 Convex Lenses (+100 mm and +200 mm) Screen Paper grid pattern (see page 41), or a 14 × 16 grid of 1 cm squares

Purpose

In this experiment, you will construct a microscope and determine its magnification.

Theory

Object

Image

Lens

+100 mm

Figure 12.1

Lens

+200 mm

A microscope magnifies an object that is close to the objective lens. The microscope in this experiment will form an image in the same place as the object (see Figure

12.1).

The lenses are thin compared to the other distances involved, which allows the Thin Lens Formula to be used:

(eq. 12.1)

where f is focal length, d

-- -

is the distance between the object and the lens, and di is the

-----

----+=

distance between the image and the lens.

The magnification, M, of a two-lens system is equal to the product of the magnifications of the individual lenses:

di1–

–

(eq. 12.2)

1M2

⎛⎞

----------

⎜⎟

⎝⎠

⎛⎞

----------

⎜⎟ ⎝⎠

Eye

Set Up

1. Tape the paper grid pattern to the screen to serve as the object.

2. The +100 m m lens is the obj ective lens (the one closer to the object). The +200

mm lens is the eyepiece lens (the one closer to the eye). Place the lenses near the

Beginning Optics System Experiment 12: Microscope

middle of the optics bench and place the screen near the end of the bench (see Figure 12.2).

+100 mm

objective lens

Screen

Figure 12.2

+200 mm eyepiece lens

Procedure

1. Put your eye close to the eyepiece lens and look through both lenses at the grid

pattern on the screen. Move the objective lens to bring the image into focus.

Screen

Objective

Figure 12.3

lens

Eyepiece

lens

Right eye

Left eye

2. In this step, you will adjust your microscope to make the image occur in the

Open both eyes. Look with one eye through the lenses at the image and with the other eye past the lenses at the object (see Figure 12.3). The lines of the image (solid lines shown in Figure 12.4) will be superimposed on the lines of the object (shown as dotted lines in Figure 12.4). Move your head left and right or up and down by about a centimeter. As you move your head, the lines of the image may move relative to the lines of the object due to the parallax. Adjust the eyepiece lens to eliminate parallax. Do not move the objective lens. When there is no parallax, the lines in the center of the lens appear to be stuck to the object lines.

Note: Even when there is no parallax, the lines may appear to move near the edges of the lens because of lens aberrations. Concentrate on the part of the image seen through the centers of the lenses. Be sure that the eye looking at the object (the left eye in Figure 12.3) is looking directly at the object and not through the objective lens.

3. Record the position s of the lenses and the object in Table 12.1.

4. Estimate the magnification of your microscope by counting the number of object

squares that lie along one side of one image square. To do this, you must view the image through the microscope with one eye while looking directly at the object with the other eye. Remember that magnification is negative for an inverted image. Record the observed magnification in Table 12.1.

Lens Holder

Figure 12.4

Model No. OS-8459 Experiment 12: Microscope

Analysis

T o calculate the magnification complete the following steps and record the answers in Table 12.1:

1. Measure d

, the distance from the object (paper pat-

tern on screen) to the objective lens.

2. Determine d

, the distance from the eyepiece lens to

3. Calculate d

using do1 and the focal length of the

objective lens in the Thin Lens Formula (Equation

12.1).

4. Calculate d

by subtracting di1 from the distance

between the lenses.

5. Calculate the mag nificati on using Equation 12.2.

6. Calculate the percent difference between the calcu-

lated magnification and the observed value.

Questions

1. Is the image inverted or upright?

Table 12.1: Results

Position of Objective Lens Position of Eyepiece Lens Position of Screen Observed magnification

Calculated Magnification Percent Difference

2. Is the image that you see through the microscope real or virtual?

Further Study

Image Formed by the Objective Lens

Where is the image formed by the objective lens? Is it real or virtual? Us a desk lamp to brightly illuminate the paper grid (or replace the screen with the light source’s crossed-arrow object). Hold a sheet of paper vertically where you think the image is. Do you see the image? Is it inverted or upright? Remove the sheet of paper and hold a pencil in the same place. Look through eyepiece lens; you will see two images, one of the pencil and one of the grid pattern. Are both images inverted? Use parallax to determine the location of the pencil image.

Increasing Magnification

While looking through your microscope, move the objective lens a few centimeters closer to the object. Which way do you have to move the eyepiece lens to keep the image in focus. How close can you move the objective lens and still see a clear image? (Make a pencil mark on the paper grid so you have something very small to focus on.) What is the theoretical limit to how close you can move the objective lens?

Beginning Optics System Experiment 12: Microscope

Model No. OS-8459 Experiment 13: Shadows

Experiment 13: Shadows

Required Equipment from Beginning Optics System (2 systems needed)

2 Benches 2 Light Sources 1 Screen

Purpose

The purpose of this experiment is to show the umbra (darker part) and the penumbra (lighter part) of the shadow.

Set Up

1. Place the two optics benches beside each other.

2. Put one light source on each bench with the point source (circular hole) facing

the other end of the bench.

3. Place the screen on one of the benches at the opposite end to the light sources.

Procedure

1. Plug in only one of the light sources.

2. Hold a pencil about 5 cm away from the screen so its shadow is cast on the

screen. Now turn the light source around so the crossed-arrow illuminates the pencil and screen. How does the shadow change?

3. Rotate the light source back to the point-source position. Plug in the second light

source. Make a sketch of the shadow of the pencil. Label the umbra and the penumbra.

4. Move the pencil away and toward the screen. How does the shadow change?

5. Block the light from each point source in succession to determine which part of

the shadow is caused by each light source. Indicate your observation on your sketch.

Beginning Optics System Experiment 13: Shadows

Model No. OS-84 59 Telescope and Microscope Test Pattern

Telescope and Microscope Test Pattern

Attach a copy of this pattern to the viewing screen for experiments 11 and 12.

1 cm grid

Beginning Optics System Telescope and Microscope Test Pattern

Model No. OS-8459 Teac her’s Guide

Te a c h er’s Guide

Experiment 1: Color Addition

Note on procedure: Student’s expectation may differ from actual results. Encourage them to carefully

observe the resulting colors and describe them accurately.

Part 1, typical results:

Table 1.1: Results of Colored Light Addition

Colors Added Resulting Color

red + blue + green slightly bluish-white red + blue pink-purple red + green yellow-orange green + blue bluish-green

Part 1, answers to questions: 1. Mixing light is not the same as mixing paint. The mixing of colored light is additive mixing; the mixing of paint is subtractive mixing. 2. In this experiment the mixture of red, green, and blue does not look pure white to most people. To produce white li g ht , th e three colors must be present in a specific ratios of intensities.

Part 2, typical results:

Table 1.2: Colored Ink Observed Under Colored Light

Color of Light Line Apparent Color of Ink Do they look different? Actual Color of Ink

Blue Light

Red Light

ABlack

Yes, slightly

BBlack Black

CBlack

Yes, slightly

DBlack Black

Red

Blue

(Step 4) Under red light, black ink is easier to see than red; red ink appears nearly the same color as white paper.

Part 2: answers to questions: 1. Red ink appears red because it reflects red light and absorbs other colors. Under blue light, red ink absorbs most of the visible light. 2. Under red light, red ink is difficult to see because both ink and paper reflect most of the visible light.

Experiment 2: Prism

Notes on procedure: (Step 3) (a) Red, Orange, Yellow, Green and Blue are seen in that order. (b) Blue is

refracted at the largest angle.(c) Blue is predicted to refract at the largest angle because its index of refraction is largest. (Step 4) When colored rays enter the prism, they do not emerge parallel to each other because of their differing indices of refraction.

Beginning Optics System Teacher’s Guide

Experiment 3: Reflection

Part 1, typical results:

Table 3.1: Plane Mirror Results

Angle of Incidence Angle of Reflection

9.0° 9.2°

16.8° 16.5°

19.0° 37.8°

Part 1, answers to questions: 1. The angle of incidence and the angle of reflection are equal. 2. The three colored rays are not reversed by the mirror.

Part 2, typical results:

Table 3.2: Cylindrical Mirror Results

Concave Mirror Convex Mirror

Focal Length 6.2 cm 6.4 cm Radius of Curvature

(determined using compass)

13.3 cm 13.2 cm

The actual radius of both curved mirrors is about 12.5 cm.

Part 2, answers to questions: 1. The radius of curvature is twice the focal length for a cylindrical mirror . The typical experimental results confirm this. 2. The radius of curvature of a plane mirror approaches infinity.

Experiment 4: Snell’s Law

Typical results:

Table 4.1: Data and Results

Angle of Incidence Angle of Refraction Calculated index of refraction of

38.0° 26.0° 1.40

51.2° 33.8° 1.40

22.0° 14.4° 1.51 Average:1.44

(4% deviation from accepted value)

Answer to question: The ray leaves the rhombus at the same angle it entered.

acrylic

Experiment 5: Total Internal Reflection

Typical results:

(Step 5) Measured critical angle: θ (Step 6) Calculated critical angle: θ (Step 7) % Difference = 1.9%

Answers to questions: 1. The internally reflected ray becomes much brighter when the incident angle is larger than the critical angle. 2. The critical angle is greater for red light. This tells us that the index of refraction is smaller.

= 41.0°

= sin−1(1/n)=sin−1(1/1.5) = 41.8°

Model No. OS-8459 Teac her’s Guide

Experiment 6: Convex and Concave Lenses

Typical results:

Table 6.1: Results

Convex Lens Concave Lens

Focal Length 13.75 cm -12.1 cm

(Step 5) When the lenses are nested together, parallel rays entering the lenses emerge nearly parallel; this tells us that the focal lengths are of approximately equal magnitude and opposite sign. (Step 6) By moving the lenses apart, the spacing of the rays can be changed, but they remain nearly parallel.

Experiment 7: Hollow Lens

Typical results:

Table 7.1: Predictions and Observations

Lens

surrounded by:

Air

Water

Section 1

filled with:

Water Air Air diverging

Air Water Air converging Air Air Water converging

Water Air Water diverging

Air Water Water converging Water Air Water diverging Water Water Air diverging

Section 2

filled with:

Section 3

filled with:

Prediction

(converging or diverging)

Observation

(converging or diverging)

Answers to questions: 1. A plano-convex lens is converging when it has a higher index of refraction than

the surrounding medium. It is diverging when it has a lower index of refraction. 2. It is not possible to predict whether a plano-concave lens of unknown material will be diverging or converging under water because its index of refraction may be less than or greater than that of water.

Experiment 8: Lensmaker’s Equation

Typical results:

(Step 3) Measured focal length: f = −12.0 cm (Step 4) Measured focal distance of reflected rays: R/2 = 6.0 cm. Radius of curvature: R = −12.0 cm (Step 5) Calculated focal length:

n 1–()1 R⁄ 1 R⁄+()[]

1–

1.5 1–()1 12.0– cm()⁄ 1 12.0– cm()⁄+()[]

1–

12.1 cm–== =

(Step 6) % Difference: 0.8%

The actual radius of curvature or the lens is about −12.7 cm.

Beginning Optics System Teacher’s Guide

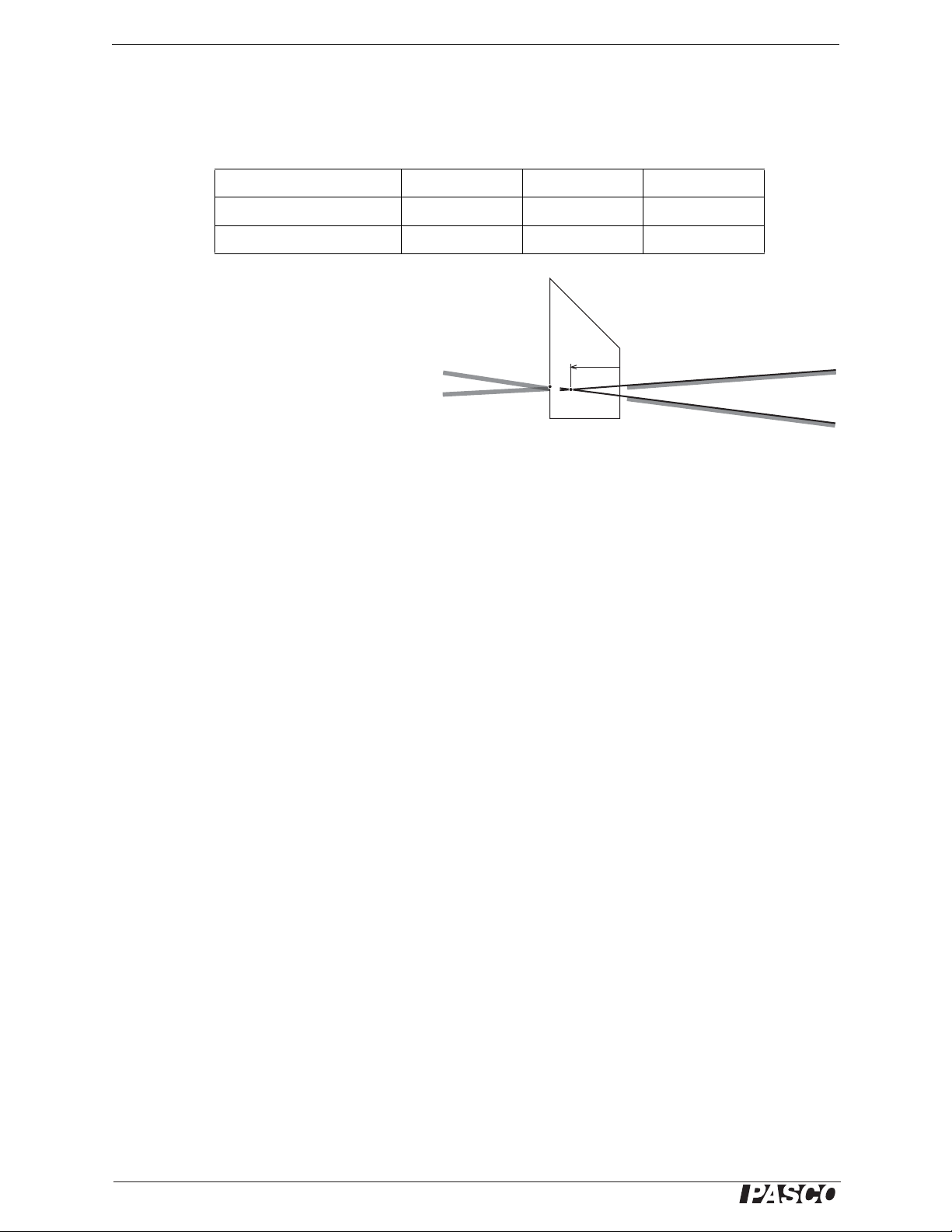
Experiment 9: Apparent Depth

Typical results:

Table 1.1: Results

dtn

Part 1: Parallax method 2.12 cm 3.18 cm 1.50 Part 2: Ray-tracing method 2.23 cm 3.18 cm 1.43

Typical ray-tracing results are represented at 50% scale in Figure TG.1. The gray regions represent the actual light beams; the black lines and dots represent the student’s actual marks. Notice that this student traced along the edges of the light beams.

The actual thickness of the rhombus is t = 3.175 ± 0.025 cm. Based on the accepted value of n = 1.49, the theoretical apparent depth is d =2.13.

2.23 cm

Figure TG.1

Answers to questions: 1. Of the two methods, the parallax method is the more precise. Using that method,

both d and t could be measured with a precision of less than 1 mm. Using the ray-tracing method, the points at which the rays crossed had a larger uncertainty due to the thickness of the light beams. 2. For the typical data above, the percent differences between the accepted and experimental values of n are 0.7% for Part 1 and 5% for Part 2.

Experiment 10: Focal Length and Magnification of a Thin Lens

Note on equipment: Provide students with the +100 mm mounted lens. Cover the focal length indicated on

the label. Other converging lenses will work, but you may have to modify the light source-to-screen values given in Table 10.1.

Part 1: For a distant object, 1/d distance of d

= f ≅ 10 cm.

approaches zero, therefore the image will form clearly with a lens-to-screen

Model No. OS-8459 Teac her’s Guide

Part 2: Typical results.

Table 10.1: Image and Object Distances

Distance from

light source to

screen

100 cm

90 cm

80 cm

70 cm

60 cm

50 cm

(cm)

88.5 11.5 0.0113 0.0870 5.5 mm 42 mm

11.0 89.0 0.0909 0.0112 81 mm 10 mm

78.3 11.7 0.0128 0.0855

11.3 78.7 0.0885 0.0127

68.0 12.0 0.0147 0.0833

11.5 68.5 0.0870 0.0146

57.7 12.3 0.0173 0.0813

11.9 58.1 0.0840 0.0172

47.1 12.9 0.0212 0.0775

12.3 47.7 0.0813 0.0210

36.0 14.0 0.0278 0.0714

13.4 36.6 0.0746 0.0273

y-intercept = 1/f = 0.0977 cm

x-intercept = 1/f = 0.103 cm

(cm)

-1

1/d

(cm

-1

)

1/d

-1

) Image Size Object Size

(cm

Table 10.2: Focal Length

Result from x-intercept 9.75 cm Result from y-intercept 10.2 cm % difference between results from intercepts 4.4% Average of results from intercepts 9.98 cm Result from Part I 10.0 cm % difference between Average of results from intercepts and result from Part I 0.2%

Table 10.3: Magnification

Point 1 Point 2

calculated from image and object distances -0.130 -8.09

calculated from image and object sizes 0.13 8.1

% difference 0% 0.1%

Answers to questions: 1. The image if inverted. 2. The image is real because it can be viewed on a screen.

3. For a given object-to-image distance, the two object distance-image distance pairs are the inverse of each other, which demonstrates the reversibility of light through a lens. 4. The magnification is negative because the image is inverted. 5. The three determined values of f are unlikely to be exactly equal, primarily due to measurement error.

Beginning Optics System Teacher’s Guide

Experiment 11: T elescope

Typical results:

Table 11.1: Results

Position of Objective Lens 63.4 cm Position of Eyepiece Lens 102.2 cm Position of Screen 0.0 cm Observed magnification -5

Calculated Magnification -4.9 Percent Difference 2%

63.4 cm

-102.2 cm

29.2 cm

9.6 cm

Answers to questions: 1. The image is inverted. 2. It is a virtual image.

Further study, Image Formed by the Objective Lens: The objective lens forms a real, upright image;

to see it, hold a sheet of paper at distance d

from the objective. When a pencil is placed at this location, it’s vir-

tual image, viewed through the eyepiece lens, coincides with the virtual image of the grid pattern viewed through both lenses.

Further study, Object at Infinity: When adjusted for a distant object, the distance between the lenses is equal to the sum of the focal lengths.

Experiment 12: Microscope

Typical results:

Table 12.1: Results

Position of Objective Lens 20.9 cm Position of Eyepiece Lens 54.9 cm Position of Screen 0.0 cm Observed magnification -3

Calculated Magnification -3.41 Percent Difference 12%

20.9 cm

-54.9 cm

19.2 cm

14.8 cm

Answers to questions: 1. The image is inverted. 2. It is a virtual image.

Further study, Image Formed by the Objective Lens: The objective lens forms a real, upright image;

to see it, hold a sheet of paper at distance d

from the objective. When a pencil is placed at this location, it’s vir-

Model No. OS-8459 Teac her’s Guide

tual image, viewed through the eyepiece lens, coincides with the virtual image of the grid pattern viewed through both lenses.

Further study, Increasing Magnification: As the objective lens is moved closer to the object, the eyepiece must be moved further away. In practice, the objective can be moved to within about 13 cm before distortion from lens aberrations becomes significant. The theoretical limit is 10 cm, or the focal length of the objective lens.

Experiment 13: Shadows

When the pencil is illuminated by the point source, the shadow appears sharper than when illuminated by a distributed light source (the crossed-arrow object). When illuminated by both point sources, the pencil casts two shadows. The area where the shadows overlap is the umbra. The areas of partial shadow are the penumbra. By moving the pencil toward the screen, the relative size of the umbra is increased. By moving the pencil away from the screen, the umbra is decreased until the two shadow separate entirely.

Beginning Optics System Teacher’s Guide

Model No. OS-8459 Technical Support

Technical Support

For assistance with any PASCO product, contact PASCO at: Address: PASCO scientific

10101 Foothills Blvd. Roseville, CA 95747-7100

Phone: 916-786-3800 (worldwide)

800-772-8700 (U.S.) Fax: (916) 786-3292 Web: www.pasco.com Email: support@pasco.com

Limited Warranty

For a description of the product warranty, see the PASCO catalog.

The PASCO scientific 012-09655A Beginning Optics System Instruction Manual is copyrighted with all rights reserved. Permission is granted to non-profit educational institutions for reproduction of any part of this manual, providing the reproductions are used only in their laboratories and classrooms, and are not sold for profit. Reproduction under any other circumstances, without the written consent of PASCO scientific, is prohibited.

Trademarks

PASCO and PASCO scientific are trademarks or registered trademarks of PASCO scientific , in the United States and/or in other countries. All other brands, products, or service names are or may be trademarks or service marks of, and are used to identify , products or services of, their respective owners. For more information visit www.pasco.com/legal.

Authors: Ann Hanks

Dave Griffith Alec Ogston

PASCO OS-8459 User Manual

Specifications and Main Features

Frequently Asked Questions

User Manual