PDSP16350
1
PDSP16350
I/Q Splitter/NCO
Supersedes version in December 1993 Digital Video & DSP IC Handbook, HB3923-1 DS3711 - 2.3 September 1996
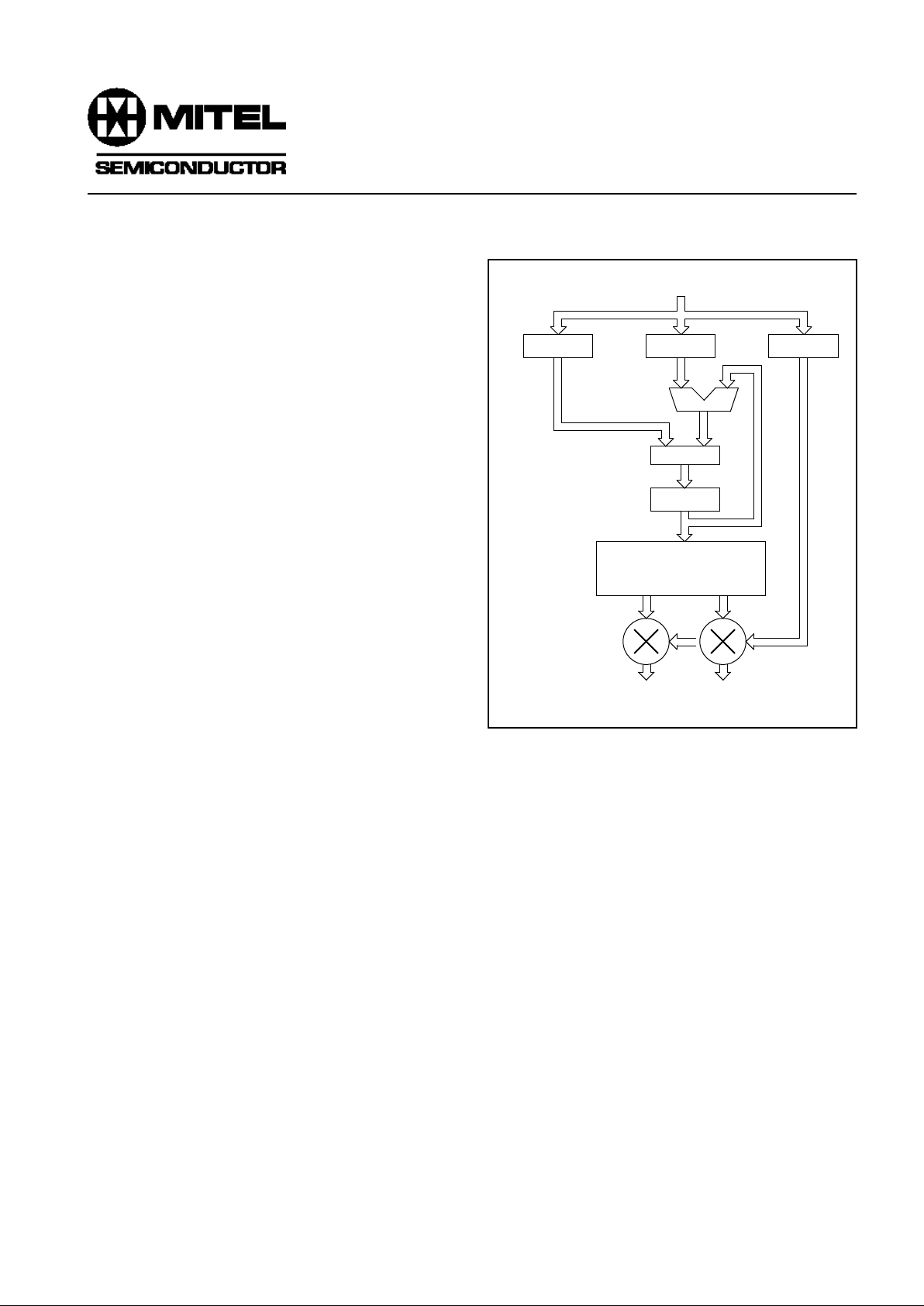
Fig. 1 Block Diagram
CORDIC PROCESSOR ARRAY
SIN COS
DIN
MUX
PHASE ACCUM
REGISTER
ACCUM
SCALING
REGISTER
PHASE OFFSET
REGISTER
PHASE INCR
REGISTER
The PDSP16350 provides an integrated solution to the
need for very accurate, digitised, sine and cosine waveforms.
Both these waveforms are produced simultaneously, with 16
bit amplitude accuracy, and are synthesised using a 34 bit
phase accumulator. The more significant bits of this provide
16 bits of phase accuracy for the sine and cosine look up
tables.
With a 20 MHz system clock, waveforms up to 10 MHz can
be produced, with 0.001 Hz resolution. If frequency modulation is required with no discontinuities, the phase increment
value can be changed linearly on every clock cycle. Alternatively absolute phase jumps can be made to any phase value.
The provision of two output multipliers allows the sine and
cosine waveforms to be amplitude modulated with a 16 bit
value present on the input port. This option can also be used
to generate the in-phase and quadrature components from an
incoming signal. This I/Q split function is required by systems
which employ complex signal processing.
FEATURES
■ Direct Digital Synthesiser producing simultaneous sine
and cosine values
■ 16 bit phase and amplitude accuracy, giving spur levels
down to - 90 dB
■ Synthesised outputs from DC to 10 MHz with accuracies
better than 0.001 Hz
■ Amplitude and Phase modulation modes
■ 84 pin PGA or 132 pin QFP
APPLICATIONS
■ Numerically controlled oscillator (NCO)
■ Quadrature signal generator
■ FM, PM, or AM signal modulator
■ Sweep Oscillator
■ High density signal constellation applications with simul-
taneous amplitude and phase modulation
■ VHF reference for UHF generators
■ Signal demodulator
ASSOCIATED PRODUCTS
PDSP16256/A Programmable FIR Filter
PDSP16510A FFT Processor
PDSP16488A 2D Convolver
PDSP16350
2
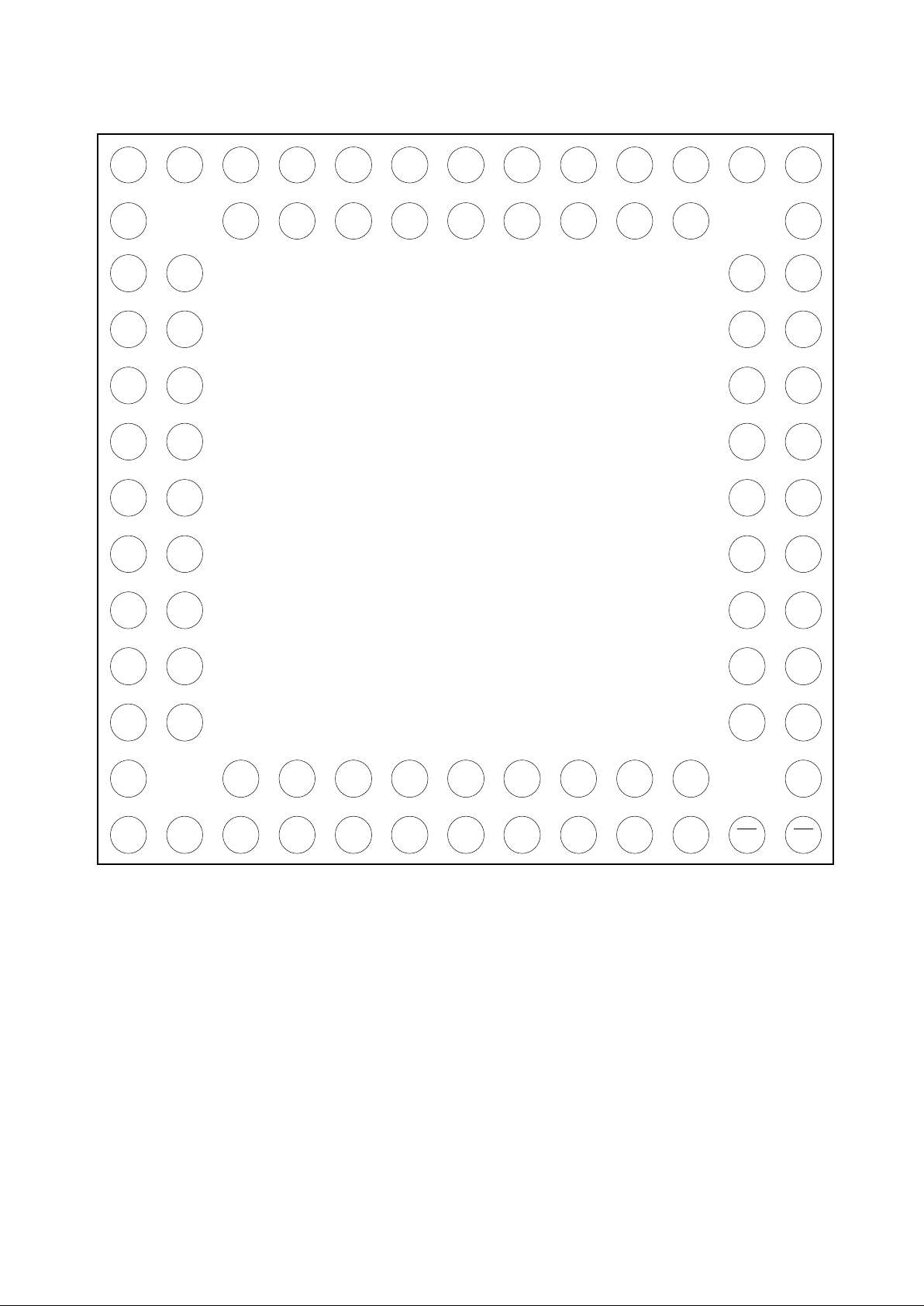
JUMP MODE DIN19 DIN21 DIN23 VDD DIN26 GND DIN28 DIN30 DIN32 VOUT RES
DIN17 DIN18 DIN20 DIN22 DIN24 DIN25 DIN27 DIN29 DIN31 DIN33 VIN
DIN15 DIN16 SIN15 SIN14
DIN13 DIN14 SIN13 SIN12
DIN11 DIN12 SIN11 SIN10
GND DIN10 SIN9 GND
DIN9 DIN8 SIN8 SIN7
VDD DIN7 SIN6 VDD
DIN6 DIN5 SIN4 SIN5
DIN4 DIN3 SIN2 SIN3
DIN2 DIN1 SIN0 SIN1
DIN0 COS15 COS13 COS11 COS9 COS7 COS6 COS4 COS2 COS0 CEN
CLOCK
GND COS14 COS12 COS10 VDD COS8 GND COS5 COS3 COS1 OES OEC
1 2 3 4 5 6 7 8 9 10 11 12 13
A
B
C
D
E
F
G
H
J
K
L
M
N
Fig. 2 A. Pin out - bottom view (84 pin PGA - AC84)
PDSP16350
3
GC
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
SIG
N/C
CEN
N/C
SIN0
SIN1
SIN2
GND
VDD
SIN3
SIN4
N/C
SIN5
SIN6
N/C
SIN7
SIN8
VDD
GND
SIN9
N/C
SIN10
SIN11
N/C
SIN12
SIN13
SIN14
VDD
GND
SIN15
VIN
N/C
N/C
RESET
GC
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
SIG
N/C
VOUT
DIN33
GND
VDD
DIN32
N/C
DIN31
DIN30
N/C
DIN29
DIN28
N/C
DIN27
GND
VDD
DIN26
DIN25
DIN24
DIN23
VDD
DIN22
GND
DIN21
VDD
DIN20
DIN19
GND
VDD
DIN18
MODE
JUMP
VDD
GC
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
SIG
GND
DIN17
N/C
DIN16
DIN15
GND
VDD
DIN14
DIN13
DIN12
N/C
DIN11
DIN10
N/C
DIN9
GND
VDD
DIN8
DIN7
DIN6
N/C
DIN5
N/C
DIN4
DIN3
VDD
GND
DIN2
DIN1
N/C
DIN0
N/C
CLK
GC
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
SIG
GND
VDD
GND
N/C
COS15
COS14
N/C
COS13
COS12
N/C
COS11
N/C
COS10
COS9
VDD
GND
COS8
COS7
N/C
COS6
COS5
N/C
COS4
N/C
COS3
COS2
N/C
COS1
VDD
GND
COS0
OES
OEC
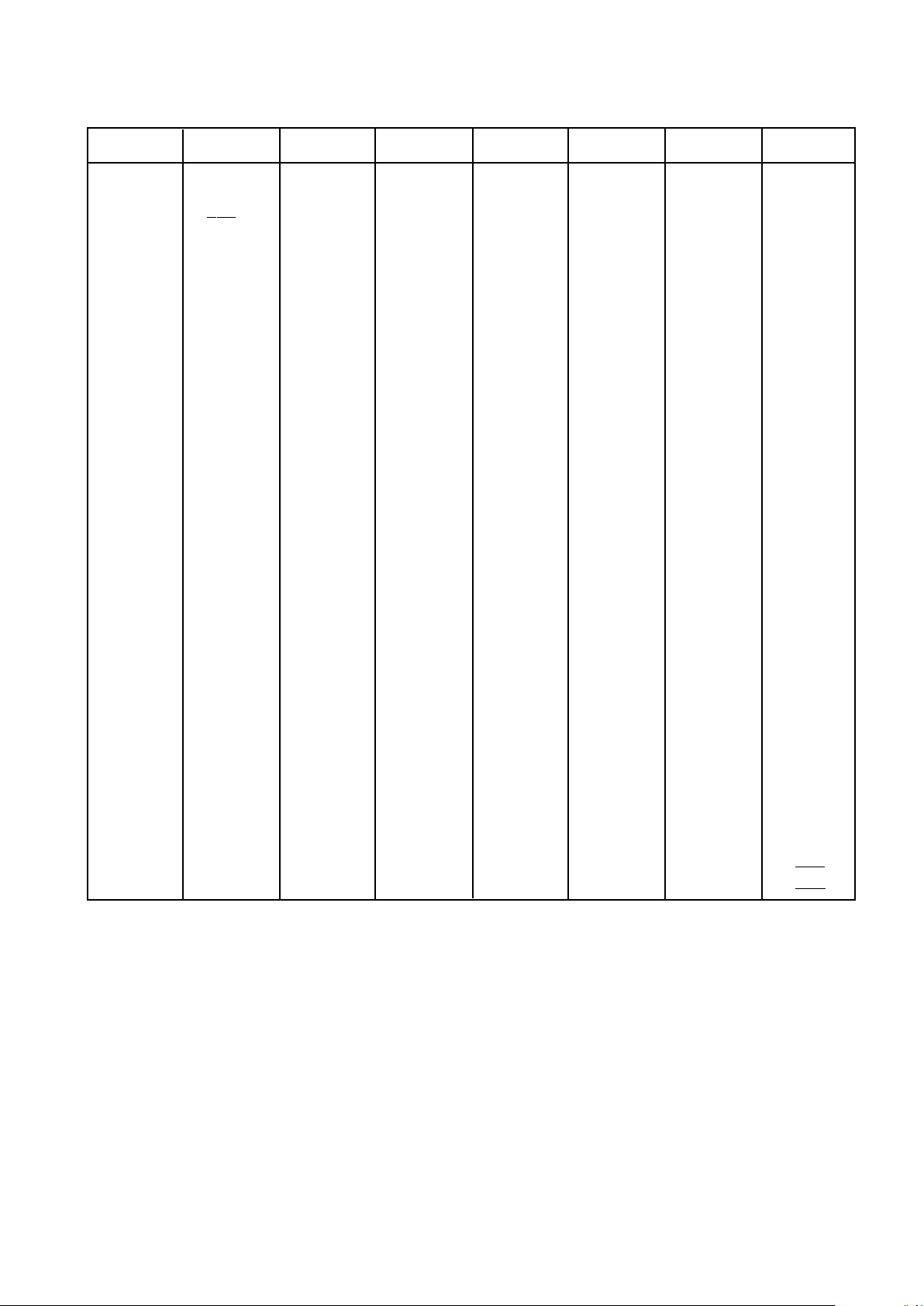
Fig.2B Pin out (132 pin ceramic QFP - GC132)
PDSP16350
4
SIGNAL DESCRIPTION
DIN33:0 Data bus for the input register. This input register provides a 34 bit, incremental or absolute, phase
value, if the mode pin is low. Alternatively if the mode pin is high, it provides either an 18 bit phase
increment value, via D17:0, and a 16 bit scale value via D33:18 or a 34 bit phase increment value
depending on the JUMP input see below.
SIN15:0 16 bit sine output data in fractional two’s complement format.
COS15:0 16 bit cosine output data in fractional two’s complement format.
CEN Clock enable for the data input register. When low, data will be latched on the rising edge of the clock.
When high data will be retained in the input register.
MODE Mode control input. When low, data in the input register is interpreted as either a 34 bit phase increment
value or a 34 bit absolute phase value. When high, the output multipliers are enabled and will scale the
waveforms with the upper 16 bits in the input register. The phase increment is loaded from the the lower
18 bits. The full 34 bit phase increment register can also be loaded using JUMP see below.
JUMP With MODE low (Frequency or Phase Modulation)
When low JUMP will allow normal phase incrementing to occur. When high, the data on the input pins
will be interpreted as a 34 bit absolute phase value to replace the present value in the accumulator.
JUMP is internally latched to match the delay through the data input register, and to allow data in the
internal pipeline to be correctly processed. CEN must also be low to latch the required data from DIN.
When Mode is high (Amplitude Modulation)
When low JUMP will allow normal phase incrementing to occur, with the phase increment value taken
from the lower 18 data inputs. When high, the data on the input pins will replace the full 34 bits of the
phase increment register. CEN must also be low to latch the required data.
RES When high will clear the phase accumulator and phase increment registers, after data in the internal
pipeline has been correctly processed.
CLK Input clock.
OES Output enable for SIN 15:0. Outputs are high impedance when OES is high.
OEC Output enable for COS15:0. Outputs are high impedance when OEC is high.
VIN Valid input flag. A delayed version of this input is available on the VOUT pin, with the delay matching
the data processing pipeline delay. This input has no other internal function.
VOUT Valid output flag. See above.
GND Five ground pins. All must be connected.
VCC Four +5V pins. All must be connected.
Table 1. Pin Description
PDSP16350
5
4) DC can be generated since the increment value can be
zero.
5) Frequency stability will match the stability of the incoming
frequency when the increment is fixed.
The residual noise characteristics of an oscillator are very
important in modern communication systems. This parameter
defines how well the device maintains its set frequency for
very short periods (nanoseconds to seconds) of time. Poor
figures will significantly affect the system signal to noise ratio
and limit the dynamic range.
The PDSP16350 will, of course, inherit the residual noise
characteristics of the source of the incoming frequency. The
output frequency is, however, always less than half the
incoming frequency in order to satisfy the Nyquist criterion.
This is in contrast to a phase locked loop synthesiser, when a
small input frequency controls a high output frequency.
The commonly used 20 log N rule states that the phase
noise at the output of a synthesiser will be no better than
twenty times the log of the ratio of the output frequency to the
input frequency. In a phase locked loop synthesiser N is large,
in the PDSP16350 it is less than half. Log N is thus less than
zero and phase noise improvement is obtained.
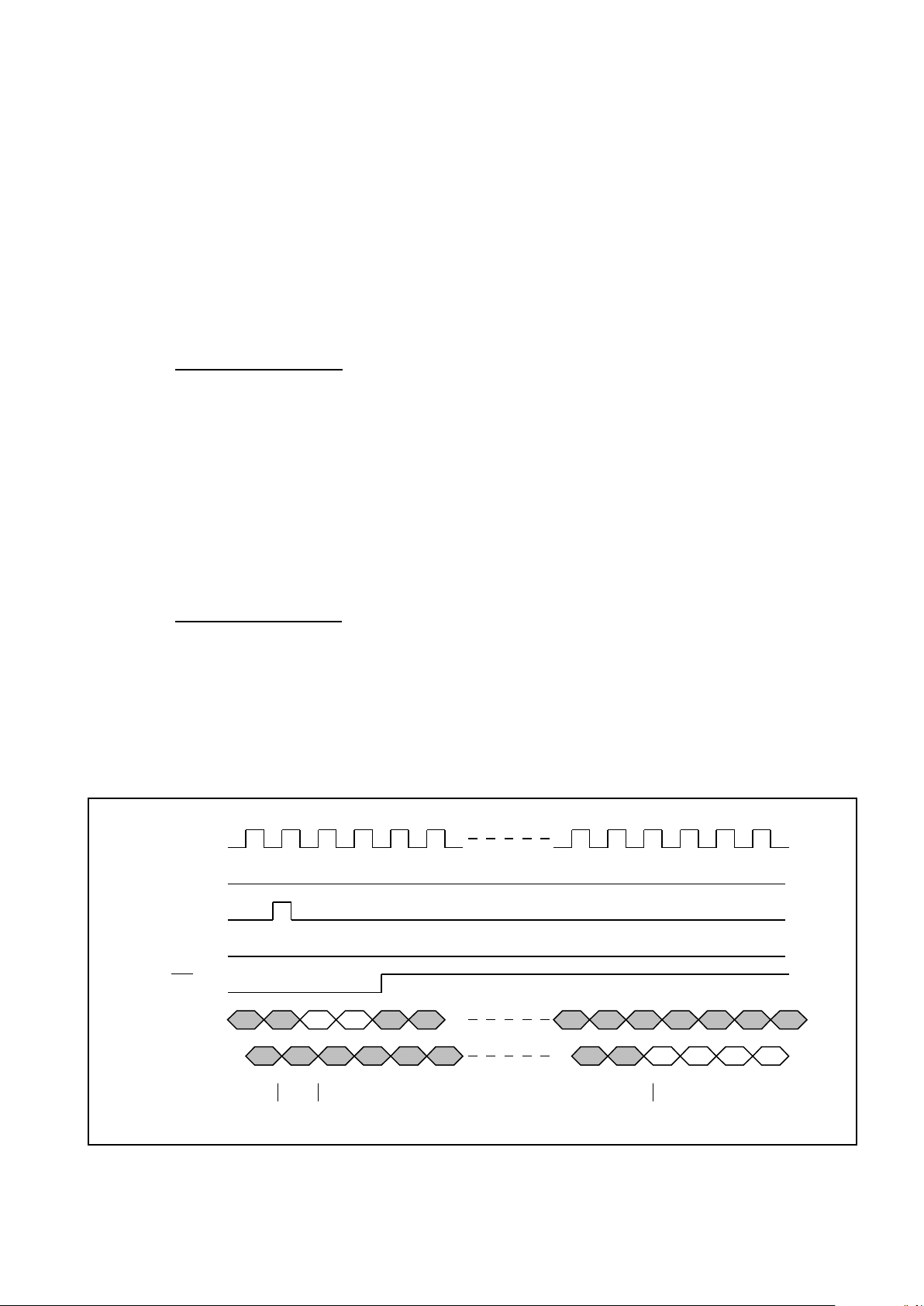
The output waveforms are produced after a pipeline delay
with respect to the DIN inputs. The effects of the JUMP or RES
commands are delayed such that all data in the internal pipe
will be processed before the discontinuity occurs. New data
may be presented to the device on the cycle following the
JUMP or RES and a valid result will be obtained after 31 clock
cycles.
DEVICE OPERATION
Sine and cosine are simultaneously produced by the
Cordic processor, which is addressed by the upper 16 bits of
the output from a 34 bit phase accumulator. The accumulator
divides the digital phase circle into a number of steps, one step
for each state of the accumulator. When the accumulator
reaches its maximum value it overflows back to zero and the
sequence is repeated.
The accumulator is incremented once per incoming clock
cycle, by an amount which defines the frequency which is to
be generated. The increment required is defined by :
Desired O/P Frequency
Increment = x 2
N
Incoming Clock Frequency
where N is the number of bits in the accumulator. Since the
Nyquist criteria for proper waveform reconstruction must still
be obeyed, the maximum output frequency is half the incoming frequency. In practice, when a return is made to the analog
world, just meeting the minimum Nyquist requirement would
require a ‘brick wall’ low pass filter to remove the alias signals.
A more useful ‘rule of thumb’ is to limit the generated waveforms to less than 40% of the clock frequency.
The resolution, or tuning sensitivity, of the waveform
generator is given by :
Incoming Clock Frequency
Resolution = Hz
2
N
These equations illustrate some very important features of
direct digital synthesisers :-
1) Tuning sensitivity is defined by both the number of bits in
the accumulator and the incoming time base frequency.
2) The oscillator tunes linearly over its entire range.
3) The frequency accuracy matches the accuracy of the
incoming increment value.
1 2 3 4 5
CLK
RESET
DATA IN
RESULT
30 31 32 33 34 35
PI PI
A B
Device Reset Apply phase increment First Result Available
C D
JUMP
MODE
CEN
Fig. 3 Fixed Frequency Timing Diagram
 Loading...
Loading...