Mettler Toledo 33360, 210260 Operating Instructions Manual
METTLER TOLEDO 33360 + 210260 1
Operating instructions
Bedienungsanleitung
Mode d'emploi
Instrucciones de manejo
Istruzioni per l'uso
METTLER TOLEDO
33360
210260
Density determination kit Page 3
to determine the density of solids (33360) and liquids (33360/210260) with
top-loading electronic METTLER TOLEDO balances with pan diameters of 80 and 130 mm
Dichtebestimmungszusatz Seite 9
für die Dichtebestimmung von Festkörpern (33360) und Flüssigkeiten (33360/210260)
auf oberschaligen elektronischen METTLER TOLEDO-Waagen mit Schalendurchmesser 80 und 130 mm
Accessoires pour la détermination de la masse volumique Page 15
des solides (33360) et des liquides (33360/210260) à l'aide de balances
électroniques METTLER TOLEDO à plateau supérieur ayant un diamètre de 80 et 130 mm
Conjunto de determinación de densidades Página 21
para la determinación de densidades de sólidos (33360) y de líquidos (33360/210260) sobre
balanzas electrónicas METTLER TOLEDO de platillo elevado con diámetros de platillo de hasta 80 y 130 mm
Kit per la determinazione della densità Pagina 27
di solidi (33360) e liquidi (33360/210260) con l'ausilio di bilance
elettroniche METTLER TOLEDO con piatto di pesata superiore del diametro di 80 e 130 mm
2 METTLER TOLEDO 33360 + 210260
LEERSEITE
33360
210260
Density Determination of Liquids
Dichtebestimmung von Flüssigkeiten
Détermination de la masse volumique de liquides
Determinación de densidades de liquidos
Determinazione della densità dei liquidi
33360
Density Determination of Solids
Dichtebestimmung von Festkörpern
Détermination de la masse volumique de solides
Determinación de densidades de sólidos
Determinazione della densità dei solidi
METTLER TOLEDO 33360 + 210260 3
Density determination kit
to determine the density of solids and liquids with top-loading electronic METTLER TOLEDO balances with pan
diameters of 80 and 130 mm.
- for the density determination of solids, density determination kit 33360.
- for the density determination of liquids, density determination kit 33360
+ displacement body 210260.
1. Definition of density
- to determine the density of solids and liquids with top-loading electronic METTLER TOLEDO balances with
pan diameters of 80 and 130 mm.
The density ρ of an homogeneous body is the relationship of its mass (m) to its volume (V) or its mass per unit
of volume.
m [g] ρ = Density
ρ = m = Mass
V [cm3] V = Volume
According to DIN (German Engineering Standards) 1305, the term "Weight" can be used instead of "Mass" (m).
2. Principle of density determination
According to the Archimedean Principle, a solid body immersed in a liquid apparently loses as much of its own
weight as the weight of the liquid it has displaced. This makes it possible to determine the unknown value.
Depending on whether the body in question is liquid or solid, a slightly different procedure is involved.
The density of a liquid is determined by using a sinker of known volume. To this end, the sinker is first weighed
while in air, and then while immersed. From these two weighings (made in grams), density ρ1 of the liquid is
calculated as follows:
As - B
s
ρ1 =+ Lsρ1= Density of liquid to be tested at a given
V
s
temperature T
As= Weight of sinker in air
Bs= Weight of sinker when immersed in liquid
or simply Vs= Volume of sinker
Ls= Air buoyancy per ml of sinker (correction of As or
Ps): about + 0,001 g/ ml; see also Section 3.2,
P
s
Air Buoyancy
ρ1 =+ LsPs= Buoyancy of sinker in liquid
V
s
(instead of As - Bs): can be read directly on
METTLER TOLEDO electronic balances; see
Section 4.1
The density of a solid body is determined by using a liquid of known density. To this end, the solid body is first
weighed in air and then immersed. From these two weighings (made in grams or in carats), density ρ2 is calculated
as follows:
A
ρ2 =• ρ
o
ρ2= Density of solid body
A - B A = Weight of solid body in air
B = Weight of solid body when immersed in test liquid
or simply ρo= Density of test liquid at a given temperature T
P = Buoyancy of solid body in test liquid (instead of
A A - B): can be read directly on METTLER TOLEDO
ρ2 =• ρ
o
electronic balances; see Section 4.2
P
The air buoyancy of a solid body is not always taken into account. If necessary, result ρ2 can be corrected by about
+ 0.001 g/ cm3 similar to what was done in the first case; see also Section 3.2, Air Buoyancy.
4 METTLER TOLEDO 33360 + 210260
3. Accuracy of density determination
The required accuracy of the result determines whether any factors will be taken into account. The following listing
should make it possible to quantitatively evaluate the influence of these factors.
3.1 Temperature
In the case of solid bodies, the change in density caused by a change in temperature is generally so small that
the temperature of a solid body can be disregarded as far as density determination is concerned (this also applies
to sinkers).
In the case of liquids, however, the density may change on the order of 0.1 … 1 %o pro °C, and this may already
appear at the third decimal place.
Example: Distilled water: Density change about 1 %o per 5 °C
Hydrocarbons + alcohols: Density change about 1 %o per 1 °C
It should be noted that such a change in the density of a liquid is directly taken into account when the density of
solid bodies is being determined because of the test liquid in which the solid body has to be immersed. For this
reason, the temperature of the liquid must always be taken into consideration when the density is to be
determined with better than 1 % accuracy.
Density table for distilled water:
(according to "Handbook of Chemistry and Physics" 66th Ed. 1985-1986, F4-F5)
Temperature (°C) Density (g/ ml) Temperature (°C) Density (g/ ml)
15.0 0.9991 24.0 0.9973
15.5 0.9990 24.5 0.9972
16.0 0.9990 25.0 0.9970
16.5 0.9989 25.5 0.9969
17.0 0.9988 26.0 0.9968
17.5 0.9987 26.5 0.9966
18.0 0.9986 27.0 0.9965
18.5 0.9985 27.5 0.9964
19.0 0.9984 28.0 0.9962
19.5 0.9983 28.5 0.9961
20.0 0.9982 29.0 0.9959
20.5 0.9981 29.5 0.9958
21.0 0.9980 30.0 0.9956
21.5 0.9979 30.5 0.9955
22.0 0.9978 31.0 0.9953
22.5 0.9977 31.5 0.9952
23.0 0.9975 32.0 0.9950
23.5 0.9974
For all other liquids, the density at temperature T must be taken from a book of tables.
METTLER TOLEDO 33360 + 210260 5
3.2 Air Buoyancy
Depending on its physical conditions, each cubic centimeter (cm3 ) of air weighs 1 … 1.2 mg. Thus, any object
that is being weighed in air is subject to this kind of buoyancy for each cm3 of its volume. This means that – with
a density of 1 g/ cm3 – an error of approximately 0.1 % would occur if the air buoyancy is not taken into
consideration. If a result with 3 or 4 decimal places is required, the result will have to be corrected for air buoyancy.
The true density is about 0.001 g/ cm3 more than the calculated density.
When the density of liquids is determined, the operating procedure (division by 10 ml, corresponding to the
volume of the sinker), provides a fourth-place result, even if the balance display shows only a three-place
indication. It is therefore advisable – in this case – to make a general buoyancy correction.
3.3 Volume tolerance of the float
German Weights and Measures Regulation EO 13-4 paragraph 9.21
The volume of the float, together with the lower portion of the suspension wire, must be adjusted so that a 30 g
float arrangement does not produce a measuring error exceeding ± 0.00059 g/ cm3 when determining the water
density at a temperature of 20 °C.
3.4 Immersion depth of the sinker or of the gem holder
The sinker (immersion body) is suspended from a platinum wire which has a diameter of 0.2 mm. In the water,
the wire thus experiences a buoyancy of 0.3 mg when 10 mm of the wire are immersed.
If the fluid level stands 10 mm above the eylet of the sinker, about 20 mm of the wire are immersed. With a density
(of liquid) of about 1, this would result in a buoyancy of 0.6 mg. But since this would again have to be divided by
10 ml, this influence can be disregarded.
The submersible part of the gem holder is made of a wire that has a diameter of 0.8 mm. With a liquid density
of approx. 1, this results in a buoyancy of about 5 mg for each 10 mm that is submerged. But since the gem holder
remains submerged while the solid body is weighed in air, and since – with electronic balances – the immersion
depth does not change from one weighing to the next (in spite of the difference in weight), the buoyancy of the
gem holder remains constant and can thus be disregarded. Condition: Do not change the liquid level. (The change
of the liquid level caused by submerging the solid body can – in most cases – be disregarded. A solid body with
a volume of 1 cm3 causes the liquid to rise by about 0.5 mm. This is equivalent to a buoyancy of about 0.15 mg,
i.e. a density error of 0.15 mg/ cm3).
3.5 Surface tension of liquid
Since the liquid adheres to the suspension wire, an apparent weight increase occurs. In the case of the sinker
(wire diameter 0.2 mm), and when water is used as the liquid, this force amounts to about 1 mg. By using wetting
agents or organic liquids, this force can be reduced to 0.3 mg. However, this value will also be divided by 10 ml
so that the resulting density error amounts to no more than 0.0001 g/ ml.
Because of the larger diameter of the wire submerged in the water, a force of up to 3 mg acts upon the gem holder.
Here, too, similar to what is described in Section 3.4, any influence on the result will be virtually eliminated by
having the gem basket submerged during both weighings (A and B). For very high accuracy requirements,
reducing the surface tension would constitute an additional precautionary measure (see also Section 3.6).
6 METTLER TOLEDO 33360 + 210260
3.6 Air bubbles
With poorly wetting liquids such as water without wetting agents, for example, it is possible that air bubbles will
adhere to the submerged solid body, sinker or wire basket of gem holder. Because of their buoyancy, these
bubbles could affect the result. Thus, a bubble 1 mm in diameter would cause a buoyancy of 0.5 mg, while a bubble
with a diameter of 2 mm would result in a buoyancy of as much as 4 mg.
Precautionary measures:
- Degrease solids that are resistant to solvents.
- Periodically clean gem holder and sinker; do not touch immersible parts with your hands.
- Gently shake gem holder when first immersing it in liquid, before suspending it from hook, so as to loosen air
bubbles that might stick to it.
- Use wetting agents or organic solvents as auxiliary liquid (e.g. Kodak Photo-Flo, Pervitro 75% 72409).
(The change of density caused by the addition of a wetting agent to distilled water is negligible. If, for
example, 0.1 ml wetting agent with a density of 1.2 is added to 250 ml of water, the overall density changes
by 0.001 g/ml.)
3.7 Porous bodies
Since the submersion of porous bodies does not generally cause a one hundred percent displacement of air within
the pores by the liquid, errors will occur as a consequence. Thus the density of the body can only be approximated.
METTLER TOLEDO 33360 + 210260 7
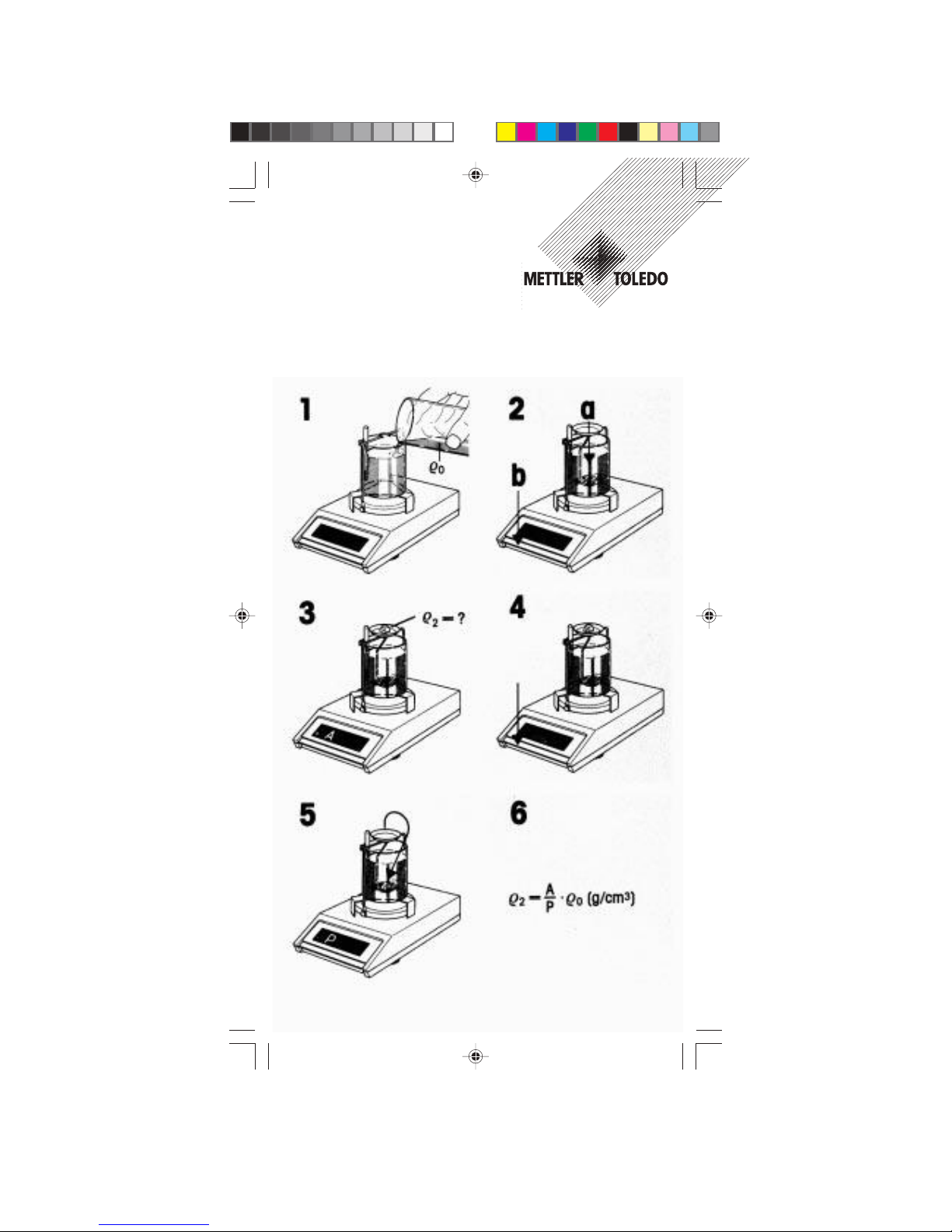
4. How to perform density determinations
Arrangement
1 Weighing pan of METTLER TOLEDO balance
2 Bracket attached to weighing pan …
3 … clamping screws hold bracket in place
4 Bridge placed on balance housing across weighing pan.
Warning: Weighing pan 1 and bracket 2 must not brush
against bridge:
5 250 ml beaker placed on bridge
6 Sinker or gem holder …
7 … suspension; the sinker or gem holder should hang
freely in center of beaker without touching.
Please note: The first weighing is dry!
8 Thermometer
The listing of the various parts is repeated on page 33 (Parts
List)
Reminders:
- It is recommended to check the calibration.
- Along with the text below, please check the illustrated instructions which are enclosed with this description.
4.1 Determining the density of liquids
- Use displacement body 210260 + density determination kit for liquids 33360.
- Place empty beaker on bridge 4, centered below suspension 7; insert thermometer 8.
- Attach sinker 6. It must not touch the beaker or the thermometer.
- Tare balance to show exactly zero.
- Pour liquid to be tested into beaker up to about 10 mm above the eyelet of the sinker. No air bubbles should
adhere to the sinker. If necessary, brush off bubbles with a fine brush or the like.
- The balance displays (with minus sign in front) buoyancy Ps of the sinker. Value Ps displayed by the balance
must now be divided by 10. After that, add 0.001 g/ ml.
The result is density ρ1 (g/ ml) at a given temperature T (to be read).
P
s
Ps [g]
ρ1 =+ L
s
= + 0.001 g/ ml at T °C (Ps = As - Bs)
V
s
10 ml
If the direct buoyancy indication is to be dispensed with, Ps can be obtained from two separate weighings: As =
sinker in air; Bs = sinker in liquid. Procedure is the same as above, but the balance is tared before the sinker is
attached. With the sinker attached, the balance then indicates value As. After the liquid is filled in, the balance
indicates (without additional taring!) value Bs.
Example with buoyancy indication: Determining the density of acetone.
7.898 g
Measured Ps= 7.898 g ρ
1
+ 0.001 g/ ml = 0.7908 g/ ml at 20 °C
values: T = 20 °C 10 ml
The density of the acetone tested amounts to 0.7908 g/ ml at 20 °C.
8 METTLER TOLEDO 33360 + 210260
4.2 Determining the density of solids
- Use density determination kit for solids (= gem holder 6) 33360.
- Pour enough auxiliary liquid into beaker so that the solid body after being placed in wire basket of gem holder
will be covered with at least 10 mm of liquid. Insert thermometer. Place beaker on bridge 4 centrally located
below the suspension 7.
- Attach gem holder (watch for air bubbles, especially at the wire basket).
- Tare balance to read exactly zero.
- Place dry solid in upper cup of gem holder. Write down weight displayed by the balance (in grams or carats).
This is weight A of the solid when weighed in air.
- Again tare balance (with solid body in cup).
Balance display indicates exactly zero.
- Remove solid body from cup and place it in lower wire basket (in liquid).
Balance now displays buoyancy P of solid body (with minus sign in front).
Divide first indication A by second indication P, then multiply this intermediate result by the density ρ0 of the
auxiliary liquid (at the given temperature T).
The final result is density ρ2 of the solid body. The unit of measurement is the same as that used for density
ρo of the auxiliary liquid, e.g. g/ cm3 (identical to g/ ml).
A A [g] Since the units of A an P cancel each other, the
ρ2 =• ρo=• ρo [g/ cm3] weighing can be performed just as easily in carats
P P [g] as in grams. Result ρ2 always has the same unit of
measurement as result ρo.
If the direct buoyancy reading is to be dispensed with, P can also be obtained from A - B. The balance will display
B instead of P if it has not been tared after result A was displayed or if it was tared with the solid body removed.
Example with buoyancy indication: Determining the density of a coin with the help of distilled water.
Measurement A = 3.011 g 3.011
values: P = 0.336 g ρ2 = • 0.997 g/ cm3 = 8.93 g/ cm
3
T = 25.5 °C →ρo = 0.997 g/ cm
3
0.336
The coin has a density of 8.93 g/ cm3.
METTLER TOLEDO 33360 + 210260 9
Dichtebestimmungszusatz
für die Dichtebestimmung von Festkörpern und Flüssigkeiten auf oberschaligen elektronischen
METTLER TOLEDO-Waagen mit Schalendurchmessern 80 und 130 mm.
- für die Dichtebestimmung von Festkörpern Dichtebestimmungs-Kit 33360.
- für die Dichtebestimmung von Flüssigkeiten Dichtebestimmungs-Kit 33360
+ Verdrängungskörper 210260.
1. Definition der Dichte
- für die Dichtebestimmung von Festkörpern und Flüssigkeiten auf oberschaligen elektronischen
METTLER TOLEDO-Waagen mit Schalendurchmessern 80 und 130 mm.
Die Dichte ρ eines homogenen Körpers ist das Verhältnis seiner Masse (m) zu seinem Volumen (V) oder,
gleichbedeutend, die Masse einer Volumeneinheit:
m [g] ρ = Dichte
ρ = m = Masse
V [cm3] V = Volumen
Anstelle der Masse (m) kann gemäss DIN 1305 der Begriff “Gewicht” verwendet werden.
2. Prinzip der Dichtebestimmung
Gemäss dem Archimedischen Prinzip verliert ein in eine Flüssigkeit getauchter Körper soviel an Eigengewicht,
wie das Gewicht der von ihm verdrängten Flüssigkeit beträgt. Damit lässt sich die gesuchte Grösse bestimmen.
Das Vorgehen bei Flüssigkeiten und Festkörpern ist etwas unterschiedlich.
Die Dichte einer Flüssigkeit wird mit Hilfe eines Senkkörpers bestimmt, dessen Volumen bekannt ist. Dazu
wiegt man diesen Senkkörper einmal in Luft, sodann in eingetauchtem Zustand. Aus den beiden Wägungen (in
Gramm) errechnet sich die Flüssigkeitsdichte ρ1 wie folgt:
As - B
s
ρ1 =+ Lsρ1= Dichte der zu prüfenden Flüssigkeit bei der
V
s
gegebenen Temperatur T
As= Gewicht des Senkkörpers in Luft
Bs= Gewicht des Senkkörpers in die Flüssigkeit
getaucht
oder einfacher Vs= Volumen des Senkkörpers
Ls= Luftauftrieb pro ml des Senkkörpers
(Korrektur von As bzw. Ps):
P
s
ca. + 0,001 g/ ml; siehe auch 3.2, Luftauftrieb
ρ1 =+ LsPs= Auftrieb des Senkkörpers in der Flüssigkeit
V
s
(anstelle von As - Bs): auf elektronischen
METTLER TOLEDO-Waagen direkt ablesbar,
siehe 4.1
Die Dichte eines Festkörpers wird mit Hilfe einer Flüssigkeit bestimmt, deren Dichte bekannt ist. Dazu wiegt
man den Festkörper einmal in Luft, sodann in eingetauchtem Zustand. Aus den beiden Wägungen (in Gramm
oder Karat) errechnet sich die Dichte ρ2 wie folgt:
A
ρ2 =• ρ
o
ρ2= Dichte des Festkörpers
A - B A = Gewicht des Festkörpers in Luft
B = Gewicht des Festkörpers in die
oder einfacher Hilfsflüssigkeit getaucht
ρo= Dichte der Hilfsflüssigkeit bei der gegebenen
A Temperatur T
ρ2 =• ρ
o
P = Auftrieb des Festkörpers in der Hilfsflüssigkeit
P (anstelle von A - B): auf elektronischen
METTLER TOLEDO-Waagen direkt ablesbar,
siehe 4.2
Der Luftauftrieb wird bei Festkörpern nicht durchgehend berücksichtigt. Bei Bedarf kann, wie im ersten Fall, am
Resultat ρ2 die Korrektur von ca. + 0,001 g/ cm3 angebracht werden; siehe auch 3.2, Luftauftrieb.
10 METTLER TOLEDO 33360 + 210260
3. Genauigkeit der Dichtebestimmung
Die allfällige Berücksichtigung von Faktoren, welche das Resultat beeinflussen können, richtet sich stets nach
der geforderten Resultatgenauigkeit. Die folgende Aufstellung im einzelnen soll eine quantitative Wertung dieser
Einflüsse erlauben.
3.1 Temperatur
Bei Festkörpern ist die Veränderung der Dichte durch Temperaturänderung im allgemeinen so gering, dass ihre
Temperatur für die Dichtebestimmung belanglos ist (dies gilt auch für den Senkkörper).
Bei Flüssigkeiten hingegen liegt die Dichteänderung in der Grössenordung von 0,1 … 1 %o pro °C, kann also
schon bei der dritten Nachkommastelle in Erscheinung treten.
Beispiele: destilliertes Wasser Dichteänderung ca. 1 %o auf 5 °C
Kohlenwasserstoffe und Alkohole Dichteänderung ca. 1 %o auf 1 °C
Es ist zu beachten, dass diese Dichteänderung von Flüssigkeiten auch bei Dichtebestimmungen von Festkör-
pern direkt ins Resultat eingeht wegen der Volumenbestimmung des Festkörpers mittels einer Hilfsflüssigkeit,
in die er getaucht wird. Bei Dichtebestimmungen von besser als 1 % Genauigkeit ist daher die Temperatur der
Flüssigkeit stets zu berücksichtigen!
Dichtetabelle für destilliertes Wasser:
(gemäss "Handbook of Chemistry and Physics" 66th Ed. 1985-1986, F4-F5)
Temperatur (C°) Dichte (g/ml) Temperatur (C°) Dichte (g/ml)
15,0 0,9991 24,0 0,9973
15,5 0,9990 24,5 0,9972
16,0 0,9990 25,0 0,9970
16,5 0,9989 25,5 0,9969
17,0 0,9988 26,0 0,9968
17,5 0,9987 26,5 0,9966
18,0 0,9986 27,0 0,9965
18,5 0,9985 27,5 0,9964
19,0 0,9984 28,0 0,9962
19,5 0,9983 28,5 0,9961
20,0 0,9982 29,0 0,9959
20,5 0,9981 29,5 0,9958
21,0 0,9980 30,0 0,9956
21,5 0,9979 30,5 0,9955
22,0 0,9978 31,0 0,9953
22,5 0,9977 31,5 0,9952
23,0 0,9975 32,0 0,9950
23,5 0,9974
Für andere Flüssigkeiten muss die Dichte bei Temperatur T einem Tabellenbuch entnommen werden.
Loading...