Leupold RANGING SYSTEM User Manual

Ranging System

For The

Mil Dot Reticl

OPERATING INSTRUCTIONS

e

Part # 54517

Artwork # 54516 Rev. C

www.leupold.com

The Leupold® Mil Dot Reticle

The Leupold® Mil Dot reticle employs a system based on the subtension of one
milliradian (mil) from the center of one dot to the center of the next. This is
also the distance between the crosshairs and the first dot.

The subtension of 1 mil equals 3.6 inches at 100 yards or 36 inches at 1,000
yards. In metric units, the correspondence is 1 mil equals 10 centimeters at 100
meters or 1 meter at 1,000 meters. Knowing this subtension and knowing the
size of the target (or a reference object near the target) allows the distance to
the target to be estimated with considerable accuracy.

FIRST VERSUS SECOND FOCAL PLANE

In variable-magnification optics, the magnification setting for the use of the
mil dot reticle is determined by whether the reticle is in the first or second
focal plane. The easiest way to determine if the mil dot reticle is in the first
focal plane is to view the reticle through the scope while changing the
magnification setting. If the size of the reticle appears to change as the
magnification setting is changed, the reticle is in the first focal plane. If it
does not appear to change size, it is in the second focal plane.

1

2

If the reticle is in the first focal plane, no specific magnification setting
is required to obtain the proper subtension to yield accurate range
estimating results.

When the Leupold Mil Dot reticle is installed in the second focal plane of
an optic, it is calibrated to a specific magnification for range estimating
purposes. In a variable-magnification scope, this is generally the highest
magnification setting and all range estimating must be performed at this
setting. However, in certain scopes it may be calibrated for a different
setting. If you are uncertain to what setting your scope is calibrated,
contact Leupold Technical Service.

THE USE OF A MIL DOT RETICLE

To use the Mil Dot reticle, you must know the actual size of the target.

1. View the target through the scope

2. Place the edge of one post against one edge (top, bottom,
or either side) of the target so that the crosshair extends along
either its width or height

3. Using the dots, measure along the crosshair to the opposite
edge of the target

If the center of the crosshair is against one edge of the target and the opposite
edge of the target is positioned behind the center of the second dot, the
target measures 2 mils. If it is exactly between the second and third dot, it
measures 2.5 mils, etc. The more specific you are in your estimation of the
size of the target in mils (2.75 mils, etc.), the more accurate your results will
be. This is especially important in estimating the range of a small target or in
estimating the range of a target at a great distance (i.e. beyond 500 yards).

Once the measurement of the target has been determined in mils, the range
can be estimated. This can be done in two ways – either by consulting the
charts in this manual or by using the following formula:

(Height of Target in Yards x 1,000) ÷ Height of Target in Mils = Range of the Target in Yards

This formula will also give results in metric terms if meters instead of yards are
used in the equation.

For your convenience, Leupold has included range estimating tables (see
Tables 1-6). Four of these tables are calculated to the nearest 0.5 mil and two
(a special sub-yard and a special sub-meter target size tables) are calculated to
the nearest 0.25 mil. To use these tables, locate the actual size of the target
along the top of the table and the apparent size of the target, as measured in
mils, along the side of the table. Follow both until they converge. This is the
estimated distance to the target.

3

4

Using the Data Obtained

With the Mil Dot Reticle

Once you have estimated the distance to the target with the Mil Dot reticle,
there are two primary methods of using this information. Both require that
you know the specific bullet drop of the ammunition you are using.

DIALING THE CORRECTION INTO THE SCOPE

The most effective way to use the estimated distance is to dial the necessary
correction into the scope using the elevation adjustment. (If your scope
features a bullet drop compensation dial, simply dial the correction directly
according to the distance marked on the elevation dial.)

1. Calculate the “drop to adjustment increment” ratio of your scope.
To do this, use the equation:

Distance to the target in yards ÷ 100 = Inch value of each minute of angle

2. Determine the correction necessary for the target using:

Known bullet drop for distance to target ÷ Inch value of each minute of angle =

Correction to be dialed in minutes of angle

HOLDING OVER USING THE MIL DOTS

Sometimes there isn’t time for correction using the scope’s adjustment
mechanisms. In these cases, holding over the target and using the reticle’s mil
dot markings as an aiming point is useful. It must be remembered that holding
over is not as exact as dialing elevation.

For ease in calculation, assume that the distance from the center of one mil
dot to the center of the next is 3.6 inches at 100 yards.

1. Calculate the holdover value of each dot for the distance to the target:

(Distance to the target in yards ÷ 100) x 3.6 =

Inch value from the center of one mil dot to the center of the next at that distance

2. Calculate the correct holdover:

Known bullet drop at target distance ÷

Inch value from the center of one mil dot to the center of the next at that distance =

Correct holdover for target distance

For quick reference on the value of mils at different distances, consult
Tables 7 and 8.

5

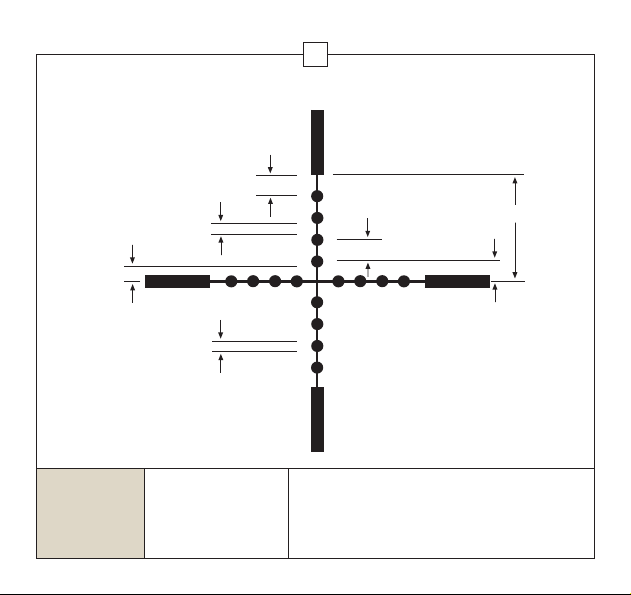
0.90mil

6

RETICLE MEASUREMENTS

1mil

0.80mil

0.20mil

5mil

1mil

1mil

@ 100 YARDS

1 mil = 3.600"

0.9 mil = 3.240"

0.8 mil = 2.880"

0.5 mil = 1.800"

0.2 mil = 0.720"
1 mil = 3.438 Minutes of Angle = 3.600"

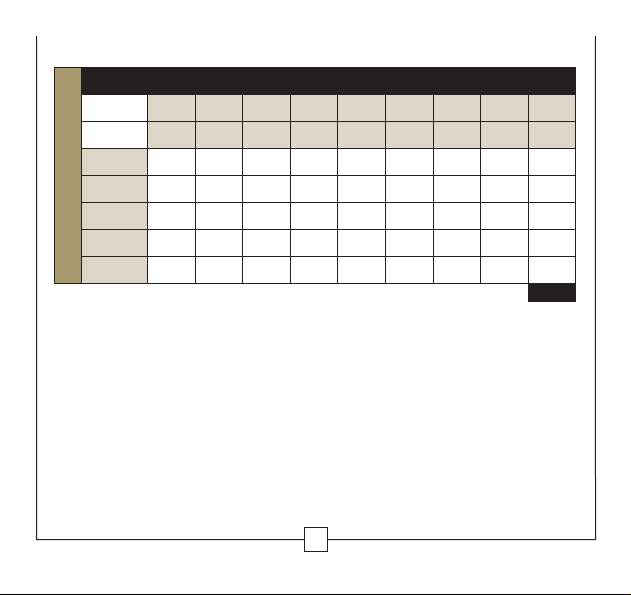
YIELDS ESTIMATED TARGET DISTANCE IN YARDS

ACTUAL SIZE OF THE TARGET IN INCHES OR YARDS

INCHES 9 12 16 18 20 22 24 28 32

YARDS 0.250 0.333 0.444 0.500 0.556 0.611 0.667 0.778 0.889

1.00 MIL 250 333 444 500 556 611 667 778 889

1.25 MIL

1.50 MIL

1.75 MIL

APPARENT SIZE OF THE TARGET IN MILS

2.00 MIL

200 267 356 400 444 489 533 622 711

167 222 296 333 370 407 444 519 593

143 190 254 286 317 349 381 444 508

125 167 222 250 278 306 333 389 444

7

Table 1