Lecroy 93XXC-OM-E20 User Manual

%

$SSHQGL[%(QKDQFHG5HVROXWLRQ

(QKDQFHG5HVROXWLRQ)LOWHULQJ

The available sampling rate of LeCroy oscilloscopes is often

higher than that required for the analyzed signal’s
bandwidth. Oversampling, particularly pronounced in the
long-memory models, can be used to increase the displayed
trace’s effective resolution: the ability of the instrument to
distinguish closely spaced voltage levels. This is done by
filtering the digitized signal using Enhanced Resolution,
available in the optional WP02 Advanced Math Package.

Although similar to signal smoothing using a simple moving­average filter, enhanced resolution filtering is more efficient both
in terms of bandwidth and the super ior passband character istics
that result. And on waveforms with single- shot characteristics, it
can be used instead of successive trace averaging.

$GYDQWDJHV Enhanced resolution filtering improves two important

characteristics of the oscilloscope.
Resolution is improved by a fixed amount for each filter. This true

increase in resolution occurs whether or not the signal is noisy, or
whether a single-shot or repetitive signal.

Signal–to–noise ratio (SNR) is improved in suc h a way as to be
dependent on the form of the noise in the or iginal signal. This is
because the enhanced resolution filtering decreases the
bandwidth of the signal, therefore filtering out some of the noise.

,PSOHPHQWDWLRQ The oscilloscope’s set of constant-phase, FIR (Finite Impulse-

Response filters provide fast computation, excellent step
response in 0.5 bit steps, and minimum bandwidth reduction for
resolution improvements of between 0.5 and 3 bits. Each step
corresponds to a bandwidth reduction of a factor of two, allowing
easy control of the bandwidth/resolution trade-off. The
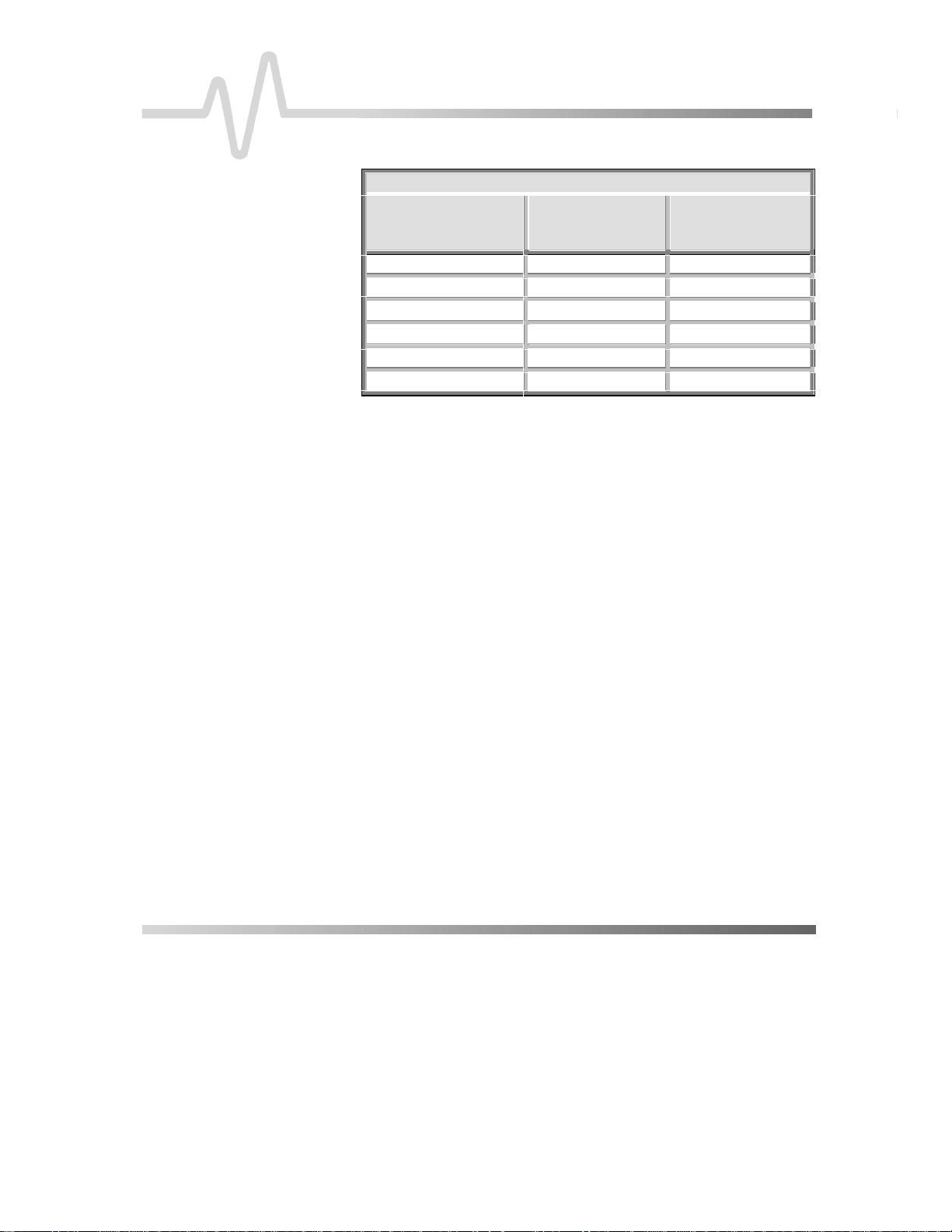
parameters of the six filters are given in the following table:

%²

$SSHQGL[%

),5(QKDQFHG5HVROXWLRQ)LOWHU3DUDPHWHUV

Resolution Increase

(Enhancement)

0.5 0.5 2

1.0 0.241 5

1.5 0.121 10

2.0 0.058 24

2.5 0.029 51

3.0 0.016 117

–3 dB Bandwidth

(× Ny quist)

Filter Length

(Samples)

With low-pass filters, the actual SNR increase obtained in any
particular situation depends on the power spectral density of the
noise on the signal. The improvem ent in SNR corr esponds to the
improvement in resolution if the noise in the signal is white —

that is, if it is evenly distributed across the f requency spectrum. If
the noise power is biased towards high frequencies, the SNR
improvement will be better than the resolution improvement.
Whereas the opposite may be true if the noise is m ostly at lower
frequencies.

SNR improvement due to the removal of c oherent noise signals
—feed-through of clock signals, f or exam ple — is decided by the
fall of the dominant frequency components of the signal in the
passband. This is easily ascertained using Spectral Analysis.

The filters have a precisely constant zero phase response. This
has two desirable properties. First, the filters do not distort the
relative position of differ ent events in the waveform, even if the
events’ frequency content is different. And second, because the
waveforms are stored, the delay normally associated with filtering
(between the input and output waveforms) can be exactly
compensated during the computation of the filtered waveform.

All the filters have been given exact unity gain at low frequency.
Enhanced resolution should thus not cause overflow if the s ourc e
data is not overflowed. If part of the source trace were to
overflow, filtering would be allowed, but the results in the vicinity
of the overflowed data — the filter impulse response length —

%²

(QKDQFHG5HVROXWLRQ

would be incorrect. This is because in some circumstances an
overflow may be a spike of only one or two samples, and the
energy in this spike may not be enough to significantly affect the
results. It would then not be desirable to disallow the whole trace.

:KHQWR8VH,W" In general, enhanced resolution is used to replace the averaging

function in situations where the data record has a single-shot or
slowly repetitive nature and averaging cannot be used.

There are two particular situations in which enhanced resolution
is especially useful. One is when the signal is noticeably noisy
and measurements of the noise are not required. T he signal can

be “cleaned up” by using the enhanced resolution f unction. The
other is when — even if the signal is not particularly noisy —
high-precision measurements of the waveform are required
(when using Expand with high vertical gain, for example).
Enhanced resolution will then increase the resolution of the
measurements.

The examples on the following pages illustrate how enhanced
resolution can be used.

%²

$SSHQGL[%

([DPSOHV

/RZSDVV)LOWHU LQJ This screen shows the spec trum of a square signal bef ore (top grid)

and after (bottom grid) enhanc ed resolution processing. The result
clearly illustrates how the filter rejects high-frequency components
from the signal. The higher the bit enhancement, the lower the
resulting bandwidth.

%²

(QKDQFHG5HVROXWLRQ

,QFUHDVLQJ9HUWLFDO
5HVROXWLRQ

In this example the bottom trace has been significantly enhanced by
a three-bit enhanced resolution function.

1RWH

The original signal being highly oversampled, the
resulting bandwidth is still high enough for the signal not to
be distorted.

%²

$SSHQGL[%

5HGXFLQJ1RLVH The following illustration shows the effec t of enhanced resolution on

a noisy signal. The original trace (top grid) has been proces sed by a
two-bit enhanced resolution filter. The result (bottom grid) shows a

“smooth” trace, where most of the noise has been eliminated.

%²

(QKDQFHG5HVROXWLRQ

&DXWLRQDU\1RWHV

The enhanced resolution function can only improve the resolution of a trace; it cannot

À

improve the accuracy or linearity of the original quantization by the eight-bit ADC.

À

The constraint of good temporal response excludes the use of maximally-flat filters.
The pass-band will therefore cause signal attenuation for signals near the cut-off
frequency. The highest frequencies passed may be slightly attenuated. The frequency
response of a typical enhanced resolution filter (the 2-bit enhancement filter) is

illustrated below, indicating the –3 dB cut-off frequency of 5.8% of the Nyquist
frequency.

À

The filtering must be performed on finite record lengths: data will lost at the start and
end of the waveform, so that the trace becomes slightly shorter after filtering.

À

The number of samples lost is exactly equal to the length of the impulse response of
the filter used, and thus varies between two and 117 samples. Owing to the
oscilloscope’s very long waveform memories, this loss — just 0.2% of a 50 000 point
trace — is not normally noticed. However, it is possible to demand filtering on a record
so short, there would be no data output, and in this case the scope will not allow
filtering.

%²