How it Works
HP
Loading...
an283
schematic
51 pgs11.4 Mb0

HP an283 schematic

Download
Page 1
f)
Application Note 283-3 Low Phase Noise Applications
of the HP 8662A and 8663A
Synthesized Signal Generators
Whp*
HEWLETT
PACKARD
Page 2
Page 3
Chapter 7 Using the HP 8662A/8663A at Microwave Frequencies With the HP 3048A Phase Noise Measurement System 26
Why Use the HP 8662A/8663A at Microwave Frequencies Effect of Multiplication on Signal Noise HP 8662A/8663A Phase Noise Performance at Microwave Frequencies Using the HP 8662A/8663A and the HP 11740A for Low-Noise Microwave Signal Generation Using the HP 3047A/11740A to Make Phase-Noise Measurements on Microwave Sources Measurement Techniques Data Interpretation HP 304 8A/11740A System Performance Measurements on Pulsed Sources Summary
Chapter 8 Voice Grade Receiver Testing With the HP 8662A/8663A 33
Receiver Test Basics: In-Channel and Out-of-Channel Testing Using the HP 8662A/8663A for Adjustment Channel Receiver Tests Using the HP 8662A/8663A for Spurious Attenuation Testing
Chapter 9 HP 8662A/8663A as an External LO with the HP 8901A/B Modulation Analyzer and HP 8902 Measuring Receiver 37
Measured Performance ■ Measurement Considerations and Procedure
Chapter 10 Using an HP 8662A/8663A With the HP 8505A RF Network Analyzer 39
Measurement Setup
Typical Operating Characteristics
Frequency Characteristics Range and Resolution Typical System Residual FM Output Characteristics Delay and Electrical Length Characteristics
Chapter 11 Using the HP 8662A/8663A as a Substitute LO With the HP 8672A
Microwave Synthesized Signal Generator 41
System Operation Hardware Modifications
System Performance Resolution Frequency Algorithm
Modulation
Chapter 12 Fast Frequency Switching With the HP 8662A/8663A 44
Standard HP-IB Frequency Control Fast Learn Frequency Switching Fast Frequency Switch Option H-50 Summary
Appendix A Calibration of Phase Noise of Three Unknown Sources .50
Appendix B 10 MHz Low-Noise Bandpass Amplifier 50
Appendix C
Low-Noise Amplifier 50 Appendix D
References 51
Hewlett-Packard Applications Notes Other References
Page 4
Chapter 1
Introduction
^^M Range (MHz) ^^m Resolution (Hz)
^H Stability
^H OUTPUT
^^M Range (dBm) ^^H Resolution (dB) ^^H Accuracy (dB)
^H MODULATION
^^H External ^H SPECTRAL PURITY
TABLE 1.1.
HP 8662A/8663A Performance Comparison
FREQUENCY
Harmonics Spurious
(-dBc)
320
to 640 MHz band
(-dBc)
in
The stringent performance requirements of modern radar and communications systems call for high frequency signals with extremely good spectral purity. The Hewlett-Packard 8662A and 8663A Synthesized Signal Generators provide extremely good overall spectral purity by combining the low close in phase noise of a frequency synthesizer with the low spurious and noise floor typically found only in cavity-tuned generators. These
characteristics make the HP 8662A and HP 8663A excellent choices for many low noise applications, particularly as local oscillators in low noise systems, low noise RF signals when multiplied up in frequency, or as versatile signal simu­lators through their flexible modulation formats. The HP 8662A operates up to 1280 MHz and is well suited to radio receiver testing by providing simulta­neous AM and FM modulation. The HP 8663A covers another frequency
octave, up to 2560 MHz, for applications in the low
S-band
range and provides simultaneous AM, FM, phase and pulse modulation. This allows simulation of radar returns and transmitted communi­cations signals.
The HP 8662A and the HP 8663A share the same frequency synthesis circuitry and therefore yield the same spectral purity. Their performance differs primar-
ily in frequency range, output power level, and modulation format. Table 1.1 and Figure 1.1 illustrate the HP 8662A and HP 8663A performance similarities and differences.
HP 8662A
0.01 to 1280
0.1 to 0.2 5xi0-10/day
+13 to -14D
0.1
±1
AM,
FM
AM,
FM
<30 <90
HP 8663A
0.1 to 2560
0.1 to 0.4 5xl0-10/day
+16 to-130
0.1
±1
AM,
FM,
Phase, Phase,
Pulse Pulse
AM,
FM,
<30 <90
Figure 1.1.
Measured Residual SSB phase noise versus offset from carrier. Carrier frequency 159 MHz, 639 MHz and 2.56 GHz
This application note discusses phase noise in detail (Chapter 2) to provide an understanding of its implications for cer­tain critical applications such as
out-of­channel receiver testing, doppler radar, and local oscillator substitution.
In Chapter 3, key design aspects of the
HP 8662A and HP 8663A, and the resulting phase noise performance, are presented, followed in Chapter 4 by a discussion of the effects of external refer­ences on their performance. Chapters 5 and 6 present techniques of applying the excellent phase-noise performance of the HP 8662A/8663A to solve problems that commonly arise in the measurement of low phase noise. Chapter 7 extends these techniques to the microwave frequency range via HP 8662A/8663A-based sys­tems specifically intended to measure
low phase noise microwave signals.
HP 8662A and at
159
MHz
The effects of signal generator phase noise on receiver testing are discussed in Chap­ter 8. The next three chapters present meth­ods of applying the HP 8662A/8663A to enhance the performance of several other Hewlett-Packard instruments. Finally, Chap­ter 12 discusses the fast frequency switching capability of the HP 8662A/8663 A.
HP
8663A "
HP 8662A and HP 8663A at 639 MHz
v-
"HP 8663A at—T
2.56 GHz «■• "
vv-j?,
o
Page 5
^tes XW*i
-,?".„,
*.
»\1i"
Chapter 2
The Phase Noise Density Spectrum and Its Implications
What is Phase Noise?
Every RF or microwave signal displays some frequency instability. A complete description of such instability is gener­ally broken into two components, long-
term and short-term. Long-term fre­quency stability, commonly known as frequency drift, describes the amount of variation in signal frequency that occurs over long time periods - hours, days, or even months. Short-term frequency sta­bility refers to the variations that occur over time periods of a few seconds or less.
This application note deals primar-
ily with short-term frequency stability.
DIFFERENT MEASURES OF SHORT­TERM FREQUENCY STABILITY
There are a number of methods for specifying short-term frequency stability. Three of these methods, fractional frequency deviation, residual FM, and single sideband (SSB) phase noise are discussed in this chapter.
Fractional frequency deviation uses a time domain measurement in which the frequency of the signal is repeatedly measured with a frequency counter, with the time period of each measurement held constant. This allows several calculations of the fractional frequency difference, y, over a time period, T. A special variance of these differences, called the Allan variance, can then be calculated. The square root of this variance is generally repeated for several different
time periods, or T, and versus T as an indication of the signal's short-term frequency stability. (See also NBS Technical Note 394, "Characterization of Frequency Stability", reference 9 in Appendix D.)
<T(T).
The whole process is
O(T)
is plotted
For this reason, the use of residual FM to specify the short-term stability of a signal generally provides the least amount of information of the methods listed. An additional disadvantage is that different post-detection bandwidths are specified in different measurement standards. For example, another common choice is 20 Hz to 15 kHz. As
a result, quite often comparisons of oscillator performance based on residual FM specifications cannot be made directly. However, for many communi­cations systems, residual FM is used because it matches the terms and condi­tions of the application.
Single sideband (SSB) phase noise mea-
sures short-term instabilities as low-level phase modulation of the signal carrier. Due to the random nature of the insta­bilities, the phase deviation must be rep­resented by a spectral density distribu­tion plot known as an SSB phase noise plot, see Figure 2.2.
Of all the methods commonly in use, SSB phase noise has the advantage of providing the most information about the short-term frequency stability of a signal. In addition, both fractional fre-
quency deviation and residual FM may be derived if the phase noise distribution of a signal is known. As a result, SSB phase noise has become the most widely used method of specifying short-term stability. For this reason, the majority of this application note is devoted to SSB phase noise to specify short-term fre­quency stability.
SSB PHASE NOISE DEFINITIONS
Due to phase noise, in the frequency domain a signal is not a discrete spectral line,
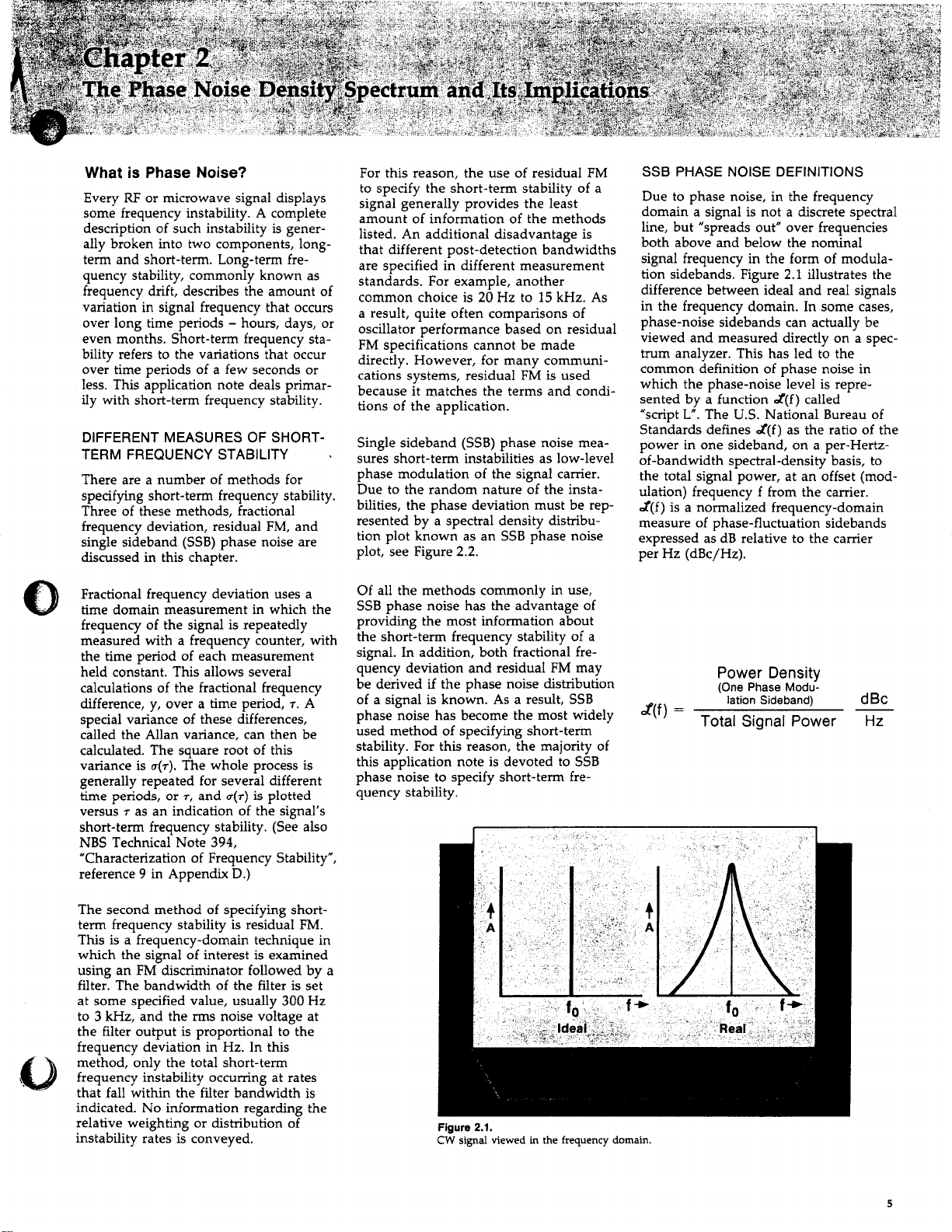
but "spreads out" over frequencies both above and below the nominal signal frequency in the form of modula­tion sidebands. Figure 2.1 illustrates the difference between ideal and real signals in the frequency domain. In some cases, phase-noise sidebands can actually be viewed and measured directly on a spec­trum analyzer. This has led to the common definition of phase noise in which the phase-noise level is repre­sented by a function <<f(f) called "script L". The U.S. National Bureau of Standards defines Jf(f) as the ratio of the power in one sideband, on a per-Hertz-
of-bandwidth spectral-density basis, to the total signal power, at an offset (mod­ulation) frequency f from the carrier. Jt({) is a normalized frequency-domain measure of phase-fluctuation sidebands expressed as dB relative to the carrier per Hz (dBc/Hz).
Power Density (One Phase Modu-
Jf(f) =
lation Sideband) dBc
Total Signal Power Hz
The second method of specifying short­term frequency stability is residual FM. This is a frequency-domain technique in which the signal of interest is examined using an FM discriminator followed by a filter. The bandwidth of the filter is set at some specified value, usually 300 Hz to 3 kHz, and the rms noise voltage at the filter output is proportional to the frequency deviation in Hz. In this
method, only the total short-term frequency instability occurring at rates that fall within the filter bandwidth is indicated. No information regarding the relative weighting or distribution of instability rates is conveyed.
Figure
2.1.
CW signal viewed in the frequency domain.
5
Page 6
As mentioned, <Jf(f) can be measured directly on a spectrum analyzer if the following conditions are met:
1.
The spectrum analyzer noise floor is lower than the level of phase noise being measured. This means that the
phase noise of the spectrum analyzer's local oscillator must be lower than the level of the noise being measured. In addition, the dynamic range and selec­tivity of the analyzer must be sufficient to discern the measured phase noise.
2.
The signal's AM noise does not make a significant contribution to the noise measured. This can be determined by measuring the AM noise of the signal, or it can be deduced by understanding the nature of the source under test.
For more information on how to mea­sure phase noise directly on spectrum analyzers, refer to Hewlett-Packard Application Note 270-2, "Automated Noise Sideband Measurements Using the HP 8568A Spectrum Analyzer".
Another function frequently encountered in phase noise work is S,(f). S.(f) is the spectral density of the phase fluctuations in radians squared per Hz. The relation­ship between S^(f) and <Jf(f) is simply:
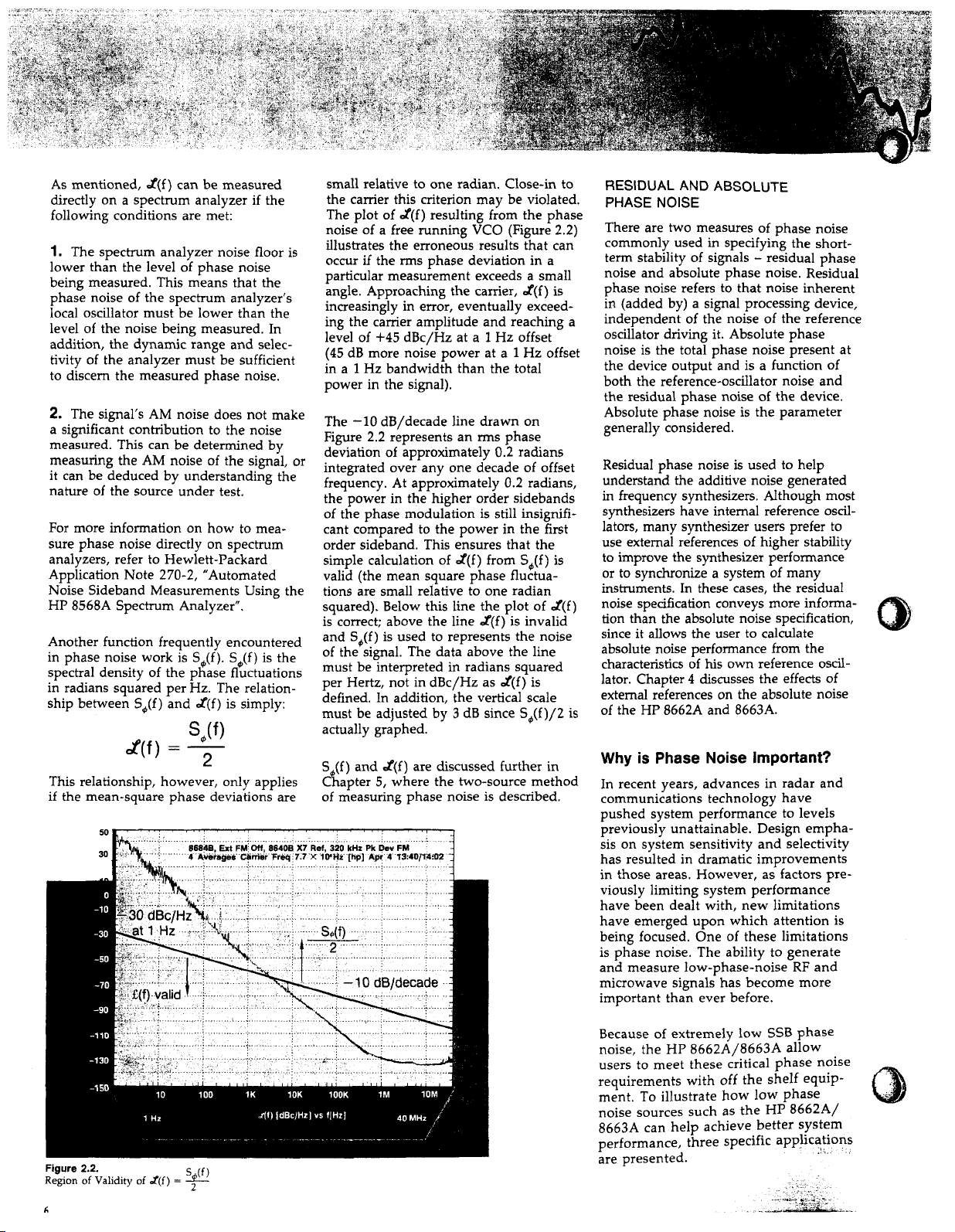
small relative to one radian. Close-in to the carrier this criterion may be violated. The plot of Jt({) resulting from the phase noise of a free running VCO (Figure 2.2) illustrates the erroneous results that can
occur if the rms phase deviation in a particular measurement exceeds a small angle. Approaching the carrier, «Jf(f) is increasingly in error, eventually exceed­ing the carrier amplitude and reaching a level of +45 dBc/Hz at a 1 Hz offset (45 dB more noise power at a 1 Hz offset in a 1 Hz bandwidth than the total power in the signal).
The —10 dB/decade line drawn on Figure 2.2 represents an rms phase deviation of approximately 0.2 radians integrated over any one decade of offset frequency. At approximately 0.2 radians, the power in the higher order sidebands of the phase modulation is still insignifi-
cant compared to the power in the first order sideband. This ensures that the simple calculation of Jf(f) from S^(f) is valid (the mean square phase fluctua­tions are small relative to one radian squared). Below this line the plot of Jf(f) is correct; above the line Jf(f) is invalid and S ,(f) is used to represents the noise of the signal. The data above the line
must be interpreted in radians squared per Hertz, not in dBc/Hz as of(f) is defined. In addition, the vertical scale must be adjusted by 3 dB since
S^(f)/2
is
actually graphed.
RESIDUAL AND ABSOLUTE PHASE NOISE
There are two measures of phase noise commonly used in specifying the short­term stability of signals - residual phase noise and absolute phase noise. Residual phase noise refers to that noise inherent in (added by) a signal processing device, independent of the noise of the reference oscillator driving it. Absolute phase noise is the total phase noise present at
the device output and is a function of both the reference-oscillator noise and the residual phase noise of the device. Absolute phase noise is the parameter generally considered.
Residual phase noise is used to help understand the additive noise generated
in frequency synthesizers. Although most synthesizers have internal reference oscil­lators,
many synthesizer users prefer to use external references of higher stability to improve the synthesizer performance or to synchronize a system of many instruments. In these cases, the residual noise specification conveys more informa­tion than the absolute noise specification, since it allows the user to calculate absolute noise performance from the
characteristics of his own reference oscil­lator. Chapter 4 discusses the effects of external references on the absolute noise of the HP 8662A and 8663A.
«*(f) = -y-
This relationship, however, only applies
if the mean-square phase deviations are
Figure 2.2.
Region of Validity of Jt(() = -|—
s (f
j
S ,(f) and «f(f) are discussed further in Chapter 5, where the two-source method of measuring phase noise is described.
Why is Phase Noise Important?
In recent years, advances in radar and communications technology have pushed system performance to levels previously unattainable. Design empha­sis on system sensitivity and selectivity
has resulted in dramatic improvements in those areas. However, as factors pre­viously limiting system performance have been dealt with, new limitations have emerged upon which attention is being focused. One of these limitations is phase noise. The ability to generate and measure low-phase-noise RF and microwave signals has become more important than ever before.
Because of extremely low SSB phase noise, the HP 8662A/8663A allow
users to meet these critical phase noise requirements with off the shelf equip­ment. To illustrate how low phase noise sources such as the HP 8662A/ 8663A can help achieve better system performance, three specific applications are presented.
Page 7
*.;.-■*:■«
.,1s *» <•-.*/.
-W!?>;
wspe.
LOCAL-OSCILLATOR APPLICATIONS
Phase noise can be a major limiting factor in high performance frequency­conversion applications dealing with
signals that span a wide dynamic range. The first down conversion in a high-performance superheterodyne receiver serves as a good example for illustration. Suppose that two signals (Figure 2.3a) are present at the input of such a receiver. These signals are to be mixed with a local oscillator signal down to an intermediate frequency (IF) where highly selective IF filters can
separate one of the signals for amplifi-
cation, detection, and baseband pro­cessing. If the desired signal is the
larger signal, there should be no diffi­culty in recovering it, if the receiver is> correctly designed.
signal can degrade a receiver's useful dynamic range as well as its selectivity. To achieve the best performance from a given receiver design, its local-oscillator phase noise must be minimized. This is where the HP 8662A/8663A can help. First the HP 8662A/8663A can provide a low-phase-noise signal to serve as the reference when measuring the phase noise of the local-oscillator signal under test. This measurement is described in detail in Chapters 5 and 6. Second, the HP 8662A/8663A can provide the local-
oscillator signal typical output power, 0.1 Hz frequency resolution, 420/510 microsecond frequency switching speed, and full HP-IB programmability, the HP 8662A/ 8663A can serve in almost any demand­ing local-oscillator application.
itself.
With +16 dBm
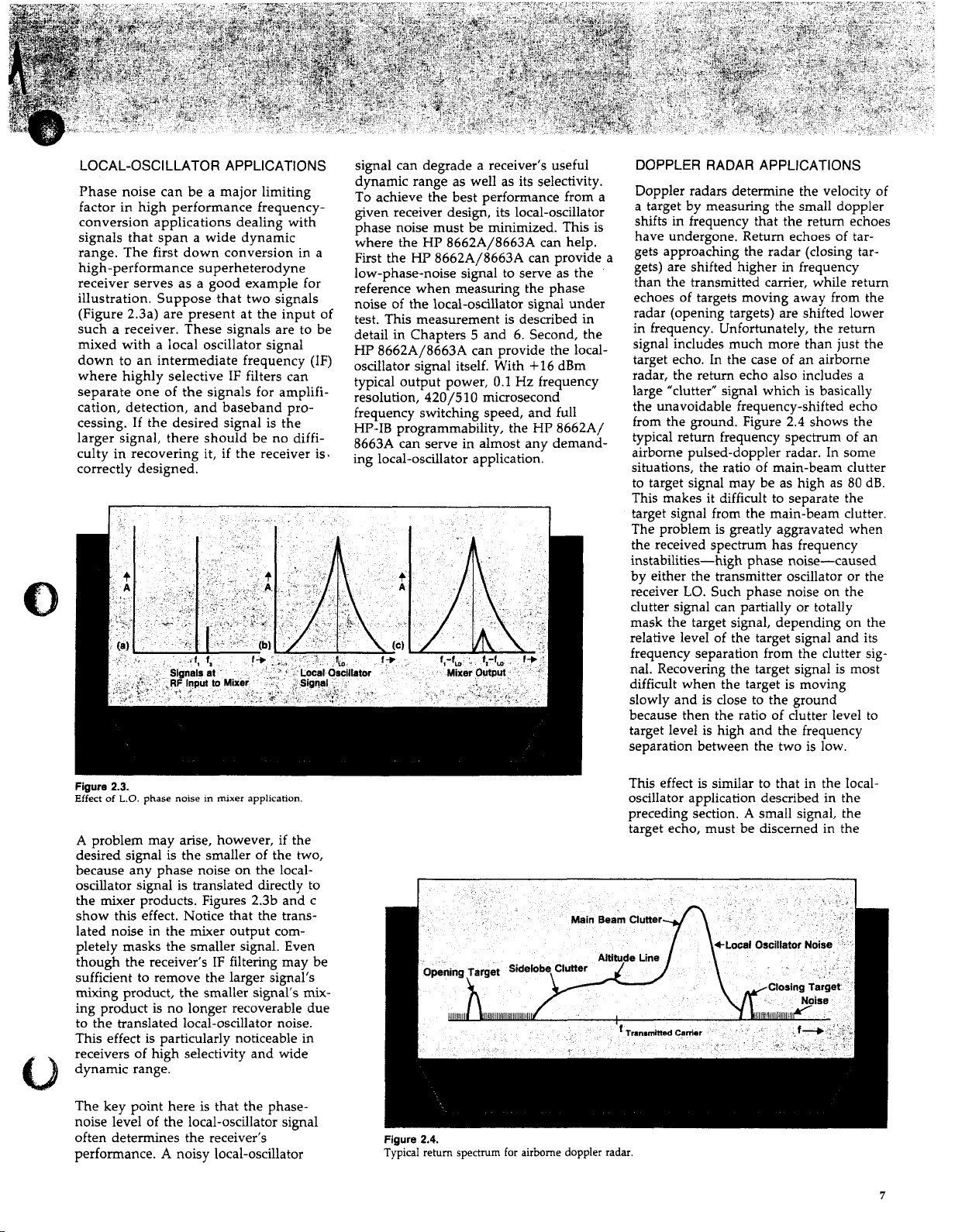
DOPPLER RADAR APPLICATIONS
Doppler radars determine the velocity of a target by measuring the small doppler shifts in frequency that the return echoes have undergone. Return echoes of tar-
gets approaching the radar (closing tar­gets) are shifted higher in frequency than the transmitted carrier, while return echoes of targets moving away from the radar (opening targets) are shifted lower in frequency. Unfortunately, the return signal includes much more than just the
target echo. In the case of an airborne radar, the return echo also includes a large "clutter" signal which is basically the unavoidable frequency-shifted echo from the ground. Figure 2.4 shows the typical return frequency spectrum of an
airborne pulsed-doppler radar. In some situations, the ratio of main-beam clutter to target signal may be as high as 80 dB. This makes it difficult to separate the target signal from the main-beam clutter. The problem is greatly aggravated when the received spectrum has frequency
instabilities—high phase noise—caused by either the transmitter oscillator or the receiver LO. Such phase noise on the clutter signal can partially or totally mask the target signal, depending on the relative level of the target signal and its frequency separation from the clutter sig­nal.
Recovering the target signal is most
difficult when the target is moving slowly and is close to the ground because then the ratio of clutter level to target level is high and the frequency separation between the two is low.
Figure 2.3.
Effect of L.O. phase noise in mixer application.
A problem may arise, however, if the desired signal is the smaller of the two, because any phase noise on the local­oscillator signal is translated directly to the mixer products. Figures 2.3b and c show this effect. Notice that the trans­lated noise in the mixer output com­pletely masks the smaller signal. Even though the receiver's IF filtering may be sufficient to remove the larger signal's mixing product, the smaller signal's mix­ing product is no longer recoverable due to the translated local-oscillator noise.
This effect is particularly noticeable in receivers of high selectivity and wide dynamic range.
The key point here is that the phase­noise level of the local-oscillator signal often determines the receiver's performance. A noisy local-oscillator
This effect is similar to that in the local-
oscillator application described in the preceding section. A small signal, the target echo, must be discerned in the
Figure 2.4.
Typical return spectrum for airborne doppler radar.
7
Page 8
• MM
■.-'
"iv***""!
presence of the much larger clutter
signal that is very close in frequency. Again, the system performance is limited by phase noise. In this case, it is the phase-noise level of either the transmit­ter oscillator or the receiver local oscilla­tor that is limiting.
The HP 8662A/8663A can improve the radar's performance by serving as a low­phase-noise source for phase-noise mea-
surement or signal substitution. Since most radars operate at microwave fre­quencies, it is usually necessary to multi­ply the generator's outputs to the micro­wave frequency range. This multiplication is discussed in Chapter 7.
OUT-OF-CHANNEL RECEIVER TESTING
Modern communications receivers have excellent selectivity and spurious rejec-
tion characteristics. These are called the out-of-channel characteristics and require very high quality test signals for verifica­tion. Typically, two signal generators are used for testing the out-of-channel char­acteristics of a receiver. One generator is tuned in channel, the other is tuned out of channel, typically one channel spacing away.
Due to the masking effect described for
\„^
local oscillator applications, the phase noise and AM noise of the out-of-channel generator may limit the selectivity that can be measured. As a result, the measured selectivity may be much worse than the actual receiver selectivity. The limiting
level of phase noise on the out-of-channel generator is determined by the level of performance of the receiver that is being measured. More selective receivers require lower phase noise on the out-of-channel generator. Out-of-channel receiver testing and the phase noise requirements of the out-of-channel generator are described in more detail in Chapter 8.
Page 9
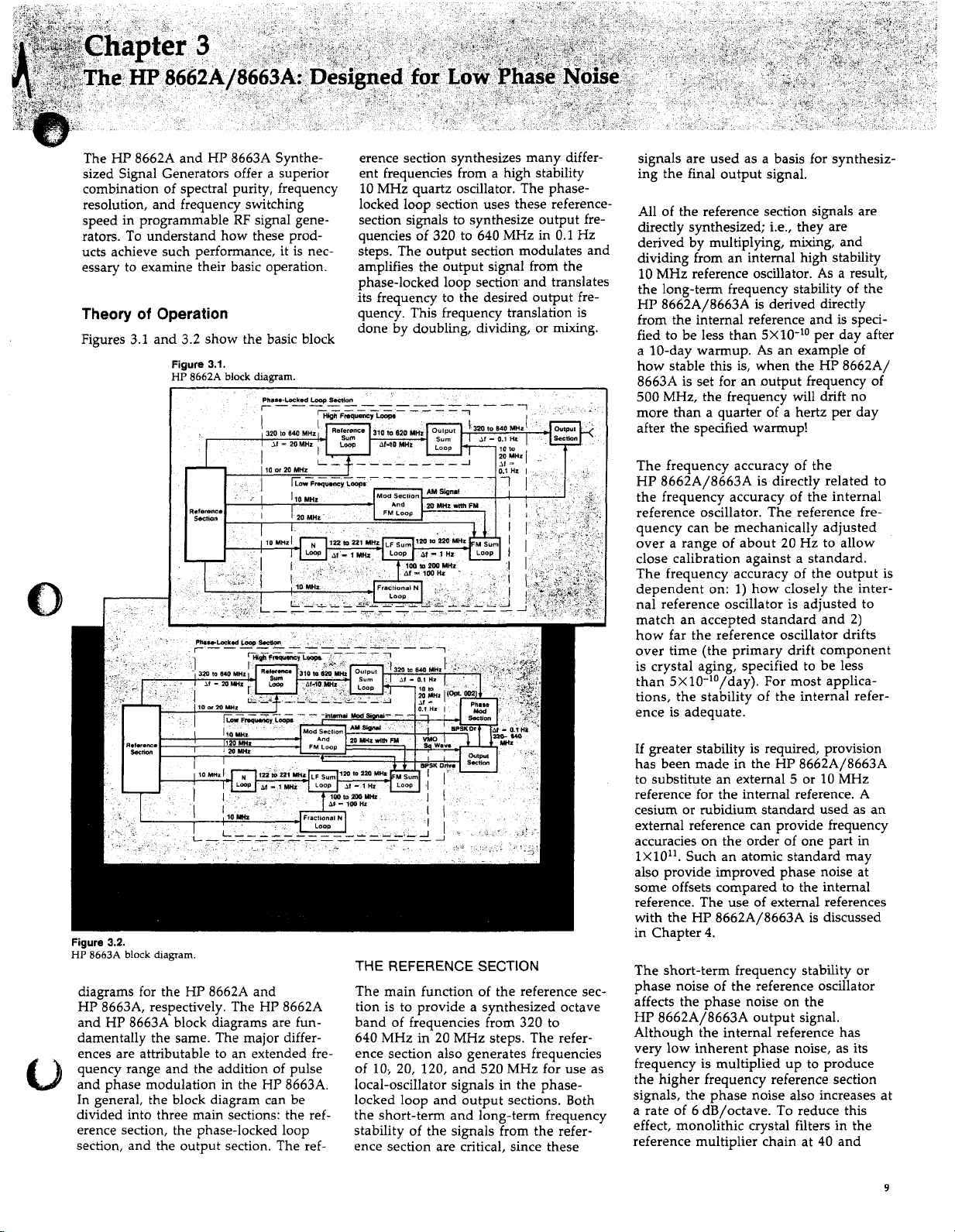
Chapter 3
The HP 8662A/8663A: Designed for Low Phase Noise
The HP 8662A and HP 8663A Synthe­sized Signal Generators offer a superior combination of spectral purity, frequency resolution, and frequency switching speed in programmable RF signal gene-
rators.
To understand how these prod­ucts achieve such performance, it is nec­essary to examine their basic operation.
Theory of Operation
Figures 3.1 and 3.2 show the basic block
Figure 3.1.
HP 8662A block diagram.
Phaee-Locked Loop Section
I ' High Frequency Loop*
erence section synthesizes many differ­ent frequencies from a high stability 10 MHz quartz oscillator. The phase­locked loop section uses these reference-
section signals to synthesize output fre­quencies of 320 to 640 MHz in 0.1 Hz steps.
The output section modulates and amplifies the output signal from the phase-locked loop section and translates its frequency to the desired output fre­quency. This frequency translation is done by doubling, dividing, or mixing.
Reference
Sum
Loop
310 to 620 MHz
* 320 to 640 MHz
signals are used as a basis for synthesiz­ing the final output signal.
All of the reference section signals are directly synthesized; i.e., they are derived by multiplying, mixing, and
dividing from an internal high stability
10 MHz reference oscillator. As a result, the long-term frequency stability of the HP 8662A/8663A is derived directly from the internal reference and is speci­fied to be less than 5X10~10 per day after a 10-day warmup. As an example of how stable this is, when the HP 8662A/ 8663A is set for an output frequency of 500 MHz, the frequency will drift no
more than a quarter of a hertz per day after the specified warmup!
The frequency accuracy of the HP 8662A/8663A is directly related to the frequency accuracy of the internal reference oscillator. The reference fre­quency can be mechanically adjusted over a range of about 20 Hz to allow close calibration against a standard. The frequency accuracy of the output is dependent on: 1) how closely the inter­nal reference oscillator is adjusted to match an accepted standard and 2) how far the reference oscillator drifts over time (the primary drift component is crystal aging, specified to be less than 5X10-10/day). For most applica­tions,
the stability of the internal refer-
ence is adequate.
Figure 3.2.
HP 8663A block diagram.
diagrams for the HP 8662A and HP 8663A, respectively. The HP 8662A and HP 8663A block diagrams are fun­damentally the same. The major differ­ences are attributable to an extended fre­quency range and the addition of pulse
o
and phase modulation in the HP 8663A. In general, the block diagram can be divided into three main sections: the erence section, the phase-locked loop section, and the output section. The ref-
ref-
THE REFERENCE SECTION
The main function of the reference sec-
tion is to provide a synthesized octave band of frequencies from 320 to 640 MHz in 20 MHz steps. The refer­ence section also generates frequencies of 10-, 20, 120, and 520 MHz for use as local-oscillator signals in the phase-
locked loop and output sections. Both the short-term and long-term frequency stability of the signals from the refer­ence section are critical, since these
If greater stability is required, provision has been made in the HP 8662A/8663A to substitute an external 5 or 10 MHz reference for the internal reference. A cesium or rubidium standard used as an external reference can provide frequency accuracies on the order of one part in
1X1011.
also provide improved phase noise at
some offsets compared to the internal
reference. The use of external references with the HP 8662A/8663A is discussed in Chapter 4.
The short-term frequency stability or phase noise of the reference oscillator affects the phase noise on the HP 8662A/8663A output signal. Although the internal reference has very low inherent phase noise, as its frequency is multiplied up to produce the higher frequency reference section signals, the phase noise also increases at
a rate of 6 dB/octave. To reduce this
effect, monolithic crystal filters in the
reference multiplier chain at 40 and
Such an atomic standard may
9
Page 10
160 MHz filter the noise sidebands at offsets greater than about 4 kHz. The resulting phase noise of the reference section output at 500 MHz is typically
-110 dBc (dB relative to the carrier) at a
10 Hz offset decreasing to a noise floor of about -148 dBc at offsets greater than 10 kHz.
The mechanical configuration of the crystal filters is critical, since any small
mechanical vibrations in the filter trans­late directly into microphonic spurious sidebands on the signal. The most common source of instrument vibration is the cooling fan which causes spurious signals at about 53 Hz offsets with 60 Hz power lines. This spurious mechanism is minimized in the HP 8662A/8663A by a special shock mounting arrangement which mechanically isolates the crystal filters from instrument vibration and by dynamically balancing each fan before
installation in the instrument.
THE PHASE-LOCKED LOOP SECTION
The phase-locked loop section consists of seven phase-locked loops that provide the frequency programmability, frequency modulation, and fine frequency resolution of the HP 8662A/
8663A without compromising the excel-
lent frequency stability and spectral pur­ity of the reference section. Using an indirect-synthesis technique (i.e., synthe­sis using phase-locked loops as con­trasted with direct synthesis by mixing, multiplying, or dividing as is done in the reference section), the phase-locked loop section takes the 320 to 640 MHz in 20 MHz steps from the reference section and synthesizes an output of 320 to 640 MHz in 0.1 Hz steps.
The phase-locked loop section is divided into two areas, the high-frequency loops and the low-frequency loops. The two high-frequency loops are nearly identical with specially designed, low-noise voltage-controlled oscillators (VCOs).
The low-frequency loops consist of five phase-locked loops; three that provide the HP 8662A/8663A's 0.1 Hz frequency resolution and two which generate frequency modulation and sum the resulting FM signal with the final output signal.
High-Frequency Loops
The first of the two high-frequency loops,
the reference sum loop, tunes
over a 310 to 620 MHz frequency range.
This loop sums the reference section's main output of 320 to 640 MHz with 10 or 20 MHz also from the reference sec­tion. The reference sum loop's primary function is to filter out spurious signals on the reference section output beyond
the loop bandwidth and to improve the resolution from 20 MHz steps to 10 MHz steps.
The loop provides 60 dB of spectral filtering, thereby reducing the spurious level from —40 dBc to
-100 dBc. Such filtering is an advantage of indirect synthesis, since the bandwidth of the phase-locked loop can be set so that the loop VCO will only track the loop reference signal within the bandwidth of the loop. Reference signal sidebands falling outside the loop band
width are therefore rejected by the loop.
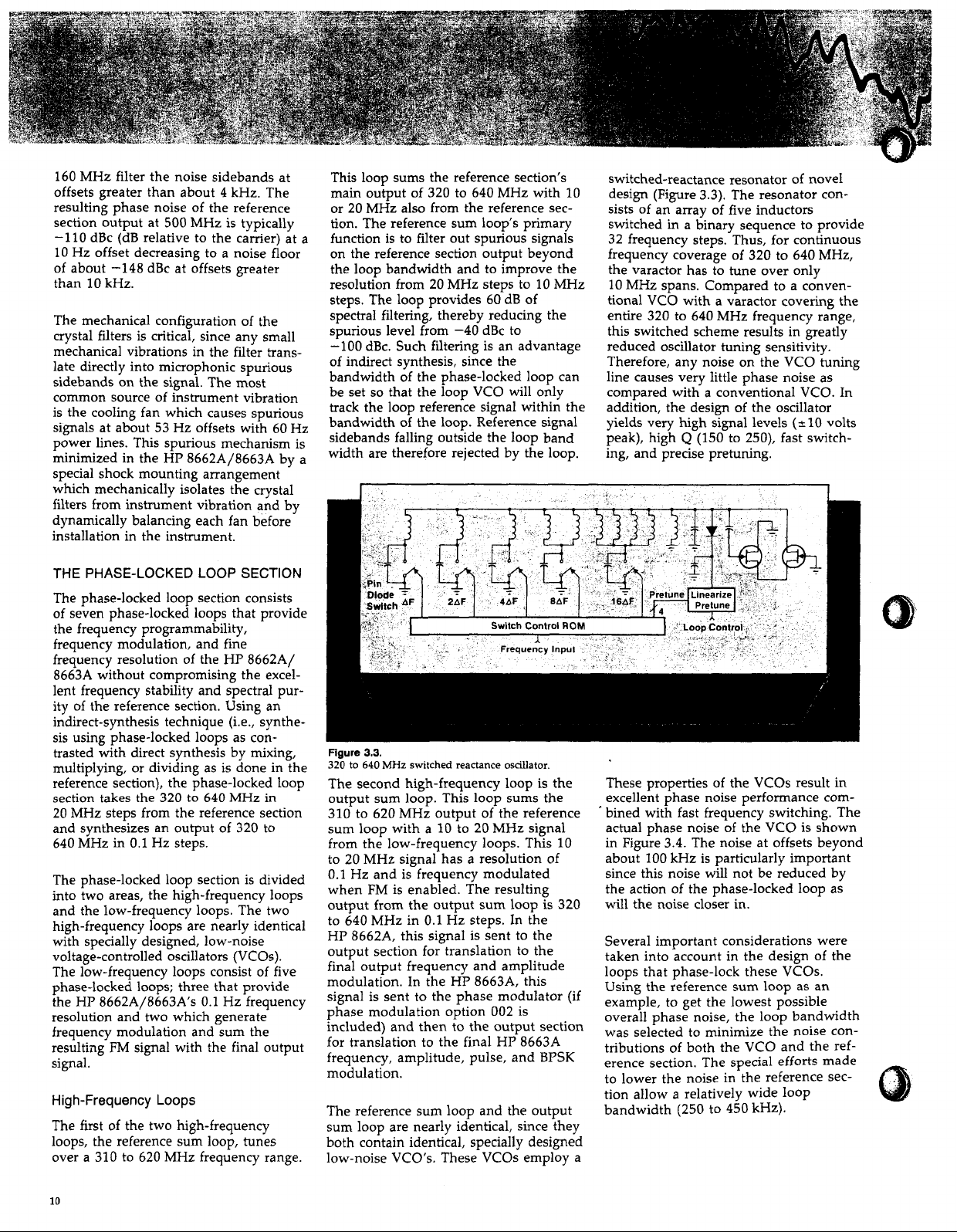
Figure 3.3.
320 to 640 MHz switched reactance oscillator.
The second high-frequency loop is the output sum loop. This loop sums the 310 to 620 MHz output of the reference sum loop with a 10 to 20 MHz signal from the low-frequency loops. This 10
to 20 MHz signal has a resolution of
0.1 Hz and is frequency modulated when FM is enabled. The resulting output from the output sum loop is 320 to 640 MHz in 0.1 Hz steps. In the HP 8662A, this signal is sent to the output section for translation to the final output frequency and amplitude modulation. In the HP 8663A, this signal is sent to the phase modulator (if phase modulation option 002 is included) and then to the output section for translation to the final HP 8663A frequency, amplitude, pulse, and BPSK modulation.
The reference sum loop and the output sum loop are nearly identical, since they both contain identical, specially designed low-noise VCO's. These VCOs employ a
switched-reactance resonator of novel design (Figure 3.3). The resonator con­sists of an array of five inductors switched in a binary sequence to provide 32 frequency steps. Thus, for continuous frequency coverage of 320 to 640 MHz, the varactor has to tune over only
10 MHz spans. Compared to a conven­tional VCO with a varactor covering the entire 320 to 640 MHz frequency range, this switched scheme results in greatly reduced oscillator tuning sensitivity. Therefore, any noise on the VCO tuning line causes very little phase noise as compared with a conventional VCO. In addition, the design of the oscillator
yields very high signal levels (±10 volts peak),
high Q (150 to 250), fast switch-
ing, and precise pretuning.
These properties of the VCOs result in
excellent phase noise performance com­bined with fast frequency switching. The actual phase noise of the VCO is shown in Figure 3.4. The noise at offsets beyond about 100 kHz is particularly important since this noise will not be reduced by the action of the phase-locked loop as will the noise closer in.
Several important considerations were taken into account in the design of the loops that phase-lock these VCOs. Using the reference sum loop as an example, to get the lowest possible overall phase noise, the loop bandwidth was selected to minimize the noise con-
tributions of both the VCO and the
ref­erence section. The special efforts made to lower the noise in the reference sec­tion allow a relatively wide loop bandwidth (250 to 450 kHz).
10
Page 11
•y;' v ■
, -.if. n
if
^
o
■■
\i'^-?'^r;W
«t90
^>
:
"V:
iff
4
■'
m
-1201
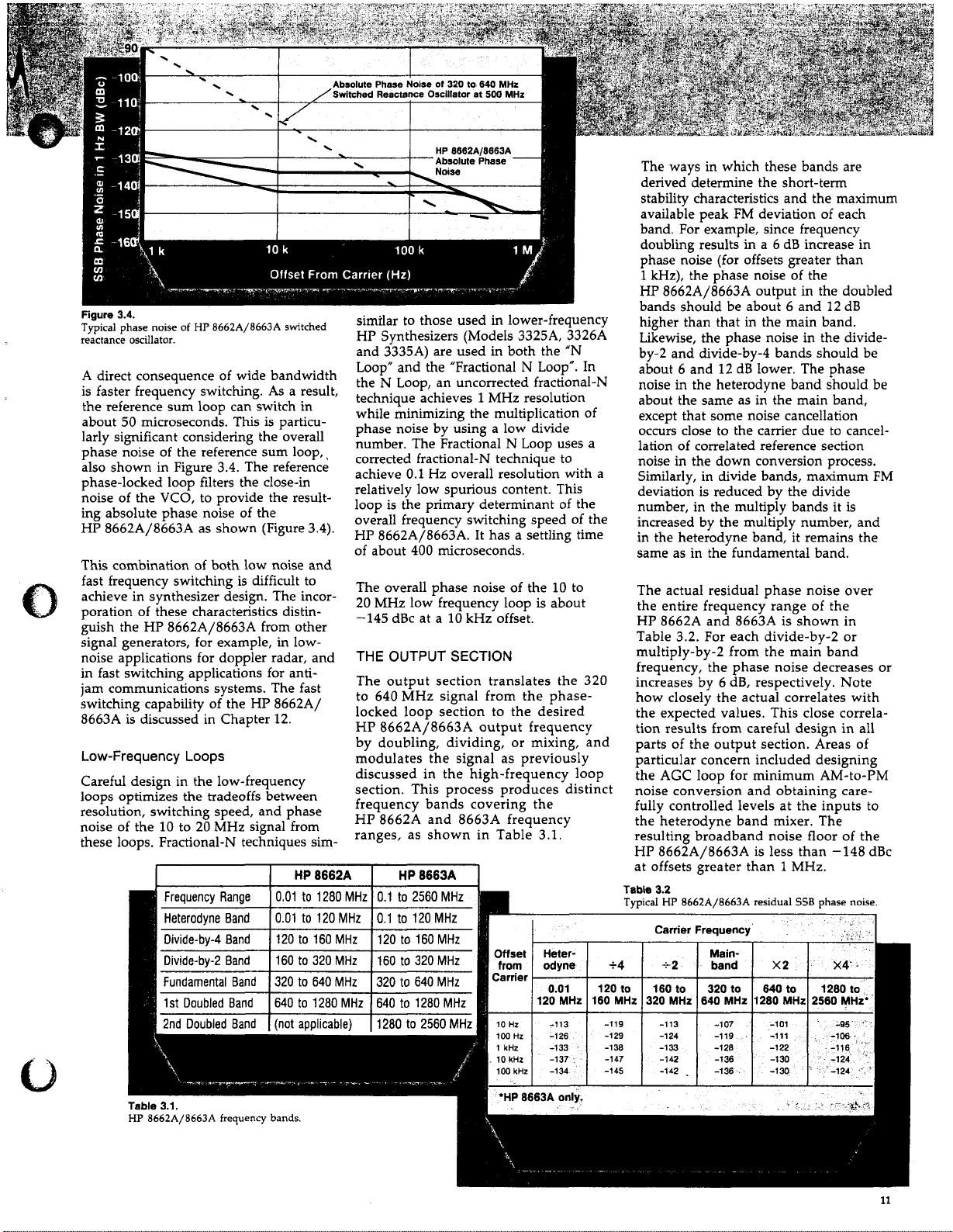
Figure 3.4.
Typical phase noise reactance oscillator.
A direct consequence
of
HP 8662A/8663A switched
of
wide bandwidth
is faster frequency switching. As a result,
the reference sum loop can switch
about 50 microseconds. This larly significant considering the overall phase noise also shown
of
the reference sum loop,
in
Figure 3.4. The reference phase-locked loop filters the close-in noise
of
ing absolute phase noise
the VCO,
HP 8662A/8663A
This combination fast frequency switching achieve
in
poration
synthesizer design. The incor-
of
to
provide the result-
of
as
shown (Figure 3.4).
of
both low noise and
is
these characteristics distin­guish the HP 8662A/8663A from other signal generators, noise applications
for
example,
for
doppler radar, and in fast switching applications jam communications systems. The fast switching capability 8663A
is
discussed
Low-Frequency Loops
Careful design
of
the HP 8662A/
in
Chapter 12.
in
the low-frequency loops optimizes the tradeoffs between resolution, switching speed, and phase noise
of
the 10
to
20 MHz signal from
these loops. Fractional-N techniques sim-
Frequency Range Heterodyne Band Divide-by-4 Band
Divide-by-2 Band Fundamental Band 1st Doubled Band 2nd Doubled Band
Table 3.1.
HP 8662A/8663A frequency bands.
s^:
^:y
V
Absolute Phase Noise of 320 to 640 MHz
'Switched Reactance Oscillator at 500 MHz
Offset From Carrier (Hz)
similar HP Synthesizers (Models 3325A, 3326A and 3335A) are used Loop"
the N Loop, technique achieves 1 MHz resolution while minimizing the multiplication phase noise number. The Fractional N Loop uses
_
corrected fractional-N technique
is
particu-
in
achieve 0.1 Hz overall resolution with relatively low spurious content. This
the
loop overall frequency switching speed
HP 8662A/8663A. of about 400 microseconds.
difficult
to
The overall phase noise
20 MHz low frequency loop
-145 dBc
in
for
low-
anti-
THE OUTPUT SECTION
The output section translates
to 640 MHz signal from locked loop section HP 8662A/8663A output frequency by doubling, dividing, modulates discussed section. This process produces distinct frequency bands covering HP 8662A
ranges,
HP 8662A
0.01
to
1280 MHz
0.01
to
120
MHz 120 to 160 MHz 160 to 320 MHz
320 to 640 MHz 640 to 1280 MHz (not applicable)
'>;>0'^$§i
HP 8662A/8663A
~
Absolute Phase Noise
to
those used
and the "Fractional N Loop".
an
by
is
the primary determinant
at a
the
in the
and
as
shown
HP 8663A
0.1
to
2560 MHz
0.1
to
120 MHz 120 tO 160 MHZ 160 to 320 MHz 320 to 640 MHz 640 to 1280 MHz 1280
to
2560 MHz
in
lower-frequency
in
both the
uncorrected fractional-N
using a low divide
It
has a settling time
of
the 10
is
10 kHz offset.
the
to the
signal
desired
or
mixing,
as
previously
high-frequency loop
the
8663A frequency
in
Table 3.1.
I Offset H from H Carrier
H
10 Hz
II
100 Hz
U 1 kHz I
10 kHz
■
100 kHz
*HP 8663A only,
"N
In
of
a
to
of
the
of
to
about
the 320
phase-
and
Heter­odyne
0.01
120 MHz
-113
-126
-133
-137.
-134
The ways
in
which these bands derived determine the short-term stability characteristics and the maximum available peak FM deviation band. For example, since frequency doubling results
in a
6 dB increase phase noise (for offsets greater than 1 kHz), the phase noise
of
HP 8662A/8663A output
bands should higher than that
be
about 6 and 12 dB
in
the main band. Likewise, the phase noise by-2 and divide-by-4 bands should about 6 and 12 dB lower. The phase noise
in
about the same as
the heterodyne band should
in
the main band,
except that some noise cancellation
a
the
occurs close
lation noise Similarly, deviation
number, increased in the heterodyne band, same
to
of
in
the carrier due
correlated reference section
the down conversion process.
in
divide bands, maximum FM
is
reduced
in
the multiply bands
by
by
the divide
the multiply number, and
it
as in
the fundamental band.
The actual residual phase noise over the entire frequency range HP 8662A and 8663A
is
shown Table 3.2. For each divide-by-2 multiply-by-2 from
frequency, increases
the
by
the
main band
phase noise decreases
6 dB, respectively. Note how closely the actual correlates with the expected values. This close correla­tion results from careful design parts
of
the output section. Areas particular concern included designing the AGC loop
noise conversion fully controlled levels
for
minimum AM-to-PM
and
obtaining care-
at the the heterodyne band mixer. resulting broadband noise floor HP 8662A/8663A
is
less than -148 dBc
at offsets greater than 1 MHz.
Table 3.2
Typical HP 8662A/8663A residual SSB phase noise.
Carrier Frequency
Main-
+4
120 to
160 MHz
-119
-129
-138
-147
-145
-H2-
160 to
320 MHz
-113
-124
-133
-142
-142
band
320 to
640 MHz
-107
-119
-128
-136
-136
X2
640 to
1280 MHz
-101
-in
-122
-130
-130
are
of
each
the
in
the doubled
in
the divide-
to
cancel-
it is
remains
of
the
in or
in all
inputs
The
of
X4
1280 to
2560 MHz*
-95
-106.
-116
-124
-124
in
be
the
of
the
'
•
be
or
to
11
Page 12
m#n,
er
4
Improving Frequent^ Stability With External References
',V
"^°1Si'
' •
<*
1^
Jv <?img&ffif - -SS'
A synthesizer source are derived from a single fixed-frequency
reference oscillator, where short-term stability translated
examines ence oscillator affects output frequency
8663A. shows bility
of the HP internal reference output signal. a specific case an external reference the close-in short-term stability as
the HP 8662A/8663A. This specific case then expanded arbitrary external reference ity parameters
Why
Use an
The internal reference 8663A absolute phase noise
frequency stability
8663A apply only with this internal erence. Often, however, erence accepts level
of 1 V^
reference 50 ohms.)
often desirable components common reference. in
the
system reference, stability altered. Since erence does alter these frequency stability parameters, ence
can be
Reference Effects
is
in
The
how the
long-term stability
defined
which
all
output frequencies
to the
how the
of the
output. This chapter
stability
the
of the HP
first part
of the
long
and
8662A/8663A's
are
The
translated
chapter then describes
of
using a cesium beam
to
to
discuss
of the HP
External Reference?
is a 10 MHz
is
used.
any
external
±0.1
at a
level
For
example,
of the
is
the
long-
of the HP
the use of an
used
in the HP
crystal oscillator.
and
of the HP
(The HP
5 MHz
V or any 10 MHz
of 0.5 to 0.7 V_
to
operate
system from If
another reference
chosen
and
8662A/8663A will
an
to
improve them.
on
as a
signal
the
long-
reference
of the
stability
short-term sta-
improve both
of the the
on the
8662A/8663A.
long-term
an
external
8662A/8663A
in a
all the
as the
short-term
external
external refer-
refer-
of the 8662A/ chapter
own
to the
as
well
effect
stabil-
8662A/
8662A/
standard
rms
system
a
common
is
of an
ref­ ref-
into
it is
ref-
Long-Term
and
as
is
The
at a
be
Stability
Frequency stability degree
to produces a specified period
of frequency stability includes cepts dental modulation, ations
which
the
of
random noise, residual
of the
can be
the
same frequency throughout
of
and any
output frequency.
defined
oscillating source
time. This definition
as the
the
con-
and
other fluctu-
inci-
synthesizers, in fractional parts week, month, ity usually results from aging components oscillating source.
For
the HP ship between the reference
of
the Because process, racy
of the
of
the or external.
The internal reference 8663A
oscillator with specified long-term stabil­ity
of 5 X 10-10 per day warmup. function rate,
temperature effects, age effects. These parameters translated output frequency.
If
an
external reference
HP 8662A/8663A long-term stability
be either degraded
long-term stability
crystal oscillators A secondary standard such ium oscillator the order mary frequency standards such cesium beams have even less frequency drift—specifying stability 5 parts
in 10"12 for the
beam tube.
it is
commonly expressed
of a
cycle
per day,
or
year. Long-term stabil-
and
materials used
8662A/8663A,
the
long-term stability
and the
output frequency
of the
nature
the
frequency drift
output signal
reference, whether
is an
oven-controlled crystal
The
frequency accuracy
of
time base calibration, aging
to the HP
or
for
is 1 X
has
of 1 X 10~n per
long-term stability
the
long-term stability
is
simple.
of the
and
is
equal
it is
in the HP
after a 10-day
and
8662A/8663A
is
used,
improved. Typical
room temperature
10"6 per
as a
month. Pri-
on the
life
of the
of the
in the
relation-
of
synthesis
accu-
to
that
internal
8662A/
is a
line volt-
are
directly
the
can
month.
rubid-
on
as
order
of
cesium
Offset from Signal
f
1
Hz
10 Hz
100 Hz
1
kHz
10 kHz-
Effect
of the
Reference
on
Short-
Term Stability
A common measure frequency stability (SSB) phase noise; discussion implications. of phase noise residual noise synthesizer; that limit synthesizer. signal residual noise.
Absolute noise present lute noise includes of
the
with different references.
To examine ence oscillator translates the absolute noise
8663A, consider HP 8662A/8663A absolute
SSB phase noise (Figure 4.1). Note that the absolute noise with erence only than about 2 kHz. than 2 kHz, same reference typical phase noise
4.1. translated at a carrier frequency
plotted
ical phase noise 8663A
of
and
absolute. Residual phase
is the
phase noise inherent
on the
for
This phase noise
noise performance
The
can
never
or
total noise
reference used,
how the
is
greater than
offsets from
the
as the
absolute noise.
in the HP
to the
on the
in
Figure
of
short-term
is
single-sideband
see
phase noise
In a
are
at the
same graph with
Chapter
synthesizer,
usually specified—
is, it is a
noise
on the
be
better than
is the
device output. Abso-
the
noise contribution
and
noise
of the HP
the
plot
the
the
For
offsets greater
residual noise
8662A/8663A
as
shown
at 10 MHz is
equivalent phase noise
of 500 MHz and is
of the HP
4.1.
2 for a
and its
two
theoretical
of the
output
the
total phase
will change
on the
to or
affects
8662A/
of
typical
and
the
internal
residual noise
carrier less
is the
The
in
8662A/
types
in the
refer-
residual
ref-
internal
has
Table
the
typ-
Phase Noise Ratio
^(f)
-90 dBc
-120 dBc
-140 dBc
-157 dBc
-160 dBc
Long-term stability, often called fre­quency drift, refers output frequency over a period usually greater than
12
to the
a few
change
of
seconds.
in
time
For
Table 4.1.
HP 8662A/8663A internal reference oscillator phase noise.
Page 13
The graph shows that the absolute phase noise of the HP 8662A/8663A closely follows the translated noise of the refer­ence to about 2 kHz offset from the car­rier. Beyond 2 kHz offset, the noise on the reference oscillator remains flat,
while the absolute noise of the HP 8662A/8663A continues to drop until it reaches the residual noise level. For offsets greater than about 2 kHz, the typical phase noise of the reference oscil­lator is actually greater than the typical absolute noise of the HP 8662A/8663A.
would be about -124 dBc at a 100 kHz offset. The filters, however, effect sub­stantial noise reduction, with about 35 dB of noise attenuation, to reduce the broadband noise floor to about —160 dBc. In addition to the noise reduc-
tion effected by the crystal filters, the bandwidths of the phase-locked loops were carefully chosen to minimize broadband noise. However, most of the noise reduction is due to the filtering. For more information on the design of the HP 8662A/8663A and the reference section, see Chapter 3.
HP 8662A/8663A Stability Using a
Cesium-Beam Reference
An excellent external reference source for improving the long-term stability of the HP 8662A/8663A is a cesium beam frequency standard. To see how the noise of a cesium standard affects the short-term stability or absolute noise of
the HP 8662A/8663A, and to expand that to the general effect of using an external reference, this section examines the measured absolute noise performance of the HP 8662A/8663A with the Hewlett-Packard Model 5061A Cesium Beam Frequency Standard (with high stability Option 004 for improved phase noise) as an external reference.
A good insight into the expected noise performance of the HP 8662A/8663A with the cesium-beam standard as an external reference can be gained by com­paring the specified single-sideband phase noise of the HP 5061A to that of the HP 8662A/8663A 10 MHz internal reference. Figure 4.2 plots these noise characteristics, with the noise of the
5 MHz HP 5061A converted up to the
equivalent noise at 10 MHz.
Figure 4.1.
Comparison of HP 8662A/8663A noise vs. noise of
internal reference.
The reference section of the HP 8662A/ 8663A was designed to ensure that this high reference noise at offsets greater than 2 kHz would not contribute to the absolute noise of the output signal; that
is,
the reference section includes filters to improve the broadband noise perform­ance over the noise of the internal refer­ence.
In the reference section, the
10 MHz reference signal is directly mul­tiplied up to 640 MHz for use in other parts of the HP 8662A/8663A.
Were nothing else done to this 640 MHz signal, the broadband noise would be translated to the output frequency. How­ever, to improve the broadband noise, monolithic crystal filters were added in the reference multiplier chain at 40 and 160 MHz. The 40 MHz filter has a band­width of about 6 kHz; the 160 MHz filter a bandwidth of about 18 kHz. With no filtering, the noise floor on the multiplied-up reference signal (640 MHz)
In summary, due to the design and fil­tering of the reference section, the noise
of the reference oscillator primarily affects the close-in absolute phase noise of the HP 8662A/8663A. Up to about 2 kHz, the dominant noise mechanism is that of the multiplied-up reference sec­tion. Beyond 2 kHz, the crystal filters in the reference multiplier chain filter the reference oscillator noise and the broad­band noise floor reaches the HP 8662A/ 8663A residual noise level. Absolute
noise can be improved by using a lower­noise reference. Again, by the definition
of residual noise, no external reference,
no matter how low in noise, could reduce the absolute noise of the HP 8662A/8663A to anything less than the residual noise. If the noise of the external reference is actually lower than the residual noise of the HP 8662A/ 8663A, the HP 8662A/8663A's residual
noise would dominate.
The phase noise of the HP 8662A/ 8663A internal reference is graphed with a dashed line for offsets from the carrier less than 1 Hz because the phase noise is actually specified only for offsets greater than 1 Hz. Phase noise information at offets greater than 1 Hz is normally
suf­ficient for those applications where a crystal would be used. However, the time domain stability (fractional­frequency deviation) for averaging times from tau equal to 10~3 to 102 seconds is specified for the HP 8662A/8663A refer­ence oscillator. These time-domain repre­sentations of short-term stability were translated to equivalent frequency­domain representations for offsets less
than 1 Hz by algebraic calculations
accepted by the U.S. National Bureau of Standards (NBS). For more information
on how to perform these translations, see NBS Technical Note 679, "Frequency Domain Stability Measurements: A Tutorial Introduction."
Figure 4.2 shows that the phase noise of the HP 5061A Cesium Beam is greater than that of the HP 8662A/ 8663A reference oscillator for offsets from the carrier greater than approxi­mately 2 Hz. Since the bandwidth of the first crystal filter in the HP 8662A/
8663A reference section at 40 MHz is
13
Page 14
J . ."wr-.■"■<-••■■•.
i. ••- * ■:
•*.*,■;•"
> ■
'©&
wasKa
-20
-40
Iv.
•
1
*Ve' *
Internal Oscillator
Figure
4.2.
Noise comparison HP 5061A cesium beam.
approximately this higher noise would about
4 kHz
fore,
the HP 8662A/8663A with as
an
external reference the absolute noise with erence
at because loop bandwidths 8663A, this higher reference noise eventually attenuated until noise
is
of
internal reference oscillator
6 kHz,
attenuation
not
from
the
absolute noise
carrier. There-
of the
the HP
is
the
offsets greater than
of the
filtering
and
in the HP
dominant.
start until
5061A
higher than
internal
2 Hz. But effect 8662A/
the
residual
femnre
Re
10
100
Offset From Carrier
vs.
standard reaches filtering
of
ues that HP 8662/8663A continues offset from though broadband noise floor.
ref-
of
is
To show tages external reference, Figure the measured absolute noise
fc =
10 MHz
5061A
Opt.
^
(Hz)
in the HP
to
attenuate
the
absolute phase noise
the
of
using a cesium beam
the
the
carrier increases, even
reference
the
advantages
10K
100K
its
noise floor. Here
8662A/8663A contin-
reference noise
of the
to
decrease
has
reached
and
its
disadvan-
as an
4.4
compares
of the
the
so
HP 8662A/8663A with
crystal reference,
the
absolute noise with the cesium frequency standard, typical residual phase noise HP 8662A/8663A. Figure
the noise than oscillator 2 Hz. is translated of references
of the
the
As
the HP
cesium standard
noise
of the
for
offsets less than about
internal crystal
expected, this same relationship
to the
absolute phase noise
8662A/8663A when these
are
used.
The phase noise (less than HP 8662A/8663A of
the HP
Standard
5061A Option
as an iting greater than at
0.01 Hz
is
improved with
external reference, exhib-
10 dB of
offset, with improvement increasing the carrier decreases.
For offsets greater than lute phase noise 8663A with Cesium Standard
greater than
of the HP
the HP
5061A Option
as a
the
absolute noise with HP 8662A/8663A internal oscillator, predicted. standard continues
as
noise with
The
noise with
to be
the
internal crystal until
HP 8662A/8663A crystal filters
ficiently attenuate reference-noise floor residual noise. Figure reduction occurs carrier
of
at
around
the
160
tent with filter approximately
the
to
at an
25
kHz. This
fact that
MHz has a
18 kHz.
4.4
the
its own
internal
and the
of the
4.2
shows that
is
lower
very close-in
1 Hz
offset)
of the
use
004
Cesium
improvement
the
amount
as
2 Hz, the
of
offset from
abso-
8662A/
004
reference
is
the
as
the
cesium
higher than
the
the
can suf-
cesium's higher
less than
the
shows that this
offset from
is
consis-
the
second crystal
bandwidth
of
two
Figure
4.3
shows
the noise results; the
HP
the
8662A/8663A with HP 5061A Option is shown 100 kHz. between
for
offsets from
To
examine
the
noise
absolute phase
absolute phase noise
004
Cesium Standard
the
of the the resultant absolute noise HP 8662A/8663A, noise
of the
to
the
equivalent noise
also plotted.
nal reference, close for offsets less than phase noise very closely follows of
the
reference used. Between
and 1
kHz, the
the
HP
8662A/8663A generally follows the noise curve except that
cesium
the
is
smoothed
offsets greater than
14
the
specified phase
cesium standard converted
at 500 MHz is
As in the
case
to the
10 Hz) the
of the HP
8662A/8663A
the
noise spectrum
absolute phase noise
of the
cesium reference,
noise "plateau"
out by
1 kHz, the
the
0.1 Hz to relationship
reference
and
of the
of the
inter-
carrier (here
absolute
10 Hz
of the
filtering.
For
cesium
of
of
Figure
4.3.
Effect
of
cesium beam frequency standard
HP 8662A/8663A absolute noise.
on
Page 15
jr->3
.Ktw >--,-( T^..
■"jf^y*
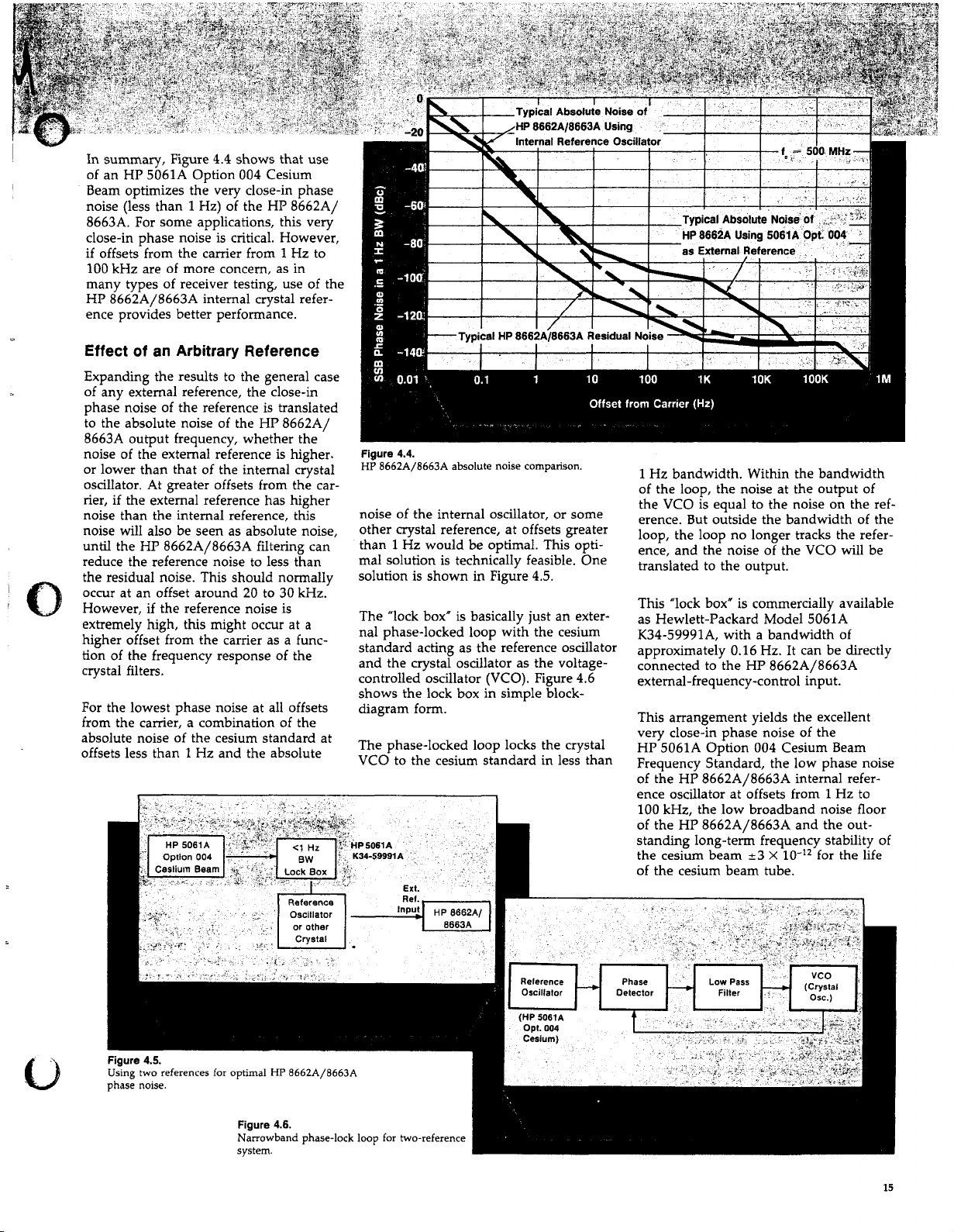
In summary, Figure
of
an HP
5061A Option Beam optimizes noise (less than 8663A.
For
some applications, this very close-in phase noise if offsets from
100
kHz are of
many types
of
4.4
shows that
004
the
very close-in phase
1 Hz) of the HP
is
the
critical. However,
carrier from
more concern,
receiver testing,
use
Cesium
8662A/
1 Hz to
as in
use of the HP 8662A/8663A internal crystal refer­ence provides better performance.
Effect
of an
Arbitrary Reference
Expanding of
any phase noise to
the 8663A output frequency, whether
noise or lower than that
oscillator.
rier, noise than noise will also until
reduce
the
results
external reference,
of the
absolute noise
of the
external reference
At
if the
the HP
greater offsets from external reference the
internal reference, this be
seen
8662A/8663A filtering
the
reference noise
to the
the
reference
of the HP
of the
internal crystal
as
absolute noise,
to
general case
close-in
is
translated
8662A/
the
is
higher.
the
has
car-
higher
can
less than the residual noise. This should normally occur
at an
However,
if the
reference noise extremely high, this might occur higher offset from tion
of the
frequency response
offset around
the
carrier
20 to 30 kHz.
is
at a
as a
func-
of the
crystal filters.
For
the
lowest phase noise
from
the
absolute noise
carrier, a combination
of the
offsets less than 1
HP 5061A
Option
Caslium Beam
~
'
Hz and the
004
at all
offsets
of the
cesium standard
absolute
*
■*
<1
BW
Lock
Reference
Oscillator
or other
Crystal
at
Hz
Box
I
in
o.01
Figure
4.4.
HP 8662A/8663A absolute noise comparison.
noise
of the other crystal reference, than 1
Hz mal solution solution
The "lock
would
is
technically feasible.
is
shown
box" is
at
be
optimal. This opti-
in
Figure
basically just
nal phase-locked loop with
internal oscillator,
standard acting and
the
crystal oscillator
as the
reference oscillator
or
offsets greater
4.5.
an
the
cesium
as the
voltage­controlled oscillator (VCO). Figure shows
the
lock
box in
simple block-
diagram form.
The phase-locked loop locks VCO
to the
cesium standard
HPS061A
K34-59991A
Ext.
Ref.
Input
HP 8662A/
8663A
the
in
less than
Offset from Carrier
some
One
exter-
4.6
crystal
100
1K 10K 100K
(Hz)
1
Hz
bandwidth. Within
of
the
loop,
But
the
loop
and the
to the
box" is
the equal outside
the
VCO is erence. loop, ence,
translated
This "lock
noise
no
noise
output.
the
bandwidth
at the
output
to the
noise
the
bandwidth
longer tracks
of the VCO
of
on the ref-
of the
the
refer-
will
commercially available as Hewlett-Packard Model 5061A K34-59991A, with a bandwidth approximately connected
0.16 Hz. It can be
to the HP
8662A/8663A
of
directly
external-frequency-control input.
This arrangement yields very close-in phase noise HP 5061A Option Frequency Standard,
of
the HP
ence oscillator
100 of
the HP
8662A/8663A internal refer-
at
kHz, the low
8662A/8663A
the
excellent
of the
004
Cesium Beam
the low
offsets from 1
phase noise
Hz to
broadband noise floor
and the
out­standing long-term frequency stability the cesium beam of
the
cesium beam tube.
±3 X 10~12 for the
life
be
of
Figure
4.5.
Using
two
phase noise.
references
for
optimal
HP
8662A/8663A
Figure
4.6.
Narrowband phase-lock loop system.
for
two-reference
Reference
Oscillator
(HP 5061A
Opt.
004
Cesium)
Phase
Detector
Low Pass
Filter
VCO
(Crystal
Osc.)
.'""
%
.-
-»
15
Page 16
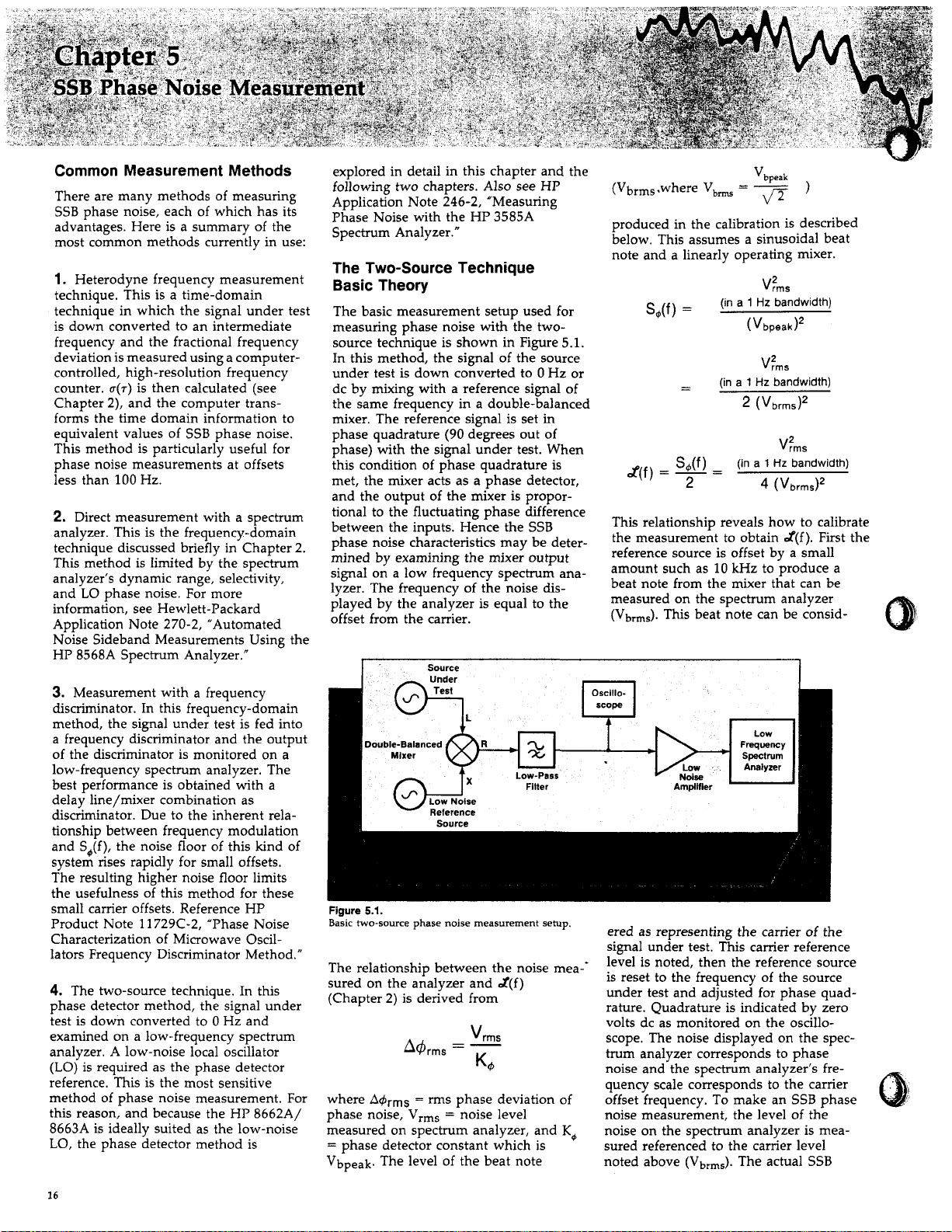
>T;SS5 Phase Noise Measurenierit
.,-f^>W
Common Measurement Methods
There are many methods of measuring SSB phase noise, each of which has its advantages. Here is a summary of the most common methods currently in use:
1.
Heterodyne frequency measurement technique. This is a time-domain technique in which the signal under test is down converted to an intermediate frequency and the fractional frequency deviation is measured using a computer­controlled, high-resolution frequency counter. a{r) is then calculated (see Chapter 2), and the computer trans­forms the time domain information to equivalent values of SSB phase noise. This method is particularly useful for phase noise measurements at offsets less than 100 Hz.
2.
Direct measurement with a spectrum analyzer. This is the frequency-domain technique discussed briefly in Chapter 2. This method is limited by the spectrum analyzer's dynamic range, selectivity, and LO phase noise. For more information, see Hewlett-Packard Application Note 270-2, "Automated Noise Sideband Measurements Using the HP 8568A Spectrum Analyzer."
3.
Measurement with a frequency
discriminator. In this frequency-domain
method, the signal under test is fed into
a frequency discriminator and the output
of the discriminator is monitored on a low-frequency spectrum analyzer. The best performance is obtained with a delay line/mixer combination as discriminator. Due to the inherent rela­tionship between frequency modulation and S if), the noise floor of this kind of
system rises rapidly for small offsets. The resulting higher noise floor limits the usefulness of this method for these small carrier offsets. Reference HP Product Note 11729C-2, "Phase Noise Characterization of Microwave Oscil-
lators Frequency Discriminator Method."
4.
The two-source technique. In this phase detector method, the signal under test is down converted to 0 Hz and examined on a low-frequency spectrum analyzer. A low-noise local oscillator (LO) is required as the phase detector reference. This is the most sensitive method of phase noise measurement. For this reason, and because the HP 8662A/ 8663A is ideally suited as the low-noise LO,
the phase detector method is
explored in detail in this chapter and the following two chapters. Also see HP Application Note 246-2, "Measuring Phase Noise with the HP 35 85A Spectrum Analyzer."
The Two-Source Technique
Basic Theory
The basic measurement setup used for
measuring phase noise with the two-
source technique is shown in Figure 5.1.
In this method, the signal of the source under test is down converted to 0 Hz or dc by mixing with a reference signal of the same frequency in a double-balanced mixer. The reference signal is set in phase quadrature (90 degrees out of phase) with the signal under test. When this condition of phase quadrature is met, the mixer acts as a phase detector, and the output of the mixer is propor­tional to the fluctuating phase difference between the inputs. Hence the SSB phase noise characteristics may be deter-
mined by examining the mixer output signal on a low frequency spectrum ana­lyzer. The frequency of the noise dis­played by the analyzer is equal to the offset from the carrier.
Source
Under
Test
Figure 5.1.
Basic two-source phase noise measurement setup.
The relationship between the noise mea­sured on the analyzer and Jf(f) (Chapter 2) is derived from
v
A0rms =
Ad>
rms
= rms
rms
where phase noise, V measured on spectrum analyzer, and K.
= phase detector constant which is
^bpeak- The level of the beat note
n
Krf
phase deviation of
= noise level
bpeak
(Vbrms-where V
= -^=
brms
)
produced in the calibration is described below. This assumes a sinusoidal beat note and a linearly operating mixer.
v?
ms
vf
ms
brms
vf
4 (V
2
)2
ms
brms
)2
(Vbpeak)
2 (V
(in a 1 Hz bandwidth)
m
of(f) =
(in a 1 Hz bandwidth)
=
(in a 1 Hz bandwidth)
_
S,(f)
2
This relationship reveals how to calibrate the measurement to obtain eJf(f). First the
reference source is offset by a small amount such as 10 kHz to produce a beat note from the mixer that can be measured on the spectrum analyzer (V
).
This beat note can be consid-
brms
ered as representing the carrier of the signal under test. This carrier reference level is noted, then the reference source is reset to the frequency of the source under test and adjusted for phase quad­rature. Quadrature is indicated by zero volts dc as monitored on the oscillo-
scope. The noise displayed on the spec­trum analyzer corresponds to phase noise and the spectrum analyzer's fre­quency scale corresponds to the carrier
offset frequency. To make an SSB phase noise measurement, the level of the noise on the spectrum analyzer is mea­sured referenced to the carrier level noted above (V
). The actual SSB
brms
16
Page 17
phase noise level ing because equation above.
There
are two calibrate One generates a very low-level sideband by angle modulating sources
generates a very low-level sideband
summing
the level carrier can The phase-noise level measured relative reference level. When angle modulating one
required, when summing signal previously sideband level SSB phase noise level when calibrating using angle modulation. When summing signal, —96 dBc/Hz levels.
The noise measured technique described above represents
combined noise test
upper limit device, however,
one rately source phase noise well characterized choice introduced bution
the
at a low
in a
of the
is
accurately known,
be
used
of the
the 6 dB
is set to
is 50 dB
the SSB
and the
of the two
the
phase noise
can be
as a
by the
of the
is 6dB
below this read-
of the
factor
of
V*
in the
other methods used
two-source measurement.
one of the two
level.
The
other
discrete low-level signal.
sideband relative
to
indicate a reference level.
can
to the
sideband
sources
is
for the
reference source.
no
correction factor
correction mentioned
needed.
—40
below
phase noise level
for the
of
reference source. This
if the
sources
determined. Since
of the HP
it is an
finite noise contri-
reference
in a
For
example,
dBc and the
the
sideband,
is
—90 dBc/Hz
same reference
by the
both
the
phase noise
phase noise
is
known accu-
of the
8662A/8663A
excellent
is
given
to the
the
sideband
then
be
discrete
in a
discrete
is
two-source
source under
of
either
other
the
The
error
by:
to
by
is
if the noise
the
the
is the
of
is
two-source technique will
0.5
last
If
dB of the
under test.
If
the
source under test within phase noise rately determined sources
ments with three different source com­binations yield sufficient data late accurately Appendix A gives formulas calculation.
Because phase noise in
a 1 Hz
from
the be corrected bandwidth This bandwidth normalization process simply requires subtracting (equivalent noise bandwidth the measured value. value
of measurement with a spectrum analyzer equivalent noise bandwidth this value must
ing
10 log Hz. Most Hewlett-Packard analyzers have equivalent noise band­widths
actual noise
10 dB of the
of the
are
bandwidth,
above measurement must also
of the
—123
(1200), yielding -153.8
of
approximately
if
available. Three measure-
the
for the
spectrum analyzer.
dBc is
be
corrected
be
of the
has
reference
source
can be
three unknown
noise
of
each source. for
is
usually specified
the
result obtained
equivalent noise
10 log
For
obtained from
in Hz)
example,
of 1.2 kHz,
by
RF
1.2
times
within
source
phase noise
the
actual
accu-
to
calcu-
this
from
if a
a
subtract-
dBc/
spectrum
the
measured using a synthesized signal generator
In addition normalization correction factors explained above, other correction factors may of spectrum analyzer used. Most analog
spectrum analyzers amplifiers amplifier amplifies peaks less than rest even though calibrated detector tends is lower than
responding these effects, noise measured analyzer actual noise level. Thus a correction factor measured value
amplification further explanation analyzer corrections, refer Packard Application Note 150-4, "Spectrum Analysis Measurements."
The Importance
The two-source technique explained above
as a
calibrated source.
to the 6 dB, and
be
required, depending
and
peak detectors.
of the
noise signal.
the
spectrum analyzer
to
read
rms
to
produce a reading that
the
true
to
random noise.
the
resulting value
on the
is
about
2.5 dB
of 2.5 dB
must to
and
peak detection.
of
may be
applied directly
bandwidth
on the
use
logarithmic
In
addition,
values,
rms
value when
Due to
spectrum
less than
be
added
compensate
of
spectrum
to
Hewlett-
...
Noise
Quadrature
type
The log
the
is
the
peak
of
the
to the for log
For
if
both
Error
(dB) = 10 LOG (1 + P
where
P
, =
Actual Noise Power
ret
Reference Source
P
=
Actual Noise Power
dut
Source Under Test
and Error
■*(f)M™0 (^ dB) =
This equation indicates that
able lowest possible noise. is known that
one-tenth under test,
is
to use a
as
defined
as
^f(f)
reference source with
For
the
reference
much noise
the
noise measured using
as the
ref/Pdut
of the
of the
(in dB)
ACTuAL
it is
desir-
example,
has
about
source
)
the
if it
the
Figure
5.2.
Typical double-balanced mixer phase detector characteristic.
3
dB
bandwidth
that
the 3 dB
is
not
necessarily equal panel resolution bandwidth setting, since the front-panel setting ure.
For
best accuracy,
width
of the
of the
analyzer. Note
bandwidth
analyzer used should
of the
to the
is a
nominal fig-
the 3 dB
analyzer
front-
band-
be
sources have sufficient long-term phase stability during tance
typical phase detector characteristic curve in Figure point the center
to
stay
in
phase quadrature
the
measurement.
of
quadrature
of a
double-balanced mixer shown
5.2. The
of
maximum phase sensitivity
of the
region
The
is
illustrated
curve shows that
of
linear opera-
impor-
by the
the
and
17
Page 18
»i T-'fW
-W^!??'
tion occur where the phase difference between the two inputs (0Lo ~~ equal to 90 degrees (phase quadrature). Any deviation from quadrature results in a measurement error given by:
error(dB) = 20 log [cos(magnitude of phase deviation from quadrature)]
where error is defined as cJf(f)measured in dB minus <Jf(f )actuai i the error in dB is always negative, that is,
the measured noise will always be
less than the actual noise.
Since the phase detector constant K^ can be measured (K0 = V acceptable measurement error the permissible deviation from zero volts dc of the average mixer output voltage can be calculated using the phase detector characteristic curve. This is given by:
deviation from zero volts dc=
K0v -io
.=-:^.-
n d
B. Note that
), for a given
bpeak
error(dB/5
>
</>RF)
;
is
As an example, suppose K^ has been measured to be 0.15 volts/radian. If it is desired to keep the measurement error due to deviation from quadrature less than -0.5 dB, the oscilloscope should be monitored during the phase noise
measurement to ensure that the average mixer output voltage is within the range of ±68 millivolts.
The quadrature condition represents not only the point of maximum phase sensi­tivity but also the point of minimum AM
noise sensitivity. As the two mixer inputs drift out of quadrature and the phase noise sensitivity decreases, the
AM noise sensitivity of the mixer increases. Such increased sensitivity to AM noise may cause an additional measurement error if the source under test has high AM noise.
Phase-Locked Measurements
If the two sources cannot stay suffi­ciently close to quadrature during the phase noise measurement, a "phase­locked" measurement must be made. This involves phase-locking one of the sources to the other by connecting the
mixer output to a frequency control line
on one of the sources. This causes that source to track the other source in phase. Thus,
if the two sources have been set in phase quadrature, they will remain in quadrature. The bandwidth of the phase­locked loop must be set much lower than the lowest offset at which phase noise is to be measured. This is necessary because the tracking of phase-
locked loops attenuates phase noise
within the loop bandwidth, and this
attenuation causes the phase noise to appear lower than it actually is. An example of a phase-locked phase-noise measurement is discussed in Chapter 6. Alternatively, if it is not possible to make the bandwidth smaller than the
offsets of interest, a correction must be made for the attenuation of the noise sidebands by the action of the loop.
~\jr
18
Page 19
'it +-, •
Chapter 6 ;X
.;'{,",
' f
Measuring SSB Phase Noise with the HP
*
-^**,v«
The extremely-low SSB phase noise and
excellent long-term stability of the HP 8662A/8663A allow them to serve in many cases as the low-noise reference source required in the two-source tech­nique, as discussed in Chapter 5. The following sections describe the use of the HP 8662A/8663A in measuring SSB phase noise and extends these tech­niques to include automation via the Hewlett-Packard Interface Bus (HP-IB). Chapter 7 discusses the use of the
HP 8662A/8663A as a low-noise refer­ence multiplied up to microwave fre­quencies for phase-noise measurement of microwave sources.
SSB Phase-Noise Measurements on Sources Operating from a
Common Reference
An HP 8662A/8663A-based system for measuring the SSB phase noise of sources that operate from a 5 or 10 MHz reference oscillator is shown in Fig­ure 6.1. Note that the system uses the basic two-source technique, except that the frequency reference for the device under test, a synthesizer in this example, is the 10 MHz rear-panel reference output of the HP 8662A/8663A. A 5 or 10 MHz external reference oscillator could also be used. Since both sources
have the same reference, they remain in phase quadrature once quadrature is set, provided that the source under test has
adequate phase stability. A second method for locking an HP 8662A/8663A in quadrature to a free running source is discussed in this chapter under the head­ing Phase-Locked Measurements Using the HP 8662A/8663A DC FM Mode.
When making a phase-noise measure­ment with the system in Figure 6.1, it is important to note that any phase noise on the output of the synthesizer under test which is correlated with the noise at the HP 8662A/8663A output will be cancelled in the double balanced mixer. That portion of the reference-oscillator noise that is present at the outputs of both sources correlates if the total signal
paths through the two sources introduce
the same time delay. Thus, under these conditions, the common reference oscil­lator noise cancels and the noise mea­sured by the system is equal to the residual noise of the source under test after correction factors for the HP 8662A/8663A residual noise contri­bution are applied. Due to the crystal fil­tering in the reference section of the HP 8662A/8663A, the absolute HP 8662A/8663A noise is correlated to its reference only at carrier offsets less than about 3 kHz.
Thus,
this system is limited to residual phase noise measurements at offsets less than 5 kHz and then only if the time delays through the HP 8662A/
8663A and the synthesizer under test are equal. At offsets greater than this, or at offsets greater than the loop band­width of the device under test, which­ever is greater, the noise measured by the system is the absolute noise of the synthesizer under test.
The HP 8662A/8663A 10 MHz reference output supplies greater than 0.5 V,^ into 50 ohms. If this is insufficient to drive the synthesizer under test, addi­tional amplification may be added pro-
8662A/8663A'''/':;' -K
vided care is taken to ensure that the amplifier does not add to the reference oscillator's noise level. A typical 10 MHz amplifier circuit that will give good results is shown in Appendix cuit is similar to that used in the
HP 8662A/8663A reference section.
COMPONENT CONSIDERATIONS
Because the components in the system of Figure 6.1 are important in determining the system's measurement limits, they
are discussed in detail below.
The Phase Detector
Any double-balanced mixer specified for operation at the frequency of the synthe­sizer under test will serve as a phase detector. The IF output port of the mixer
must be DC coupled to make measure-
ments very close to the carrier. Mixers
specified for higher power levels provide more sensitivity by accommodating higher carrier levels and thus increased carrier-to-noise floor ratios. Linear mixer operation is especially important to avoid errors during system calibration. (To avoid operating in the non-linear region of the mixer, input power levels can be reduced at the cost of reduced sensitivity.) Several excellent mixers for this purpose are available from commer­cial sources. This system uses a Hewlett-
Packard Model 10514A for measure­ments up to 500 MHz.
The Low-Pass Filter
The low-pass filter prevents LO feed­through and mixer sum products from overloading the low-noise amplifier or the input of the spectrum analyzer. In theory, any general-purpose low-pass network with a cutoff frequency suffi­ciently above the highest offset fre­quency of interest may be used. How­ever, many passive filters terminate the mixer in a reactive load at RF frequen­cies.
As a result, the mixer sum products are reflected back into the mixer, causing distortion of the phase slope. To avoid this,
the low-pass filter should be pre-
ceded by a simple decoupling network
that terminates the mixer in 50 ohms at the sum product frequency (twice the
carrier frequency of the signal under test).
•'"'
•:*/^vf*'^
B.
This cir-
$&
Figure 6.1.
Measuring phase noise on sources with a common reference.
Figure 6.2 shows an example of a two­pole,
low-pass filter that correctly termi-
nates the mixer sum frequencies above
10 MHz, yet unloads the mixer at the
lower frequencies where the noise volt-
19
Page 20
age fluctuations of interest occur. Rl and Cl terminate the mixer properly. R2 and C3 provide a decoupled means of monitoring quadrature on the oscillo­scope without introducing further noise. The values given for LI and C2 set a 90 kHz
cutoff.
not introduced into the measurement. The linear input range should be approximately 30 to 50 dB below the carrier level for unattenuated beat note calibration. The reasons for this con­straint are made clear by the system cal­ibration explanation in the following
3.
bandwidth normalization allowing
noise levels to be read directly in dBV/Hz.
4.
relative amplitude values presented
directly in dB.
5.
digital display with alphanumeric readout of spans, marker frequency, and marker amplitude.
An additional feature of the HP 35 82A is its high speed. It is well suited for low frequency, close-in measurements. The HP 3585A provides measurements at wide offsets.
MEASUREMENT PROCEDURE
The manual measurement discussed in this section uses the HP 35 82A Spectrum Analyzer because of its speed in swept close-in measurements. The automated SSB measurements which follow demon­strate the efficiency of the HP 3585A Spectrum Analyzer for automated spot
measurements at predetermined offsets.
Figure 6.2.
Low-pass filter for two-source measurement
The Quadrature Monitor
Any general-purpose, dc-coupled oscillo­scope will do for determining the phase detector constant K^ (volts/radian) as discussed in chapter 5, and for setting and monitoring quadrature. The Hewlett-Packard 1745A works well for
this purpose. Although a dc voltmeter can be used to set and monitor quadra­ture,
an oscilloscope is much more useful for time domain inspection of the phase noise signal. Digital voltmeters have the added disadvantage of introducing noise
in very sensitive measurements.
The Low-noise Amplifier
The low-noise amplifier (LNA) improves the sensitivity and noise figure of the spectrum analyzer. The
requirements of this amplifier are deter­mined by the levels of phase noise to be measured and the dynamic range of the spectrum analyzer. In some
instances, the LNA may not be required. However, critical low-noise measurements call for this additional amplification. In general, the amplifier
should have a low-frequency cutoff well below the lowest offset frequency to be measured. Consideration must also be given to the noise floor and 1/f noise of the amplifier so that additional noise is
section. A circuit for a typical low noise amplifier that meets these requirements is shown in Appendix C. If the device used (2N6428) is hand selected for low
1/f noise, noise figures as low as 10 dB at 10 Hz may be achieved. This is the LNA used in the system of Figure 6.1.
The Spectrum Analyzer
The spectrum analyzer should be a high­sensitivity, low-frequency (up to highest offset measured) analyzer capable of providing narrow resolution bandwidths. The HP 3585A Spectrum Analyzer is a good choice for automated spot measure­ments of SSB phase noise over a wide offset range (20 Hz to 40 MHz). The HP 3582A Spectrum Analyzer uses Fast Fourier Transform Techniques and is
efficient for rapid measurements of close-in phase noise (0.02 Hz to
25.5 kHz). Both manual and automated measurements will be discussed in detail in this chapter.
Following are spectrum analyzer features of the HP 3582A and 3585A that are useful for phase noise measurements:
1.
programmability.
2.
rms-averaging mode for enhanced
noise measurement repeatability.
Calibration
The system is easily calibrated by offset-
ting one of the sources and observing the resultant beat signal on an oscillo­scope or spectrum analyzer. As discussed in Chapter 5, the slope at the zero cross­ing in volts per radian is K^ and for sinusoidal beat signals is equal to the peak voltage of the signal (V
bpeak
). The beat signal as viewed on an analyzer is the rms value and so is 3 dB less than
'the peak.
In order to determine the beat signal
zero crossing slope in volts per radian:
1.
Set the synthesizer under test to the desired carrier frequency, Fc, at a level sufficient to drive the LO port of the mixer.
2.
Set the HP 8662A/8663A frequency
to Fc. Set a frequency increment of
10 kHz. Press
to generate a 10 kHz beat note for cali­bration. Set the HP 8662A/8663A ampli­tude to a level sufficient to drive the RF port of the mixer. For the HP 10514A, the LO should be +10 dBm and the RF 0 dBm. Set an amplitude increment of
40 dB. Press
The attenuation is added to ensure that the low noise amplifier will not be over-
20
Page 21
driven by the 10 kHz beat note. Here, 40 dB is chosen for illustration. The actual amount of attenuation necessary
will vary, depending on the sensitivity required of the measurement, the linear operating range of the mixer, the charac-
teristics of the low noise amplifier, and the output level characteristics of the synthesizer under test.
Note that it makes no difference which source is connected to which mixer input as long as the proper levels are main-
tained. If the synthesizer under test has sufficient output to drive the LO port of the mixer, it is usually more convenient to connect the HP 8662A/8663A to the RF input, since 40 dB of attenuation can be added by simply decrementing the HP 8662A/8663A output level by 40 d&. If the HP 8662A/8663A must be used to
provide the +10 dBm LO drive an exter­nal attenuator such as the Hewlett­Packard Model 355D may be used to provide the required attenuation for the test signal at the RF mixer port.
3.
Set the HP 3582A Spectrum Analyzer for a 0 to 25 kHz span, 10 dB/division, flat top passband, averaging off. Enable the marker and set it on the 10 kHz beat note.
Set a reference at this carrier level
by pressing
a
Enter the relative mode by pressing
To obtain readings in dBc/Hz, enable the
automatic bandwidth normalization by
reSSm
P
S
+
Calibration is complete.
Setting Quadrature
Quadrature setting consists of offsetting the HP 8662A/ 8663A frequency by
0.1 Hz until the two sources are in quad­rature, then resetting the HP 8662A/ 8663A frequency to exactly Fc.
4.
On the HP 8662A/8663A, press
IFREQUENCYj
5.
Set an HP 8662A/8663A frequency
increment of 0.1 Hz (0.2 Hz above
640 MHz). Press
REL
^BW
With the HP 1745A Oscilloscope set at
0.1 volts/div and dc coupled, monitor the 0.1 Hz beat note on the oscilloscope. As the trace passes through 0 volts dc press
to hold the mixer inputs in quadrature. Note: due to the need for phase­continuous HP 8662A/8663A frequency switching in performing this step, the
frequency offset sequence, or the reverse,
depends on the carrier frequency. If the
level on the oscilloscope jumps abruptly to a new offset when the second INCRE­MENT button is pressed use the reverse sequence.
Measurement
6. Set the HP 3582A Spectrum Analyzer to span the desired offset frequency and
increase the input sensitivity in 10 dB steps until the "overload" indicator just remains unlit, then back off one step.
7.
Place the HP 3582A in the RMS aver­age mode, select the desired number of averages and press
RESTART
□
As the HP 3582A takes readings, moni­tor the HP 1745A to ensure that the inputs to the mixer remain within the
desired limits about quadrature.
8. When the HP 3582A is finished aver-
aging, move the marker to the desired offset frequency and note the reading on the screen.
9. Correct the reading taken above by
the following corrections factors: minus 40 dB for the attenuation added during calibration minus 6 dB to convert measured reading to «/(f).
The resulting number is equal to the SSB
phase noise level in dBc/Hz provided
the phase noise level of the reference is at least 10 dB below that of the source under test. If not, the SSB phase noise level is the upper limit of either source. Notice that the HP 3582A does not require any of the spectrum analyzer correction factors discussed previously. This is due to its automatic bandwidth
normalization feature and digital Fast
Fourier Transform operation.
10.
If the phase noise at other offsets not currently displayed on the HP 35 82A is required, repeat steps 6 through 9. Generally, recalibration is not necessary if power levels are unchanged, but quad-
rature may have to be reset from time to
time,
depending upon the stability of the
synthesizer under test.
PRECAUTIONS
The following potential problems should be considered when making the above measurements.
• Non-linear operation of the mixer, due to over-driving, can result in cali­bration error.
• RF signal harmonics can cause K^ to deviate from Vt, tion error.
• The amplifier or spectrum analyzer input can be saturated during calibra­tion or by high spurious signals such as line frequency multiples.
• Closely-spaced spurious may give the appearance of continuous phase noise
when spectrum analyzer resolution is insufficient.
• Interface impedances should remain unchanged between calibration and measurement.
• In residual measurement systems, phase noise of the common reference oscillator may be insufficiently can-
celled due to delay-time differences between the two branches.
• Noise from power supplies can be a dominant contributor to measured phase noise.
• Peripheral instrumentation such as oscilloscopes, analyzers, counters, and DVMs can inject noise.
• Microphonic noise might excite icant phase noise in devices.
This list of potential problems points out that much care must be exercised when very low SSB phase-noise measurements
are made. However, if these points are considered carefully, the system of Figure 6.1 will measure SSB phase noise as low as the phase noise level of the HP 8662A/8663A itself (Figure 4.1). Fig­ure 6.4 shows the SSB phase noise of the HP 8660C Synthesized Signal Generator (top) and the HP 8662A/8663A (bottom) as seen on the HP 3582A Spectrum Ana-
lyzer display. Note the flattening effect of displaying phase noise on a linear fre­quency scale.
k/ causing calibra-
pea
signif-
21
Page 22
Figure 6.4.
Phase-locked two-source phase noise measurement.
■■V**
--M.V-
Phase-Locked Measurements Using the HP 8662A/8663A DC FM Mode
One of the most common phase noise measurements involves measuring the SSB phase noise of a free-running oscil­lator using the two-source technique. Since such an oscillator does not operate from a reference oscillator, phase quad­rature must be maintained by phase­locking one of the two sources to the other. To avoid phase-noise cancellation by loop tracking, the bandwidth of the
phase-locked loop must be much less than the lowest offset frequency of inter­est. Although it makes no difference which source is phase-locked to which, it is generally most convenient to phase­lock the HP 8662A/8663A used as the low-noise reference to the source under test. A system for making phase-locked
phase noise measurements using the DC FM capability of the HP 8662A/ 8663A is shown in Figure 6.3.
The output of the mixer is connected to
the dc-coupled FM input of the HP 8662A/8663A. Because the resulting phase-locked loop is essentially first order, the loop bandwidth can be calcu­lated and is given by the formula
BWf(3 dB) = K0 K
0
where K0= the HP 8662A/8663A "VCO gain constant", in Hz/volt and is just equal to the HP 8662A/8663A front panel FM deviation setting, and K^= phase detector constant, in volts/radian
as
(vbPeak)
gi
ven in
Chapter 5.
When the HP Model 10514A Double-
Balanced Mixer is used with input levels of 0 dBm at the RF port and +10 dBm at the LO port, the following rule of thumb applies: phase noise measurements made at carrier offsets greater than or equal to the HP 8662A/8663A front panel FM peak deviation setting will result in a loop attenuation error of <0.5 dB.
PHASE-LOCKED MEASUREMENT PROCEDURE
The procedure for manual phase-locked measurements of absolute phase noise using the system shown in Figure 6.3 is as follows:
Calibration
The calibration procedure involves mea­suring the level of the carrier so that the spectrum analyzer can make measure­ments of phase noise levels relative to that carrier.
1.
Set the HP 8662A/8663A frequency to the approximate frequency of the oscillator under test. Press
Set the HP 8662A/8663A amplitude to 0 dBm and set an amplitude increment of 40 dB. Press
Set a frequency increment of 10 kHz. Press
, 2. Adjust the HP 8662A/8663A fre-
quency to obtain a beat frequency at the mixer output of approximately 10 kHz.
3.
Set the HP 3582A Spectrum Analyzer for a 0 to 25 kHz span, 10 dB/division, flat top passband shape, averaging off. Enable the marker and set it on the 10 kHz beat note from the mixer. Set a
reference at this carrier level by pressing
Enter the relative mode by pressing
To obtain readings in dBc/Hz, enable the automatic bandwidth normalization by pressing + V BW . Calibration is complete.
Setting Quadrature
The following procedure phase-locks the HP 8662A/8663A to the source under test and adjusts the phase relationship to phase quadrature.
22
Page 23
4.
On the HP 8662A/8663A press
5.
Set the HP 8662A/8663A FM devia-
tion to 1 kHz. Press
o
Adjust the HP 8662A/8663A frequency slowly until phase locking is observed
on the HP 1745A. This is indicated by a constant level on the scope. Adjust the HP 8662A/8663A frequency until that dc level is equal to 0 volts.
Measurement
6. Set the HP 3582A Spectrum Analyzer to span the desired offset frequency and increase the input sensitivity in 10 dB . steps until the "overload" indicator just remains unlit, then back off one step.
7.
Place the HP 3582A in the RMS aver­age mode, select the desired number of averages, and press
from time to time, depending upon the stability of the source under test.
Comments
With very stable sources under test, HP 8662A/8663A FM deviations as
small as 0.1 kHz may be used, enabling phase noise measurements to be made as close to the carrier as 100 Hz. In this
case,
the HP 3582A Spectrum Analyzer can be placed in the single sweep mode and the trigger can be manually "armed" by the operator as the HP 8662A/8663A frequency is adjusted to maintain quad-
rature. The averaging feature can still be used, except that the averages must be taken manually.
This system can measure absolute SSB phase noise as low as that of the HP 8662A/8663A in the DC-FM mode (Figure 6.5).
-80
as phase noise measurements, the most obvious being speed. A second advan­tage lies in the inherent repeatability of automated measurements that results from the elimination of operator error
and inconsistency. Still another advan­tage is apparent in the tremendous data gathering and documentation ability of a desktop computer used in conjunction with a printer, plotter, or CRT display.
An example of an automated system for
residual phase noise measurements is shown in Figure 6.6. This system is based on the Hewlett-Packard Model 9836 Computer and uses the HP 3585A Spectrum Analyzer. Typical system soft­ware written for the HP 9836 is pre­sented in Figure 6.7. The software flow­chart in Figure 6.8 shows that the
software structure corresponds to the manual measurement procedure described in the preceding section.
1
As the HP 3582A takes readings, moni-
tor the HP 1745A to ensure that the inputs to the mixer remain within the desired limits about quadrature.
8. When the HP 3582A is finished aver­aging, move the marker to the desired offset frequency and note the reading on
the screen.
9. Correct the reading taken above by applying the following correction factors:
minus 40 dB for the attenuation during
calibration.
minus 6 dB to convert measured reading to Jf(f).
As in the previous procedure, the result­ing number is equal to the maximum SSB phase noise level in dBc/Hz of either source. Notice that the HP 3582A does not require any of the spectrum analyzer correction factors discussed in Chapter 5. This is due to its automatic
bandwidth normalization feature and
digital Fast Fourier Transform operation.
10.
If the phase noise at other offsets not currently displayed on the HP 3582A is required, repeat steps 6 through 9. Generally, recalibration is not necessary, but quadrature may have to be reset
. Typical HP 8662A/8663A Absolute Phase Noise.
Offset from Carrier (Hz)
Figure 6.5.
Typical HP 8662A/8663A absolute phase noise in DC-FM mode.
Automated SSB Phase Noise
Measurements Using the HP-IB
The phase noise measurement systems shown in Figures 6.1 and 6.3 can be automated. In the example program, the HP 3585A Spectrum Analyzer is substi­tuted for the HP 3582A Spectrum Ana­lyzer used in the manual measurement system. The HP 3585A is well suited to
automated measurements since it can be programmed to make measurements at specific offsets, rather than over a band of frequencies. The addition of an HP 9836 computer to control the spec­trum analyzer and collect and display data via the Hewlett-Packard Interface Bus (HP-IB) make the system fully auto­mated. There are many advantages to automating complex measurements such
in DC FM Mode
The routine is automated, except for cali­brating the beat note and setting quadra­ture.
To calibrate the beat note, set up a beat note on the spectrum analyzer or the oscilloscope and measure its level, as described in the Measurement Procedure Calibration section. The calibration factor as measured on the spectrum analyzer is the rms value and is 3 dB less than peak. Measured on the oscilloscope it is read directly in peak volts. This calibration
factor, in peak volts, is the slope at the zero crossing in volts/radian.
To set quadrature, follow steps 4 and 5 of the measurement procedure section.
Once quadrature is set, the program asks
for the gain of the amplifier, if appli­cable, and then instructs the user to connect the amplifier output to the input of the analyzer. The analyzer settings
( = 500 MHz
^rysr
■■
& &&
. ...
*
-■ „y
23
Page 24
and resolution bandwidth are automati­cally selected. </(f) is computed from the level in dBv, as read from the spectrum analyzer, minus the gain of the ampli­fier, minus the calibration factor.
The phase-noise curve in dBc/Hz versus log frequency is plotted on an HP 2671G Printer. The printing and plotting subroutines may be changed to meet individual documentation requirements.
The number of offsets at which phase noise is measured is determined by the number of steps chosen in the beginning of the program. The measurement range, 20 Hz to 10 kHz, is scaled in logarithmic steps accordingly.
As an example of the power of HP-IB
automation, refer to the phase noise
graph in Figure 6.9. This graph was
obtained from a system similar to that Figure 6.6.
shown in Figure 6.6 using 100 offset points. Automated system for phase noise measurement.
Figure 6.8.
Phase noise measurement software flowchart.
24
Page 25
Si--? -
O
Figure 6.7.
HP 9836 Software for Automatic Phase Noise Measurements.
Figure 6.9
HP 8662A/8663A Residual SSB Phase Noise at F
c
420 MHz
25
Page 26
i ■»V >FVA
iChaptei^?:**--
■ ■*.' ■, -, ■ *■ ■
\ •*>; .
-JSffiS
-,%t;%m
. ,c««B the Iff8662A/8663A atjkficrowave'Fmtfendes^ttf"fli^1ffl3b4^ :
ssb
Af
/!r
:^.
;■
v^v.iir.'B??"fe..-'•'£#£.•• > -v. -
cos27rfmt
peak
27TU)
sin
m
peak
m
Af „
f peak
Af
peak
- 6dB
m
when a signal is frequency multiplied. Modulation theory says that when a signal f± Af is doubled, the frequency deviation is doubled, but the rate of modulation remains the same. Consid­ering phase noise as angular modulation on a carrier, doubling the carrier frequency will yield twice the frequency deviation at the same rate. Substituting for Jf(f) in equation 7.1 yields
2Af
Jf(2f) = 20log
and
«*(2f)
peak
= 6dB
Jf(f)
Therefore, each doubling of the carrier
frequency results in 6 dB higher phase
HP 8662A/8663A Phase Noise Per-
formance at Microwave
Frequencies
The above relationship shows that multi­plying a 1000 MHz signal directly from the HP 8662A/8663A front-panel output 10 times to a frequency of 10 GHz increases the phase noise 20 log 10 or
20 dB. Figure 7.1 is a plot of the resul­tant phase noise of the multiplied signals versus the phase noise of the Hewlett­Packard Model 8672A Microwave Synthesized Signal Generator at the same frequency.
The graph shows that the signal from the HP 8662A/8663A multiplied up to 10 GHz has noise 20 dB lower at offsets
from 100 Hz to 10 kHz than that noise provided by a typical microwave gener­ator. However, generating a low noise
signal by simply multiplying the front­panel output has trade-offs. First, the broadband noise of a multiplied front panel HP 8662A/8663A signal is
somewhat higher than that of typical microwave synthesizers. Second, whenever a signal is externally multi­plied, unwanted spurious responses are also created, and the output level cali­bration is lost. AM modulation perform­ance is severely limited, and the maximum available output power is
significantly reduced. The following section will discuss an alternate multipli­cation scheme, employed in the HP 3048A option 300 Phase Noise Measurement System, which minimizes these disadvantages.
■'
Phase Noi8e^Measurementj^vstein#i?5^-'
Why Use the HP 8662A/8663A at
Microwave Frequencies?
As discussed in Chapter 2, in recent years the importance of phase noise in radar and communications systems has grown significantly. Modern systems such as two-way voice-grade radio, digital communications, and doppler radar have become increasingly depen­dent on low phase-noise signals, both for signal simulation and system testing.
Two-way radios usually operate over frequencies within the range of the HP 8662A, up to 1280 MHz (see Chapter 8), and the HP 8663A which operates up to 2560 MHz satisfies most LO requirements (see Chapter 9). However, many other phase-noise­dependent systems operate at frequen­cies well above the HP 8663A frequency range. For example, airborne doppler radar operates at a frequency around
10 GHz. Low-phase-noise signals are absolutely critical for these systems, both close to the carrier (representing slow­moving objects), and far away from the carrier (echoes from objects moving at
higher velocities). These low-phase-noise microwave signals can be realized by
frequency multiplying the output of the
HP 8662A/8663A.
signal noise. In Chapter 2, Jt(() was defined as the ratio of the single­sideband phase noise power in a 1 Hz
bandwidth, fm hertz away from the
carrier frequency, to the total signal power. This definition of Jf(f) is primarily applied to random noise. To determine the effect of multiplication, a signal with sinusoidal frequency modula­tion is considered first.
f(t) = f0 + Af
0(t) = j27Tf(t)dt
V(t) = Vscos[27rf0t + tf>(t)]
V(t) = Vs cos (2xf0t + ^
For the first order sideband the single­sideband-to-total-carrier-power ratio is
given by:
V
ssb —
V f
For small modulation index,
Af
peak
«1
In addition to signal simulation, the multiplied low-phase-noise output from the HP 8662A/8663A can be used for phase-noise measurements on micro­wave sources and systems. This chapter discusses multiplying the HP 8662A/ 8663A to microwave frequencies and using it as the low noise reference in a
microwave phase-noise measurement system, the HP 3048A option 100/200 and option 300.
The HP 3048A with options 100/200 and 300 is a complete, automated system for phase-noise measurements from 5 MHz to 18 GHz. It consists of an
HP 3048A Phase Noise Measurement System with an HP 8662A/8663A synthesized signal generator (option
100/200), an HP 11729C Carrier Noise
Test Set (option 300), and an HP Series 200 or 300 Desktop Computer to control the system.
Effect of Multiplication on Signal Noise
Basic modulation theory and spectral­density relationships can be used to derive the effect of multiplication on
f
The single-sideband-to-carrier ratio is approximated by:
Af
peak
-11 f
and all other sidebands are negligible
m
Jf(f) -
or in logarithmic form
Jf(f) =
Equation 7.1
For a more complete derivation of of(f), see "Today's Lesson—Learn about Low-Noise Design", Part I and Part II, Microwaves, April and May 1979.
Equation 7.1 is in a convenient form for calculating the increase in phase noise
20log-p
W
6dB
26
Page 27
a*,?
?
D
•'•^w^wv $«?'
-30
1\
V
V
J^*-^
V
1
II
HP
II at
Figure 7.1.
Phase noise comparison of HP 8662A/8663A and HP8672Aat 10 GHz.
Note: If signal generator characteristics
are needed at microwave frequencies, but the phase noise of the HP 8672A is not adequate for the application, there is a simple technique which uses the HP 8662A/8663A as an LO substitute for the VCO in one of the HP 8672A's phase lock loops. This method results in improved phase noise performance over the standard HP 8672A, while main­taining the maximum output level,
output level calibration, amplitude modulation, and spurious performance of the HP 8672A. At the same time, increased frequency resolution and frequency-modulation capability are
provided. See Chapter 11 for the block
diagram and system performance.
Using the HP 8662A/8663A and the HP 11740A for Low-Noise Micro-
wave Signal Generation
X
8662A/8663A*",S
Hz
10
G
10 100
"^ HP 8"79A at 1fl fiHz
^
Offset from Carrier (Hz)
lc = 10GHz
I
10k 100k 1M
of six frequency doublers (Figure 7.2). Theoretically this frequency multiplica­tion would increase the phase noise of
the internal reference by 20 log(640/10) or 36 dB, resulting in a noise character­istic as shown in Figure 7.3. However, to reduce sideband noise, monolithic crystal filters were added in the reference multi­plier chain at 40 and 160 MHz. These filter the noise sidebands at offsets greater than about 4 kHz (6 kHz band­width at 40 MHz) and 10 kHz (18 kHz BW at 160 MHz), to yield a 640 MHz signal with phase noise typically —95 dBc at 10 Hz offset from the carrier, decreasing to a noise floor of greater
than —160 dBc at offsets greater than 20 kHz.
This directly synthesized, low-phase-
noise 640 MHz signal is available from
the rear panel of all HP 8662As and 8663As. The HP 8662A/8663A option
003 provides specified absolute phase
noise for this 640 MHz signal, as shown in Table 7.1. The signal is tapped off the
640 MHz signal from the reference sec­tion, before it is input to any of the phase locked loops. Since the additive noise of the output phase locked loops is not present, it has significantly lower phase noise than the signals available at the front panel of the instrument.
The low-noise rear-panel 640 MHz output from the HP 8662A/8663A is uti­lized by the HP 3048A option 003 to provide a low noise microwave reference signal. This critical multiplication is per­formed by the HP 11729C Carrier Noise Test Set, shown in Figure 7.4, which is option 003 of the HP 3048A System (Figure 7.5 shows how the HP 11729C interfaces with the HP 3048A).
The 640 MHz reference input to the HP 11729C multiplier chain first passes
through a 640 MHz SAW bandpass filter, to reject 10 MHz and 20 MHz reference harmonic spurious sidebands which are caused by the synthesis process in the HP 8662A/8663A, and to reduce noise 1 MHz away from the carrier and beyond. A power amplifier then provides sufficient drive level to a step-recovery-diode multiplier* which
generates a comb of frequencies spaced
640 MHz apart extending up to 18 GHz.
A circulator isolates the diode multiplier from bandpass filter reflections, and the microwave bandpass filter selects the comb line close to the frequency of the device under test. The result is a clean
multiple of the 640 MHz signal, within
1280 MHz of the signal to be tested. This low-noise reference signal downconverts the signal under test to an
Figure 7.1 shows the level of phase­noise performance that can be achieved by multiplying a signal from the front
panel of the HP 8662A/8663A. Though useful for many applications, this does not represent the maximum performance level which can be obtained. Chapter 3 discussed the design of the HP 8662A/ 8663A reference section, a critical subblock where low noise design was emphasized. This carefully designed low noise HP 8662A/8663A reference section can be utilized in a low-phase-noise multiplication scheme for microwave signal generation.
In the reference section of the HP 8662A/8663A, the 10 MHz reference signal is directly multiplied up to a frequency of 640 MHz through the use
Figure 7.2.
Direct synthesized 640 MHz signal.
27
Page 28
:
•'• u V '*/ ,?
IF that
is
measurement technique
detector method. Refer
Note 11729B-1 discussion for measuring phase noise.) noise spectral density low-frequency signal analyzed
less than 1280 MHz.
for a
of the
by the HP
Offset from carrier
to HP
more detailed
phase detector method
of
is
3048A.
is the
this
detected
The
The
-i, > "•!'
(The
basic
phase
Product
phase-
and
detec-
Absolute Phase Noise (dBc)
Option 003
10Hz
100 Hz
1 kHz
10 kHz
100 kHz
1 MHz
Table 7.1.
HP 8662A/8663A Opt. 003 specified absolute phase
noise
at
640 MHz.
tion
and
analysis portion HP 3048A Phase Noise Measurement System which
*For more information frequency multiplication, of
Low
HP RF
Paper, Dieter Scherer, Packard Application Note 983, Comb Generator Simplifies Multiplier Design.
is
shown
Phase Noise Microwave Signals",
and
Microwave Symposium
-60
is the
in
on
see
and
Figure
methods
"Generation
Hewlett-
-104
-121
-145
-157
-157 |
7.5.
of
-„•
-'X/?Si..
ting
.-"•'
the
HP 3047A/11740A
Make Phase-Noise Measurements
on Microwave Sources
Chapter 6 describes a measurement system which uses as
a low
noise reference source
manual
or
automatic
measurements
I
I
at RF
the HP
8662A/8663A
SSB
phase-noise
frequencies.
-84
components discussed phase detector measurements lumped into three catagories: ence section, quadrature circuitry, analysis section. HP 3048A Microwave System fill these catagories:
HP 11729C provide ence,
the circuitry provided The
HP
the
the HP
quadrature
and
baseband analysis
by the HP
3048A comes complete with
in
Chapter
can be
phase-detector/
and the
The
components
8662A/8663A
the low
and 3048A Interface
the
baseband-
noise refer-
phase-detector
to
to
make
The
6 for
refer-
of the
and the
is
box.
vrw
software HP 3048A performance specifications listed
MEASUREMENT TECHNIQUE
The extensive software package that accompanies designed software system and following prompts, measurements
are automatically executed results plotted. three measurement techniques: phase­detector with voltage control, phase­detector without voltage control, frequency-discriminator. be displayed Jf(f), S.(f) Chapter 2. square frequency fluctuations mean square fractional frequency fluctu­ations, respectively, HP 3048A Phase Noise Measurement System Operating Manual.
DATA INTERPRETATION
The measures The system will monitor quadrature alert during a measurement.
The HP3048A also identifies spurs. Spurs
and electrical phenomena. Figure typical the appearing line-related spurs spurs.
to
control
in
Table
for
is
simply loaded into
and by
S,(f), S„(f),
and
<T(T)
S^f) and
HP
304 8A
and
the
user
are
caused
HP
HP
304 8A phase noise graph
8662A/8663A.
in the
the
system. Basic
7.2.
the
system
simple operation.
selecting from menus
The HP
in any of
Sy(f) and
were discussed
is a
displays phase-noise data.
to
out-of-lock conditions
by
graph
and
has
been
and the
3048A provides
The
data
five formats:
cr(r). </(f),
Sy(f),
are
powerful system that
both mechanical
in
the
mean
and the
discussed
The
spurs
are
most likely
microphonic
are
The the
and
can
in the
and
7.6 is a of
Figure
7.3.
Phase noise
28
of
640 MHz reference signal.
Markers
exact frequency particular spur, the relative amplitude change versus offset frequency.
HP
Figure floor viated specifications In addition the offsets optioning other spectrum analyzers
HP 304 8A will measure with with
are
available
3048A
SYSTEM PERFORMANCE
7.6
shows specified system noise
and
spurious. Table
to
HP
of
± 2 dB ± 4 dB
excellent spectral purity,
3048A
is
0.01
Hz to
accuracy accuracy.
to
and
and
accurate within
pinpoint
amplitude
slope lines indicate
7.2
for the HP
100 kHz.
out to 1 MHz
and out to 40 MHz
of a
of
lists abbre-
3048A.
2 dB for
By
the
noise
the
Page 29
Figure 7.5.
HP 3048A Opt. 003 system block diagram.
The HP 3048A also has internal sources to provide complete system calibration.
MEASUREMENTS ON PULSED
SOURCES
The HP 3048A system is also capable of making measurements on pulsed sources. These measurements create their own set of limitations, mainly due to duty cycle. Since the reference is on all
the time, but the DUT pulsed; the phase detector sensitivity decreases as a function of duty cycle. As duty cycles become very low the noise of the measurement system predominates.
In order to make pulsed measurements with the HP 3048A system, an external
phase detector may be necessary at the HP 11729C IF output port. The L port
drive is provided by the front panel of the HP 8662A/8663A. After the phase detector, a low pass filter removes the sum mixing products and the PRF lines. The resulting signal is applied to the HP 3048A Signal Input port. This config­uration provides measurements on pulsed sources with duty cycles down to approximately 20%.
SUMMARY
The HP 8662A/8663A's low phase noise properties can be used to provide state-of-the-art phase noise performance at microwave frequencies. The standard HP 8662A/8663A front panel signal can be multiplied up to microwave, offering close-in phase noise improvements of tens of dB's over other available micro­wave sources. Alternatively, to produce
lower noise performance at microwave
frequencies, a very-low-noise HP 8662A/8663A reference signal can be used in a low noise multiplication scheme for microwave signal genera­tion. This technique can be used to produce signals with absolute noise —71 dBc at a 10 Hz offset, with a noise
floor greater than —135 dBc for a carrier frequency of 10 GHz. The same low-noise reference signal can also be used as the basis for an automated microwave phase noise measurement system such as the HP 3048A Phase Noise Measurement System.
29
Page 30
Specifications describe
intended
to
warranted performance parameters. These
the
provide information useful
instruments' warranted performance. Supplemental characteristics
in
applying are
denoted
the
instrument
as
'typical,' 'nominal,'
by
PHASE DETECTOR PORTS
Frequency
Amplitude
Maximum Signal (dBm) Minimum Signal (dBm)
Offset Frequency Range
Accuracy (measurement
the
System Phase Noise
■> ' 0 01
(
'*_, ' Offset Frequency
System Noise with Input Level Decrease
;
Range:
5 MHz
to
Additional Range with Option 201:
1.6 GHz
(high-frequency inputs)
(low-frequency inputs)
1.2 to
(The
frequency range extended/with a customer-supplied phase detector frequency discriminator.)
0.01
Hz
to
Hz to
40 MHz 2 MHz
0.01 (Assumes addition system, otherwise offset range limited
two
inputs
to
±2
dB
±4
dB
(Does
not
the
for
0.01
Hz to 1 MHz
for 1 MHz
include phase noise
-.-..,'
■-.
Low-Frequency Inputs
Lin +23 415
for
carriers from
for
carriers from 5 MHz
of
40 MHz
of
spectrum analyzer
all
noise
phase detector
offsets
to
40 MHz
and
offsets
Spurious Responses
and
'--
R in
+23
-5
95 MHz
to
and
spurious present
and
system contribution):
spurious signals
from a reference source.)
:
-00
-100
-120
140
160
01 1 10 100 -IK 10k 100k 1M 10M 40M .
and
Spurious Response Increase
L input signal:
1 1
Spurious Responses
i
(Hz)
I
I 1
>+
15
dBm Low
>
+ 7
dBm
(dBc)
Frequency Input
High Frequency Input
giving typical,
18
or
GHz
can
but not
"approximate."
be
or
r
High-Frequency Inputs
Lin
+10
+7
to
to
95 MHz
R in
+10
-0
18 GHz
to the
100
kHz.)
at
"
I
i
are
level increases from and
the
system's maximum noise level
-112
frequencies increases from
to
-102
-170
dBc
at all
at
to
>10 kHz
-160dBc/Hz.
NOISE INPUT PORT
(For
use
with external phase detector
or
frequency
discriminator)
Frequency:
0.01
Hz to
40 MHz Amplitude: 1 Volt peak maximum Typical Input Impedance: Accuracy: External phase detector
measurements calibrated with
±2
dB
for
0.01
±4
dB
System Noise
Hz to
for 1 MHz
and
100
120
140
0.01
01 1 10 100 1k 10k 100k 1M 10M 40M
50Q;
return loss >9.5 dB (<2:1 SWR)
or
frequency discriminator
± 1
1 MHz
to
40 MHz
dB
offsets
offsets
Spurious Responses
1
j
|
j
R
' dR 1
pu
IOUS
esponses
1 m
Offset Frequency
(Hz)
accurate signals.
TUNING VOLTAGE OUTPUT
Voltage Range:
Current:
Output Impedance:
± 20
±10
mA
volts
maximum
50Q
nominal
SOURCE OUTPUT TYPICAL PERFORMANCE
10 MHz Source
Amplitude:+15 Tuning: ± 100
10 MHz Source
Amplitude: Tuning:
350-500
Amplitude:+17 dBm.
400 MHz
Amplitude: —5 Tuning: Fixed Frequency
Typical Noise
± 1
MHz
A
dBm
Hz
B
+2 dBm
kHz
dBm
and
Spur Levels
TYPICRL NOISE
OF HP
ue-tefi BUILT-IN SOURCES
offset frequencies
offset
I
■
To determine system noise the dB degradation graph
and add to if a +15 dBm and a +5 dBm +
10
dB. Therefore,
Table
7.2.
HP 3048A phase noise measurement specifications.
30
Low Frequency Input Amplitude Range
ill'
H,gh Frequency Input
Amplitude Range
at the
the
signal
is
signal
(he
„, .y> ;• ■
and
spurious response levels, find
signal input level from
curves
of
applied
to
to
the R Input,
specified maximum spurious signal
the
the Low
-
+20
upper graph.
Frequency L Input
the
degradation
+25
the
lower
For
is
example,
Page 31
The HP 3048A can be ordered with any of several optional signal generators as a reference source for phase noise meas­urements. The following specifications address system opera-
tion with these signal generators. The data that follows is in addition to that given previously under the heading of HP 3048A System Specifications. Refer to the data sheet for each signal generator for more complete information on each model.
OPTIONS 001 OR 002: ADDING THE HP 8662A
OR 8663A SIGNAL GENERATOR
The following data applies only if either the HP 8662A Opt. 003 or 8663A Opt. 003 is used as the reference source to demodulate the test signal.
Frequency-
Range: 100 kHz to 1280 MHz (to 2560 MHz with HP 8663A). Resolution: 0.1 Hz, 0.2 Hz: 640 to 1280 MHz, 0.4 Hz above
1280 MHz.
Accuracy and Stability (internal 10 MHz quartz oscillator):
Aging rate <5 X 10"lo/day after 10-day warm-up (typically 24 hrs in normal operating environment).
EFC:
Provides a drift tracking range "of ±10"8 with no degrada-
tion of phase noise or spurious.
Spectral Purity
2
Absolute Phase Noise
Offset from Carrier (Hz)
1
10
100
1k
0.1 to 120 MHz
120 to 160 MHz
160to 320 MHz
320
10
640 MHz
640 to 1280 MHz
1280 to 2560 MHz*
* HP 8663A Option 003 only.
Typ.
Spoc.
Typ.
Spec.
Typ.
Spec.
Typ.
Spec.
Typ.
Spec.
Typ.
Spec.
-78
-68
-76
-66
-70
-60
-64
-54
-58
-48
-52
-42
-108
-98
-106
-96
-100
-90
-94
-84
-88
-78
-82
-72
-126
-116
-125
-115
-119
-109
-114
-103
-108
-97
-102
-92
-132
-126
-135
-129
-130
-124
-125
-118
-119
-112
-113
-106
10k
-138
-132
-148
-142
-142
-136
-136
-131
-130
-124
-124
-118
100k
-139
-133
-148
-142
-144
-138
-136
-132
-130
-126
-124
-120
1M
-145
-150
-144
-145
-140
-134
OPTIONS 003 OR 004: ADDING THE HP 11729C OR 11729C OPT 130 CARRIER NOISE TEST SET
The following data is applicable to using the HP 11729C to downconvert the test signal to an IF of between 5 MHz and 1280 MHz for subsequent demodulation using the Low Frequency phase detector of the HP 3048A system. The HP 8662A Opt. 003 or 8663A Opt. 003 Signal Generators
provide a 640 MHz reference signal for this downcoversion process. These signal generators also provide a signal of between 5 MHz to 1280 MHz to demodulate the downconverted IF noise. The specifications that follow assume this measurement set-up is used.
* Measurements <5 MHz require external phase detector.
2
Specified only with FM off.
Input Requirements
Frequency
Measurement Frequency Range: 5 MHz to 18 GHz in 8
bands,
excluding ±5 MHz around band center frequencies.
Band Center Frequencies: 1.92 GHz, 4.48 GHz, 7.04 GHz,
9.60 GHz, 12.16 GHz, 14.72 GHz, 17.28 GHz.
Amplitude
For carrier frequencies <1.28 GHz: -5 dBm minimum to
+23 dBm maximum.
For carrier frequencies >1.28 GHz: +7 dBm minimum to +20 dBm maximum.
Measurement Specifications
Offset Frequency Range
For carriers between 5 and 95 MHz from band centers:
1
0.01 Hz to 2 MHz.
For carriers >95 MHz from band center: 0.01 Hz to
40 MHz. (Assumes addition of 40 MHz spectrum analyzer to the system, otherwise offset frequency range limited to
100 kHz.)
System Noise Floor
3
Absolute System Noise Floor (dBc/Hz), when used with the HP 11729C and HP 8662A Option 003 or HP 8663A Option 003 as the reference source, phase locking via the signal generator's EFC.
Offset from Carrier (Hz)
1
10 100 | 1k 10k 100k
0.1 to 1280 MHz
1280 lo 3200 MHz
3.2
to
5.76 GHz
5.76 to
8.32 GHz
8.32 to
10.88 GHz
10.88 to
13.44 GHz
13.44 to
16.0 GHz
16.0 to
18.0 GHz
See HP3048A Option 001 or 002,
Absolute Phase Noise table on page 13.
Typ.
-52
-82
-102
-113
Spec.
-42
Typ.
Spec.
Typ.
Spec.
Typ.
Spec.
Typ.
Spec.
Typ.
Spec.
Typ.
Spec.
-47
-37
-43
-33
-40
-30
-38
-28
-37
-27
-35
-25
-72
-77
-67
-73
-63
-70
-60
-68
-58
-67
-57
-65
-55
-92
-97
-87
-93
-83
-90
-80
-88
-78
-87
-77
-85
-75
-106
-109
-104
-105
-100
-102
-97
-100
-95
-99
-94
-97
-92
-124
-118
-127
-123
-125
-121
-123
-119
-122
-118
-121
-116
-119
-115
System Noise of HP 3048A Options 001 or 002, and 003 or 004 at 10 GHz (Phase locking via EFC)
>ical
Ty
OftMt Froquoncy (Hz)
-124
-120
-130
-126
-129
-125
-129
-125
-128
-125
-127
-124
-127
-123
1M
-134
-138
-135
-134
-132
-131
-129
Table 7.2.
HP 3048A phase noise measurement specifications.
Page 32
i
-'d^b*
'
-'flHfenSaBS-*-
i'SKS
i '-"■"Haas
p^wr .1'-:
BBpr'A',
$fcS*:
k
*** -
*
,
*I-J-"O-;
^
!
,i
•
->V«A,
«* -
",r
System Spurious
System spurious signals 004 arise baseband signal processing, than other spurious signals are translated downconversion process gives rise signals whose frequency relation between center frequency. does
AM Noise Detection
The using either built-in 004
Table
7.2.
HP 3048A phase noise measurement specifications.
in
three ways. First, from
0.2 Hz
from
to the
not
affect
the
HP
3048A
can be
an
external
to the HP
of the HP
3048A).
in the HP
the
the
The
typical measurement
11729C Option
<-104 dBc for
carrier. Second,
on the HP
noise spectrum output. Third,
and
test signal frequency
presence
used
AM
AM
8662A
level
of
for AM
detector
130
measurements with
3048A Options
the
detection
any
or
to
system spurious
are
determined
and the
system spurious signals
of
noise measurements
or the AM
(ordered
003 or
and
offsets greater
line-related
8663A outputs
random noise.
or
the
by the
band
detector
as
Option
the
HP 3047A Noise Floor
4 Averages Carrier Freq =1.000E+09
HP 11729C Option tivity
of -165
130 can be
dBc/Hz
made with a typical sensi-
at a 1 MHz
offset.
COMPATIBLE SPECTRUM ANALYZERS
The
HP
3048A
is
spectrum analyzers 40 MHz. Those spectrum analyzers include (orderable 8567A, 8568A, 71100A, each
of
these spectrum analyzers specifications apply fully when these compatible spectrum analyzers
analyzer
is
specifications.
designed
as
Option
are
included
operating properly
& HP
to use
to
extend
101 to the HP
and
in the
8662A/8663A Phase Noise
several Hewlett-Packard
the
offset range from 100
71200A. Automatic control
is
system
and
meets
Hz [hp] may 20 1985
3048A),
provided.
as
long
its
the HP
the HP
as the
performance
3585A
8566A,
The HP
spectrum
@ 1 GHz
15:45/16:16
kHz to
of
3048A
32
Figure
Typical
7.6.
HP
3048A Specified System Noise Floor.
100
1K 10K 100K 1M 10M
Jt(i) [dBc/Hz]
vs f[Hz]
Page 33
Chapter 8 ' ; X-.;--J;\
- *-'-•. -■
^i-<^-^'HQ^-:
'v -->
Voice Grade Receiver Testing with the OT§i62A2^663A 7''?\>Jf
Programmable, low-phase-noise synthe­sized signal generators are used exten­sively in receiver testing. The design of the HP 8662A/8663A yields a noise spectrum at typical receiver channel spacings that lends itself readily to
receiver test.
The spectral purity of the HP 8662A/
8663A is most commonly measured in terms of single-sideband phase noise, but it can also be expressed in terms of residual FM and spurious. Residual FM is the total noise measured in some post­detection bandwidth. Spurious signals are those unwanted signals generated as a result of the various nonlinear opera­tions such as mixing that are part of the
synthesis process. These measures of spectral purity are important in defining
the performance requirements necessary
for a signal generator to make receiver measurements.
There are many receiver tests and many test standards for these measurements used around the world. These include the Institute of Electrical and Electronic Engineers (IEEE) and the Electronic Industries Association (EIA) standards in the United States and the Conference of
European Postal and Telecommunica-
tions Administration (CEPT), British Post Office (BPO), and International Electro­technical Commission (IEC) standards in Europe. Though the details of these tests vary considerably, the receiver parame­ters that must be tested are basically the same.
This chapter describes the two basic categories of receiver testing, the signal generator performance required by them, and how the HP 8662A/8663A meets these test requirements.
Receiver Test Basics: In-Channel and Out-of-Channel Testing
Receiver tests can be roughly subdivided into two basic types: in-channel and out­of-channel. In-channel testing is exactly what the name implies—evaluating the performance of the receiver when the test signal is applied at the exact fre­quency to which the receiver is tuned. These tests determine how well the receiver responds to the signal that it is
intended to receive. An example of this
type of test is sensitivity—the smallest
level of RF signal applied at the input of
the receiver that will give intelligible
information at the output. The definition
of 'intelligible' information varies with the test standard being used.
Many receiver tests use a calculation called 'SINAD' as a measure of the received signal quality. SINAD is equal to the ratio of (signal plus noise plus dis­tortion) to (noise plus distortion) at the same output level; that is,
SINAD (dB) = 20 log
The measuring instrument at the audio output of the receiver is generally some type of distortion analyzer. For a SINAD measurement, the analyzer first acts as a broadband voltmeter, measuring the total output of the receiver. Then a filter notches out the audio modulation tone, and the resultant noise plus distortion is measured. The ratio of the two measure­ments is SINAD, and is commonly expressed in dB. The CEPT standard
defines sensitivity as that RF input level which produces 20 dB SINAD weighted per CCITT requirements.
Almost all areas of signal generator per­formance are important for in-channel testing, with the level of performance needed dependent on the receiver being tested. All three primary performance areas—frequency, output level, and modulation—must be considered. The HP 8662A/8663A provides high per­formance in every specification including frequency resolution, accuracy, and sta­bility; output level resolution and accu­racy; and AM or FM with either ac or dc coupled input.
Certain measures of spectral purity can be important for in-channel testing. The low close-in phase noise of the HP 8662A/8663A translates into extremely low residual FM. Typical residual FM in a 300 Hz to 3 kHz post-
Figure 8.1.
Receiver adjacent channel selectivity and receiver spurious attenuation
measurement.
S + N + D
1(
N + D
Generator 1 In-Channel
Generator 2
Out-of-Channel
HP 8662A/8663A
detection bandwidth is a few tenths of a hertz. Residual FM can be an important specification for in-channel tests such as receiver residual hum and noise, where the residual FM results in a small amount of detected noise, falsely increas­ing the measured signal noise.
Out-of-channel testing determines how well the receiver rejects those signals that it is not intended to receive. Here the test signal is applied not at the fre­quency that the receiver is tuned to but at some other frequency. An example of this kind of test is adjacent channel selectivity, a measure of the ability of the receiver to select the desired in-channel signal while rejecting a signal that is present one channel spacing away.
Out-of-channel testing is more demand­ing on the test signal generator than in-channel testing. The primary perform-
ance requirements needed from the signal generator to make these tests are low spurious and low phase noise at sets from the carrier equal to the channel spacings of the receiver. An examination of two of these out-of-channel tests shows why.
off-
Using the HP 8662A/8663A for Adjacent Channel Receiver Tests
The adjacent channel selectivity test defined above is one of the most common out-of-channel tests. Two generators are used in this test, one in-channel to simulate the desired signal and the other out-of-channel to simulate an unwanted signal. The following example procedure follows the EIA standard for FM receivers—specification RS-204-B.
Generator #1 produces the in-channel
signal, generator #2 the out-of-channel
signal (see Figure 8.1). With generator #2
HP8903A
Audio Analyzer
/ W ■"
ee
33
Page 34
turned off, generator #1 is set in-channel and modulated with a 1 kHz tone at 60%
of the maximum rated deviation of the receiver. The level of generator #1 is set to the sensitivity of the receiver (12 dB SINAD for the EIA-FM standard).
Again, the measurement instrument at the audio output of the receiver is gener­ally some type of distortion analyzer. Figure 8.1 shows a Hewlett-Packard Model 8903B Audio Analyzer, which automatically makes the two measure­ments necessary for a SINAD ratio, then internally calculates and displays SINAD directly in dB. The HP 8903B is fully programmable, allowing the entire test to be automated.
With signal generator #1 set to the sen­sitivity level of the receiver plus 3 dB, generator #2 is tuned to the adjacent channel of the receiver. It too is modu­lated at 60% of the receiver's maximum deviation, but with a 400 Hz tone.
The level of generator #2 is then increased until the measured SINAD ratio of the receiver drops to 12 dB as defined in the EIA test standard. This
drop in signal quality is a result of inter­ference by the adjacent-channel signal. The difference between the two output settings on the generators is then defined as the receiver's selectivity. The higher the receiver's selectivity, the greater the level of out-of-channel inter­ference it is able to reject.
Phase noise and AM noise are probably the most important speicfications which
determine whether the signal generator can make an adjacent channel selectivity measurement. Figure 8.2 shows the transfer characteristic of a receiver's IF filter; the selectivity test is designed to show how well the IF filters in the
receiver reject signals outside the normal pass-band. If a generator's phase noise or AM noise (even for FM receivers) is inadequate, as the level of the channel generator is increased, the high
level of phase noise at the channel spac­ing would appear within the bandwidth of the selected channel and would con­tribute to the distortion being measured. As a result, the test would not be mea­suring the receiver's ability to reject a signal one channel away, but rather how much noise the signal generator itself had at a channel spacing offset from the
out-of-
Figure 8.2.
Signal generator phase noise in adjacent channel test.
Figure 8.2 shows the noise spectrum of two signals used as the out-of-channel signal. The solid line is an example of a signal generator with inadequate noise performance to make an out-of-channel test; its noise power at a channel offset appears within the bandwidth of the selected channel at a higher level than
the desired signal. The dashed line rep­resents a signal with phase noise at a channel spacing low enough to not add significantly to the measured noise within the bandwidth of the selected channel.
To make a valid measurement of the
receiver the phase noise performance of the adjacent channel signal generator must be determined. The conversion from the selectivity specification on the receiver to the needed signal generator performance can be easily calculated as shown below.
*TW
Receiver adjacent, channel^ii^S'
specification ,: 4*f ■.,
■:
-<
J.
■J****'
.._>?£;
+
Conversion of the total noise inla
1 Hz BW'specified"on the signal-^
generator;to the noise BW.'of.the^*
receiver
Signal generator absolute noise,: specification at 1 channel offset, from carrier.'
L
\~?
?/.
''-,
'•>»'"--ir ■tfrt
Measurement margin'
■'•-"•
',:. - .*■ ••' .-•,
'<•
'■■"•^
■
?**lf'
34
Page 35
The first factor is the receiver's adjacent-channel-rejection specifica­tion. In the El A standard, the mini­mum standard is 70 dB. The second factor is a conversion of the noise of
the signal generator, generally speci­fied in a 1 Hz bandwidth, to the equivalent noise in the bandwidth of the receiver under test. For a receiver with a 14 kHz IF band­width, this conversion is
dB = lOlog^1!^ =10(4.2) =
42 dB
The third factor, measurement margin, is the most arbitrary factor. In the adjacent-channel test, the ana-
lyzer measures the noise contributions from two sources: any noise generated by the receiver as a result of the inter­ference of the adjacent channel signal (desired measurement), and the phase noise of the signal generator that falls in-channel (undesired). If, for exam­ple,
these noise levels are equal, the distortion analyzer will measure noise 3 dB higher than the actual noise gen­erated by the receiver. Measurement margin is added to the phase noise
requirement on the out-of-channel generator to ensure that its noise con­tribution is much less than the noise generated by the receiver. Requiring the phase noise of the signal genera­tor to be lower than the selectivity of
the receiver by the amount of the measurement margin yields more repeatable measurements. Experience has shown that 6 to 10 dB measure­ment margin is sufficient.
These three factors add up to the actual phase noise specification required for the signal generator. For the EIA standard, a 14 kHz BW receiver with an adjacent channel selectivity of 70 dB for channel spac­ings of 20 kHz requires a signal gener­ator with specified phase noise of 70 + 42 + 10 = 122 dB below the carrier
at a 20 kHz offset from the carrier. It should be noted that this phase noise requirement is for the total or abso­lute noise on the generator (including AM noise), not the residual noise. For most synthesizers, the absolute noise will be equal to the residual noise at offsets from the carrier equal to chan­nel spacings (20 kHz, for example),
but it should be checked for each syn-
thesizer. The difference between abso-
lute and residual noise becomes more
pronounced as channel spacings nar­row. For a more thorough discussion of absolute versus residual noise, see Chapter 2.
Many high-quality receivers specify a selectivity of greater than the 70 dB
for example, requiring even lower
phase noise for these out-of-channel
applications than the —122 dBc com­puted above. It is for these high qual­ity receiver test applications that the HP 8662A/8663A makes major con­tributions. With specified SSB phase noise at a 10 kHz offset from a 500 MHz carrier of -132 dBc (typi­cally -136 dBc), the HP 8662A/ 8663A has low enough phase noise to automatically make most stringent measurements. This means both in-channel and out-of-channel meas-
Figure 8.3.
Signal generator spurious in adjacent channel test.
urements can be made with the HP 8662A/8663A in a programmable system. For more information on Hewlett-Packard programmable sys­tems for making receiver measure­ments, see HP Technical Data for the HP 8953A Semi-Automatic Trans­ceiver Test Set and the HP 8955A RF Test System.
Not only can the HP 8662A/8663A automatically make these
out-of­channel measurements on receivers with channel spacings of 20 to 50 kHz, but it is also designed for outstanding performance on receivers with narrower channel spacings. As
the frequency spectrum becomes more congested, channel spacings will be narrowed, as exemplified by the
12.5 kHz channel spacings now employed in Europe. For many RF signal generators, the phase noise rises very quickly for offsets from the carrier less than 20 kHz. However, the design of the HP 8662A/8663A yields a phase noise spectrum that remains fairly flat in to about a 7 kHz offset from the carrier. Thus, as chan­nel spacings become closer (5.0 kHz channel spacings are already pro-
posed),
the phase noise of the HP 8662A/8663A will still allow automatic out-of-channel receiver testing.
Spurious performance is also an important criterion for the adjacent­channel-selectivity test. If a spurious output from the signal generator
occurs at an offset from the carrier equal to the receiver channel spacing, the spurious will fall into the receiver IF passband, as shown in Figure 8.3. This will have the effect of reducing the receiver's measured adjacent­channel rejection. To prevent this, non-harmonic spurious generated in the signal generator should be attenu­ated at least below the receiver's
adjacent-channel rejection. The
HP 8662A/8663A specifies non­harmonically related spurious to be greater than 90 dB below the carrier in the primary band of 320 to 640 MHz.
Page 36
Using the HP 8662A/8663A for
Spurious Attenuation Testing
A second common out-of-channel test is the spurious attenuation test, a measure of the receiver's ability to discriminate between a desired and an undesired signal. Basically a figure of merit for the input RF filters of the receiver, the test checks if the receiver responds to RF image frequencies, incoming signals at the IF that would feed directly into the audio section, or
any other incoming signals that would generate spurious responses within the receiver.
This test, as defined by the EIA (see Figure 8.1), uses two signal genera­tors.
Generator #1 is tuned to the nominal frequency of the receiver and set to the receiver sensitivity level plus 3 dB. Generator #2 is tuned to
ous responses of the receiver from
spurious outputs of the generator. As
shown in Figure 8.4, if a spurious output from the signal generator falls into the receiver IF pass-band, it will have the same effect as a spurious response in the receiver fore,
spurs generated in the signal generator should be attenuated at least below the level of the receiver's own spurious attenuation. The low spurious output of the HP 8662A/ 8663A minimizes the possibility of causing what would appear to be spurious response of the receiver.
Broadband noise floor is a second aspect of spectral purity that is impor­tant for this test. Figure 8.5 shows a large out-of-channel signal "punching through" the IF filter (that is, at a level high enough to exceed the IF
itself.
There-
rejection), thereby introducing a spur­ious response in the receiver seen in the IF passband. It is this spurious response that the spurious attenuation test is designed to measure. However, if the signal generator has a high broadband noise floor, the spurious response of the receiver will be masked by the noise of the generator. The phase noise of a signal generator is generally specified in a 1 Hz band­width. With a 14 kHz receiver band-
width, the noise seen by the receiver is 10 log (14 kHz/1 Hz) or 42 dB higher. If the receiver has very good spurious attenuation, the generator must have a very low broadband noise floor. If not, as the RF level of the generator is increased, that part of the generator's noise floor that falls within the tuned bandwidth of the
receiver will actually be seen before spurious generated in the receiver, causing the output to always be noisy (Figure 8.5).
The HP 8662A/8663A specifies a broad­band noise floor of —145 dBc per Hz (-148 dBc/Hz typical) for f. between 120 and 640 MHz. This noise in a
14 kHz receiver bandwidth will be 42 dB higher, or —108 dB below the carrier, which is sufficient performance for most high quality receivers specifying 90 or
100 dB spurious attenuation.
o
Figure 8.4.
Signal generator spurious in spurious attenuation test.
the adjacent channel frequency of the receiver and set to a very high level (90 dB nV for example). Signal genera­tor #2 is then tuned over the fre­quency range of the receiver, as well as the IF and image frequencies. If a response is observed the output level of generator #2 is varied until the measured SINAD ratio of the receiver
is 12 dB, as defined in the EIA test standard. The difference in output levels between the two signal genera­tors is the Spurious Response Attenuation.
The spurious output of the signal gen­erator is critical for this test because
the analyzer cannot distinguish spuri-
36
Combining outstanding RF specifica­tions, ease of programming, the HP 8662A/ 8663A provides all the performance necessary to automate the whole range of receiver tests, both in-channel and out-of-channel.
Figure 8.5.
Broadband noise floor in spurious attenuation test.
excellent spectral purity, and
Page 37
Chapter 9
HP 8662A/8663A as an External LO with the HP 8901A/B Modulation Analyzer and HP 8902A Measuring Receiver
o
200
1
"A
200 1 400
Frequency (MHz)
Figure 9.2.
HP 8901 A/B and HP 8902A typical residual FM with no filtering.
I i _.i
H
= 8901A/B
HP 8902A —
anc
INT
_ LO
;
;
-HP 8
EXT
LO |
4
600
r-
800 1000
!M
Figure 9.3.
HP 8901A/B and HP 8902A typical residual FM with 15 kHz LPF.
Frequency (MHz)
"" 8901 A/I
HP 8662A/8663A |
HP 89
IN1
IO
LO
3
and I
D2A "
* I
•
(O
Figure 9.1.
HP 8662A/8663A as external local oscillator for HP8901A/BorHP8902A.
The HP 8662A/8663A can be used as a low noise substitute local oscillator (LO). In this application, it can significantly improve the stability and performance of other instruments and measurement systems. In particular, the HP 8662A/ 8663A can be used with the Hewlett­Packard 8901A/B Modulation Analyzer and HP 8902A Measurement Receiver to improve residual FM.
The HP 8901 A/B and HP 8902A are cal­ibrated receivers that measure modula­tion (AM, FM, </>M), frequency, and power automatically for input frequen­cies from 150 kHz to 1300 MHz. The HP 8901A/B and HP 8902A feature low noise local oscillators; therefore, low residual FM is one of the key contribu­tions.
However, for some applications— measuring hum and noise on FM mobile transmitters, for example—even lower noise performance may be desired. Option 003 allows the HP 8901A/B and the HP 8902A to accept an external local oscillator signal for improved stability and noise performance.
Measured Performance
Figure 9.1 shows how to connect the HP 8662A/8663A as the external LO.
Figures 9.2 and 9.3 show typical HP 8901 A/B and HP 8902A residual FM performance using first the internal receiver LO, and then the HP 8662A/
8663A as the external LO. The noise when the HP 8662A/8663A is used is as much as an order of magnitude lower than when the internal local oscillator is used.
Figure 9.2 shows typical receiver residual FM performance without any internal fil­tering. Notice that above 640 MHz the HP 8662A/8663A improves the noise by
greater than a factor of 4, reducing the residual FM to <40 Hz. Using the receiv­ers internal 15 kHz low-pass filter (Figure 9.3) with the HP 8662A/8663A as an external LO the typical residual noise is less than 3 Hz across the entire frequency range, as compared to <30 Hz with the internal LO.
Notice the effect of frequency on the residual FM of the receiver. The HP 8901A/B and HP 8902A's internal LO operates from 320 to 650 MHz. All
other frequency ranges are obtained by dividing or multiplying this base band. Therefore the residual noise for fc > 650 MHz is approximately twice that for
320 MHz < fc < 650 MHz. (For a discus-
sion of the effect of multiplication or
division on the noise of a signal, see Chapter 7, "Using the HP 8662A/8663A at Microwave Frequencies with the HP 3048A Phase Noise Measurement System".)
The same effect occurs when the HP 8662A/8663A is used as the external LO for analogous reasons. The HP 8662A/ 8663A's main band is 320 to 640 MHz. Frequencies from 640 to
1280 MHz are obtained by doubling; as a result, the noise in this doubled band
is approximately twice that of the base
band. Frequencies from 160 to 320 MHz are in the divide-by-2 band; 120 to 160 MHz is the divide-by-4 band. The noise in these bands is therefore one-
half and one-fourth that of the main band. Frequencies from 0.01 to 120 MHz are obtained by heterodyning the funda­mental band, yielding noise performance similar to the noise of the 320 to 640 MHz range.
37
Page 38
Measurement Considerations and Procedure
When the HP 8662A/8663A is used as an external LO for the receivers there are several considerations to take into account. Using an external LO requires that the internal LO be essentially disabled, so that it does not wander and
introduce spurious signals into the mea-
surement. This can be accomplished by manually tuning the HP 8901A/B or HP 8902A's LO to a known frequency. Tuning it to the high end is acceptable
except when the application is at the upper frequency limit of the receiver. To fix the internal LO at the high end, key in
In frequency mode, the receiver mea­sures input frequency automatically by first counting the internal local oscillator and then the intermediate frequency (IF). The input frequency F^ is then calcu­lated from F^ = FL0 (receiver) — FIF. When the HP 8662A/8663A is used as an external LO, the receiver's internal LO is manually fixed at 1300 MHz; con­sequently, the standard frequency mea­surement is invalid. The receiver can still, however, indirectly count the incoming frequency. Keying
into the receiver keyboard sets up the HP 8901A and keying
sets up the HP 8901B and HP 8902A to measure the signal frequency being amplified in the IF (F[F). Then the input frequency can be externally calculated from
F,., = F,
8662A/8663A
- F„
that F
8662A/8663A > FIN'
Set the
HP 8662A/8663A to FIN + 455 kHz for input frequencies from 2 to 10 MHz. For frequencies > 10 MHz, the HP 8662A/8663A should be set to F
IN
+ 1.5 MHz. For increased sensitivity the 455 kHz IF may also be selected for input frequencies above 10 MHz, but modulation rates and FM devia­tions are restricted.
Since the receiver cannot count the input
signal unless the IF is in the proper
range, the input frequency must be
known to within the IF bandwidth in
order to set the HP 8662A/8663A to the
proper LO frequency. For most transmit-
ter measurements, this is not a problem,
since the BW is approximately ± 1 MHz
for the 1.5 MHz IF, and ±100 kHz for
the 455 kHz IF. Once the difference
between the input signal and the
HP 8662A/8663A LO frequency is
within the IF bandwidth, the receiver
can be used to count the incoming fre-
quency with increased resolution. Then
the HP 8662A/8663A can be offset by
exactly the IF center frequency for opti-
mal performance.
A convenient way to offset the
HP 8662A/8663A by the proper IF fre-
quency is to use the HP 8662A/8663A
Special Function 11, "+ Frequency
Off­set". Special Function 11 makes the actual HP 8662A/8663A output fre­quency equal to the sum of the fre­quency shown on the display and the entered offset. Then only the desired signal frequency need be entered into the HP 8662A/8663A, and the necessary frequency offset will be obtained trans­parent to the operator. For example, if the 1.5 MHz IF is desired, key into the HP 8662A/8663A:
For measurements on the HP 8901A/B or HP 8902A key in the frequency to be applied to the receiver into the HP 8662A/8663A keyboard. The IF offset will be set without any external calculations on the part of the user.
The HP 8662A/8663A can be used as an external LO to improve receiver noise performance. For more information on the HP 8901A/B and HP 8902A, see HP 8901A Technical Data Sheet and HP Application Note
286-1,
Applications and Operation of the HP 8901A Modula­tion Analyzer, HP 8901B Technical Data Sheet, and HP 8902A Technical Data Sheet.
The receivers operate with two IF frequencies—1.5 MHz and 455 kHz. The HP 8662A/8663A must be manu­ally set to the proper offset frequency to produce one of these intermediate frequencies in the receiver. In normal operation, it is recommended that the HP 8662A/8663A always be set such
38
Page 39
Using an HP8662A/8663Atwith the HP8505A RF Network Analyzer
i.
&
Network analyzers measure device trans-
mission and reflection characteristics in
terms of magnitude and phase. A key component of a network analyzer is the signal source. When devices are charac­terized as a function of frequency, partic­ularly over a broad frequency range, sweep oscillators are commonly used as the signal source. For measurements on narrowband devices, or devices whose magnitude and/or phase characteristics change rapidly with frequency, signal generators or synthesizers are preferred
because of improved residual FM and frequency resolution.
The Hewlett-Packard Model 8505A RF Network Analyzer Option 005 allows the HP 8505A to be phase-locked to a . synthesizer, thus improving frequency accuracy and stability. The low phase noise performance of the HP 8662A/ 8663A makes them an excellent choice for use as the HP 8505A source. When used with an HP 8662A/8663A in the
phase-lock mode, the HP 8505A pro­vides crisp CRT displays and high reso­lution digital readouts of transmission magnitude and delay over swept fre­quency widths ranging from only a few hertz to 1 megahertz. In addition to transmission magnitude and delay meas­urements, the HP 8505A can provide calibrated displays of return loss, reflec­tion coefficient, phase, and phase devia­tion over its 500 kHz to 1.3 GHz fre­quency range. The HP 8662A/8663A
provide 0.1 or 0.2 hertz center frequency resolution.
Measurement
The HP 8662A/8663A can be configured with the HP 85 05A Option 005 in one of
Setup
two ways, depending on the desired measurement. Figure 10.1 shows how to set up the HP 8662A/8663A with the HP 85 05A Option 005 for making trans-
mission magnitude and delay measure-
ments.
The system can also be config-
ured for return loss and reflection coefficient measurements. For detailed instructions on these setups refer to the Operating and Service Manual for the HP 8505A Network Analyzer Option 005 Phase-Lock, Option Supplement Chapter F, Supplement Part Number 08505-90070.
In either setup, the HP 8505A generates a maximum ramp voltage of ±1.3V (the
±AF output of the HP 8505A) used to externally frequency modulate the HP 8662A/8663A and provide a real­time,
stable, calibrated swept display on the HP 8505A. Whenever an external source is used with the HP 8505A
Option 005, it is necessary to calibrate the modulation index of the phase­locked system in order to obtain an accurate measurement of group delay and to allow easy and exact settings of sweep width. This is essentially a cali­bration of the external source frequency deviation.
The external frequency modulation of
the HP 8662A/8663A simplifies this cali-
bration. The external modulation input
of the HP 8662A/8663A requires a IV
peak signal, the ±AF output of the
HP 8505A is easily adjusted to this level.
Calibration of the system is accom-
plished with the two front panel annun-
ciators of the HP 8662A/8663A which
indicate when the IV peak signal is
within ±2%. Simply key in the desired
frequency deviation on the HP 8662A/
8663A (which is the desired sweep
width on the HP 8505A) and adjust the
±AF output of the HP 8505A until the "HI-LO" of the HP 8662A/8663A remain extin­guished. The deviation and thus the dis­play is then calibrated and accurate to the specification of the HP 8662A/ 8663A. For standard operation of the HP 8505A, these deviations will be
±1.3 kHz (13 MHz range), ±13 kHz (130 MHz range) and ±130 kHz (1300 MHz range). For additional flexi­bility in range and resolution, the HP 8662A/8663A can be set to produce other peak deviations, where the maxi-
mum range and resolution are computed by the formulas below. The frequency deviation will retain its specified accu­racy as long as the required IV peak signal is applied.
The HP 8662A/8663A provide for both ac and dc coupling of the external FM
input. For very narrowband devices, the
DC-FM mode will normally be selected,
as slow sweep speeds on the HP 8505A are required. Center frequency stability of the HP 8662A/8663A is somewhat degraded in the DC-FM mode (see HP 8662A/8663A Technical Data Sheets for specifications).
For other applications, ac mode, which allows rates down to 20 Hz is acceptable, yielding higher frequency stability (±5 X 10"10/day stability).
annunciators on the front panel
Maximum Range =
1.04 x 10
5
MS
(±AF)
Maximum Resolution =
Figure 10.1.
HP 8505A phase-lock test setup with, HP 8662A/8663A.
130
MS/DIV
(±AF)
Typical Characteristics
The HP 8662A/8663A improves the performance of the HP 8505A Net­work Analyzer. The following sec­tions describe typical performance of a phase-locked system using the HP 8662A/8663A with the HP 8505A Option 005.
Operating
39
Page 40
>
4^
'TT'*«r
I ^A'klC-i
m?.
J^^M^/M:^
Frequency Characteristics Range and Resolution
HP 8505 Frequency Range
CW Resolution (set HP 8662A/8663A)
±AF Resolution (set
HP 8505A)
Table 10.1
HP 8505A frequency characteristics when locked to
HP
8662A/8663A.
NOTE:
The
by maximum
maximum ±AF
FM allowed on the HP 8662A/8663A at the frequency frequencies HP 8662A/8663A tions
100 kHz
range
of
interest.
0.5 < f < 13 has
to
100 kHz. Therefore, a ±AF
can be
of the
used
8505A, provided group delay and electrical length readings rescaled. Maximum FM peak deviations of
the HP
8662A/8663A
Table 10.2 below.
Center
Frequency
1
(MHz)
0.01/0.1—120 120—160 160—320
320—640 640—1280
1280—2560*
*HP 8663A only.
on
on
is
limited
peak deviation
For
example,
MHz,
for
the
specified devia-
of
at the
13 MHz
are
are
listed
in
ac Mode (kHz)
The smaller
100
or f
mod
25orf
mod
50
0r
'mod
100orf
mod
200orf
mod
400
or f
mod
X 2
x 500
0.5
to
13 MHz
0.1
Hz
1
Hz
Maximum Peak Deviation
of:
x 500
x125
50
x 1000
x
2000
0.5
to
130 MHz
0.1
Hz
10
Hz
dc Mode (kHz)
100
100 200 400
0.5
to
1300 MHz
0.2
Hz
100
Hz
25 50
Typical system residual
The total phase noise source used with lates into residual FM. Residual limits
the
rate frequency change
at
of the
and
still maintain a stable
display. The residual FM
locked HP 8505A approaches that HP 8662A/8663A, which
of the
the HP which
device under test
FM
signal
8505A trans-
phase
of a
phase-
is
less than
FM
the
or
can
of the
0.1 Hz, allowing very sharp filter skirts to
be
measured.
Output characteristics
Output power, harmonics, spurious, phase noise mined phase noise HP 8505A also affects capability.
of the
by the HP
of the
In the
system
are
8662A/8663A.
source used with
the
measurement
measurement narrow bandwidth notch filter, may attenuate
several kilohertz from
practically
200 kHz from
the
no
attenuation.
the
carrier
but
the
carrier with
If the
carrier mixes with
HP 8505A local oscillator (LO frequency
= RF frequency ±100 kHz) 100 kHz
IF
response <-110 dBm, response will "fill in" the attenuation than
its
limit
the HP 8505A. the
HP
8662A/8663A minimizes this
effect.
The
offset from
of the
true value. This
dynamic range
The low
SSB phase noise
the
carrier
to
the
notch, making
notch appear less
can
effectively
of the
SSB phase noise
at a
is
typically <—136 dBc (Fc= 500 MHz), reducing possibility
of
mixing with
the LO of the
HP 8505A.
and
deter-
The
the
of a the
filter
pass noise
noise
the
produce
a
the
of
200 kHz
the
Delay and electrical length characteristics
The delay
acteristics the improved HP 8662A/8663A. Refer HP 8505A Option Sheet Manual characteristics.
HP
or the
and
electrical length char-
are
primarily a function
8505A,
or
degraded
and
005
Operating
for
more information
thus
are not
by use of the
to the Technical Data and
Service
on
of
these
o
Table 10.2.
Specified
HP
8662A/8663A
FM
deviation.
Page 41
if-
o
Chapter 11
■iSIWcSR.
Using the HP 8662A/8663A as a Substitute LO with the HP 8672A Micro-
wave Synthesized Signal Generator
The low phase noise of the HP 8662A/ 8663A makes it an ideal substitute local oscillator. It is also an excellent substi-
tute for a variable oscillator such as a voltage controlled oscillator (VCO) as it is tunable over a wide range of frequen­cies.
The HP 8662A/8663A can therefore be used as a substitute VCO inside the Hewlett-Packard Model 8672A or 8673A Microwave Synthesized Signal Generator to improve the HP 8672A/8673A phase noise performance and frequency resolu­tion over their 2-to-18 or 2-to-26 GHz frequency range.
System Operation
The HP 8672A is a microwave synthe-
sized signal generator that derives its output frequency from four phase-lock loops (Figure 11.1). The LFS (Low Fre­quency Section) loop determines the four
Rel
Loop
10 MHz
2D
MHz
LFS
Loop
M/N
Loop
Figure 11.2.
HP 8672A YTO loop.
(Figure 11.2), the output frequency of the M/N loop (177.5 to 197.4 MHz) is multi-
plied up to microwave (2 to 6.2 GHz) by
a harmonic mixing process. The sampler
20 to 30 MHz
177.5
10
197.4 MHz
YTO Loop
,
■
2.0 to 6.2 GHz
,
Within the bandwidth of the YTO loop, the noise of the YTO tracks the phase noise of the multiplied signal from the M/N loop. If a very low phase noise signal is substituted for the output of the M/N loop, the improvement in phase noise is translated to the output. Substi­tution of the HP 8662A/8663A for the M/N loop frequency yields the excellent close-in phase noise performance of the HP 8662A/8663A within the YTO band­width (approximately 10 kHz) while still providing good broadband noise per­formance at greater offsets from the carrier.
}&;:*
o
Figure 11.1.
HP 8672A phase-lock loops.
least significant digits of the output fre­quency, while the M/N loop generates the higher-order digits. The outputs from these two loops are inputs to the YTO (YIG-tuned oscillator) loop, a sum loop that translates these inputs directly to microwave frequencies.
Within the bandwidth of a phase-lock loop,
the output VCO noise tracks the noise of the reference. In a sum loop, such as the YTO loop in the HP 8672A,
where two frequencies are used as refer-
ences,
the output VCO noise tracks the
sum of the noise of the two references.
In the HP 8672A, the noise on the output of the M/N loop is the primary
contributor to the phase noise of the final output signal. As indicated in the block diagram of the YTO loop
generates harmonics of the output of the M/N loop and mixes them with the microwave output of the YTO to gener­ate a 20 to 30 MHz difference signal.
The 20 to 30 MHz output of the sampler
thus has the phase noise of the micro-
wave signal generated by multiplying
the 177.5 to 197.4 MHz signal. The phase' noise on the 20 to 30 MHz output from the LFS loop is added to the noise on this microwave signal, but the noise on the 20 to 30 MHz signal is at a much lower level, as it is generated by effec­tively multiplying the 10 MHz reference signal by a factor of only 2 to 3. Com-
pared to the noise on the signal at microwave frequencies, this noise contri­bution is negligible. For more informa­tion on the block diagram of the HP 86 72A, see Hewlett-Packard Applica-
tion Note formance of the 8671A and 8672A Microwave Synthesizers."
218-1,
"Applications and Per-
Hardware Modifications
The necessary modifications to the HP 8672A are easy to do. They involve simple cable re-routing to substitute a signal from the HP 8662A/8663A for the M/N loop frequency in the HP 8672A. Refer to the interior layout photo of the HP 8672A (Figure 11.3) for location of the necessary cabling.
1.
Disconnect green cable from Jl of
A2A3.
2.
Disconnect cable from "20 MHz
OUT" of Reference Loop.
3.
Reconnect the green cable that pre-
viously went to Jl of A2A3 to the "20 MHz OUT" of Reference Loop.
4.
Disconnect the orange/white cable
from "M/N OUT" and reconnect it to the HP 8662A/8663A RF output jack.
5.
Set the HP 8662A/8663A output
level to +4 dBm.
6. Connect the 10 MHz Reference Output from the rear panel of the HP 8662A/8663A to the HP 8672A External Reference Input.
7.
Select EXT REF on the rear panel of
the HP 8672A.
41
Page 42
*'<$■«*
•"1 JT."
Figure 11.3.
HP 8672A A2A3 board.
SYSTEM PERFORMANCE
Spectral Purity
Figure 11.4 shows the measured absolute SSB phase noise of the HP 8672A at 6 GHz using its internal M/N loop and the phase noise with the HP 8662A/
-40
Figure 11.5 shows the analogous results for higher frequencies. Note first that the phase noise of the HP 8672A using its internal M/N loop increased by 6 dB for the 6.2 to 12.4 GHz band, and by 10 dB for 12.4 to 18 GHz, over the noise in the 2 to 6.2 GHz band. This increase in noise is due to the YIG-tuned multiplica­tion of the YTO fundamental output fre-
quency. Similarly, phase noise using the HP 8662A/8663A in place of the M/N loop frequency increases for the higher output frequencies.
Also plotted in Figure 11.4 is the typical phase noise of the HP 8662A/8663A multiplied directly to 6 GHz. Note that a microwave signal generated in this manner has even better close-in phase
noise performance, but the broadband noise is degraded. (For more information on how to multiply and use the
HP 8662A/8663A at microwave frequen-
cies see Chapter 7). For some applica­tions where the lowest possible phase noise is desired, a multiplied HP 8662A/
8663A is the best solution. However, this method of obtaining a microwave signal sacrifices some of the benefits of using a signal generator—calibrated and variable output level, for example. Multi­plication also severely limits AM per­formance; only very low depths of mod­ulation can be multiplied without prohibitive distortion. Harmonic and spurious levels also increase when the
HP 8662A/8663A is multiplied. When these performance parameters cannot be sacrificed, substitution of the HP 8662A/
8663A for the M/N loop in the
HP 8672A provides a better solution. This yields a broad range of 2 to 18 GHz signals with low noise and full modula­tion and output level capability.
o
Figure 11.4.
Effect of HP 8662A/8663A substitution on HP 8672A phase noise at 6 GHz.
8663A substituted for this loop.Note that the close-in phase noise is improved as
much as 20 dB by substituting the
HP 8662A/8663A. The data also shows the relationship between the bandwidth of the YTO phase-lock loop and the resultant phase noise. For offsets greater than the bandwidth of the YTO loop (about 10 kHz), the measured phase noise follows the typical phase noise of the HP 8672A.
10k 100k 1M
Offset from Carrier (Hz)
;
"H20'
Figure 11.5.
Effect of HP 8662A/8663A substitution on HP 8672A phase noise at 18 GHz.
10 100 1K 10K 100K 1M /
Offset from Carrier (Hz)
Page 43
1^5 * t
-,?>'- _-
-? t -J»Tt~
Tax -
"
Resolution
The standard frequency resolution of the HP 8672A is 1 to 3 kHz, depending on output frequency band. Though this is
sufficient for most applications, substitut­ing the HP 8662A/8663A for the M/N loop also results in increased resolution. The frequency resolution varies with output frequency, and is a function of two factors: 1) the harmonic of the HP 8662A/8663A that must be mixed with the 2 to 6.2 GHz output of the YTO
to yield a 20 to 30 MHz difference signal, and 2) the band the HP 8672A is operating in. To determine the resolution it is necessary to examine the frequency algorithm.
Frequency Algorithm
For a desired HP 8 6 72A output fre­quency the necessary 177.5 to
197.4 MHz signal from the HP 8662A/
8663A and HP 8672A setting can be
readily calculated. First, the output band of the desired HP 8672A signal must be determined. The fundamental frequency band of the HP 8672A is 2.0 to 6.2 GHz, the range of the YTO in the block dia-
gram of Figure 11.1. The other frequency bands are obtained with a YIG-tuned multiplier, selecting either the second or third harmonic of the fundamental band. Let F be the desired frequency in MHz and B, the output frequency band of the HP 8672A, where
1,
2<F<6.2GHz
2,
B
6.2 < F < 12.4 GHz
3, 12.4 < F < 18.6 GHz
Then the frequency that the YTO must tune to is
F -f
YTO B
This YTO frequency requires an N in the M/N loop of
F
+
300
N = INT
where INT(X) is the integer value< the value of X.
The necessary HP 8662A/8663A fre­quency is then
8662A/8663A
YT0
200
f\TO + 20
and the HP 8672A should be set to
F
10
X 10
INT
Note: All above frequencies have
units of MHz.
The output resolution will then be equal to
(resolution of HP 8662A/8663A) X N X B
As an example, if the desired HP 8672A output frequency is
10.5 GHz, B = 2,
F
= 10.5/2 =
YT0
5.25 GHz. Then N = INT [(5250 +
300)/200] = INT (27.75) = 27. The HP 8662A/8663A should therefore be set to
c
t*Au
F
aae2A
/86
i 5250 + 20
MHz
63A(
) = jjy =
195.1851852 MHz
and the HP 8672A tuned to F
(MHz) = INT ^°-x10 =
8672A
10
10500 MHz
The resolution of this output signal is
0.1 Hz X 27 X 2 = 5.4 Hz.
Note: When the HP 8672A is operated in this mode, the "not phase-locked" annunciator on the HP 8672A remains on. This is because the M/N loop is
unlocked, but this loop is not used to derive the HP 8672A output fre­quency. The signal at the HP 8672A output port is phase-locked if the "REF LOOP", "YTO LOOP", and "LFS LOOP" LED's are glowing on the HP 8672A A2A7 Interface Assembly Board and if the HP 8662A/8663A does not display a hardware status
message.
Modulation
This configuration also allows the HP 8672A to have increased modula­tion capability. The standard modula­tion capability of the HP 8672A remains unchanged, but the modula­tion performance of the whole system can be expanded by modulating the
177.5 to 197.4 MHz signal. A standard
HP 8672A's FM is limited by modula­tion index: m must be less than 5 for carrier frequencies from 2 to 6.2 GHz,
less than 10 from 6.2 to 12.4 GHz, and less than 15 from 12.4 to 18 GHz. However, because any frequency modulation on the 177.5 to
197.4 MHz signal is translated with the signal up to microwave frequency
by the YTO loop, it is possible to fre­quency modulate the carrier with a very high modulation index.
It is possible to FM at rates up to the YTO loop bandwidth, approximately 10 kHz. Frequency modulation is lim-
ited by the ability of the YTO loop to respond, and at low rates peak devia­tions in excess of 1 MHz are possible (Figure 11.6). Switching the HP 8672A to the FM mode (with no modulation
input to the HP 8672A) allows the FM OVERMOD indicator on the front panel to be used to determine if the frequency deviation applied to the
177.5 to 197.4 MHz signal is so large the YTO loop cannot respond prop­erly. For modulation applied to the
substituted M/N loop frequency, there is no FM meter indication on the HP 8672A.
Figure 11.6.
Increased FM performance with HP 8662A/8663A substitution.
The HP 8662A/8663A for output fre­quencies between 177.5 and
197.4 MHz allows peak deviations up
to the smaller of 50 kHz or up to
f
mod
X 250. However, the frequency devia­tion set on the HP 8662A/8663A gets
translated up in the YTO loop. The HP 8672A deviation is then equal to the deviation set on the HP 8662A/ 8663A X N X B. For low rates, this yields frequency modulation with a very high modulation index.
43
Page 44
-*; n ;■■'«
mmmi
■1&*&tz
Chapter 12
:.fc>
Fast/Ffequency Switching with the
HP
8662A/8663A
The combination of low noise and fast frequency switching is unusual and diffi­cult to achieve in synthesized signal gen­erator design. The HP 8662A and HP 8663A optimize these conflicting design requirements providing excellent SSB phase noise, as discussed in the pre­vious chapters, and frequency switching as fast as 420 ^sec for the HP 8662A and 510 Msec for the HP 8663A, to be within 100 Hz accuracy.
Standard HP-IB Frequency Control
An understanding of the standard HP-IB frequency control of the HP 8662A/
8663A is helpful for programming and utilizing the fast frequency switching capabilities of the instrument. In the normal operating mode of the HP 8662A/8663A, programming a spe­cific frequency is accomplished in a three step process. First a string of binary fre­quency data is sent to the instrument over the HP-IB. Secondly, the instru­ment microprocessor operates on this string breaking it into binary data seg­ments necessary to control the phase
locked loops and output circuitry. This processed binary data is sent to the fre­quency control board where it is loaded into latches and clocked out to the instrument to set the frequency of the output signal. In the third step of the process the phase locked loops and output circuitry switch and settle to the desired final frequency.
The last two steps contribute to a total switching time of 12.5 milliseconds. Since the time contribution from the first step is determined by the external
controller it will not be discussed. The dominant contributor to instrument switching time is step two, the data processing time of the microprocessor. This consumes approximately 12.1 msec. During this time, the microprocessor does two things to the frequency control data. It scales the desired frequency to a frequency in the fundamental band of the instrument and selects the range information necessary to transform the fundamental frequency to the desired output frequency. The scaled frequency
data is necessary for the phase locked loops to synthesize the correct funda­mental frequency (320 to 640 MHz in
.1 Hz steps), as discussed in Chapter 3.
The range information is necessary for the output section to select the correct
means of translating that fundamental to
the desired frequency, either by multi-
plying, dividing, or heterodyning, if it
does not already lie in the fundamental band. For example, to output a
frequency of 100 MHz, the micro­processor scales the frequency to the fundamental band by adding 520 MHz. A 620 MHz signal is synthesized in the PLL section and sent to the output section. Range data representing the 10 to 120 MHz range alerts the output section that the desired frequency is in the heterodyne band and switches in the output section mixer. Table 12.1, Frequency Scaling and Ranging, lists
desired output frequency, the corre­sponding fundamental frequency, the scaling factor to get from the desired frequency to the fundamental band, and the appropriate range information.
Overall switching time is depicted in Figure 12.1 Typical Frequency Switching Times. The microprocessor time to scale and range the frequency command is the dominant contributor, and switching and settling time add approximately 420/510 fisec to settle to within 100 Hz. Depending on the switching accuracy required, switching and settling time can
be as fast as 250 jtsec for settling to
within 1 kHz. As can be seen from the graph, substantial improvement in switching speed can be achieved by
eliminating the microprocessor time.
1 Desired Output
j Frequency
I (MHz)
- .1 to
119.9999999
120 to
159.9999999 160 to
319.9999999 320 to
639.9999999 640 to
1279.9999998
•1280 to
2559.9999996
•8663A only. |
Fundamental
Frequency
(MHz)'
520.1 to
639.9999999
480 to
639.9999996
320 to
639.9999998 320 to
639.9999999 320 to
639.9999999 320 to
639.9999999
Fast Learn Frequency Switching
The fast learn mode eliminates HP 8662A/8663A data interpretation time by providing a means for an external controller to "learn" the appro­priate binary data segments in advance. Outputting only the binary frequency data and bypassing the instrument microprocessor significantly decreases switching time of the synthesizer. Settling time becomes the determinant switching speed factor, settling time
being primarily due to the response and
transient settling time of the phase
locked loops. In the fast learn mode,
switching times of 420/510 fisec are possible for the HP 8662A/8663A with the majority of this time attributed to instrument settling to within 100 Hz.
To eliminate the microprocessor time of the HP 8662A/ 8663A binary data is
sent via HP-IB that has already been ranged and scaled to the fundamental band of the instrument. Processing the frequency command beforehand allows strings of frequency data to be output to the instrument and executed immedi­ately. The data string for the fast learn mode consists of 11 characters for the HP 8662A and 16 characters for the
Table 12.1
Frequency Scaling and Ranging
Scaling
Factor
Frequency I
Range ^_
(MHz) ■
+520 MHz
to ^H
to ^M
i to ^M
10 to 120 ^M
X4
X2
XI
2
4
120 to 160 ^fl
160 to 200 ^M
220 to 320 ^M 320 to 450 ^M
450 to 640 ^H 640 to 900 ^H
900 to 1280 ^M 1280 to 1800 ^H
1800 to 2560 ^H
o
Q
Page 45
o
HP 8663A. Each character consists of 1
byte,
8 bits per byte. This string contains 2 "fast learn" characters to instruct the instrument to interpret the subsequent data as fast learn information, 5 charac-
ters that contain the fundamental band frequency data, 1 character that contains
the range data, and the final characters
contain modulation information. The string configuration is shown in Figure 12.2, Fast Learn Character String.
The data strings can be set up by either of two methods, reading or "learning" the string from the synthesizer and stor-
ing it to be output later, or by program­ming the controller to assemble the string. When the string is read from the synthesizer in the fast learn mode, the
Figure 12.1.
Typical frequency switching times.
instrument configures the data string or
the controller. Bytes 3 through 7 repre­sent the frequency digits, byte 3 being
the least significant digit. Two decimal characters are contained in each byte in BCD format. Byte 8 contains the range information, the range information is not coded in any particular manner and is listed in Table 12.3, Fast Learn Charac­ters.
Bytes 9 through 11/16 contain the
modulation control data.
Figure 12.2
Fast Learn Character String
front panel of the instrument is set to
the desired frequency and modulation
(all functions except phase modulation and amplitude can be programmed in the fast learn mode). The controller reads the ranged and scaled binary data from the HP 8662A/8663A and stores it in an array. The data in the array is then output to the HP 8662A/8663A for fast switching. This alleviates the need for the operator to know how to format the fast learn string.
If many frequencies are to be output to the HP 8662A/ 8663A, in a random or real time fashion, it may be more practi­cal for the external controller to format the fast learn strings. Figure 12.2 and Table 12.2 show the structure of the
J
binary coded data and give an example to realize 812.62345 MHz. The first two bytes of the string are the fast learn mode prefix, these are always the same in the fast learn mode, whether the
From Table 12.1, to scale the example frequency of 812.62345 MHz to the fundamental band, the frequency is divided by 2. Each digit in the resulting fundamental frequency is converted to a
Byte
1 2
3 4 5 6
7 8
9-11/16 Example: Scale to fundamental: 812.62345/2 = 406311725.0 Hz"~i~;;*tf$£.7
Table 12.2
Fast Learn Character String Example
Bits
0100 0000 0011 1001
0101 0000
0111 0010 0001 0001 0110 0011 0100 0000 0001 0101
•
Frequency = 812.62345 MHz
Information
Fast Learn mode prefix -•_ -~ "J Fast Learn Least significant digits: \ i * ,
gjg 6311725.0 . Hz ' l\ Range 640 to 899.999998 MHz",' Modulation control bytes*- - y-
mode'
40631172 ffigg-- Hz • ... i
406311 §g| 5.0 Hz­4063 jg| 725.0 Hz r^-^' 40 g§ 11725.0Hz
:-.jm?£
prefix
t u
_"
"
;• •
:•*,.,
45
Page 46
4-bit binary equivalent, bytes 3 through
7 in Table 12.2. The range data is found
in Table 12.3. The complete string of
11/16 bytes is stored by the controller and output over the HP-IB in bytes.
On receipt of the ll/16th byte,
8-bit
the HP 8662A/8663A clocks the binary data from the frequency control board out to the rest of the instrument.
Figure 12.3 presents typical fast learn
software using an HP 9836 series 200 controller. The program first reads the front panel setting of the HP 8662A/
8663A and stores this string in an array. This sets up a fast learn string that contains the fast learn mode characters, frequency and range data for 100 MHz, and preset modulation conditions. The program then manipulates bytes 3 through 8, the frequency and range bytes.
As each frequency is input it is scaled to the fundamental band, the digits are converted to binary by trans­lating them two at a time to their ASCII equivalent (starting with the most
signif-
icant digits). The appropriate range infor-
mation is selected, and the resulting characters replace bytes 3 through 8 in
the original fast learn string. After the
last frequency is input, the program concatenates the 11/16 byte words and outputs them to the HP 8662A/8663A. In the fast learn mode this frequency data bypasses the microprocessor, is fed directly to the frequency latch board and instrument switching time is reduced from 12.5 msec to 420/510 /*sec.
Byte
Bits/Byte
0100
0000
0011
1001
xxxx xxxx xxxx xxxx
xxxx xxxx xxxx xxxx xxxx xxxx
"Fast" learn mode prefix "Fast" learn mode prefix
Bytes 3-7 contain the frequency digits scaled to the fundamental band of the ­HP 8662A/8663A (320 to
639.9999999 MHz). The first four bits of byte 7 contain the most significant digit,
Comments
all digits are in BCD.
xxxx xxxx
Range control data, the following binary codes represent valid range data:
0000 0100 320-449.9999999 MHz
0000 0000 450-639.9999999 MHz 0110 1001 .1-.1499999 MHz 0100 1001 .15-.9999999 MHz 0100 1001 .15-.9999999MHz 0010 1001 1-10 MHz 0000 1001 10-119.9999999 MHz 0000 0011 120-159.9999999 MHz
0110 160-219.9999999 MHz
0000
0001 220-319.9999999 MHz
0000
0101 640-899.999998 MHz
0001
0001 900-1279.9999998 MHz.
0001
1101 1280-1799.9999996 MHz,
0001
HP 8663A only. 0001
9-11/16
1001 1800-2559.9999996 MHz
Modulation I/O control, preset condition.
Note: X's represent data inputs that will change according to the
T frequency to be programmed.
Table 12.3
"Fast* Learn Characters
Figure 12.3.
Fast learn programming
46
I FAST LEARN PROGRAMMING I 168 MainiCALL Clearscreen
Page 47
Page 48
Fast Frequency Switching Option H-50
The H-50 Option is similar to the fast learn mode in that it circumvents the HP 8662A/8663A microprocessor, send­ing data directly to frequency and range data latches. The basic difference
between fast learn and option H-50 is
that the data to the frequency and range latches is input from an external 50-pin parallel interface on the back panel of the instrument. This provides direct binary frequency input to the HP 8662A/
8663A so that it can be interfaced with a device under test or other equipment in a test system. Frequency can be con­trolled through HP-IB with an appropri­ate interface board to provide the paral­lel inputs to the H-50 connector. Option H-50 will control only frequency, other functions must be set either from the front panel or via the normal HP-IB port.
1 Connector | Pin
1 l
2 3
4
5
1
6
7 8 9
10 11 12 13
14 15 16 17 18 19 20 21
22 23 24 25 26
Bit
Designation
DF9-1
DF9-2 DF8-1 DF8-2 DF8-4 DF8-8 DF7-1 DF7-2 DF7-4 DF7-8 DF6-1 DF6-2 DF6-4 DF6-8 DF5-1 DF5-2 DF5-4
DF5-8 DF4-1 DF4-2 DF4-4 DF4-8
DF3-1 DF3-2 DF3-4 DF3-8
Digit/
Range
100's MHz
10's MHz
l's MHz
100'sKHz
10's KHz
l's KHz
100's Hz
Table 12.4 defines option H-50 connector pins and corresponding frequency and range control inputs. The frequency and range data inputs respond to TTL positive-true logic levels, the DFI (Direct
Frequency Interface) line requires a TTL low level to enable the H-50 option. The Data Valid line clocks the frequency and range data into the DFI latches on a TTL positive transition. For example, to switch the HP 8662A/8663A to
812.62345 MHz, the frequency is first scaled to the fundamental band, to
406.311725 MHz, and each digit trans­lated to a 4 bit binary equivalent as shown in the example column of Table 12.4. The appropriate coded range data, 640 to 900 MHz, is selected from Table 12.5. TTL levels corresponding to a
"1"
or "0" applied to the appropriate connector pins cause the HP 8662A/ 8663A to switch in 400 fisec to within 100 Hz accuracy upon receipt of a Data
Example:
812.62345 MHz/2 = 4063117250 Hz
Binary Decimal
0 4"Note 0 0 0 0
0 . . .
0
0 6
1 1 0 1 3 1 0 0 1 1 0 0 0
"11.
0 0 0
1 7
1 1
0
Connector
Pin
27 28 29 30 31 32
33 34 35 36 37 38 39 40 41 42 43 44
45 46 47 48 49 50
Bit
Designation
DF2-1 DF2-2 DF2-4 DF2-8 DF1-1 DFI-2
DFI-4 DFI-8 DF0-1 DF0-2 DFO-4 DFO-8 R0 Rl R2 R3 R4 R5 R6 N/C N/C
DFI Enable Data Valid Ground
Valid line TTL positive transition.
Summary
The Fast Learn mode and Option H-50 provide extremely fast switching of the HP 8662A/8663A while maintaining the
spectral purity of the synthesizer. The fast learn mode is advantageous where programming flexibility is required, as in ATE systems. In this mode simple HP-IB control of the HP 8662A/8663A pro­vides fast frequency switching with mod­ulation control, and remote program­ming of other normal instrument functions. The H-50 option is well suited to dedicated dynamic testing of secure
communications receivers and frequency hopping systems, or other tests that require synchronous frequency switching.
Digit/
Range
10's Hz
l's Hz
.l's Hz
Range Select Range Select Range Select Range Select
Range Select Range Select Range Select
0 2
1 1
0 |
0 j
15 I
0 1
1 I 0 1 0 0 1
o
I
0 1 0 I
1 1
0 1
1 1
0 E i E 0 I 0 1 X I X 1 0 8 1 1
x
8
Table 12.4
H-50 connector pin outs.
NOTE:
The lower 9 digits of the frequency data,
10's MHz through .l's Hz (DF8-DF0), are represented by a 4-bit BCD code. The weighting of each of the bits is indicated by the
"-1"
through "-8" suffix to the bit
designation (e.g., DF8-1 is the LSB for
digit 8 and DF8-8 is the MSB for digit 8). The 100's MHz digit is represented by the lower two bits of a 4-bit BCD code for decimal values between 3 and 6 inclusive. Since these are the only values that this digit can validly assume, there is no need for a full 4-bit BCD representation.
100's MHz
Decimal Value
3 4 5 6
DF9-1
1 0 1 0
DF9-2
1 0 0
1
Page 49
The range data is not coded in any particular manner. The values that the individual bits must assume for a given frequency range are indicated in Table 12.5.
Frequency
Range
1
(MHz)
.15 < f0 < 1
1 < f0 < 10
10 < f0 < 120
120 < f0 < 160
160 <f0< 220 220 < f0 < 320 320 < f0 < 450 450 < f0 < 640 640 < f0 < 900
900 < f0 < 1280
•1280 < f0 < 1800 *1800 < f0 < 2560
f0 = Output frequency of the instrument
•8663A only.
Table 12.5
H-50 range data coding.
R0
f0 < .15
Range Data
R1
R2
R3
R4
R5
R6
1
0
1
0
1
0
1
0
1
1 1
0
1
0 0
0 0
0
1
0
1
0
1
0
1
0
1
0 0 0 0 0 1 0 1
0
1
0
1
0
0
1
1
1
0
1
.0
1
0
0
0
0
0
0
0
0
0
0
0
0
1
0
1
1
1
1
1
1
0 1
0 0
0 0
0
0
0
0
0 0
0 0
0 0
0
0
0
0
0
0
0
C Start J
Select 8662A 8663A?
Enter Frequencies
'Frequency \
\ ~07 /
Create Bytes
Create Fast Leam String
T
Enter # Cycles
I
Output Fast Learn String to 62/63
Stop Fast Output (Send Dummy Byte)
E"d
(
)
Figure 12.4.
Flow chart for Fast Leam Program
49
Page 50
Calculation of Phase Noise of Three
Unknown Sources
Given three unknown sources: 1,2, and 3.
Using the two source technique, measure each source against each other source in three measurements, yielding
P12 = Jt measured of sources
1 and 2 in dBc,
P23 = Jt measured of sources
2 and 3 in dBc,
P13 = Jt measured of sources
1 and 3 in dBc.
The phase noise performance of each source may be calculated from the fol­lowing formulas:
B
10 MHz Low Noise Bandpass
Amplifier
Hewlett-Packard assumes no responsibil­ity for the use of any circuits described herein and makes no representations or
warranties, express or implied, that such circuits are free from patent infringement.
Jf, in dBc = 10 log
Jt2 in dBc = 10 log
Jt3 in dBc = 10 log
P-I2 F*13 ^23
10 10 10
10 +10 -10
Low Noise Amplifier
Page 51
Appendix
D
References
Hewlett-Packard Application Notes
AN 207 Understanding and Mea-
suring Phase Noise in the Frequency Domain
AN 150-4 Spectrum Analysis ...
AN 270-2
AN 225
AN 286-1
AN 218-1
AN 246-2
AN 983
PN 11729B-1
Noise Measurements
Automated Noise Side­band Measurements Using the HP 85 68A Spectrum Analyzer
Measuring Phase Spectral Density of Synthesized Signal Sources Exhibiting f and f Noise Characteris­tics with the 5390A Fre-
quency Stability Analyzer
Applications and Opera­tion of the 8901A Modu­lation Analyzer
Applications and Perform­ance of the 8671A and 8672A Microwave Synthesizers
Measuring Phase Noise with the HP 35 85A Spec­trum Analyzer
Comb Generator Simpli­fies Multiplier Design
Phase Noise Characteriza­tion of Microwave Oscilla­tors Phase Detector Method
Other References
1.
D. Scherer (H-P), "Design Principles
and Test Methods of Low Phase Noise RF and Microwave Sources",
Hewlett-Packard RF and Microwave
Symposium, October 1987. (Also edited version in Microwaves, "Today's Lesson—Learn about Low­Noise" Part 1, pp. 116-122, April
1979,
Part pp. 72-77, May 1979.)
2.
Hewlett-Packard Journal, February
1981,
Volume 32, No. 2.
3.
Fischer, M. (H-P), "Frequency Stabil-
ity Measurement Procedures", pre­sented at the 8th Annual Precision Time and Time Interval Meeting, December 1976.
4.
A.L. Lance, W.D. Seal, F.G. Men-
doza, and N.W. Hudson (TRW), "Automated Phase Noise Measure­ments", Microwave Journal, June
1977.
5.
"Frequency Domain Stability Meas-
urements: A Tutorial Introduction", David A. Howe, N.B.S. Technical Note 679, National Bureau of Stan­dards,
Boulder Colorado, 80302,1976.
6. McNamee, M.(H-P), "Automate for Improved Phase Noise Measure-
ment", Microwaves, May 1979.
7.
Giacoletto, L., "Electronics Design-
er's Handbook", Second Edition,
McGraw-Hill, 1977, pp. 26-32.
8. D. Scherer (H-P), "Generation of Low Phase Noise Microwave Sig­nals",
HP RF and Microwave Mea-
surement Symposium, May 1981.
9. R. Temple (H-P), "Choosing a Phase Noise Measurement Technique", HP RF and Microwave Measurement Symposium, Jan 1983.
10.
D. Scherer (H-P), "Art of Phase
Noise Measurements", HP RF and Microwave Measurement Sympo­sium, May 1983.
11.
R. Burns (H-P), "8901B Adjacent
Channel Power", HP RF and Micro-
wave Measurement Symposium, May 1983.
PN 11729C-2
Phase Noise Characteriza-
tion of Microwave Oscilla­tors Frequency Discrimi­nator Method
51
Loading...
Buy Points How it Works FAQ Contact Us Questions and Suggestions Privacy Policy Cookie Policy Terms of Use