GBS Elektronik MCA166-USB User Manual
Application Note
Behavior of the MCA 166 at different Temperatures and
Gain settings and limits of centroid accuracy
10.1.2001
Jörg Brutscher
GBS Elektronik GmbH
1. Problem
2. Basic limits
3. Centroid error
4. Temperature drift of the MCA 166
5. Temperature drift of detectors
6. Stabilisation
7. Linearity and accuracy of gain setting
8. Conclusions
1. Problem
For very accurate measurements, all possible influences on measurement performance have to be known and
understood. This helps to estimate errors and to improve measurement conditions. In this document, the
temperature drift and the linearity of the gain and its dependence on settings have been examined.
2. Basic limits
Gamma spectroscopy can be a very accurate measurement method in terms of measuring photon energy. The
limits are mostly given by detector resolution. For example, the FWHM of the Co60 1333 keV peak with
measured with a HPGe (High purity Germanium) detector is typical 1.85 keV. A useful assumption is that the
centroid of a peak can be calculated with an error of 10% of its FWHM. This is a measurement error in this case
of 1.4E-4=140 ppm.
With other detectors the error is larger.
Table 1: typical photon energy measurement errors for different detectors.
detector
condition
relative error
HPGe
1.85 keV FWHM at 1333 keV
1.4E-4
CZT
12 keV FWHM at 662 keV
1.8E-3
NaI
7.5% FWHM at 662 keV
7.5E-3
Gamma spectroscopy with semiconductor or scintillation detectors is a relative measurement method which relies
on calibration by standard photon sources. So main requirements are linearity and stability. The most interesting
point is the peak drift caused by temperature change. Temperature drift may be caused by the detector crystal, the
preamplifier and the main amplifier within the MCA. For accurate measurements it is desirable to keep
conditions such that the drift does not exceed the relative errors mentioned in table 1.
Absolute values of gain are not critical because the MCA has to be calibrated anyway. The absolute accuracy of
the MCA166 gain setting is a few percent.
2. Centroid error
Centroid
i
Spectrum
Spectrum
i
i
l
h
i
i
l
h
=
=
=
∑
∑
*
(1)
The peak centroid is the sum of the background corrected channel
contents multiplied by the channel number and divided by the sum of
the background corrected channel contents. Only the channels above
the half maximum are used (MCA measurement software algorithm).
From this definition it can be derived that there is a systematical and a
statistical component of the centroid error.
Statistical error
The measurement of a peak in a spectrum can be seen as N repeated measurements of the corresponding photon
energy, where N is the peak area. The standard deviation of a single measurement is 42.5% of the FWHM of the
gaussian distribution. The standard deviation of the average is normally the standard deviation of a single
measurement divided by the square root of the number of single measurements. In the definition only the
channels above half maximum are used, which represent 76% of the total peak area. This yields in a statistical
centroid error depending on peak area N of
∆
E
FWHM
N
≅ ×
05.
(2).
systematic error:
From the definition above, it can be derived that the centroid is not a linear function of the
photon energy, but has some discontinuities. This discontinuities occur, when a channel at the edge has about half
of the maximum counts and it is decided whether to include it into the centroid calculation or not. The difference
when including another channel is
∆
n
issmsis
s
s
iim
i
m
i
= −
⋅ +
+
∑
∑
∑
∑
.
The edge channel m is about 0.5*FWHM from the centroid:
is
s
m
FWHM
i
i
∑
∑
− = ⋅
05.
,
the gaussian distribution s of the peak is
( )
( )
s
area
e
area
FWHM
e
i
ini
n
FWHM
= =
⋅
−
−
σ π π
σ
2
8
2
2
0
2
2
0
2
2
2
8
2
2
ln
*
ln
,
the sum of the channels above FWHM is
s
area
i
∑
= ⋅
076.
, the content of the edge channel is just half of the
maximum channel
s
area
FWHM
m
=
⋅ ⋅
128
22ln
π
and the ratio is
s
s
FWHM
FWHM
m
i
∑
=
⋅
⋅
=
2
2
0762
0
618
ln..
π
.
Assuming now that the FWHM (in channels) is large (>4), the difference can be estimated as
∆
n
FWHM
s
s
s
s
FWHM
FWHM
m
i
m
i
=
⋅ ⋅
+
≅ ⋅ ⋅ =
∑
∑
0
5
1
0
5
0
618
0
309....
(3)
So almost independent from FWHM, this centroid calculation algorithm causes discontinuities of about 0.3
channels, which can be seen as an systematic error of +/- 0.15channels. As this discontinuities are caused both by
channels on the left and the right side of the peak, it is better to multiply this value with 2which results in an
error of +/- 0.21 channels to be assumed.
101001000
Peak area
0.0
1
0.1
1
Centro id erro r (in FWHM units)
351.9
9
609.1
4
351.9
6
609.2
3
352.0
7
1118.
3
FWHM 3channel
s
FWHM 11.5 channel
s
FWHM 46 channel
s
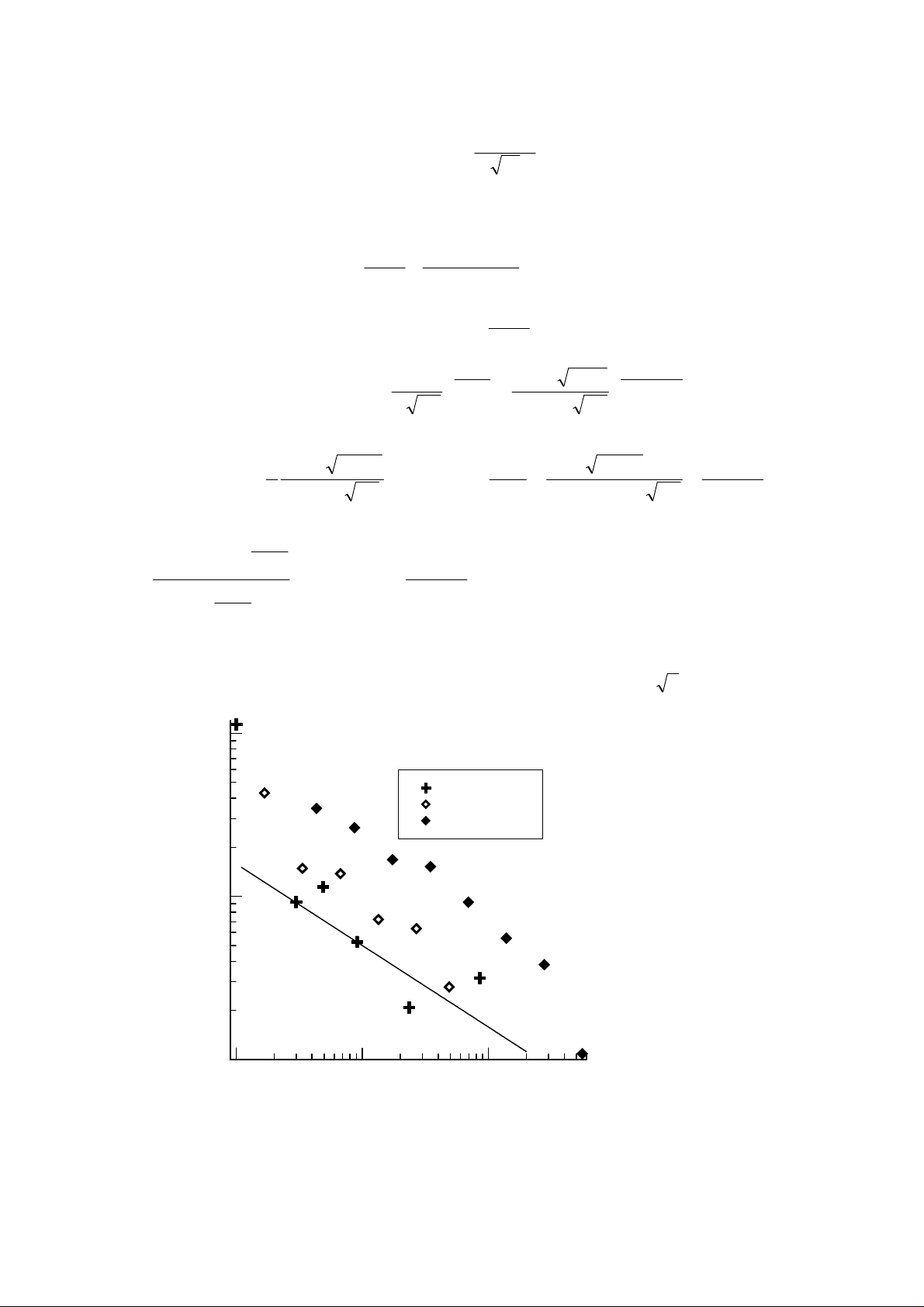
Fig. 1. Centroid error dependend on peak area using peaks which are large compared to background. If the
FWHM is only a few channels, the formula derived for statistical centroid error can be applied. If FWHM is too
large, then the centroid error is increased due to strong fluctuations of the channels used for evaluation.
In an experimental study of the centroid error it was found that there is a third contribution to the centroid error if
the peak area is distributed to many channels. In this case, the statistics of a single channel content is very bad.
This causes the FWHM determination algorithm to work incorrect and the fluctuation of channels used for
centroid calculation is much larger than one channel. This also leads to an increased centroid error. To avoid this,
it is recommended to adjust the MCA resolution in a way that the FWHM is about 4 - 8 channels.
To reduce the range of possible centroid errors, it can be stated that peaks with an area <30 counts are hardly
recognizable and centroid errors < 5%FWHM seem hard to believe. So for practical purposes the following may
be assumed (if FWHM=3...12channels and the peak is large compared to background):
Table 2
Area
Centroid error
peak area<30
not a peak
30<peak area <400
∆
E
FWHM
peak
area
≅
peak area>400
FWHM*0.05
4. Temperature drift of the MCA 166
At first, it is evaluated how the drift changes with time. Knowing the thermal time constant allows to judge how
long it necessary to wait until the MCA runs stable.
It also tells that for measurement times short compared to the time constant, resolution losses due to gain drift can
be reduced.
05010015020025030035040
0
Time (min
)
0.988
0.9
9
0.992
0.994
0.996
0.998
1
Centroi d drift
MCA 166 warming up from about -7°
C
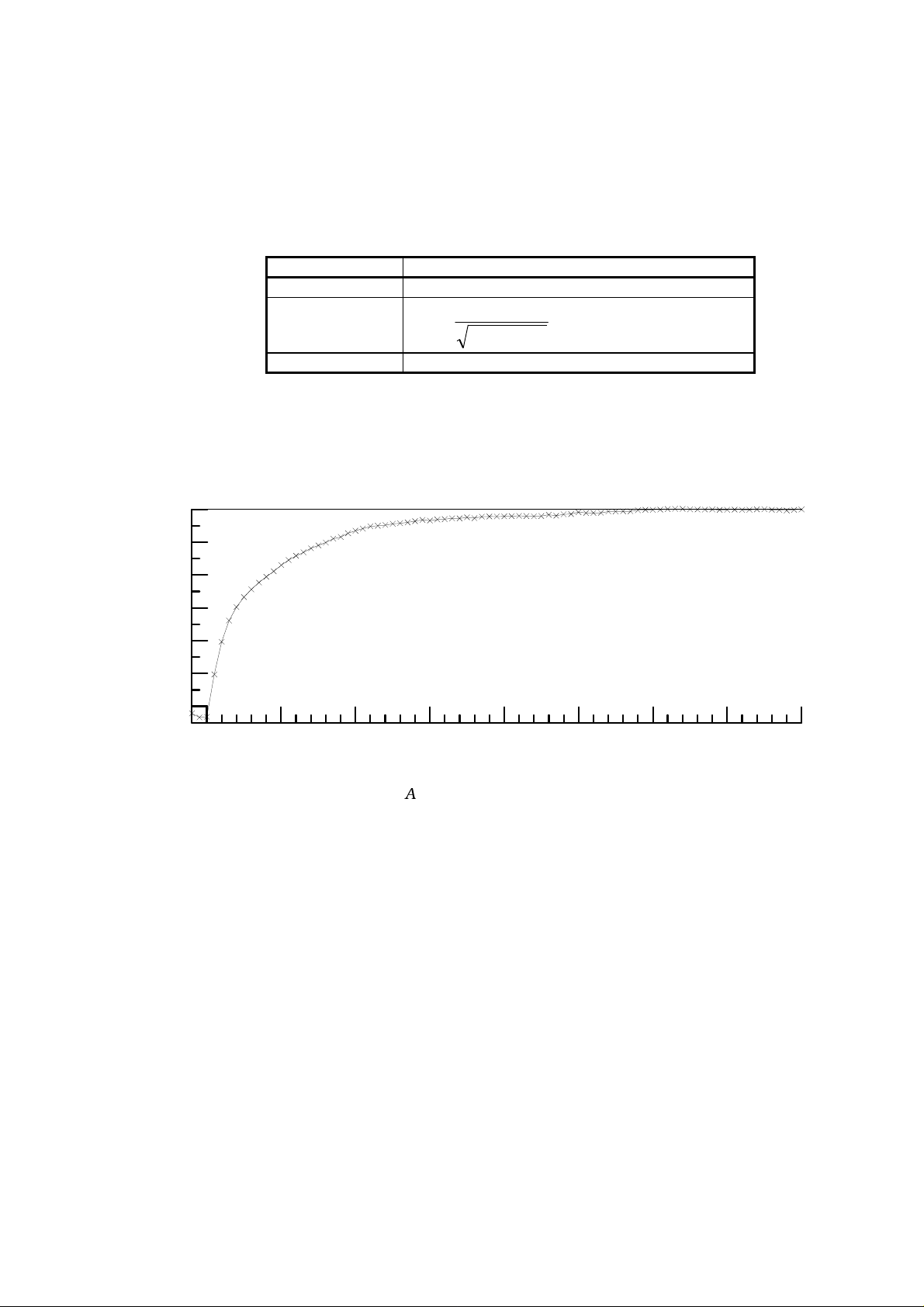
Fig. 2. Reaction of the MCA166 on a sudden temperature change from -7°C to +20°C. The thermal time
constant (1/e) can be evaluated as 33 minutes. planar high resolution HPGe detector type GL0310 which was
kept at constant temperature measuring a Co60 source was connected to the MCA.Spectra were taken in 5
minute intervals and the drift of the 1333 keV peak was evaluated. Shaping time was 2µs, Gain =5*0.6.
The drift reaction caused by switching on the device is similar to that on a thermal change of 4-7°C. So, for
perfect stability it is a good idea to leave the MCA at least 3 hours running to come to a thermal equilibrium. For
short measurements (few minutes) drift will not affect resolution, as drift is very slow. However, energy
calibration may have to be readjusted a few times.
In the next experiment it was measured how gain changes with temperature and if there are other dependencies.
For this experiment a GL1015R with a Ra226 source was used. The MCA was serial number 140 (one of the first
series). The count rate was about 6500 cps and 13% dead time (2µs shaping time). The MCA was in a climatic
chamber and all the time connected to the charger. After temperature adjustments the next measurement was
started earliest after 4h to allow the MCA to find its thermal equilibrium. This temperature test was also meant to
check the reliability of the MCA electronics. Below -10°C, the battery voltage was too low to allow correct MCA
operation without charger. The tests were stopped at -40°C due to lack of time and because this is far out of
specifications (normal minimum temperature -5°C).
In first order and within the specified temperature range, the gain change with temperature can be considered
linear. Only at very low temperatures there seems to be a nonlinear effect. Much more interesting is that the drift
depends strongly on the gain setting. The drift is sometimes positive, sometimes negative and sometimes nearly
negligible.
Loading...