Analog Devices EE183v04 Application Notes

Engineer-to-Engineer Note EE-183

Technical notes on using Analog Devices DSPs, processors and development tools

Contact our technical support at dsp.support@analog.com and at dsptools.support@analog.com Or vi sit our o n-li ne r esou rces htt p:/ /www.analog.com/ee-notes and http://www.analog.com/processors

Rational Sample Rate Conversion with Blackfin® Processors

Contributed by Jeff Sondermeyer, Senior DSP FAE Rev 4 – March 18, 2004

Introduction

The process of converting the sampling rate of a signal

from one rate to another is called sampling rate conversion

(or SRC). This technique is encountered in many application areas such as:

• Digital Audio (the focus of this paper)

• Communications systems

• Speech Processing

• Antenna Systems

• Radar Systems

Sampling rates may be changed upward or downward. Increasing the sampling rate is called interpolation, and decreasing the sampling rate is called decimation.

Reducing the sampling rate by a factor of M is achieved by discarding every M-1 samples, or, equivalently keeping every M’th sample. Increasing the sampling rate by a factor of L (interpolation by factor L) is achieved by inserting L-1 zeros into the output stream after every

sample from the input stream of samples.

This system can perform SRC for the following cases:

reduction of the sampling rate and interpolation is the increasing of the sample rate.

Decimation

A reduction of sample rate (decimation) by a factor of M is achieved by sequentially discarding M-1 samples and retaining every M’th sample. While discarding M-1 of every M input samples reduces the original sample rate by a factor of M, it also causes input frequencies above one-

half the decimated sample rate to be aliased into the frequency band from DC to the decimated Nyquist frequency. To mitigate this effect, the input signal must be lowpass filtered to remove frequency components from portions of the output spectrum which are required to be alias free in subsequent signal processing steps. A benefit of the decimation process is that the lowpass filter may be designed to operate at the decimated sample rate, rather than the faster input sample rate, by using a FIR filter structure, and by noting that the output samples associated

with the M-1 discarded samples need not be computed.

• Decimation by a factor of M

• Interpolation by a factor of L

• SRC by a rational factor of L/M.

SRC by L/M requires performing an interpolation to a sampling rate which is divisible by both L and M. The final output is then achieved by decimating by a factor of M.

Appropriate lowpass filtering is required to prevent both imaging and aliasing. This system employs the polyphase, multistage technique in the process of the sampling rate conversion for computational savings.

1.1 Sample Rate Conversion Designs

SRC designs use the basic properties of decimation and interpolation to change sampling rates. Decimation is the

Copyright 2004, Analog Devices, Inc. All rights reserved. Analog Devices assumes no responsibility for customer product design or the use or application of customers’ products or for any infringements of patents or rights of others which may result from Analog Devices assistance. All trademarks and logos are property of their respective holders. Information furnished by Analog Devices Applications and Development Tools Engineers is believed to be accurate and reliable, however no responsibility is assumed by Analog Devices regarding technical accuracy and topicality of the content provided in Analog Devices’ Engineer-to-Engineer Notes.

Interpolation

An increase in sample rate (interpolation) by a factor of L is achieved by inserting L-1 uniformly spaced, zero value samples between each input sample. While adding L-1 new

samples between each input sample increases the sample

rate by a factor of L, it also introduces images of the input

spectrum into the interpolated output spectrum at frequencies between the original Nyquist frequency and the higher interpolated Nyquist frequency. To mitigate this effect, the interpolated signal must be lowpass filtered to remove any image frequencies which will disturb subsequent signal processing steps. A benefit of the interpolation process is that the lowpass filter may be designed to operate at the input sample rate, rather than the faster output sample rate, by using a FIR filter structure,

and by noting that the inputs associated with the L-1

inserted samples have zero values.

Sample rate changes using both interpolation and decimation

When the specified SRC factor is not an integer factor, SRC design uses interpolation to increase the sample rate to a rate which is divisible by both the input and final output sample rates. This interpolation is then followed by decimation to achieve the specified output rate. Note that the output sample rate may be faster or slower than the original input rate. In cases where both interpolation and decimation are performed in tandem it is possible to combine the anti-imaging filter of the interpolator and the anti-aliasing filter of the decimator into a single filter which satisfies both requirements. The filters which run at the low data rate are actually implemented as a particular structure known as a polyphase filter, which will be discussed shortly.

1.2 Decimation

If the sampling rate is decreased by a factor M, in order to

avoid aliasing, a lowpass filter is needed with the specific restrictions that the ratio of the half sample frequency to

the passband frequency must be less than or equal to M.

Let x(m) be the input signal, h(k), 0 <= k < K, be the coefficients of a given lowpass filter and z(m) be the

output signal before decimating by a factor M, then:

−

kmxkh

z(m) = (1)

Now let the output signal after the decimator be y(r) =

z(rM) where the sampling rate is reduced by a factor M.

Clearly, y(r) = z(rM) if the output signal is decimated by a factor M.

y(r) =

Looking carefully at this equation, one can see that the filter is in effect using the downsampled signal. Thus the operations of downsampling and lowpass filtering have

∑

)()(

−

krMxkh

)()(

(2)

been embedded in such a way that the lowpass filter is operating at the reduced data rate and the average number of computations to generate one output sample is reduced

by M.

1.3 Interpolation

Given an incoming sample rate of F

factor of L, then the resulting output sampling frequency is

= L*F

out

output signal is required such that the cutoff frequency is

/2.

Let x(n) be the original input sequence, v(n) the sequence

with L-1 zeros inserted, y(n) the output sequence of the

lowpass filter and let h(0), ..., h(K-1) be the coefficients of the lowpass filter, then:

y(n) = (3)

However, v(n-k) = 0 unless n-k is a multiple of L, since L1 zeros were inserted in the sequence x(n) to get v(n).

Again let x(n) be the input signals, and h(k) be the filter coefficients. Then the output signal y(r) has a simple formula:

y(r) = (4)

The average number of computations during one sampling time is reduced by L, the interpolation factor.

. To prevent imaging, a lowpass filter on the

−

knvkh

∑

−

)()(

nxLnrh

)()(

and an interpolation

1.4 Sample Rate Conversion by Rational Factor L/M

To perform sample rate conversion by a rational factor

L/M, the incoming signal is first interpolated by a factor M.

The interpolation must be performed first to preserve the spectral content of the signal. Graphically, this process can be represented by the following diagram:

x(n)

Sampling rate

Figure 1. Block Diagram of a Rational SRC

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 2 of 26

Up Sampler

by L

Anti-imaging

lowpass filter

sample rate LFx

Anti-Aliasing

lowpass filter

Down

Sampler

by M

y(m)

Sampling rate

(L/M)Fx

The anti-aliasing and anti-imaging lowpass filters can be combined into a single low-pass filter.

1.5 Polyphase Filters

Polyphase filters are used to implement multirate filters. The polyphase filters for interpolation-only and decimation-only filters have a simpler structure than the polyphase filter used between an interpolator and a decimator.

1.5.1 Interpolator-Only Polyphase Filters

The computational efficiency of the Interpolator filter structure can also be achieved by reducing the large FIR filter of length K into a set of smaller filters. These smaller filters will have a length N = K/L, where K is selected to be a multiple of L. Since the interpolation process inserts L

- 1 zeros between successive values of x(n), only N out of the K input values stored in the FIR filter at any one time are nonzero. At one time instant, these nonzero values coincide and are multiplied by the filter coefficients h(0),h(L), h(2L),...,h(K - L). In the following instant, the nonzero values of the input sequence coincide and are multiplied by the filter coefficients h(1), h(L + 1), h(2L +

1),...h(K - L + 1), and so on. This observation leads us to define a set of smaller filters called polyphase filters, with unit sample responses:

pk (n)=h(k+nL) k = 0,1,...,L – 1 n = 0,1,...,N – 1 (5) where N = K/L is an integer.

Additional insight can be gained about the characteristics of the set of polyphase subfilters by noting that p obtained from h(n) by decimation with a factor L. Consequently, if the original filter frequency response H(w) is flat over the range each of the polyphase subfilters will possess a relatively flat response over the range (i.e. the polyphase subfilters are basically allpass filters and differ primarily in their phase characteristics). This explains the reason for the term “polyphase” in describing these filters. The polyphase filter can also be viewed as a set of L subfilters connected to a common delay line. Ideally, the kth subfilter will generate a forward time shift of (k/L)F subfilter. Therefore, if the zeroth filter generates zero delay, the frequency response of the kth subfilter is:

pk(w) =e

in, for k = 0, 1 2,..., L - 1, relative to the zeroth

(6)

(n) is

1.5.2 Decimator-Only Polyphase Filters

By transposing the interpolator structure we obtain a commutator structure for a decimator that is based on the parallel bank of polyphase filters. The unit sample responses of the polyphase filter are now defined as:

pk(n) = h(k+nM) k = 0,1,...,M - 1

n = 0,1,...,N – 1 (7)

where N = K/M is an integer when K is selected to be a multiple of M. The commutator rotates in a counterclockwise direction starting with filter p

1.5.3 Simultaneous Interpolator and Decimator Polyphase Filter

A Polyphase filter which is used to perform lowpass filtering between an interpolator and decimator function is more complicated than the structures previously discussed for either the Decimator-Only or Interpolator-Only phases. In the Interpolator-Only case, one input leads to several outputs, and in the Decimator-Only case, many inputs lead to a single output. Thus, there is a relatively simple relationship between the polyphase subfilters and h(n), the lowpass filter coefficients. An interpolator of L samples followed by a decimator of M samples means that L input values must lead to M output values.

y(m) is the output of the polyphase filter

g(n,m) is the polyphase filter coefficients

h(n) is the lowpass filter used for both antiimaging of the interpolator and anti-aliasing of the decimator

(n).

[x] denotes the largest integer in x (8)

g(n,m) = h(nL +mM - [

]* l)

n=0,…,N-1 and m=0,…,L-1 (9)

y(m) =

−=10N

∑

g(n,m-[

] L) x([•

]-n)

where K is the filter length of h(n) and L|K with N = K/L, m=0,…,L-1 (10)

In a multistage implementation, this type of polyphase filter is used between the interpolator and the decimator stage. All other stages are either simple decimation or

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 3 of 26

interpolation stages. The polyphase filters are exactly those described in Section1.5.1 and Section1.5.2. An excellent discussion of this topic is available in Chapter 10 of

Digital Signal Processing by Proakis and Manolakis.

1.6 Polyphase, Multistage Filter Design

Given an input sampling frequency F output sampling frequency F frequency F of both F

is the Least Common Multiplier (or LCM)

min

and F

. The decimator of the sample rate

out

conversion is defined as D = F is defined as U = F

/ F

min

(integer), then the smallest

out

min

. The number of primes in the

decimator is the maximum number of stages in the decimation structure design. If the decimator is 24= 2 * 2 * 2 * 3, then the maximum number of stages is 4. Likewise, the number of primes in the interpolator is the maximum number of stages in the interpolation structure design. Thus it is possible to have a different optimum multirate structure for a multistage decimation structure as opposed to a multistage interpolation structure.

If you choose M = D, L = U, then you are in a design of a SRC system (U/D), but you can also choose M = RD, and L = RU to get an equivalent system (RU/RM) for any positive integer R. The user can choose R = 1, 2, 4,...

A design of a SRC requires the selection of a structure:

decimation or interpolation, over-sample rate R = 1, 2,

4,…, number of stages, a factor for each stage, and a lowpass filter for each stage. The product of all the stage factors should be equal to the decimator if a decimation structure is selected or interpolator if an interpolation

structure is selected, times the over-sample rate R.

Momentum Data Systems (MDS) has developed a program to create and optimize SRC structures and generate

coefficients: Advanced QED Series Sample Rate

Conversion System (Windows 95/NT Version only) Version

www.mds.com). This program has two methods for

2.2. (

best design of decimation and interpolation structures: minimizing the sum of filter lengths, and minimizing the number of computations of the signal filtering. The number of computations is calculated as follows:

If U1, U2 and U3 are up-sample factors for a 3-stage interpolation structure, and L1, L2 and L3 are the filter

lengths for 3-stages respectively, then the number of computations is

L1 + L2 * U1 + L3 * U1 * U2, or equivalent L3/U3 + L2/(U2*U3) + L1/(U1*U2*U3)

If D1, D2 and D3 are down-sample factors for a 3-stage

decimation structure, then the number of computations is

(integer) and an

/ F

and the interpolator

out

L3 + L2 * D3 + L1 * D3 * D2, or equivalent L1/D1 + L2/(D1*D2) + L3/(D1*D2*D3)

This design problem is not a single-objective optimization problem. The number of computations, the number of filter taps and the complexity of the multi-structure enter in the calculations. The problem becomes particularly complicated if the number of stages is greater than 3.

This EE-Note used the QED Series Sample Rate Conversion System to determine the optimum SRC

structures and all coefficients.

1.7 SRC Code Overview

The work described in this EE-Note was based on the

principles discussed in Section 1.1 through Section 1.6.

From this, a polyphase multistage SRC was implemented on the ADSP-BF535 Blackfin® Processor.

A zip file (SRC.zip) containing the VisualDSP++™ 3.1

projects discussed here can be obtained from Analog Devices ( imported into later versions of VisualDSP++. The Default

C Linker Description File (*.ldf) for the latest version of

VisualDSP++ should be used to recompile/relink these

projects. Make sure BUFIN is defined in the assembly options (see Section 1.7.3). The SRC and main program C shell (SRC.c) were developed using the ADSP-BF535 EZ-

KIT Lite™ Evaluation Platform. The C shell contains function calls and routines to initialize the state of the ADSP-BF535 as well as the SRC. Since this code does not use any DMA capabilities or peripherals, this ‘core’ code should port directly to next generation ADSP-BF5xx Blackfin Processors. All code for this project is listed in

the Appendix.

The following were the design objectives used in developing the SRC functions:

• The optimized assembly routines are to be C callable

• All input and output data should be 16 bits.

• All intermediate calculations should be 32-bit double-

• All filter coefficients should be 32-bit.

• All filters were designed for audio applications with

0.2dB passband ripple 58dB stopband ripple

• The MIPS budget should be ≤ 2 MIPS for all SRC

www.analog.com). These files can be easily

(See src_init.asm and src_flt.asm in the Appendix).

precision (maintaining 31.5 bits of precision per MAC).

these criteria:

examples.

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 4 of 26

The program assumes input data comes from a 16-bit

buffer (initialized as ‘x’ in the shell). This data is copied into a 32-bit buffer ‘in1’ within src_flt.asm. At the end of src_flt.asm, the last 32-bit buffer ‘inx’ (where ‘x’ is the last stage) is copied into a 16-bit buffer (‘y’ in the shell). These

16-bit input/output buffers can be eliminated to conserve data space. In this case, you will need to undefine

‘BUFIN’ and preload 'in1' with 32-bit data and then use the 32-bit output data from ‘inx’.

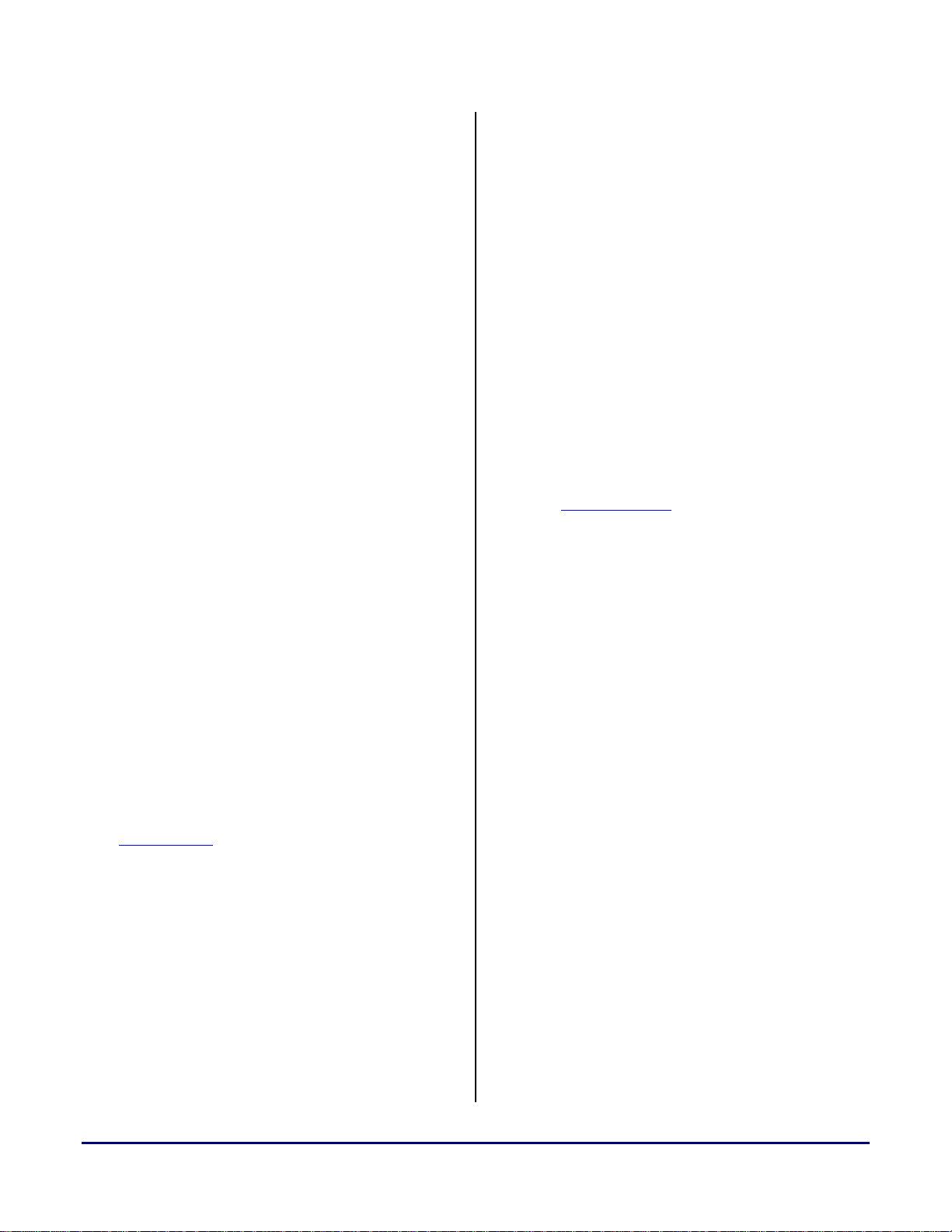
The filters were designed to convert between selected standard audio sample rates (Hz): 48000, 44100, 32000,

22050, 16000, 11025, and 8000. See Figure 1 for the audio SRC matrix. Note that an ‘x’ in the matrix denotes that the SRC filter was designed and is included in SRC.zip. If you

have the SRC program from MDS (or similar) you can

generate coefficients for any SRC. See Section 1.7.2

below.

Figure 3. ‘x’ Input Data for 44.1KHz sampling of a 250Hz sine wave.

Figure 2. Audio SRC Matrix

The #2 workspace in this project has all the necessary plots of the input/output stages as well as the intermediate buffers. You can look at the data in the time domain or apply the VisualDSP++ built-in FFT plotting function to analyze the frequency domain. Load

‘plots_xxxxtoxxxx.vdw’ for a particular SRC. A ‘SINE_xxxxx_16bit_1024.dat’ input file was generated

to test every SRC. This is a 16-bit, 1024-sample, 1KHz or 250Hz sine wave at the input sample rate. These input files were generated using MATLAB® scripts (see

‘gen_sine_wave_comma_16.m’). It's easy to verify proper

SRC functionality by counting samples in one period at

both the input rate (in the ‘x’ plot) and the output rate (in the ‘y’ plot) in workspace #2. See Figure 2 and Figure 3.

Figure 4. 'y' Output Data for 48KHz SRC of a 250Hz sine wave

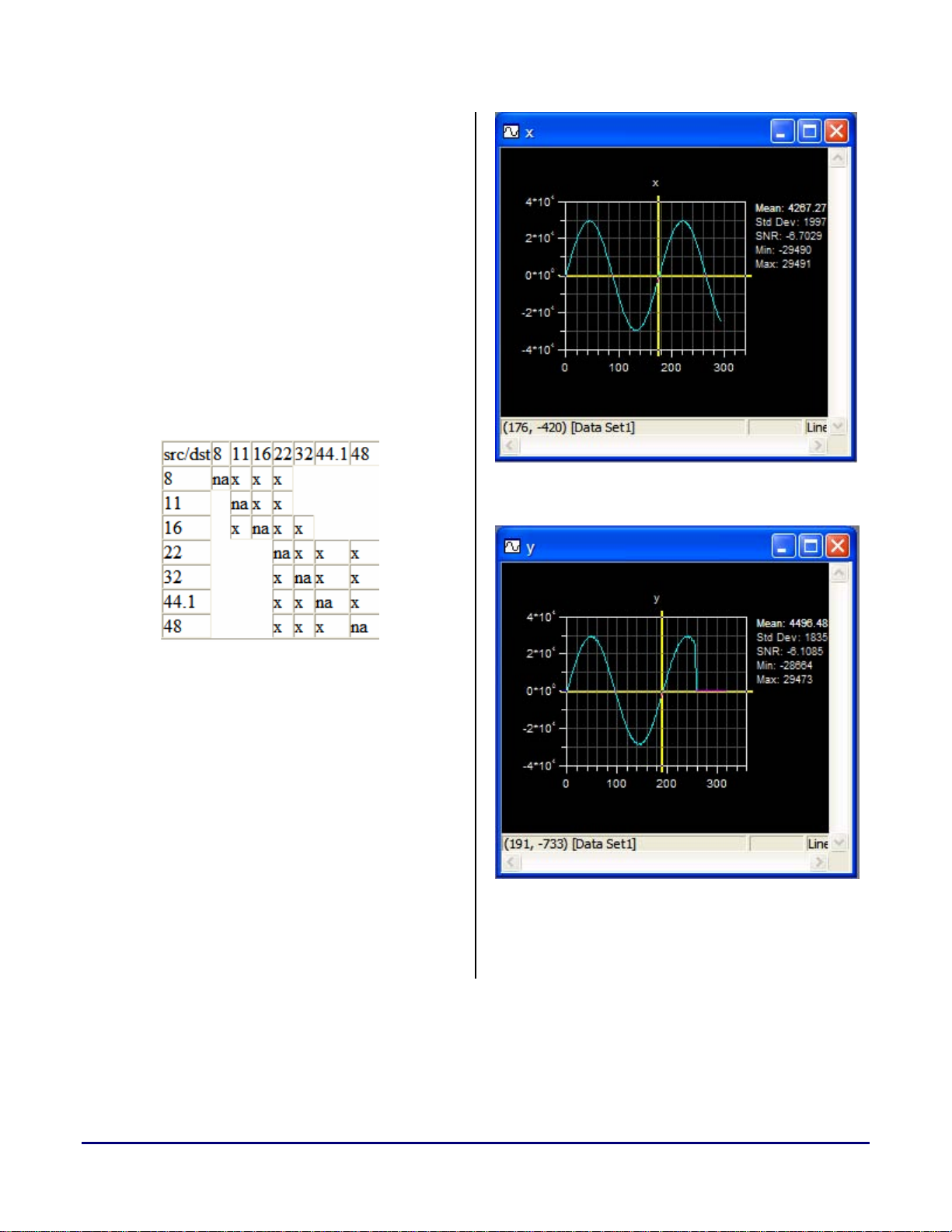
The built-in FFT plotting functions were also used to

analyze input and output data. See Figure 4 and Figure 5.

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 5 of 26

Figure 5. FFT of 'x' Input Data at 250Hz

Figure 6. FFT of 'y' Output Data at 250Hz

1.7.1 Input/Output Data Sizes and the GCD

The size of NINPS and NOUTS can be modified in each ‘src_xxxxtoxxxx.h’ file (see example of src_441to48.h in the Appendix). This will allow the user to vary the size of

the input/output buffers according to system block processing needs. It was envisioned that the end application would be operating on blocks of audio samples. Note that the smallest block size can be no less than the

LCM discussed in Section 1.6. However, an integer

multiple of the LCM can be applied to increase the

processed block size. The user can increase or decrease the integer multiple of the LCM (or Greatest Common

Denominator, GCD, in Table 2) by changing the buffer sizes NINPS and NOUTS. These two numbers must be at

least half of the greatest filter coefficient count times the

INTPx to ensure valid output data. Table 1 was generated

from a simple C program:

GCD=48000, Original=48000/48000, NEW=1/1 GCD=300, Original=48000/44100, NEW=160/147 GCD=16000, Original=48000/32000, NEW=3/2 GCD=150, Original=48000/22050, NEW=320/147 GCD=16000, Original=48000/16000, NEW=3/1 GCD=75, Original=48000/11025, NEW=640/147 GCD=8000, Original=48000/8000, NEW=6/1 GCD=300, Original=44100/48000, NEW=147/160 GCD=44100, Original=44100/44100, NEW=1/1 GCD=100, Original=44100/32000, NEW=441/320 GCD=22050, Original=44100/22050, NEW=2/1 GCD=100, Original=44100/16000, NEW=441/160 GCD=11025, Original=44100/11025, NEW=4/1 GCD=100, Original=44100/8000, NEW=441/80 GCD=16000, Original=32000/48000, NEW=2/3 GCD=100, Original=32000/44100, NEW=320/441 GCD=32000, Original=32000/32000, NEW=1/1 GCD=50, Original=32000/22050, NEW=640/441 GCD=16000, Original=32000/16000, NEW=2/1 GCD=25, Original=32000/11025, NEW=1280/441 GCD=8000, Original=32000/8000, NEW=4/1 GCD=150, Original=22050/48000, NEW=147/320 GCD=22050, Original=22050/44100, NEW=1/2 GCD=50, Original=22050/32000, NEW=441/640 GCD=22050, Original=22050/22050, NEW=1/1 GCD=50, Original=22050/16000, NEW=441/320 GCD=11025, Original=22050/11025, NEW=2/1 GCD=50, Original=22050/8000, NEW=441/160 GCD=16000, Original=16000/48000, NEW=1/3 GCD=100, Original=16000/44100, NEW=160/441 GCD=16000, Original=16000/32000, NEW=1/2 GCD=50, Original=16000/22050, NEW=320/441 GCD=16000, Original=16000/16000, NEW=1/1 GCD=25, Original=16000/11025, NEW=640/441 GCD=8000, Original=16000/8000, NEW=2/1 GCD=75, Original=11025/48000, NEW=147/640 GCD=11025, Original=11025/44100, NEW=1/4 GCD=25, Original=11025/32000, NEW=441/1280 GCD=11025, Original=11025/22050, NEW=1/2 GCD=25, Original=11025/16000, NEW=441/640 GCD=11025, Original=11025/11025, NEW=1/1 GCD=25, Original=11025/8000, NEW=441/320 GCD=8000, Original=8000/48000, NEW=1/6 GCD=100, Original=8000/44100, NEW=80/441 GCD=8000, Original=8000/32000, NEW=1/4 GCD=50, Original=8000/22050, NEW=160/441 GCD=8000, Original=8000/16000, NEW=1/2 GCD=25, Original=8000/11025, NEW=320/441 GCD=8000, Original=8000/8000, NEW=1/1

Table 1. Greatest Common Denominator for Audio SRC

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 6 of 26

1.7.2 Coefficient Generation and Formatting

Assuming a program that is similar to the MDS tool is used, some data formatting must be performed. The following must be done to convert the raw decimal filter

coefficients. With MDS, a *.dsp file is produced. Table 2 is an example of the MDS data format for the *.dsp file.

This file must be properly formatted as a 32-bit

hexadecimal VisualDSP++ input data file (*.dat). This is

then read (by VisualDSP++) into the corresponding variable at initialization:

a. Use Microsoft Excel to import the *.dsp file (space

delimited). Select the "D" column and erase everything but the decimal filter coefficients. Save the file as a

‘Formatted Text (Space Delimited)(*.prn)’ file.

/* External References */ .external src_init; .external src_flt;

#define STAGE 3 /* Number of stages */ #define INTP0 160 /* Interpolation factor */ #define DOWN0 147 /* Decimation factor */

/* ------------------------------------------------------------ */

/* parameters for each stage */ #define INTP1 2 #define DOWN1 1 #define LENG1 223 #define PLEN1 112 #define MLEN1 224 #define SHFT1 0 #define NINP1 147 #define SZIN1 512

#define INTP2 5 #define DOWN 2 1 #define LENG2 27 #define PLEN2 6 #define MLEN2 30 #define SHFT2 0 #define NINP2 94 #define SZIN2 512

#define INTP3 16 #define DOWN3 147 #define LENG3 49 #define PLEN3 4 #define MLEN3 64 #define SHFT3 0 #define NINP3 1470 #define SZIN3 2048

#define NINP4 160 #define SZIN4 256

/* ------------------------------------------------------------------------ */

.VAR/DM flt1[MLEN1]; .INIT flt1: 0xffc8, /* -1.72471041e-003 cf 000 pp 000 ft 1 */ 0xfffe, /* -8.01035724e-005 cf 002 pp 000 ft 1 */ 0x000f, /* 4.65568547e-004 cf 004 pp 000 ft 1 */

0xfffa, /* -2.00361260e-004 cf 006 pp 000 ft 1 */ 0x000c, /* 3.90279025e-004 cf 008 pp 000 ft 1 */ 0xfff2, /* -4.43292360e-004 cf 010 pp 000 ft 1 */ 0x0013, /* 6.04802800e-004 cf 012 pp 000 ft 1 */ 0xffe8, /* -7.54936936e-004 cf 014 pp 000 ft 1 */ 0x001e, /* 9.43581218e-004 cf 016 pp 000 ft 1 */ ., . . . ., . . . ., . . .

Listing 1. Coefficient Format from MDS

b. Use the included MATLAB® script ‘dec_file_to_hex_file_converter.m’. This script will read in decimal

(exponential) data from the *.prn file and convert to a 32-bit Hexadecimal format (*.dat file) suitable to be read

by VisualDSP++ within a data initialization section. This MATLAB® script can be easily modified for other formats.

1.7.3 BUFIN Define

When ‘BUFIN’ is undefined (under VisualDSP++: PROJECT OPTIONS / ASSEMBLER / ADDITIONAL OPTIONS: ‘-D BUFIN’), the SRC program assumes that buffer ‘in1’ is preloaded with 32-bit input data AFTER the src_init is accomplished (buffer zeroing). This requires that

the shell program preload ‘in1’ from a 32-bit source. Define ‘BUFIN’ to include the 16-bit buffer transfer code within src_flt.asm. ‘x’ and ‘y’ 16-bit input buffers are not

necessary for a final application but they do allow for easier data manipulation for test purposes.

1.7.4 Zeroing Filter Delays

To "zero" out filter delays, use the following equations as offsets to first valid output data:

1st Offset = (LENG1-1)/(2*DOWN1) 2nd Offset = INTP2/DOWN2*1st Offset + (LENG2-1)/(2*DOWN2) 3rd Offset = INTP3/DOWN3*2nd Offset + (LENG3-1)/(2*DOWN3)

See the constants generated in the 'src_xxxxtoxxxx.h’ files. DOFSx is actually the offset from the end of the buffer.

Therefore it is the number of valid output data samples. This will determine how often this routine needs to be executed in a block processed system. Be careful with this number. The preprocessor in VisualDSP++ will not generate fractional constants. Therefore, depending on the

math here, DOFSx could have an error of ±1 sample. For a particular SRC, check the first sample in ‘y’ and adjust the DOFSx accordingly.

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 7 of 26

1.7.5 Reducing Intermediate Buffers

One idea to reduce the number of intermediate buffers is to

implement a ‘zero_buf’ function (not included) that would

re-zero the buffers between filter sections. This would reduce the number of intermediate buffers to two at the expense of more MIPS to accomplish the SRC. However, the MIPS increase would be negligible and is on the order of the size of the buffer times the number of times it is zeroed. These two intermediate buffers should be sized to the maximum needed for any SRC.

1.7.6 Restrictions

If there is a large interpolation constant INTPx, this

severely reduces the number of valid data samples in the final output buffer. For example, in the 44.1K to 48K SRC, there is an interpolation constant of 16 in the 3rd stage. If we only use L1 data sections (max = 4096 bytes) we only get 111 valid data samples in the final output buffer. However, if we can use L2 (like what is available in the ADSP-BF535) and make this intermediate buffer as large as 4096 words (16K bytes), we can get a relatively large number of valid output data samples. Depending on interpolation constants and the need to run out of single cycle L1 memory, the limiting factor appears to be the L1 section size. We can maximize all the filters based on this L1 section size (4096 bytes or 1024 32-bit words) or assume we can use L2 (internal or external) and make the intermediate buffers larger. In the latter case, the number of valid output data samples greatly increases.

1.7.7 Unresolved Issues

The following SRCs produced corrupted output data when using a 3-stage interpolator structure:

passband ripple = 0.0001 and a stopband ripple = 98dB. This provided a overall SNR of 90dB through all 3 stages

of the filter. This was tested using Cooledit 2000 software.

If a lesser system SNR is desirable (50-70dB), a 32-bit implementation will provide a SNR that is close to the stopband attenuation. For higher system SNR's (above 90dB), much higher stopband attenuations are required.

Conclusions

The code and filters in this EE-Note were generated specifically with audio SRC in mind. Notwithstanding, by generating new filter input files with tighter passband and stopband ripple, this code could be used unaltered for many different applications. Keep in mind that we gained computational efficiency by eliminating the LxL multiply and thus only retaining 31.5 bits of precision for each Multiply And Accumulate (MAC). This amount of precision is more than enough for most applications.

Notice in src_flt.asm that the inner MAC loops are only 2

cycles, enabling double precision math with very little overhead. This “low cycle” double precision capability of Blackfin™ is one of the great advantages of this architecture over competing single MAC architectures.

Placement of data and code sections (i.e. L1, internal or external L2) is up to the user. However, coefficients and data should be placed in separate banks to avoid stalls (only applies to L1). Also, whether cache or SRAM is used will greatly impact the overall cycle counts. Since there are many filters required for all the various audio SRC combinations, it was assumed that coefficients would be placed in a larger external L2 SRAM or SDRAM. These coefficients could either be cached internally or brought into L1/L2 via DMA concurrent to block processing.

11025to16, 16to2204, and 8to11025

Therefore, a 2-stage filter decimator structure was used instead and produced valid results. It appears that the MDS filter generator produced corrupted 3rd stage outputs for all SRCs that up-converted between two similar rates. The MDS program chose by default a 3-stage interpolator structure in each of these cases.

1.7.8 Case Study of Total SNR

Two common SRC changes are 44.1KHz to 48KHz and 48KHz to 44.1KHz. Instead of using the stopband and passband ripples above, a filter was generated with

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 8 of 26

Recommendations for Further Development

The code developed in this EE-Note can be applied to any application requiring SRC. For example, many video applications require the ability to scale images to change the video size (D1 to CIF, etc.). This Polyphase multistage SRC approach could be modified to work on byte-wide single precision video data. The basic structure of this code would not require many alterations. Instead of working on one time-domain double-precision data sample per cycle, the SRC would be modified to operate on two byte-wide frequency-domain data samples per cycle.

Finally, the code has not been completely optimized. Improvements can be made to reduce overall cycles particularly the elimination of pipeline stalls.

Appendix

SRC.c

/**************************************************************************** * File: SRC.c * Date Started: Sept 26 2002 * Created: Jeff Sondermeyer ****************************************************************************/

File Name: SRC.c

Date Modified: 3/12/2004 Jeff Sondermeyer Rev 0.3

Purpose: The Sample Rate Converter (SRC) and Main Program Shell Developed using the ADSP-21535 EZ-KIT Lite Evaluation Platform. This C shell contains function calls and routines to initialize the state of the 21535 as well as the SRC. This program assumes input data comes from a 16-bit buffer (initialized as 'x' in this shell). This data is copied into a 32-bit buffer 'in1' within src_flt.asm. At the end of src_flt.asm, the last 32-bit buffer 'inx' (where 'x' is the last stage) is copied into a 16-bit buffer ('y' in this shell). These 16-bit input/output buffers can be eliminated to conserve data space. In this case, you will need to undefine 'BUFIN' and preload 'in1' with 32-bit data and then use the 32-bit output data from 'inx'. The converter was designed to convert between any of the following rates: 48000, 44100, 32000, 22050, 16000, 11025, and 8000. If you have the SRC program from Momentum Data Systems you can generate coefficients for any SRC. Follow #3 below. The #2 workspace in this project has all the necessary plots of the input/output stages as well in the intermediate buffers. You can look at the data in the time domain or apply the built-in FFT plotting function to analyze the frequency domain. Load "plots_xxxxtoxxxx.vdw" for a particular SRC. I have generated a "SINE_xxxxx_16bit_1024.dat" input file to test every SRC. This is a 16-bit, 1024-sample, 1KHz sine wave at the input sample rate. These were generated using MATLAB (see 'gen_sine_wave_comma_16.m'). It's easy to verify proper conversion by counting samples in one period at both the input rate (in the 'x' plot) and the output rate (in the 'y' plot) in workspace #2. Notes:

1. You can modify the size of NINPS and NOUTS in each 'src_xxxxtoxxxx.h' file. However, it MUST be the same multiple of the GCD.

2. Buffer sizes, NINPS and NOUTS must be at least half of the filter coefficient sizes times the INTPx value to ensure valid output data.

3. Do the following to convert the decimal filter coefficients from Momentum Data Systems SRC *.dsp file to properly format this data as 32-bit Hexidecimal value. This is then read into the corresponding variable at initialization: a. Use Excel to import the *.dsp file (space delimited). Select the "D" column and erase everything else. Save the file as a "Formatted Text (Space Delimited)(*.prn)" file. b. Use the MATLATB program "dec_file_to_hex_file_converter.m". This MATLAB program will read in decimal (exponential) data from a file (*.prn) and convert to a 32-bit Hexidecimal format (*.dat file) suitable to be read by VisualDSP within a data initialization section.

4. When 'BUFIN' is undefined, the program assumes that 'in1' is preloaded with 32-bit input data AFTER the src_init is accomplished (buffer zeroing). This requires that the shell program preload 'in1' from a 32-bit source. Define 'BUFIN' to include the 16-bit buffer transfer code within src_flt.asm. x and y 16-bit buffers are nice but is just another chunk of memory that is necessary.

5. To "zero" out filter delays, use the following equations as offsets to first valid data: 1st Offset = (LENG1-1)/(2*DOWN1) 2nd Offset = INTP2/DOWN2*1st Offset + (LENG2-1)/(2*DOWN2) 3rd Offset = INTP3/DOWN3*2nd Offset + (LENG3-1)/(2*DOWN3) See the constants generated in the 'src_xxxxtoxxxx.h' files.

6. DOFSx (in src_xxxxtoxxxx.h) is the offset and also is the number of valid output data samples. This will allow you to figure how often this routine needs to be executed in a block-processed system. Be careful with this number. The preprocessor in VDSP will not generate fractional constants. Therefore, depending on the math here, DOFSx could have an error of +/-1. For a particular SRC, check the first sample in 'y' and adjust the DOFSx accordingly.

7. One idea of reducing the number of intermediate buffers is to call a 'zero_buf' function that would rezero the buffers between filter sections. This would reduce the number of intermediate buffers to two at the expense of more MIPs. However, the MIPs increase would be negligable and is on the order of the size of the buffer. These two intermediate buffers should be sized to the maximum needed for any SRC.

8. If there is a big interpolation constant, this severely reduces the number of valid data samples in the final output buffer. For example, in the 44.1K to 48K case, there is an interpolation constant of 16 in the 3rd stage. If we only use L1 data sections (max = 4096 bytes) we only get 111 valid data samples in the final output buffer. However, if I use L2 and make this intermediate buffer as large as

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 9 of 26

4096 words (16K bytes), I can get a relatively large number of valid output data samples. The point here is that.. depending on interpolation constants, the limiting factor appears to be the L1 section size. I can maximize all my filters based on this L1 section size (4096 bytes or 1024 words) ...OR.. assume someone can use L2 and make the intermediate buffers larger. In the later case, the number of VALID output data samples greatly increases.

9. The half band code was not implemented. Therefore, the HALFB define is not used.

10. 11025to16, 16to2204, and 8to11025 produced corrupted data with 3-stage filters. Had to use 2stages. MDS filter generator produces corrupted 3rd stage output for close sample rate conversions that required up sampling??? Not sure why.

11. The latest revision of the code was debugged on a Momentum Systems Hawk PCI board. All FileIO was done over the PCI bus. Several things need to change in this code to work with the Hawk board: a. Define ‘HAWK’ b. Add idle.c and the basiccrt.s file for the Hawk board to the project.

/* ------------------------------------------------------------------------ */ #include "fract_math.h" #include <defBF535.h> #include "src_inc.h" #include "src_441to48.h" #include <stdlib.h> #include <stdio.h> //#include <device.h>

/* ------------------------------------------------------------------------ */

// 16-bit input/output buffers

static segment("L1_data_b") short x[NINPS];

static segment("L1_data_b") short y[NOUTS];

FILE *inFile,*outFile;

// 32-bit intermediate buffers

segment("L1_data_a") int in1[SZIN1];

segment("L1_data_a") int in2[SZIN2];

#if STAGE>=2 segment("L1_data_a") int in3[SZIN3]; #endif

#if STAGE==3 segment("L1_data_a") int in4[SZIN4]; #endif

// Filter Coefficients

static segment("L1_data_b") int filter_h1[MLEN1] = { #include "441to48_32bit_flt1.dat" };

#if STAGE>=2 static segment("L1_data_b") int filter_h2[MLEN2] = {

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 10 of 26

#include "441to48_32bit_flt2.dat" }; #endif

#if STAGE==3 static segment("L1_data_b") int filter_h3[MLEN3] = { #include "441to48_32bit_flt3.dat" }; #endif

/////////////////////////////////////////////////////////////////// // This line enables the PCI as the default device for file I/O //#pragma retain_name //extern int __default_io_device = PCI_IO; //extern int __default_io_device = FILEIO; ///////////////////////////////////////////////////////////////////

/* ------------------------------------------------------------------------ */

static void init_first_stage(STAGE_HANDLE *S) {

FIRST_STAGE_ENTRY *V;

V = S->V; V->in_s = &in1[0]; V->in_z = SZIN1; V->out_s = &in2[0]; V->out_z = SZIN2; V->h = &filter_h1[0]; V->plen = PLEN1-1; V->up = INTP1; V->dn = DOWN1; V->nis = NINP1; V->nos = NINP2; V->nshft = SHFT1; V->in_c = &in1[0]; V->out_c = &in2[0];

}

#if STAGE>=2 static void init_sec_stage(STAGE_HANDLE *S) {

SEC_STAGE_ENTRY *M;

M = S->M; M->in_s = &in2[0]; M->in_z = SZIN2; M->out_s = &in3[0]; M->out_z = SZIN3; M->h = &filter_h2[0]; M->plen = PLEN2-1; M->up = INTP2; M->dn = DOWN2; M->nis = NINP2; M->nos = NINP3; M->nshft = SHFT2; M->in_c = &in2[0]; M->out_c = &in3[0]; } #endif

#if STAGE==3

static void init_last_stage(STAGE_HANDLE *S) {

THIRD_STAGE_ENTRY *L;

L = S->L; L->in_s = &in3[0]; L->in_z = SZIN3;

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 11 of 26

L->out_s = &in4[0]; L->out_z = SZIN4; L->h = &filter_h3[0]; L->plen = PLEN3-1; L->up = INTP3; L->dn = DOWN3; L->nis = NINP3; L->nos = NINP4; L->nshft = SHFT3; L->in_c = &in3[0]; L->out_c = &in4[0]; } #endif

/* ------------------------------------------------------------------------ */

static segment("L1_data_b") FIRST_STAGE_ENTRY vst; static segment("L1_data_b") SEC_STAGE_ENTRY mst; static segment("L1_data_b") THIRD_STAGE_ENTRY lst; static segment("L1_data_b") STAGE_HANDLE sth; static segment("L1_data_b") FUNDAMENT_DATA_ENTRY vfd;

/* ------------------------------------------------------------------------ */

void init_stage_handle (void) {

STAGE_HANDLE *S; S = &sth; S->V = &vst;

#if STAGE>=2 S->M = &mst; #endif

#if STAGE==3 S->L = &lst; #endif init_first_stage (S);

#if STAGE>=2 init_sec_stage (S); #endif

#if STAGE==3 init_last_stage (S); #endif

}

/* ------------------------------------------------------------------------ */

void init_src (void) {

FUNDAMENT_DATA_ENTRY *F; F = &vfd; F->S = &sth;

F->half_band = HALFB; F->up_stage = NUPST; F->pivot_stage = PVTFL; F->down_stage = NDWNS; F->nstages = STAGE; F->ninputs = NINPS; F->noutputs = NOUTS;

src_init (F);

}

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 12 of 26

/* ------------------------------------------------------------------------ */

Shell test program.. eventually I will use Fread and Fwrite to input/output ASCII files (JWS)

*/ int i,j=0,count,file_status;

int getInput(short *inBuf,int count); int writeOutput(short *outBuf,int count);

int main() {

//int i,j=0,count,file_status;

/* initialize filter */

inFile = fopen("C:\\DSP\\sin_1khz_44khz.dat","rb"); outFile = fopen("C:\\DSP\\out_1khz_44to48.dat","wb");

init_stage_handle(); init_src();

count = getInput(x,NINPS);

while(count==NINPS) { /* filter samples */ j=j+1; #if STAGE==3 src_flt (x, y, DOFS3, &vfd); #endif

#if STAGE==1 src_flt (x, y, DOFS1, &vfd); #endif

#if STAGE==2 src_flt (x, y, DOFS2, &vfd); #endif

count = writeOutput(y,NOUTS);

count = getInput(x,NINPS); }

fclose(inFile); fclose(outFile);

}

int getInput(short *inBuf,int count) {

int wordsRead=0;

wordsRead = fread(inBuf,sizeof(short),count,inFile);

return wordsRead;

}

int writeOutput(short *outBuf,int count) {

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 13 of 26

int wordsRead=0;

wordsRead = fwrite(outBuf,sizeof(short),count,outFile);

return wordsRead;

}

Listing 2. SRC.c

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 14 of 26

Src_flt.asm

/* File: src_flt.asm Version 0.1

fundemental structure order:

1. stage data handle

2. half band flag (0,1, or 2)

3. number of up stages

4. pivot flag (0 or 1)

5. number of down stages

6. number of stages (total)

7. number of input samples per block

8. number of output samples per block

P0 -> fundamental structure P1 -> input samples P2 -> output samples P3 -> memory storage and retreival P4 = temporary pointer P5 = loop counter

R0 = Loop counters R1 = temporary storage R2 = Loop counters R3 = Shift count R4 = inner loop calculations R5 = inner loop calculations R6 = temporary storage R7 = temporary storage

I0 = dedicated to input buffer 'inx' I1 = general use...reading 'inputData' plus others I2 = general use...reading 'inx' for output data I3 = general use...

Input Data Structure (VAR_SIZE words) AIS: address of input signal (circular), updated after return, SIS: circular size of AIS, AOS: address of output signal (circular), updated after return, SOS: circular size of AOS, AFA: address of filter array, LEN: poly-phase filter length, UPR: up sample rate >= 2, DNR: down sample rate = 1 is assumed NIS: number of input signals NOS: number of output signals SHF: number of shift counter, 0 or 1

.SECTION L1_data_a; .align 4;

.byte4 pt_fundst; // pointer to fundamental structure .byte4 pt2_fundst; // pointer to fundamental structure .byte4 st_handle; // pointer to stage data handle .byte2 inputs; // number of inputs .byte2 outputs; // number of outputs .byte2 diff_offset; // Offset difference

.GLOBAL _src_flt;

.SECTION program;

_src_flt:

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 15 of 26

[--SP]=(R7:4,P5:3); // Push R7... P1 = R0; // Address of input data P2 = R1; // Address of output data P0 = [SP+40]; // Address of fundemental structure

p3.l = diff_offset; p3.h = diff_offset; w[p3] = r2; // save DOFS3 (difference offset to strip filter delay off of final buffer

p3.l = pt_fundst; // p3 -> to fundemental structure p3.h = pt_fundst; [p3] = p0; // save fundemental stage pointer in memory pointed at by p3

#ifdef BUFIN

p5 = 24; // 6*4 = 24 bytes (post increment points to number input samples per block) r6 = [p0++p5]; p4 = r6; // p4 -> stage handle

r6 = [p0++]; // r6 = number of inputs samples per block p3.l = inputs; // p3 -> to number of input samples per block p3.h = inputs; w[p3] = r6; // save number of input samples per block

r6 = [p0++]; // r6 = number of output samples per block p3.l = outputs; // p3 -> to number of output samples per block p3.h = outputs; w[p3] = r6; // save number of output samples per block p0 = [p4++]; // p0 -> fist data structure /******** IPDC comment *******/ // r6 = [p0++]; // r6 -> first input buffer 'inx' // i0 = r6; // i0 -> first input buffer 'inx' // b0 = r6; // b0 -> base of first input circular buffer /******************************/

/*********IPDC addition*******/ p5 = 44; r6 = [p0++p5]; // r6 -> first input buffer 'inx' i0 = r6; // i0 -> first input buffer 'inx' p5=-40; r6 = [p0++p5]; b0 = r6; // b0 -> base of first input circular buffer /******************************/ r6 = [p0++]; r6 = r6 << 2; // double length (4 bytes per word) l0 = r6; // l0 = first input circular buffer size 'SZINx'

go_back:

// p3.l = num_blocks; // p3.h = num_blocks; // r6 = w[p3]; // get number of blocks // r6 += -1; // Decrement number of blocks // w[p3] = r6; // save decremented number of blocks // CC = r6 < 0; // IF CC JUMP RETURN_TO_SENDER; // Return if less than 1 block

p3.l = inputs; // p3 -> to number of input samples per block p3.h = inputs; r7 = w[p3]; p5 = r7; // p5 = number of input samples per block

i1 = p1; // load i1 with address of 'inputData' l1 = 0;

LSETUP(READ_INPUTS_BEGIN, READ_INPUTS_END) LC0 = p5; READ_INPUTS_BEGIN: r6.h = w[i1++]; // read the input buffer 'inputData' r6.l = 0;

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 16 of 26

READ_INPUTS_END: [i0++] = r6; // write input into input buffer 'inx'

// p1 = i1; // save i1 into p1

p3.l = pt_fundst; // p3 -> to fundemental structure p3.h = pt_fundst; r7 = [p3]; p0 = r7; // p0 -> to fundemental structure

#endif

src_core:

r7 = [p0++]; p3.l = st_handle; p3.h = st_handle; [p3] = r7; // store stage data handle

r6 = [p0++]; // r6 = half band flag (move past this for now)

r2 = [p0++]; // r2 = # of up stages p3.l = pt2_fundst; p3.h = pt2_fundst; [p3] = p0; // save pointer to current fundemental structure

CC = r2 <= 0; IF CC JUMP over_upstage; // if upstage = 0, jump over

UPSTAGE_BEGIN:

p3.l = st_handle; p3.h = st_handle; p4 = [p3]; // p4 -> current stage data handle

r7 = [p4++]; p0 = r7; // p0 -> stage data

[p3] = p4; // save pointer to stage data handle

up_src: /******** IPDC comment *******/ // r7 = [p0++]; // r7 -> input signal 'inx' // b3 = r7; // b3 set for circular buffering /******************************/

/*********IPDC addition*******/ p4 = 44; r7 = [p0++p4]; // r7 -> input signal 'inx' p4 = -40; r5 = [p0++p4]; b3 = r5; // b3 set for circular buffering /******************************/

r5 = [p0++]; r5 = r5 << 2; // double the length (4 bytes per word) l3 = r5; // l3 = Size of Input Stage (SIS)

/******** IPDC comment *******/ // r6 = [p0++]; // i2 = r6; // i2 -> output signal 'inx'+1 buffer (output buffer) // b2 = r6; // b2 set for circular buffering

/******************************/

/*********IPDC addition*******/

p4 = 40; r6 = [p0++p4]; i2 = r6; // i2 -> output signal 'inx'+1 buffer (output buffer) p4 = -36; r6 = [p0++p4];

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 17 of 26

b2 = r6; // b2 set for circular buffering /******************************/

r6 = [p0++]; r6 = r6 << 2; // double the output size (4 bytes per word) l2 = r6; // l2 = Size of Output Stage (SOS)

r3 = [p0++]; // r3 -> the filter coefficients r6 = [p0++]; // r6 = poly-phase filter size p3 = r6; // save poly-phase into p3

p4 = 8; // always skip over DNR (2*4bytes) in the up SRC

r4 = [p0++p4]; // r4 = UPR p5 = r4; // p5 = Up Sample Rate (UPR) r0 = [p0++p4]; // r0 = NIS

p4 = -40; // Backup 10 words (10x4bytes) r6 = [p0++p4]; // r6 = number of shifts (always a arithmatic left shift..upshift) m2 = r6; // Save in m2

UP_SRC_OUTER_BEGIN:

i1 = r3; // i1 -> filter coefficients l1 = 0; // linear addressing???

LSETUP(UP_SAMPLE_BEGIN, UP_SAMPLE_END) LC0 = p5;

UP_SAMPLE_BEGIN: i3 = r7; // i3 - > 'in' buffer A1=A0=0 || R6=[I1++] || R5=[I3--]; // r6=filter coef, r5='inx' buffer

LSETUP(POLY_PHASE_BEGIN, POLY_PHASE_END) LC1 = p3;

POLY_PHASE_BEGIN: R4=(A0+=R6.H*R5.H), A1+=R6.H*R5.L (M); POLY_PHASE_END: R1=(A1+=R5.H*R6.L) (M) || R6=[I1++] || R5=[I3--];

// R1=R1>>16; // R4=R4+R1 (S);

r5=m2; // load r5 with number of shifts /******** IPDC comment *******/ // A1 = A1>>16; /******************************/

/*********IPDC addition*******/ A1=A1>>>15; /******************************/ A0+=A1; A0 = ASHIFT A0 BY r5.l; r4 = A0; // high half-word extraction with 16-bit saturation. Rounding cntrl by // RND_MOD. 0 = unbiased rounding = default

// A0 = A0 >>> 1; // R4 = A0;

UP_SAMPLE_END: [i2++] = R4; // save output into 'inx'+1

i3 = r7; // get input back at beginning of 'inx' m3 = 4; i3 += m3; // increment by 1 word (4 bytes) r7 = i3; // update r7 -> 'inx' buffer

UP_SRC_OUTER_END: r0 += -1; // Check number of input samples (NIS) CC = r0 <= 0; IF !CC JUMP UP_SRC_OUTER_BEGIN; // if NIS equal to 0, jump to UP_SRC_OUTER_BEGIN

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 18 of 26

p4 = 8; // 2 words (2*4bytes per word) [p0++p4] = r7; // save the input signal address

r6 = i2; [p0] = r6; // save the output signal address

UPSTAGE_END: r2 += -1; // Check number of stages CC = r2 <= 0; IF !CC JUMP UPSTAGE_BEGIN; // if upstage not equal to 0, jump to UPSTAGE_BEGIN

over_upstage:

p3.l = pt2_fundst; p3.h = pt2_fundst; p0 = [p3]; // p0 -> fundamental structure

r6 = [p0++]; // r6 = pivot flag [p3] = p0; // save fundamental structure

CC = r6 <= 0; IF CC JUMP over_pivotstage; // if pivotstage = 0, jump over

p3.l = st_handle; p3.h = st_handle; p4 = [p3]; // p4 -> current stage data handle

r7 = [p4++]; p0 = r7; // p0 -> stage data

[p3] = p4; // save pointer to stage data handle

pvt_src:

/******** IPDC comment *******/ // r7 = [p0++]; // r7 - > input signal ('in*' buffer) // i3 = r7; // i3 - > input signal // b3 = r7; // b3 set for circular buffering

/******************************/

/*********IPDC addition*******/ p4 = 44; r7 = [p0++p4]; // r7 -> input signal 'inx' i3 = r7; p4 = -40; r5 = [p0++p4]; b3 = r5; /******************************/

r5 = [p0++]; r5 = r5 << 2; // double the length (4 bytes per word) l3 = r5; // l3 = Size of Input Stage (SIS)

/******** IPDC comment *******/ // r6 = [p0++]; // i2 = r6; // i2 -> output signal 'inx'+1 buffer (output buffer) // b2 = r6; // b2 set for circular buffering /******************************/

/*********IPDC addition*******/ p4 = 40; r6 = [p0++p4]; i2 = r6; // i2 -> output signal 'inx'+1 buffer (output buffer) p4 = -36; r6 = [p0++p4]; b2 = r6; // b2 set for circular buffering /******************************/

r6 = [p0++]; r6 = r6 << 2; // double the output size (4 bytes per word)

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 19 of 26

l2 = r6; // l2 = Size of Output Stage (SOS)

r3 = [p0++]; // r3 -> the filter coefficients r6 = [p0++]; // r6 = poly-phase filter size p3 = r6; // save poly-phase into p3

r6 = [p0++]; // r6 = UPR (filter step) r6 = r6 << 2; // post increment must be two bytes m1 = r6; // post increment set to UPR p4 = 8; // always skip over UPR (2*4bytes) in the up SRC

r0 = [p0++p4]; // r0 = DNR r0 = r0 << 2; // four bytes per word r6 = [p0++]; // r6 = NOS p5 = r6; // p5 = Number of Outputs (NOS)

p4 = -40; // Backup 10 words (10x4) // r1.l = w[p0]; // r1.l = Number of shifts (can be left shift=upshift or right shift=downshift) r6 = [p0++p4]; m2 = r6; // m2 = Number of shifts

r2 = 0; // set poly index value to 0 i1 = r3; // i1 -> filter coefficients

// CC = r6 <= 0; // IF !CC JUMP pvt_positive; // if # of shifts > 0, jump over // CC = r6 < 0; // IF CC JUMP pvt_negative; // if # of shifts < 0, jump over

LSETUP(PVT_OUT_BEGIN, PVT_OUT_END) LC0 = p5; PVT_OUT_BEGIN:

m3 = i3; // save i3 into m3; A1=A0=0 || R6=[I1] || R5=[I3--]; // r6=filter coef, r5='inx' buffer // i1 += m1; LSETUP(PVT_FILTER_BEGIN, PVT_FILTER_END) LC1 = p3;

PVT_FILTER_BEGIN: R4=(A0+=R6.H*R5.H), A1+=R6.H*R5.L (M)||i1 += m1; PVT_FILTER_END: R1=(A1+=R5.H*R6.L) (M) || R6=[I1] || R5=[I3--]; i1 += m1;

// R1=R1>>16; // R4=R4+R1 (S);

r5 = m2; /******** IPDC comment *******/ // A1 = A1>>16; /******************************/

/*********IPDC addition*******/ A1=A1>>>15; /******************************/ A0+=A1; A0 = ASHIFT A0 BY r5.l; r6 = A0; // high half-word extraction with 16-bit saturation. Rounding cntrl by // RND_MOD. 0 = unbiased rounding = default [i2++] = r6; // save output into 'inx'+1

// R6.H=(A1+=R6.L*R5.H) || NOP || NOP;

// [i2++] = r4; // save output into 'inx'+1

//new_poly:

r7 = r2; // r7 = poly_index r7 = r7 + r0; // r7 = poly_index + DNR i3 = m3; // restore i3 r6 = m1; // r6 = UPR

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 20 of 26

test_address: r5 = r7 - r6; // (poly_index + DNR)-UPR CC = r5 < 0; IF CC JUMP next_address; // if true, jump over r7 = r5; // r7 = new poly_index m0 = 4; i3 += m0; // increment by 1 word (4 bytes)

JUMP test_address; // test the new poly_index next_address:

r2 = r7; // save the new address r7 = r7 + r3; // r7 -> adjusted filter address

PVT_OUT_END: i1 = r7; // i1 -> poly-phase filter

pvt_return:

r7 = i3; // update r7 -> 'inx' buffer p4 = 8; // 2 words (2*4bytes per word) [p0++p4] = r7; // save the input signal

r6 = i2; [p0] = r6; // save the output signal address

over_pivotstage:

p3.l = pt2_fundst; p3.h = pt2_fundst; p0 = [p3]; // p0 -> fundamental structure

r0 = [p0++]; // r0 = number of down stages CC = r0 <= 0;

IF CC JUMP return_src_core; // if number of down stages = 0, RTS

DOWNSTAGE_BEGIN:

p3.l = st_handle; p3.h = st_handle; p4 = [p3]; // p4 -> current stage data handle

r7 = [p4++]; p0 = r7; // p0 -> stage data

[p3] = p4; // save pointer to stage data handle

dn_src:

/******** IPDC comment *******/ // r7 = [p0++]; // r7 -> input signal 'inx' // i3 = r7; // i3 -> input signal 'inx' // b3 = r7; // b3 set for circular buffering /******************************/

/*********IPDC addition*******/ p4 = 44; r7 = [p0++p4]; // r7 -> input signal 'inx' i3 = r7; p4 = -40; r5 = [p0++p4]; b3 = r5; /******************************/

r5 = [p0++]; r5 = r5 << 2; // double the length (4 bytes per word) l3 = r5; // l3 = Size of Input Stage (SIS)

/******** IPDC comment *******/ // r6 = [p0++]; // i2 = r6; // i2 -> output signal 'inx'+1 buffer (output buffer) // b2 = r6; // b2 set for circular buffering /******************************/

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 21 of 26

r6 = [p0++]; r6 = r6 << 2; // double the output size (2 bytes per word) l2 = r6; // l2 = Size of Output Stage (SOS)

p4 = 8; // always skip over DNR (2*4bytes) in the up SRC

r3 = [p0++]; // r3 -> the filter coefficients r6 = [p0++p4]; // r6 = filter length p3 = r6;

r4 = [p0++p4]; // r4 = DNR r4 = r4 << 2; // Four bytes per word m3 = r4;

p5 = [p0++]; // p5 = number of outputs

p4 = -40; // Backup 10 words (10x4) r2 = [p0++p4]; // r2 = number of shifts

LSETUP(DN_OUT_BEGIN, DN_OUT_END) LC0 = p5;

DN_OUT_BEGIN:

i1 = r3; // i1 -> filter coefficients m1 = i3; // save i3 into m1

A1=A0=0 || R6=[I1++] || R5=[I3--]; // r6=filter coef, r5='inx' buffer LSETUP(DOWN_FILTER_BEGIN, DOWN_FILTER_END) LC1 = p3; DOWN_FILTER_BEGIN: R4=(A0+=R6.H*R5.H), A1+=R6.H*R5.L (M); DOWN_FILTER_END: R1=(A1+=R5.H*R6.L) (M) || R6=[I1++] || R5=[I3--];

// R1=R1>>16; // R4=R4+R1 (S); /******** IPDC comment *******/ // A1 = A1>>16; /******************************/

/*********IPDC addition*******/ A1=A1>>>15; /******************************/ A0+=A1; A0 = ASHIFT A0 BY r2.l; r6 = A0; // high half-word extraction with 16-bit saturation. Rounding cntrl by // RND_MOD. 0 = unbiased rounding = default [i2++] = r6; // save output into 'inx'+1 // JUMP shiftDone;

//shiftPos: // Left Shift = Up shift = positive number // A1 = ASHIFT A1 BY r2.l; // r6.h = A1; // high half-word extraction with 16-bit saturation. Rounding cntrl by // RND_MOD. 0 = unbiased rounding = default

// w[i2++] = r6.h; // save output into 'inx'+1

//shiftDone: i3 = m1; // restore i3

DN_OUT_END: i3 += m3; // increment by 4 bytes per word

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 22 of 26

r7 = i3;

p4 = 8; // 2 words (2*4bytes per word) [p0++p4] = r7; // save the input signal address

r6 = i2; [p0] = r6; // save the output signal address

DOWNSTAGE_END:

r0 += -1; // Check number of downstages CC = r0 <= 0; IF !CC JUMP DOWNSTAGE_BEGIN; // if # equal to 0, jump to DOWNSTAGE_BEGIN

return_src_core:

#ifdef BUFIN

p3.l = diff_offset; // p3 -> Offset difference to strip leading zeroes off of final buffer p3.h = diff_offset; r7 = w[p3]; // r7 = number of outputs samples per block // r7 = 147; p5 = r7; // p5 = number of outputs samples per block (OSPB)

r7 = r7 << 2; // 4 bytes per word. m2 = r7; i2 -= m2; // modify i2 = i2-#OSPB (backup pointer by output block size)

i3 = p2; // i3 -> 'outputData' l3 = 0; // non-circular

LSETUP(READ_OUTS_BEGIN, READ_OUTS_END) LC0 = p5;

READ_OUTS_BEGIN: r6 = [i2++]; // get 32-bit output from buffer

READ_OUTS_END: w[i3++] = r6.h; // write 16-bit output to 'outputData'

// p2 = i3; // save i3 into p2

// JUMP go_back; // jump if more inputs

#endif

RETURN_TO_SENDER:

(R7:4,P5:3)=[SP++]; // Pop R7 ...P5 L0=0; L1=0; L2=0; L3=0;

RTS;

_src_flt.end:

Listing 3. Src_flt.asm

initial.asm

/* ------------------------------------------------------------------------

File: initial.asm

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 23 of 26

Sample Rate Conversion Version 0.1

P0 -> fundamental structure

Registers used: P0, P1, P2, P5, R2, R3, R4, R5, R6, R7

------------------------------------------------------------------------ */

.GLOBAL _src_init;

.SECTION program;

/* initialize all the buffers (inputs and delay) P0 -> a fundamental structure */

_src_init: [--SP]=(R7:4,P5:3); // Push R7 and

P0 = R0; // Address of fundemental structure p5 = 20; // 5*4 bytes = 20 byte-wide increment r6 = [p0++p5]; // Pointer to fundemental structure 'fs_x' post increment of 5 32-bit words //jws p1 = r2; // p1 = 32-bit pointer 'st_handle'

r7 = [p0++]; // load number of stages p5 = r7; p1 = r6; // p1 = 32-bit pointer 'st_handle'

LSETUP(L_BEGIN, L_END) LC0 = p5; L_BEGIN: r2 = [p1++];

p2 = r2; // p2 -> 'datax' r3 = [p2++]; // r3 -> first element 'inx' r4 = [p2++]; // r4 = length 'SZINx'

i0 = r3; // i0 -> 'inx' buffer p5 = r4; r5 = 0; l0 = 0; // l0 = length of 'inx' buffer SZINx LSETUP(SET_ZERO_BEGIN, SET_ZERO_END) LC1 = p5; SET_ZERO_BEGIN: SET_ZERO_END: [i0++] = r5; // zero out a 32-bit word

L_END: nop;

_src_init_end1: (R7:4,P5:3)=[SP++]; // Pop R7 and P5 RTS;

Listing 4. initial.asm

src_441to48.h

//Include file for 44.1KHz to 48KHz. Greatest Common Denominator (GCD) = 147/160.

#define HALFB 0 // Half band flag #define NUPST 2 // Number of up stages #define PVTFL 1 // Pivot flag #define NDWNS 0 // Number of down stages #define STAGE 3 // Number of total stages #define NINPS 147 // Number of imput samples (Should be a even multiple of the GCD) #define NOUTS 160 // Number of output samples (Should be the same multiple as above of the GCD)

#define INTP1 2

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 24 of 26

#define DOWN1 1 #define LENG1 509 // LENG1 = length of stage filter #define PLEN1 255 // PLEN1 = MLEN1/INTP1 (polyphase length) #define MLEN1 510 // MLEN1 = LENG1 + enough to make even length for polyphase #define SHFT1 0 #define NINP1 NINPS // NINPS (...or NOUTS) = 160 #define SZIN1 (NINP1 + ((LENG1-1)/INTP1) + 1) // 160 + 48/147 + 1 = 161

#define INTP2 5 #define DOWN2 1 #define LENG2 61 // LENG2 = length of stage filter #define PLEN2 13 // PLEN2 = MLEN2/INTP2 (polyphase length) #define MLEN2 65 // MLEN2 = LENG2 + enough to make even length for polyphase #define SHFT2 1 #define NINP2 ((NINP1*INTP1)/DOWN1) // (NINPx*INTPx)/DOWNx = 160*147/16 = 1470 #define SZIN2 (NINP2 + ((LENG2-1)/INTP2) + 1) // 1470 + 26/1 + 1 = 1497

#define INTP3 16 #define DOWN3 147 #define LENG3 113 // LENG3 = length of stage filter #define PLEN3 8 // PLEN2 = MLEN2/INTP2 (polyphase length) #define MLEN3 128 // MLEN2 = LENG2 + enough to make even length for polyphase #define SHFT3 0 #define NINP3 ((NINP2*INTP2)/DOWN2) // (NINPx*INTPx)/DOWNx 1470*1/5 = 294 #define SZIN3 (NINP3 + ((LENG3-1)/INTP3) + 1) // 294 + 222/1 + 1 = 517

#define NINP4 ((NINP3*INTP3)/DOWN3) // (NINPx*INTPx)/DOWNx = 294*1/2 = 147 #define SZIN4 NINP4 + 1 // for last decimation stage only = 148

#define OFFS1 (LENG1-1)/(2*DOWN1) // #define OFFS2 (LENG2-1)/(2*DOWN2) // #define OFFS3 (LENG3-1)/(2*DOWN3) //

#if OFFS3 < 1 #define OF2S3 1 #else #define OF2S3 OFFS3 #endif

#define TOFS1 OFFS1 // #define TOFS2 ((INTP2*TOFS1)/DOWN2 + OFFS2) // #define TOFS3 ((INTP3*TOFS2)/DOWN3 + OF2S3) //

/*********IPDC comment *******/ //#define DOFS3 (NOUTS-TOFS3) // Used to strip filter delays off buffers /******************************/

/*********IPDC addition*******/ #define DOFS3 NOUTS /******************************/

Listing 5. src_441to48.h

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 25 of 26

References

1. Advanced QED Series Sample Rate Conversion System (Windows 95/NT) Version 2.2, Momentum Data Systems,

1994-1998.

2. Digital Signal Processing, Chapter10, Proakis and Manolakis.

3. Discrete-Time signal Processing, A.V. Oppenheim and R. W. Schäfer, 1989

4. Multirate Systems and Filter Banks, P.P. Vaidyanathan, 1993.

5. MATLAB®, V5.3 or later, MathWorks

6. VisualDSP++ 3.1 or later, Analog Devices Inc.

Document History

Revision Description

Rev 4 – March 18, 2004

by Jeff Sondermeyer

October 28, 2003

by Jeff Sondermeyer

June 05, 2003

by Jeff Sondermeyer

January 20, 2003

by Jeff Sondermeyer

Problem in the base register initialization. Now runs with RT data. Also runs under VisualDSP++ 3.5

Corrected bug: A1=A1>>16 should be A1=A1>>>15

Updated according to new naming conventions. Ported example code from VisualDSP++ 3.0 to VisualDSP++ 3.1

Initial Release

Rational Sample Rate Conversion with Blackfin® Processors (EE-183) Page 26 of 26

Analog Devices EE183v04 Application Notes

Specifications and Main Features

Frequently Asked Questions

User Manual

Introduction

Decimation

1.1 Sample Rate Conversion Designs

Interpolation

Sample rate changes using both interpolation and decimation

1.2 Decimation

1.3 Interpolation

1.4 Sample Rate Conversion by Rational Factor L/M

Figure 1. Block Diagram of a Rational SRC

1.5 Polyphase Filters

1.5.1 Interpolator-Only Polyphase Filters

1.5.2 Decimator-Only Polyphase Filters

1.6 Polyphase, Multistage Filter Design

1.7 SRC Code Overview

Figure 2. Audio SRC Matrix

Figure 5. FFT of 'x' Input Data at 250Hz

Figure 6. FFT of 'y' Output Data at 250Hz

1.7.1 Input/Output Data Sizes and the GCD

Table 1. Greatest Common Denominator for Audio SRC

1.7.2 Coefficient Generation and Formatting

Listing 1. Coefficient Format from MDS

1.7.3 BUFIN Define

1.7.4 Zeroing Filter Delays

1.7.5 Reducing Intermediate Buffers

1.7.6 Restrictions

1.7.7 Unresolved Issues

Conclusions

1.7.8 Case Study of Total SNR

Recommendations for Further Development

Appendix

SRC.c

Listing 2. SRC.c

Src_flt.asm

Listing 3. Src_flt.asm

initial.asm

Listing 4. initial.asm

src_441to48.h

Listing 5. src_441to48.h

References

Document History